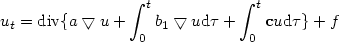A Numerical Approximation Structured by Mixed Finite Element and Upwind Fractional Step Difference for Semiconductor Device with Heat Conduction and Its Numerical Analysis
- Part of
-
- Journal:
- Numerical Mathematics: Theory, Methods and Applications / Volume 10 / Issue 3 / August 2017
- Published online by Cambridge University Press:
- 20 June 2017, pp. 541-561
- Print publication:
- August 2017
-
- Article
- Export citation
Two-Grid Method for Miscible Displacement Problem by Mixed Finite Element Methods and Mixed Finite Element Method of Characteristics
- Part of
-
- Journal:
- Communications in Computational Physics / Volume 19 / Issue 5 / May 2016
- Published online by Cambridge University Press:
- 17 May 2016, pp. 1503-1528
- Print publication:
- May 2016
-
- Article
- Export citation
Error estimates for Stokes problem with Tresca frictionconditions
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 48 / Issue 5 / September 2014
- Published online by Cambridge University Press:
- 13 August 2014, pp. 1413-1429
- Print publication:
- September 2014
-
- Article
- Export citation
A Posteriori Error Estimates of a Combined Mixed Finite Element and Discontinuous Galerkin Method for a Kind of Compressible Miscible Displacement Problems
-
- Journal:
- Advances in Applied Mathematics and Mechanics / Volume 5 / Issue 2 / April 2013
- Published online by Cambridge University Press:
- 03 June 2015, pp. 163-179
- Print publication:
- April 2013
-
- Article
- Export citation
Pseudostress-Based Mixed Finite Element Methods for the Stokes Problem in ℝn with Dirichlet Boundary Conditions. I: A Priori Error Analysis
-
- Journal:
- Communications in Computational Physics / Volume 12 / Issue 1 / July 2012
- Published online by Cambridge University Press:
- 20 August 2015, pp. 109-134
- Print publication:
- July 2012
-
- Article
- Export citation
A multiscale mortar multipoint flux mixed finite element method
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 46 / Issue 4 / July 2012
- Published online by Cambridge University Press:
- 03 February 2012, pp. 759-796
- Print publication:
- July 2012
-
- Article
- Export citation
A mixed finite element method for Darcy flow in fracturedporous media with non-matching grids∗
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 46 / Issue 2 / March 2012
- Published online by Cambridge University Press:
- 19 December 2011, pp. 465-489
- Print publication:
- March 2012
-
- Article
- Export citation
Extrapolation of Mixed Finite Element Approximations for the Maxwell Eigenvalue Problem
-
- Journal:
- Numerical Mathematics: Theory, Methods and Applications / Volume 4 / Issue 3 / August 2011
- Published online by Cambridge University Press:
- 28 May 2015, pp. 379-395
- Print publication:
- August 2011
-
- Article
- Export citation
Superconvergence of Mixed Methods for Optimal Control Problems Governed by Parabolic Equations
-
- Journal:
- Advances in Applied Mathematics and Mechanics / Volume 3 / Issue 4 / August 2011
- Published online by Cambridge University Press:
- 03 June 2015, pp. 401-419
- Print publication:
- August 2011
-
- Article
- Export citation
Mixed approximation of eigenvalue problems:A superconvergence result
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 43 / Issue 5 / September 2009
- Published online by Cambridge University Press:
- 08 April 2009, pp. 853-865
- Print publication:
- September 2009
-
- Article
- Export citation
A residual based A POSTERIORI error estimator for an augmented mixed finite element methodin linear elasticity
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 40 / Issue 5 / September 2006
- Published online by Cambridge University Press:
- 16 January 2007, pp. 843-869
- Print publication:
- September 2006
-
- Article
- Export citation
Multicomponent flow in a porous medium. Adsorption and Soret effect phenomena: local study and upscaling process
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 35 / Issue 3 / May 2001
- Published online by Cambridge University Press:
- 15 April 2002, pp. 481-512
- Print publication:
- May 2001
-
- Article
- Export citation
An upwinding mixed finite element method for a mean field model of superconducting vortices
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 34 / Issue 3 / May 2000
- Published online by Cambridge University Press:
- 15 April 2002, pp. 687-706
- Print publication:
- May 2000
-
- Article
- Export citation
L∞(L2) and L∞(L∞) error estimates for mixed methodsfor integro-differential equations of parabolic type
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 33 / Issue 3 / May 1999
- Published online by Cambridge University Press:
- 15 August 2002, pp. 531-546
- Print publication:
- May 1999
-
- Article
- Export citation
Multi-parameter asymptotic error resolution of the mixed finiteelement method for the Stokes problem
-
- Journal:
- ESAIM: Mathematical Modelling and Numerical Analysis / Volume 33 / Issue 1 / January 1999
- Published online by Cambridge University Press:
- 15 August 2002, pp. 89-97
- Print publication:
- January 1999
-
- Article
- Export citation

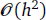 to
to 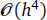 when applying the lowest order Nédélec mixed finite element and a nonconforming mixed finite element. To our best knowledge, this is the first superconvergence result of the Maxwell eigenvalue problem by the extrapolation of the mixed finite element approximation. Numerical experiments are provided to demonstrate the theoretical results.
when applying the lowest order Nédélec mixed finite element and a nonconforming mixed finite element. To our best knowledge, this is the first superconvergence result of the Maxwell eigenvalue problem by the extrapolation of the mixed finite element approximation. Numerical experiments are provided to demonstrate the theoretical results.