Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-18T22:17:03.276Z Has data issue: false hasContentIssue false
  • English
  • Français

THE TREE PROPERTY AT 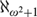${\aleph _{{\omega ^2} + 1}}$ AND 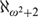${\aleph _{{\omega ^2} + 2}}$

Published online by Cambridge University Press:  01 August 2018

DIMA SINAPOVA  and
SPENCER UNGER
DIMA SINAPOVA
Affiliation:
DEPARTMENT OF MATHEMATICS, STATISTICS, AND COMPUTER SCIENCE UNIVERSITY OF ILLINOIS AT CHICAGO CHICAGO, IL60607-7045, USAE-mail:sinapova@math.uic.edu
SPENCER UNGER
Affiliation:
SCHOOL OF MATHEMATICAL SCIENCES TEL AVIV UNIVERSITY TEL AVIV69978, ISRAELE-mail:sunger@math.ucla.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We show that from large cardinals it is consistent to have the tree property simultaneously at ${\aleph _{{\omega ^2} + 1}}$ and ${\aleph _{{\omega ^2} + 2}}$ with 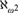${\aleph _{{\omega ^2}}}$ strong limit.

Keywords

03E0503E3503E55tree propertyPrikry forcingsingular cardinals hypothesis
Type
Articles
Information
The Journal of Symbolic Logic , Volume 83 , Issue 2 , June 2018 , pp. 669 - 682
Copyright
Copyright © The Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abraham, U., Aronszajn trees on ${\aleph _2}$ and ${\aleph _3}$. Annals of Pure and Applied Logic, vol. 24 (1983), no. 3, pp. 213–230.CrossRefGoogle Scholar
Cox, S. and Krueger, J., Quotients of strongly proper forcings and guessing models, this Journal, vol. 81 (2016), no. 1, pp. 264–283.Google Scholar
Cummings, J. and Foreman, M., The tree property. Advances in Mathematics, vol. 133 (1998), no. 1, pp. 1–32.CrossRefGoogle Scholar
Friedman, S.-D. and Halilović, A., The tree property at the double successor of a measurable cardinal κ with 2k large. Fundamenta Mathematicae, vol. 223 (2013), no. 1, pp. 55–64.CrossRefGoogle Scholar
Friedman, S.-D., Honzik, R., and Stejskalová, S., The tree property at the double successor of a singular with a larger gap. Preprint, 2015. Available at logika.ff.cuni.cz/sarka/papers/Friedman_Honzik_Stejskalova_A-tree-gap.pdf.Google Scholar
Gitik, M. and Sharon, A., On SCH and the approachability property. Proceedings of the American Mathematical Society, vol. 136 (2008), no. 1, pp. 311–320.CrossRefGoogle Scholar
König, D., Sur les correspondence multivoques des ensembles. Fundamenta Mathematicae, vol. 8 (1926), pp. 114–134.CrossRefGoogle Scholar
Kurepa, D., Ensembles ordonnés et ramifiés. Publications de l’Institut Mathématique, vol. 4 (1935), pp. 1–138.Google Scholar
Magidor, M. and Shelah, S., The tree property at successors of singular cardinals. Archive for Mathematical Logic, vol. 35 (1996), no. 5–6, pp. 385–404.CrossRefGoogle Scholar
Mitchell, W., Aronszajn trees and the independence of the transfer property. Annals of Pure and Applied Logic, 5 (1972/73), pp. 21–46.Google Scholar
Neeman, I., Aronszajn trees and failure of the singular cardinal hypothesis. Journal of Mathematical Logic, vol. 9 (2009), no. 1, pp. 139–157.CrossRefGoogle Scholar
Neeman, I., The tree property up to ${\aleph _{\omega + 1}}$, this Journal, vol. 79 (2014), no. 2, pp. 429–459.Google Scholar
Sinapova, D., The tree property and the failure of the singular cardinal hypothesis at ${\aleph _{{\omega ^2}}}$, this Journal, vol. 77 (2012), no. 3, pp. 934–946.Google Scholar
Sinapova, D., The tree property at the first and double successors of a singular. Israel Journal of Mathematics, vol. 216 (2016), no. 2, pp. 799–810.CrossRefGoogle Scholar
Specker, E., Sur un problème de Sikorski. Colloquium Mathematicum, vol. 2 (1949), pp. 9–12.CrossRefGoogle Scholar
Unger, S., Aronszajn trees and the successors of a singular cardinal. Archive for Mathematical Logic, vol. 52 (2013), no. 5–6, pp. 483–496.CrossRefGoogle Scholar
Specker, E., Fragility and indestructibility II. Annals of Pure and Applied Logic, vol. 166 (2015), no. 11, pp. 1110–1122.Google Scholar
Specker, E., The tree property below ${\aleph _{\omega \cdot 2}}$. Annals of Pure and Applied Logic, vol. 167 (2016), no. 3, pp. 247–261.Google Scholar