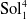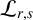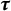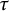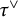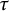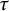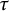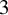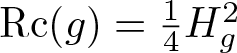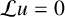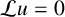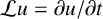459 results in 53Cxx
Codazzi and totally umbilical hypersurfaces in
 $\mathrm {Sol}_1^4$
$\mathrm {Sol}_1^4$
- Part of
-
- Journal:
- Glasgow Mathematical Journal , First View
- Published online by Cambridge University Press:
- 03 April 2025, pp. 1-7
-
- Article
- Export citation
DISTANCE FUNCTIONS ON CONVEX BODIES AND SYMPLECTIC TORIC MANIFOLDS
- Part of
-
- Journal:
- Nagoya Mathematical Journal , First View
- Published online by Cambridge University Press:
- 02 April 2025, pp. 1-23
-
- Article
- Export citation
GAUSSIAN HOLOMORPHIC SECTIONS ON NONCOMPACT COMPLEX MANIFOLDS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu , First View
- Published online by Cambridge University Press:
- 12 March 2025, pp. 1-66
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
COMPLETE HYPERSURFACES WITH LINEARLY RELATED HIGHER ORDER MEAN CURVATURES IN THE HYPERBOLIC SPACE
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 10 March 2025, pp. 1-24
-
- Article
- Export citation
BOTT MANIFOLDS WITH VANISHING FUTAKI INVARIANTS FOR ALL KAHLER CLASSES
- Part of
-
- Journal:
- Nagoya Mathematical Journal , First View
- Published online by Cambridge University Press:
- 24 February 2025, pp. 1-20
-
- Article
- Export citation
Anisotropic weighted isoperimetric inequalities for star-shaped and F-mean convex hypersurface
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 10 February 2025, pp. 1-17
-
- Article
- Export citation
ON MARCH’S CRITERION FOR TRANSIENCE ON ROTATIONALLY SYMMETRIC MANIFOLDS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 10 February 2025, pp. 1-11
-
- Article
- Export citation
Free torus actions and twisted suspensions
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 13 / 2025
- Published online by Cambridge University Press:
- 20 January 2025, e3
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
A Bonnet–Myers rigidity theorem for globally hyperbolic Lorentzian length spaces
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 13 January 2025, pp. 1-42
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On infinitesimal
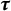 $\boldsymbol { \tau }$-isospectrality of locally symmetric spaces
$\boldsymbol { \tau }$-isospectrality of locally symmetric spaces
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 68 / Issue 1 / March 2025
- Published online by Cambridge University Press:
- 09 January 2025, pp. 246-261
- Print publication:
- March 2025
-
- Article
- Export citation
New classes of quasigeodesic Anosov flows in
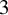 $3$-manifolds
$3$-manifolds
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems , First View
- Published online by Cambridge University Press:
- 23 December 2024, pp. 1-37
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
An isoperimetric type inequality in De Sitter space
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 12 December 2024, pp. 1-20
-
- Article
-
- You have access
- HTML
- Export citation
The algebraic and analytic compactifications of the Hitchin moduli space
- Part of
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Generalized Ricci flow on aligned homogeneous spaces
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 68 / Issue 1 / February 2025
- Published online by Cambridge University Press:
- 26 November 2024, pp. 226-246
-
- Article
-
- You have access
- HTML
- Export citation
On Riemannian and Ricci curvatures of homogeneous Finsler manifolds
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 68 / Issue 1 / March 2025
- Published online by Cambridge University Press:
- 25 November 2024, pp. 73-90
- Print publication:
- March 2025
-
- Article
-
- You have access
- HTML
- Export citation
CYT and SKT manifolds with parallel Bismut torsion
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 18 November 2024, pp. 1-26
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
GRADIENT ESTIMATES FOR POSITIVE EIGENFUNCTIONS OF THE
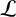 $\mathcal {L}$-OPERATOR ON CONFORMAL SOLITONS AND THEIR APPLICATIONS
$\mathcal {L}$-OPERATOR ON CONFORMAL SOLITONS AND THEIR APPLICATIONS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 111 / Issue 2 / April 2025
- Published online by Cambridge University Press:
- 18 November 2024, pp. 344-355
- Print publication:
- April 2025
-
- Article
-
- You have access
- HTML
- Export citation
Principal bundle structure of the space of metric measure spaces
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 18 November 2024, pp. 1-31
-
- Article
-
- You have access
- HTML
- Export citation
Surfaces of section for geodesic flows of closed surfaces
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems , First View
- Published online by Cambridge University Press:
- 15 November 2024, pp. 1-36
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Sharp bounds on the height of K-semistable Fano varieties I, the toric case
- Part of
-
- Journal:
- Compositio Mathematica / Volume 160 / Issue 10 / October 2024
- Published online by Cambridge University Press:
- 10 October 2024, pp. 2366-2406
- Print publication:
- October 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation