Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-01T21:28:42.475Z Has data issue: false hasContentIssue false
  • English
  • Français

Modern Dynamical Systems Theory

Published online by Cambridge University Press:  14 August 2015

L. Markus
L. Markus*
Affiliation:
University of Minnesota, Minneapolis, Minn. 55455, U.S.A. University of Warwick, Coventry, Warwickshire CV 4 7AU, U.K.

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In order to analyse generic or typical properties of dynamical systems we consider the space 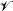of all C1-vector fields on a fixed differentiable manifold M. In the C1-metric, assuming M is compact, 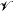is a complete metric space and a generic subset is an open dense subset or an intersection of a countable collection of such open dense subsets of 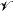. Some generic properties (i.e. specifying generic subsets) in are described. For instance, generic dynamic systems have isolated critical points and periodic orbits each of which is hyperbolic. If M is a symplectic manifold we can introduce the space 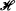of all Hamiltonian systems and study corresponding generic properties.

Type
Research Article
Information
Symposium - International Astronomical Union , Volume 62: The Stability of the Solar System , 1974 , pp. 11 - 18
Copyright
Copyright © Reidel 1974 

References

Markus, L.: 1971, Lectures in Differentiable Dynamics , Regional Conference Series in Mathematics No. 3.CrossRefGoogle Scholar
Markus, L. and Meyer, K.: 1974, Mem. Am. Math. Soc. (in press).Google Scholar
Robinson, R. C.: 1971, Lectures on Hamiltonian Systems , Instituto de Matematica Pura e Aplicado, Rio de Janeiro, Brazil.Google Scholar
Siegel, C. L. and Moser, J.: 1971, Lectures on Celestial Mechanics , Springer Verlag, New York.CrossRefGoogle Scholar
Smale, S.: 1967, Bull. Am. Math. Soc. 73, 747.CrossRefGoogle Scholar