Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-18T04:29:51.217Z Has data issue: false hasContentIssue false
  • English
  • Français

On a Set of Conform-Invariant Equations of the Gravitational Field

Published online by Cambridge University Press:  20 January 2009

H. A. Buchdahl
H. A. Buchdahl
Affiliation:
Department of Physics, University of Tasmania.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Eddington has considered equations of the gravitational field in empty space which are of the fourth differential order, viz. the sets of equations which express the vanishing of the Hamiltonian derivatives of certain fundamental invariants. The author has shown that a wide class of such equations are satisfied by any solution of the equations

where Gμν and gμν are the components of the Ricci tensor and the metrical tensor respectively, whilst λ is an arbitrary constant. For a V4 this applies in particular when the invariant referred to above is chosen from the set

where Bμνσρ is the covariant curvature tensor. K3 has been included since, according to a result due to Lanczos3, its Hamiltonian derivative 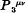is a linear combination of 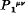and , i.e. of the Hamiltonian derivatives of K1 and K2. In fact

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 10 , Issue 1 , January 1953 , pp. 16 - 20
Copyright
Copyright © Edinburgh Mathematical Society 1953

References

REFERENCES

1.Eddington, A. S., The Mathematical Theory of Relaticity (2nd ed., Cambridge, 1930), §62, 141.Google Scholar
2.Buchdahl, H. A., Proc. Nat. Acad., 38 (1948), 66.CrossRefGoogle Scholar
3.Lanczos, C., Annals of Math. (2) 39 (1938), 842.CrossRefGoogle Scholar
4.Gregory, C., Phys. Rev., 72 (1947), 72.CrossRefGoogle Scholar
5.Eisenhart, L. P., Riemannian Geometry (Princeton, 1926), Ch. II, 90.Google Scholar
6.Schouten, J. A., Der Ricci-Kalkiil (Berlin, 1924), 25 and 31.Google Scholar
7.Buchdahl, H. A., Oxford Quart. J. Math., 19 (1948), 150.CrossRefGoogle Scholar
8.Eddington, A. S., Reference 1, §45, 100.Google Scholar
9.Eddington, A. S., Reference 1, §61, 140.Google Scholar
10.Weyl, H., Space, Time, Matter (London, 1922), §§35–36.Google Scholar
11.Weyl, H., Math. Ztschr., 2 (1918), 404.CrossRefGoogle Scholar
12.Bergmann, P. G., Introduction to the Theory of Relativity (New York, 1946), Ch. XVI, 253.Google Scholar