Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-17T21:29:21.312Z Has data issue: false hasContentIssue false
  • English
  • Français

Paracompactness and Strong Screenability

Published online by Cambridge University Press:  22 January 2016

Keiô Nagami
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

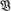Throughout this paper, a space means a T1-space. A space is called fully normal if every open covering of it has a Δ-refinement , that is, an open covering for which the stars (x, ) form a covering which refines 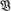. A space is called paracompact if every open covering 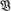of it has a locally finite (= neighborhood finite) open covering which refines 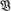. It is well known that paracompactness is identical with full normality in a Hausdorff space ([3], [7]).

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 8 , February 1955 , pp. 83 - 88
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1955

References

[1] Bing, R. H.: Metrization of topological spaces, Canadian J, Math. 3 (1951) 175–186.CrossRefGoogle Scholar
[2] Cohen, H. J.: Sur un problème de M. Dieudonné, C. R. Acd. Sci. Paris 234 (1952) 290–292.Google Scholar
[3] Dieudonné, J.: Une généralisation des espaces compacts, J. Math. Pures Appl. 23 (1944) 65–76.Google Scholar
[4] Dowker, C. H.: On countably paracompact spaces, Canadian J. Math. 3 (1951) 219–224.CrossRefGoogle Scholar
[5] Nagami, K.: Baire sets, Borel sets and some typical semi-continuous functions, Nagoya Math. J, 7 (1954), 85–93.CrossRefGoogle Scholar
[6] Lefschetz, S.: Algebraic topology, New York (1942).Google Scholar
[7] Stone, A. H.: Paracompactness and product spaces, Bull. Amer. Math. Soc. 54 (1948) 977–982.CrossRefGoogle Scholar