Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-18T10:28:25.643Z Has data issue: false hasContentIssue false
  • English
  • Français

On the thermodynamics of SPARC plasma and its role in reducing uncertainties on the way to large fusion gain

Published online by Cambridge University Press:  30 June 2022

Andrea Di Vita Open the ORCID record for Andrea Di Vita [Opens in a new window]
Andrea Di Vita*
Affiliation:
DICCA, Università di Genova, via Montallegro 1, 16145 Genova, Italy
*
†Email address for correspondence: andrea.divita.1@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

Independent assessment of the feasibility of controlled nuclear fusion in the proposed SPARC tokamak (Creely et al., J. Plasma Phys., vol. 86, 2020, 865860502; Rodriguez-Fernandez et al., J. Plasma Phys., vol. 86, 2020, 865860503) is difficult because of the uncertainties concerning energy transport in the plasma. We discuss a SPARC scenario – where a burning plasma is obtained – with the help of a well-known general constraint on transport in weakly collisional, axisymmetric, toroidal, low-$\beta$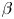 turbulent plasma (Rogister et al., Phys. Fluids B, vol. 4, 1992, p. 804). This constraint is useful in reducing uncertainties on auxiliary heating as the fusion gain begins to be large. No particular ad hoc model for transport coefficients is invoked. The crucial roles of both suitable tuning of ion cyclotron radiofrequency power and high-temperature pedestal are highlighted.

Keywords

fusion plasmaplasma confinement
Type
Research Article
Information
Journal of Plasma Physics , Volume 88 , Issue 3 , June 2022 , 905880318
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Balescu, R. 1991 Is Onsager symmetry relevant in the transport equations for magnetically confined plasmas? Phys. Fluids B 3, 564–579.CrossRefGoogle Scholar
Bombarda, F., Coppi, B., Airoldi, A., Cenacchi, G., Detragiache, P. & the Ignitor Project Group. 2004 Ignitor: physics and progress towards ignition. Braz. J. Phys. 34 (4B), 1786–1791.CrossRefGoogle Scholar
Brear, M.J., Nicoud, F., Talei, M., Giauque, A. & Hawkes, E.R. 2012 Disturbance energy transport and sound production in gaseous combustion. J. Fluid Mech. 707, 53.CrossRefGoogle Scholar
Brusati, M. & Di Vita, A. 1993 Thermodynamic stability of a tokamak plasma. J. Plasma Phys. 50 (2), 201–230.CrossRefGoogle Scholar
Bruzzone, P., Fietz, W.H., Minervini, J.V., Novikov, M., Yanagi, N., Zhai, Y. & Zheng, J. 2018 High temperature superconductors for fusion magnets. Nucl. Fusion 58, 103001.CrossRefGoogle Scholar
Chandler, D. 2021 MIT-designed project achieves major advance toward fusion energy. https://news.mit.edu/2021/MIT-CFS-major-advance-toward-fusion-energy-0908.Google Scholar
Coppi, B., Airoldi, A., Bombarda, F., Cenacchi, G., Detragiache, P. & Sugiyama, L.E. 2001 Optimal regimes for ignition and the Ignitor experiment. Nucl. Fusion 41, 1253–1257.CrossRefGoogle Scholar
Creely, A.J., Greenwald, M.J., Ballinger, S.B., Brunner, D., Canik, J., Doody, J., Fülöp, T., Garnier, D.T., Granetz, R., Gray, T.K., et al. 2020 Overview of the SPARC tokamak. J. Plasma Phys. 86, 865860502.CrossRefGoogle Scholar
DeGroot, S.R. & Mazur, P. 1962 Non-Equilibrium Thermodynamics. North Holland.Google Scholar
Di Vita, A. 1991 Magnetic fluctuations and kinetic equations in a two-species plasma. J. Plasma Phys. 46 (3), 423–436.CrossRefGoogle Scholar
Di Vita, A. 2010 Maximum or minimum entropy production? How to select a necessary criterion of stability for a dissipative fluid or plasma. Phys. Rev. E 81, 041137.CrossRefGoogle ScholarPubMed
Di Vita, A. 2010 On the thermodynamics of IGNITOR plasma. Nucl. Fusion 50, 115001.CrossRefGoogle Scholar
Di Vita, A. & Brusati, M. 1995 Minimum entropy production principle due to ohmic dissipation in tokamaks and determination of non-inductive current density profiles on JET. Plasma Phys. Control. Fusion 37, 1075–1094.CrossRefGoogle Scholar
Glansdorff, P. & Prigogine, I. 1964 On a general evolution criterion in macroscopic physics. Physica 30 (2), 351–374.CrossRefGoogle Scholar
Guazzotto, L. & Betti, R. 2019 Two-fluid burning-plasma analysis for magnetic confinement fusion devices. Plasma Phys. Control. Fusion 61 (8), 085028.CrossRefGoogle Scholar
Hinton, F.L. & Hazeltine, R.D. 1976 Theory of plasma transport in toroidal confineraient systems. Rev. Mod. Phys. 48 (2I), 239–308.CrossRefGoogle Scholar
Landau, L.D. & Lifshitz, E.M. 1959 Statistical Physics. Pergamon.Google Scholar
Lin, Y., Wright, J.C. & Wukitch, S.J. 2020 Physics basis for the ICRF system of the SPARC tokamak. J. Plasma Phys. 86, 865860506.CrossRefGoogle Scholar
Mayoral, M.L., Buttery, R., Jones, T.T.C., Kiptily, V., Sharapov, S., Mantsinen, M.J., Coda, S., Sauter, O., Eriksson, L.-G., Nguyen, F., et al. 2004 Studies of burning plasma physics in the Joint European Torus. Phys. Plasmas 11, 2607–2615.CrossRefGoogle Scholar
Navratil, G.A. 2001 Introduction to burning plasma physics. In 43rd American Physical Society Division of Plasma Physics Annual Meeting, Long Beach, Vol. 1. http://fire.pppl.gov/aps_dpp_tutorialr_gon.pdf.Google Scholar
Pegoraro, F. 1994 Implications of fusion plasma studies to other collective nonequilibrium systems. Fusion Technol. 26 (4), 1243–1249.CrossRefGoogle Scholar
Rodriguez-Fernandez, P., Howard, N.T., Greenwald, M.J., Creely, A.J., Hughes, J.W., Wright, J.C., Holland, C., Lin, Y., Sciortino, F. & the SPARC team 2020 Predictions of core plasma performance for the SPARC tokamak. J. Plasma Phys. 86, 865860503.CrossRefGoogle Scholar
Rogister, A. & Li, D. 1992 Kinetic and transport theories of turbulent, axisymmetric, low-collisionality plasmas and turbulence constraints. Phys. Fluids B 4 (4), 804–830.CrossRefGoogle Scholar
Scott, S.D., Kramer, G.J., Tolman, E.A., Snicker, A., Varje, J., Särkimäki, K., Wright, J.C. & Rodriguez-Fernandez, P. 2020 Fast-ion physics in SPARC. J. Plasma Phys. 86, 865860508.CrossRefGoogle Scholar
Shimada, M., Campbell, D.J., Mukhovatov, V., Fujiwara, M., Kirneva, N., Lackner, K., Nagami, M., Pustovitov, V.D., Uckan, N. & Wesley, J. 2007 Progress in the ITER physics basis. Chapter 1: overview and summary. Nucl. Fusion 47 (6), S1–S17.CrossRefGoogle Scholar
Sorbom, B.N., Ball, J., Palmer, T.R., Mangiarotti, F.J., Sierchio, J.M., Bonoli, P., Kasten, C., Sutherland, D.A., Barnard, H.S., Haakonsen, C.B., et al. 2015 ARC: a compact, high-field, fusion nuclear science facility and demonstration power plant with demountable magnets. Fusion Engineering Des. 100, 378–405.CrossRefGoogle Scholar
Sweeney, R., Creely, A.J., Doody, J., Fülöp, T., Garnier, D.T., Granetz, R., Greenwald, M., Hesslow, L., Irby, J., Izzo, V.A., et al. 2020 MHD stability and disruptions in the SPARC tokamak. J. Plasma Phys. 86, 865860507.CrossRefGoogle Scholar
Tolman, E.A., Loureiro, N.F., Rodrigues, P., Hughes, J.W. & Marmar, E.S. 2019 Dependence of alpha-particle-driven Alfvén eigenmode linear stability on device magnetic field strength and consequences for next-generation tokamaks. Nucl. Fusion 59, 046020.CrossRefGoogle Scholar