Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-18T16:40:38.546Z Has data issue: false hasContentIssue false
  • English
  • Français

On annihilation of the relativistic electron vortex pair in collisionless plasmas

Published online by Cambridge University Press:  26 November 2018

K. V. Lezhnin ,
F. F. Kamenets ,
T. Zh. Esirkepov  and
S. V. Bulanov
K. V. Lezhnin*
Affiliation:
Department of Astrophysical Sciences, Princeton University, Princeton, NJ 08544, USA National Research Nuclear University MEPhI, Kashirskoe sh. 31, 115409, Moscow, Russia
F. F. Kamenets
Affiliation:
Moscow Institute of Physics and Technology, Institutskiy per. 9, Dolgoprudny, Moscow Region 141700, Russia
T. Zh. Esirkepov
Affiliation:
National Institutes for Quantum and Radiological Sciences and Technology, 8-1-7 Umemidai, Kizugawa, Kyoto 619-0215, Japan
S. V. Bulanov
Affiliation:
National Institutes for Quantum and Radiological Sciences and Technology, 8-1-7 Umemidai, Kizugawa, Kyoto 619-0215, Japan Institute of Physics of the Czech Academy of Sciences v.v.i. (FZU), Na Slovance 1999/2, 18221, Prague, Czech Republic Prokhorov General Physics Institute of the Russian Academy of Sciences, 119991 Moscow, Russia
*
†Email address for correspondence: klezhnin@princeton.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

In contrast to hydrodynamic vortices, vortices in a plasma contain an electric current circulating around the centre of the vortex, which generates a magnetic field localized inside. Using computer simulations, we demonstrate that the magnetic field associated with the vortex gives rise to a mechanism of dissipation of the vortex pair in a collisionless plasma, leading to fast annihilation of the magnetic field with its energy transforming into the energy of fast electrons, secondary vortices and plasma waves. Two major contributors to the energy damping of a double vortex system, namely, magnetic field annihilation and secondary vortex formation, are regulated by the size of the vortex with respect to the electron skin depth, which scales with the electron $\unicode[STIX]{x1D6FE}$ factor, 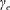$\unicode[STIX]{x1D6FE}_{e}$, as 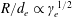$R/d_{e}\propto \unicode[STIX]{x1D6FE}_{e}^{1/2}$. Magnetic field annihilation appears to be dominant in mildly relativistic vortices, while for the ultrarelativistic case, secondary vortex formation is the main channel for damping of the initial double vortex system.

Keywords

plasma nonlinear phenomenaplasma simulationplasma waves
Type
Research Article
Information
Journal of Plasma Physics , Volume 84 , Issue 6 , December 2018 , 905840610
Copyright
© Cambridge University Press 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bulanov, S. S., Bychenkov, V. Y., Chvykov, V., Kalinchenko, G., Litzenberg, D. W., Matsuoka, T., Thomas, A. G. R., Willingale, L., Yanovsky, V., Krushelnick, K. et al. 2010 Generation of gev protons from 1 pw laser interaction with near critical density targets. Phys. Plasmas 17 (4), 043105.Google Scholar
Bulanov, S. S., Esirkepov, T. Z., Kamenets, F. F. & Pegoraro, F. 2006 Single-cycle high-intensity electromagnetic pulse generation in the interaction of a plasma wakefield with regular nonlinear structures. Phys. Rev. E 73, 036408.Google Scholar
Bulanov, S. V., Esirkepov, T. Zh., Lontano, M. & Pegoraro, F. 1997 The stability of single and double vortex films in the framework of the Hasegawa–Mima equation. Plasma Phys. Rep. 23, 660.Google Scholar
Bulanov, S. V., Lontano, M., Esirkepov, T. Zh., Pegoraro, F. & Pukhov, A. M. 1996 Electron vortices produced by ultra intense laser pulses. Phys. Rev. Lett. 76, 3562.Google Scholar
Califano, F., Pegoraro, F. & Bulanov, S. V. 1997 Spatial structure and time evolution of the weibel instability in collisionless inhomogeneous plasmas. Phys. Rev. E 56, 963–969.Google Scholar
Ertel, H. 1942 Ein neuer hydrodynamischer Wirbelsatz. Meteorol. Z. 59, 277.Google Scholar
Esirkepov, T., Bulanov, S. V., Nishihara, K. & Tajima, T. 2004 Soliton synchrotron afterglow in a laser plasma. Phys. Rev. Lett. 92, 255001.Google Scholar
Esirkepov, T. Zh. 2001 Exact charge conservation scheme for particle-in-cell simulation with an arbitrary form-factor. Comput. Phys. Commun. 135, 144.Google Scholar
Fukuda, Y., Faenov, A. Ya., Tampo, M., Pikuz, T. A., Nakamura, T., Kando, M., Hayashi, Y., Yogo, A., Sakaki, H., Kameshima, T. et al. 2009 Energy increase in multi-mev ion acceleration in the interaction of a short pulse laser with a cluster-gas target. Phys. Rev. Lett. 103, 165002.Google Scholar
Gordeev, A. V. 2010 Nonquasineutral current equilibria as elementary structures of plasma dynamics. Plasma Phys. Rep. 36, 30.Google Scholar
Gu, Y. J., Klimo, O., Kumar, D., Liu, Y., Singh, S. K., Esirkepov, T. Zh., Bulanov, S. V., Weber, S. & Korn, G. 2016 Numerical studies of barotropic modons. Dyn. Atmos. Oceans 93, 013203.Google Scholar
Hasegawa, A. & Mima, K. 1978 Pseudothreedimensional turbulence in magnetized nonuniform plasma. Phys. Fluids 21, 87.Google Scholar
Hide, R. 1983 The magnetic analogue of Ertels potential vorticity theorem. Ann. Geophys. 1, 59.Google Scholar
Hobson, D. D. 1991 A point vortex dipole model of an isolated modon. Phys. Fluids B 2 (3), 3027.Google Scholar
Kazimura, Y., Sakai, J. I., Neubert, T. & Bulanov, S. V. 1998 Generation of a small-scale quasi-static magnetic field and fast particles during the collision of electron–positron plasma clouds. Astrophys. J. Lett. 498, 183–186.Google Scholar
Kono, M. & Horton, W. 1991 Point vortex description of drift wave vortices: dynamics and transport. Phys. Fluids B 3, 3255.Google Scholar
Krasheninnikov, S. I. 2016 On the origin of plasma density blobs. Phys. Lett. A 380, 3905.Google Scholar
Kuvshinov, B. N. & Schep, T. J. 2016 The stability of single and double vortex films in the framework of the Hasegawa–Mima equation. Plasma Phys. Rep. 42, 523.Google Scholar
Larichev, V. D. & Reznik, G. M. 1976 Two-dimensional solitary Rossby waves. Dokl. Akad. Sci. SSSR 231, 12.Google Scholar
Lezhnin, K. V., Kamenets, F. F., Esirkepov, T. Zh., Bulanov, S. V., Gu, Y. J., Weber, S. & Korn, G. 2016 Explosion of relativistic electron vortices in laser plasmas. Phys. Plasmas 23, 093116.Google Scholar
Lezhnin, K. V., Kniazev, A. R., Soloviev, S. V., Kamenets, F. F., Weber, S. A., Korn, G., Esirkepov, T. Zh. & Bulanov, S. V. 2017 Evolution of relativistic electron vortices in laser plasmas. Proc. SPIE 10241, Y–1.Google Scholar
McWilliams, J. C., Flierl, G. R., Larichev, V. D. & Reznik, G. M. 1981 Numerical studies of barotropic modons. Dyn. Atmos. Oceans 5, 219.Google Scholar
Nakamura, T., Bulanov, S. V., Esirkepov, T. Zh. & Kando, M. 2010 High-energy ions from near-critical density plasmas via magnetic vortex acceleration. Phys. Rev. Lett. 105, 135002.Google Scholar
Nakamura, T. & Mima, K. 2008 Magnetic-dipole vortex generation by propagation of ultraintense and ultrashort laser pulses in moderate-density plasmas. Phys. Rev. Lett. 100, 205006.Google Scholar
Naseri, N., Bochkarev, S. G., Ruan, P., Bychenkov, V. Y., Khudik, V. & Shvets, G. 2018 Growth and propagation of self-generated magnetic dipole vortices in collisionless shocks produced by interpenetrating plasmas. Phys. Plasmas 25, 012118.Google Scholar
Naumova, N. M., Koga, J., Nakajima, K., Tajima, T., Esirkepov, T. Zh., Bulanov, S. V. & Pegoraro, F. 2001 Polarization, hosing and long time evolution of relativistic laser pulses. Phys. Plasmas 8, 4149.Google Scholar
Nettel, S. 2009 Wave Physics: Oscillations – Solitons – Chaos. Springer.Google Scholar
Nycander, J. & Isichenko, M. B. 1990 Motion of dipole vortices in a weakly inhomogeneous medium and related convective transport. Phys. Fluids B 2 (2), 2042.Google Scholar
Overman, E. A. II & Zabusky, N. J. 1982 Evolution and merger of isolated vortex structures. Phys. Fluids 25 (8), 1297–1305.Google Scholar
Pegoraro, F. 2018 Lorentz invariant ‘potential magnetic field’ and magnetic flux conservation in an ideal relativistic plasma. J. Plasma Phys. 84, 725840403.Google Scholar
Ridgers, C. P., Kirk, J. G., Duclous, R., Blackburn, T. G., Brady, C. S., Bennett, K., Arber, T. D. & Bell, A. R. 2014 Modelling gamma-ray photon emission and pair production in high-intensity lasermatter interactions. J. Comput. Phys. 260, 273.Google Scholar
Romagnani, L., Bigongiari, A., Kar, S., Bulanov, S. V., Cecchetti, C. A., Galimberti, M., Esirkepov, T. Zh., Jung, R., Liseykina, T. V., Macchi, A. et al. 2010 Experimental observation of organized magnetic vortices in laser–plasma channels. Phys. Rev. Lett. 105, 175002.Google Scholar
Saffman, P. G. 1993 Vortex Dynamics. Cambridge University Press.Google Scholar
Sylla, F., Flacco, A., Kahaly, S., Veltcheva, M., Lifschitz, A., Sanchez-Arriaga, G., Lefebvre, E. & Malka, V. 2012 Anticorrelation between ion acceleration and nonlinear coherent structures from laser-underdense plasma interaction. Phys. Rev. Lett. 108, 115003.Google Scholar
Wang, Y. Y., Li, F. Y., Chen, M., Weng, S. M., Lu, Q. M., Dong, Q. L., Sheng, Z. M. & Zhang, J. 2016 Magnetic field annihilation and reconnection driven by femtosecond lasers in inhomogeneous plasma. Sci. China Phys. Mech. Astron. 93, 013203.Google Scholar
Wei, M. S., Beg, F. N., Clark, E. L., Dangor, A. E., Evans, R. G., Gopal, A., Ledingham, K. W. D., McKenna, P., Norreys, P. A., Tatarakis, M. et al. 2004 Observations of the filamentation of high-intensity laser-produced electron beams. Phys. Rev. E 70, 056412.Google Scholar
Yi, L., Pukhov, A., Shen, B., Pusztai, I & Fülöp, T.2018 Proton acceleration in a laser-induced relativistic electron vortex. arXiv:1808.04749.Google Scholar
Zhang, Y. & Krasheninnikov, S. I. 2016 Blobs in the framework of drift wave dynamics. Phys. Plasmas 23, 124501.Google Scholar