Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-17T21:19:45.021Z Has data issue: false hasContentIssue false
  • English
  • Français

Two-dimensional oscillation of convection roll in a finite liquid metal layer under a horizontal magnetic field

Published online by Cambridge University Press:  25 January 2021

Y. Tasaka Open the ORCID record for Y. Tasaka [Opens in a new window] ,
T. Yanagisawa Open the ORCID record for T. Yanagisawa [Opens in a new window] ,
K. Fujita ,
T. Miyagoshi Open the ORCID record for T. Miyagoshi [Opens in a new window]  and
A. Sakuraba
Y. Tasaka*
Affiliation:
Laboratory for Flow Control, Faculty of Engineering, Hokkaido University, Sapporo060-8628, Japan
T. Yanagisawa
Affiliation:
Laboratory for Flow Control, Faculty of Engineering, Hokkaido University, Sapporo060-8628, Japan Japan Agency for Marine–Earth Science and Technology (JAMSTEC), Yokosuka237-0061, Japan
K. Fujita
Affiliation:
Laboratory for Flow Control, Faculty of Engineering, Hokkaido University, Sapporo060-8628, Japan
T. Miyagoshi
Affiliation:
Japan Agency for Marine–Earth Science and Technology (JAMSTEC), Yokosuka237-0061, Japan
A. Sakuraba
Affiliation:
Department of Earth and Planetary Science, University of Tokyo, Tokyo113-0033, Japan
*
†Email address for correspondence: tasaka@eng.hokudai.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

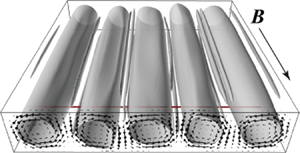
We investigate the two-dimensional (2-D) oscillation of quasi-2-D convection rolls in a liquid metal layer confined by a vessel of aspect ratio five with an imposed horizontal magnetic field. Laboratory experiments were performed in the range of Rayleigh $Ra$ and Chandrasekhar $Q$ numbers of $7.9 \times 10^4 \le Ra \le 1.8 \times 10^5$ and $2.5 \times 10^4 \le Q \le 1.9 \times 10^5$ by decreasing $Q$ at set $Ra$-number intervals to elucidate the features and mechanisms of oscillatory convection. Ultrasonic velocity profile measurements and supplemental numerical simulations show that the 2-D oscillations are caused by oscillations of recirculation vortex pairs between the main rolls, which are intensified by periodic vorticity entrainment from the vortex pair by the main rolls. The investigations also suggest that the oscillations occur at sufficiently large Reynolds $Re$ numbers to induce instabilities on the vortex pair. The $Re$ number is smaller for larger $Q/Ra$ in the 2-D oscillation regime and the variations can be approximated by the effective $Ra$ number; namely, the value reduced by the critical value for the onset of convection depending on $Q$. The variations steepen with further large $Q/Ra$ and approach a scaling law of the velocity reduction as $(Ra/Q)^{1/2}$, which is established assuming that viscous dissipation is dominated by Hartmann braking at the walls perpendicular to the magnetic field. The results suggest that these phenomena are organized by the relationship between buoyancy and magnetic damping due to Hartmann braking.

JFM classification

MHD and Electrohydrodynamics: Magneto convection Instability: Absolute/convective instability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 911 , 25 March 2021 , A19
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.CrossRefGoogle Scholar
Bodenschatz, E., Pesch, W. & Ahlers, G. 2000 Recent developments in Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 32, 709–778.CrossRefGoogle Scholar
Burr, U. & Müller, U. 2002 Rayleigh–Bénard convection in liquid metal layers under the influence of a horizontal magnetic field. J. Fluid Mech. 453, 345–369.CrossRefGoogle Scholar
Busse, F.H. 1978 Non-linear properties of thermal convection. Rep. Prog. Phys. 41, 1929–1967.CrossRefGoogle Scholar
Busse, F.H. & Clever, R.M. 1983 Stability of convection rolls in the presence of a horizontal magnetic field. J. Méc. Théor. Appl. 2, 495–502.Google Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Oxford University Press.Google Scholar
Clever, R.M. & Busse, F.H. 1987 Nonlinear oscillatory convection. J. Fluid Mech. 176, 403–417.CrossRefGoogle Scholar
Davidson, P.A. 1995 Magnetic damping of jets and vortices. J. Fluid Mech. 299, 153–186.CrossRefGoogle Scholar
Davidson, P.A. 2017 Introduction to Magnetohydrodynamics, 2nd edn. Cambridge University Press.Google Scholar
Drazin, P.G. & Reid, W.H. 1998 Hydrodynamic Stability. Cambridge University Press.Google Scholar
Eckert, S., Cramer, A. & Gerbeth, G. 2007 Velocity measurement techniques for liquid metal flows. In Magnetohydrodynamics (ed. S. Molokov, R. Moreau & H.K. Moffatt), pp. 275–294. Springer.CrossRefGoogle Scholar
Knaepen, B. & Moreau, R. 2008 Magnetohydrodynamic turbulence at low magnetic Reynolds number. Annu. Rev. Fluid Mech. 4, 25–45.CrossRefGoogle Scholar
Koschmieder, E.L. 1993 Bénard Cells and Taylor Vortices. Cambridge University Press.Google Scholar
Krishnamurti, R. & Howard, L.N. 1981 Large-scale flow generation in turbulent convection. Proc. Natl Acad. Sci. USA 78, 1981–1985.CrossRefGoogle ScholarPubMed
Lappa, M. 2010 Thermal Convection: Patters, Evolution and Stability. Wiley.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.CrossRefGoogle Scholar
Mashiko, T., Tsuji, Y., Mizuno, T. & Sano, M. 2004 Instantaneous measurement of velocity fields in developed thermal turbulence in mercury. Phys. Rev. E 69, 036306.CrossRefGoogle ScholarPubMed
Moreau, R., Thess, A. & Tsinober, A. 2007 MHD turbulence at low magnetic Reynolds number: present understanding and future needs. In Magnetohydrodynamics (ed. S. Molokov, R. Moreau & H.K. Moffatt), pp. 231–246. Springer.CrossRefGoogle Scholar
Morley, N.B., Burris, J., Cadwallader, L.C. & Nornberg, M.D. 2008 GaInSn usage in the research laboratory. Rev. Sci. Instrum. 79, 056107.CrossRefGoogle ScholarPubMed
Plevachuk, Y., Sklyarchuk, V., Eckert, S., Gerbeth, G. & Novakovic, R. 2014 Thermophysical properties of the liquid Ga-In-Sn eutectic alloy. J. Chem. Engng Data 59, 757–763.CrossRefGoogle Scholar
Sommeria, J. & Moreau, R. 1982 Why, how, and when, MHD turbulence becomes two-dimensional. J. Fluid Mech. 118, 507–518.CrossRefGoogle Scholar
Takeda, Y. 2012 Ultrasonic doppler velocity profiler for fluid flow. Fluid Mechanics and Its Applications, vol. 101. Springer Science & Business Media.CrossRefGoogle Scholar
Tasaka, Y., Igaki, K., Yanagisawa, T., Vogt, T., Zuerner, T. & Eckert, S. 2016 Regular flow reversals in Rayleigh–Bénard convection in a horizontal magnetic field. Phys. Rev. E 93, 043109.CrossRefGoogle Scholar
Tsuji, Y., Mizuno, T., Mashiko, T. & Sano, M. 2005 Mean wind in convective turbulence of mercury. Phys. Rev. Lett. 94, 034501.CrossRefGoogle ScholarPubMed
Vogt, T., Ishimi, W., Yanagisawa, T., Tasaka, Y., Sakuraba, A. & Eckert, S. 2018 Transition between quasi-two-dimensional and three-dimensional Rayleigh–Bénard convection in a horizontal magnetic field. Phys. Rev. Fluids 3, 013503.CrossRefGoogle Scholar
Yanagisawa, T., Hamano, Y., Miyagoshi, T., Yamagishi, Y., Tasaka, Y. & Takeda, Y. 2013 Convection patterns in a liquid metal under an imposed horizontal magnetic field. Phys. Rev. E 88, 063020.CrossRefGoogle Scholar
Yanagisawa, T., Hamano, Y. & Sakuraba, A. 2015 Flow reversals in low-Prandtl-number Rayleigh–Bénard convection controlled by horizontal circulations. Phys. Rev. E 92, 023018.CrossRefGoogle ScholarPubMed
Yanagisawa, T., Yamagishi, Y., Hamano, Y., Tasaka, Y. & Takeda, Y. 2011 Spontaneous flow reversals in Rayleigh–Bénard convection of a liquid gallium. Phys. Rev. E 83, 036307.CrossRefGoogle Scholar
Yanagisawa, T., Yamagishi, Y., Hamano, Y., Tasaka, Y., Yoshida, M., Yano, K. & Takeda, Y. 2010 Structure of large-scale flows and their oscillation in the thermal convection of liquid metal. Phys. Rev. E 82, 016320.CrossRefGoogle Scholar

Tasaka et al. supplementary movie 1

See word file for movie caption

Download Tasaka et al. supplementary movie 1(Video)
Video 4.1 MB

Tasaka et al. supplementary movie 2

See word file for movie caption

Download Tasaka et al. supplementary movie 2(Video)
Video 750.2 KB
Supplementary material: File

Tasaka et al. supplementary material

Captions for movies 1-2

Download Tasaka et al. supplementary material(File)
File 12.8 KB