Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-19T09:09:22.146Z Has data issue: false hasContentIssue false
  • English
  • Français

Conformal trace theorem for Julia sets of quadratic polynomials

Published online by Cambridge University Press:  04 December 2017

A. CONNES ,
E. MCDONALD ,
F. SUKOCHEV  and
D. ZANIN
A. CONNES
Affiliation:
Collège de France, IHES, 3, rue d’Ulm, 75231 Paris cedex 05, France email alain@connes.org
E. MCDONALD
Affiliation:
School of Mathematics and Statistics, University of New South Wales, Sydney, NSW 2052, Australia email edward.mcdonald@unsw.edu.au, f.sukochev@unsw.edu.au, d.zanin@unsw.edu.au
F. SUKOCHEV
Affiliation:
School of Mathematics and Statistics, University of New South Wales, Sydney, NSW 2052, Australia email edward.mcdonald@unsw.edu.au, f.sukochev@unsw.edu.au, d.zanin@unsw.edu.au
D. ZANIN
Affiliation:
School of Mathematics and Statistics, University of New South Wales, Sydney, NSW 2052, Australia email edward.mcdonald@unsw.edu.au, f.sukochev@unsw.edu.au, d.zanin@unsw.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

If $c$ is in the main cardioid of the Mandelbrot set, then the Julia set $J$ of the map 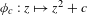$\unicode[STIX]{x1D719}_{c}:z\mapsto z^{2}+c$ is a Jordan curve of Hausdorff dimension 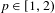$p\in [1,2)$. We provide a full proof of a formula for the Hausdorff measure on $J$ in terms of singular traces announced by the first named author in 1996.

Type
Original Article
Information
Ergodic Theory and Dynamical Systems , Volume 39 , Issue 9 , September 2019 , pp. 2481 - 2506
Copyright
© Cambridge University Press, 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Carleson, L. and Gamelin, T. W.. Complex Dynamics (Universitext: Tracts in Mathematics) . Springer, New York, 1993.Google Scholar
Connes, A.. Noncommutative Geometry. Academic Press, San Diego, CA, 1994.Google Scholar
Connes, A.. Brisure de symétrie spontanée et géométrie du point de vue spectral. Séminaire Bourbaki, Vol. 1995/96 (Astérisque, 241, Exp. No. 816, 5) . Société de Mathématique de France, Paris, 1997, pp. 313–349.Google Scholar
Connes, A.. Noncommutative differential geometry and the structure of space time. Deformation Theory and Symplectic Geometry (Ascona, 1996) (Mathematical Physics Studies, 20) . Kluwer Academic, Dordrecht, 1997, pp. 1–33.Google Scholar
Connes, A., Sukochev, F. and Zanin, D.. Trace theorem for quasi-Fuchsian groups. Mat. Sb. 208(10) (2017), 59–90 (in Russian).Google Scholar
Douady, A. and Hubbard, J. H.. Étude dynamique des polynômes complexes. Partie I (Publications Mathématiques d’Orsay [Mathematical Publications of Orsay], 84) . Université de Paris-Sud, Département de Mathématiques, Orsay, 1984.Google Scholar
Falconer, K.. Fractal Geometry: Mathematical Foundations and Applications, 2nd edn. John Wiley, Hoboken, NJ, 2003.Google Scholar
Federer, H.. Geometric Measure Theory (Grundlehren der mathematischen Wissenschaften, 153) . Springer, New York, 1969.Google Scholar
Garnett, J. B. and Marshall, D. E.. Harmonic Measure (New Mathematical Monographs, 2) . Cambridge University Press, Cambridge, 2005.Google Scholar
Hedenmalm, H., Korenblum, B. and Zhu, K.. Theory of Bergman Spaces (Graduate Texts in Mathematics, 199) . Springer, New York, 2000.Google Scholar
Lord, S., Sukochev, F. and Zanin, D.. Singular Traces: Theory and Applications (De Gruyter Studies in Mathematics, 46) . De Gruyter, Berlin, 2013.Google Scholar
Milnor, J.. Dynamics in One Complex Variable (Annals of Mathematics Studies, 160) , 3rd edn. Princeton University Press, Princeton, NJ, 2006.Google Scholar
Peller, V. V.. Hankel Operators and Their Applications (Springer Monographs in Mathematics) . Springer, New York, 2003.Google Scholar
Rudin, W.. Real and Complex Analysis, 3rd edn. McGraw-Hill, New York, 1987.Google Scholar
Semenov, E., Sukochev, F., Usachev, A. and Zanin, D.. Banach limits and traces on L1, ∞ . Adv. Math. 285 (2015), 568–628.Google Scholar
Stein, E. M.. Singular Integrals and Differentiability Properties of Functions (Princeton Mathematical Series, 30) . Princeton University Press, Princeton, NJ, 1970.Google Scholar
Sullivan, D.. Conformal dynamical systems. Geometric Dynamics (Rio de Janeiro, 1981) (Lecture Notes in Mathematics, 1007) . Springer, Berlin, 1983, pp. 725–752.Google Scholar
Urbański, M.. Measures and dimensions in conformal dynamics. Bull. Amer. Math. Soc. (N.S.) 40(3) (2003), 281–321.Google Scholar
Zhu, K. H.. Analytic Besov spaces. J. Math. Anal. Appl. 157(2) (1991), 318–336.Google Scholar