Hostname: page-component-76fb5796d-dfsvx Total loading time: 0 Render date: 2024-04-25T09:02:58.192Z Has data issue: false hasContentIssue false
  • English
  • Français

On the number of conjugacy classes of the sylow p-subgroups of GL(n,q)

Published online by Cambridge University Press:  17 April 2009

Antonio Vera-López ,
J.M. Arregi  and
F.J. Vera-López
Antonio Vera-López
Affiliation:
Departamento de MatemáticasFacultad de CienciasUniversidad del País VascoBilbaoSpain, e-mail: mtpveloa@lg.ehu.es, mtparlij@lg.ehu.es
J.M. Arregi
Affiliation:
Departamento de MatemáticasFacultad de CienciasUniversidad del País VascoBilbaoSpain, e-mail: mtpveloa@lg.ehu.es, mtparlij@lg.ehu.es
F.J. Vera-López
Affiliation:
Departamento de MatemáticaFacultad de InformaticaCampus de EspinardoMurciaSpain, e-mail: pacovera@fcu.um.es
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

If G is a finite p-group of order pn, P. Hall determined the number of conjugacy classes of G, r(G), modulo (p2 − 1)(p − 1). Namely, he proved the existence of a constant k ≥ 0 such that r(G) = n(p2 − 1) + pe + k(p2 − 1)(p − 1). In this paper, we denote by 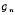the group of the upper unitriangular matrices over 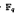, the finite field with q = pt elements, and we determine the number of classes of 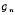modulo (q − 1)5.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 52 , Issue 3 , December 1995 , pp. 431 - 439
Copyright
Copyright © Australian Mathematical Society 1995

References

[1]Vera-López, A. and Arregi, J.M., ‘Conjugacy classes in Sylow p-subgroups of GL(n, q)’, J. Algebra 152 (1992), 1–19.CrossRefGoogle Scholar
[2]Vera-López, A. and Arregi, J.M., ‘Some algorithms for calculating conjugacy classes in Sylow's p-subgroups of GL(n, q)’, J. Algebra (to appear).Google Scholar