No CrossRef data available.
Article contents
GRAPHS DETERMINED BY THEIR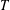 
$T$-GAIN SPECTRA
Part of:
Graph theory
Published online by Cambridge University Press: 03 July 2020
Abstract
An undirected graph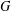 
$G$ is determined by its 
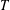$T$-gain spectrum (DTS) if every 
$T$-gain graph cospectral to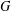 
$G$ is switching equivalent to 
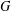$G$. We show that the complete graph 
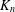$K_{n}$ and the graph 
$K_{n}-e$ obtained by deleting an edge from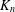 
$K_{n}$ are DTS, the star 
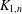$K_{1,n}$ is DTS if and only if 
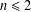$n\leq 2$, and an odd path 
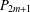$P_{2m+1}$ is not DTS if 
$m\geq 2$. We give an operation for constructing cospectral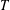 
$T$-gain graphs and apply it to show that a tree of arbitrary order (at least 
$5$) is not DTS.
MSC classification
Secondary:
05C22: Signed and weighted graphs
- Type
- Research Article
- Information
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
Footnotes
The first author is supported by the National Natural Science Foundation of China (No.11971474). The third author is supported by the Natural Science Foundation of Shandong Province (No. ZR2019BA016).
References
Collatz, L. and Sinogowitz, U., ‘Spektren endlicher Grafen’, Abh. Math. Semin. Univ. Hambg.
21 (1957), 63–77.Google Scholar
van Dam, E. R. and Haemers, W. H., ‘Which graphs are determined by their spectrum?’, Special Issue on the Combinatorial Matrix Theory Conference, Pohang, 2002’, Linear Algebra Appl.
373 (2003), 241–272.Google Scholar
van Dam, E. R. and Haemers, W. H., ‘Developments on spectral characterizations of graphs’, Discrete Math.
309 (2009), 576–586.Google Scholar
Godsil, C. D. and McKay, B. D., ‘Constructing cospectral graphs’, Aequationes Math.
25 (1982), 257–268.Google Scholar
Günthard, H. H. and Primas, H., ‘Zusammenhang von Graphentheorie und MO-Theorie von Molekelnmit Systemen konjugierter Bindungen’, Helv. Chim. Acta
39 (1956), 1645–1653.Google Scholar
Lu, Y., Wang, L. G. and Xiao, P., ‘Complex unit gain bicyclic graphs with rank 2, 3 or 4’, Linear Algebra Appl.
523 (2017), 169–186.Google Scholar
Lu, Y., Wang, L. G. and Zhou, Q., ‘The rank of a complex unit gain graph in terms of the rank of its underlying graph’, J. Comb. Optim.
38 (2019), 570–588.Google Scholar
McKay, B. D., ‘On the spectral characterisation of trees’, Ars Combin.
3 (1977), 219–232.Google Scholar
Reff, N., ‘Spectral properties of complex unit gain graphs’, Linear Algebra Appl.
436 (2012), 3165–3176.Google Scholar
Schwenk, A. J., ‘Almost all trees are cospectral’, in: New Directions in the Theory of Graphs, Proceedings of the 3rd Ann Arbor Conference, University of Michigan, Ann Arbor, Michigan, 1971 (Academic Press, New York, 1973), 275–307.Google Scholar
Wang, Y., Gong, S. C. and Fan, Y. Z., ‘On the determinant of the Laplacian matrix of a complex unit gain graph’, Discrete Math.
341 (2018), 81–86.Google Scholar
Yu, G. H., Qu, H. and Tu, J. H., ‘Inertia of complex unit gain graphs’, Appl. Math. Comput.
265 (2015), 619–629.Google Scholar
Zaslavsky, T., ‘Biased graphs. I. Bias, balance and gains’, J. Combin. Theory Ser. B
47 (1989), 32–52.Google Scholar