Book contents
- Frontmatter
- Contents
- Introduction
- Algorithms for matrix groups
- Residual properties of 1-relator groups
- Words and groups
- The modular isomorphism problem for the groups of order 512
- Recent progress in the symmetric generation of groups
- Discriminating groups: a comprehensive overview
- Extending the Kegel Wielandt theorem through π-decomposable groups
- On the prime graph of a finite group
- Applications of Lie rings with finite cyclic grading
- Pronormal subgroups and transitivity of some subgroup properties
- On Engel and positive laws
- Maximal subgroups of odd index in finite groups with simple classical socle
- Some classic and nearly classic problems on varieties of groups
- Generalizations of the Sylow theorem
- Engel groups
- Lie methods in Engel groups
- On the degree of commutativity of p-groups of maximal class
- Class preserving automorphisms of finite p-groups: a survey
- Symmetric colorings of finite groups
- References
Residual properties of 1-relator groups
Published online by Cambridge University Press: 05 July 2011
- Frontmatter
- Contents
- Introduction
- Algorithms for matrix groups
- Residual properties of 1-relator groups
- Words and groups
- The modular isomorphism problem for the groups of order 512
- Recent progress in the symmetric generation of groups
- Discriminating groups: a comprehensive overview
- Extending the Kegel Wielandt theorem through π-decomposable groups
- On the prime graph of a finite group
- Applications of Lie rings with finite cyclic grading
- Pronormal subgroups and transitivity of some subgroup properties
- On Engel and positive laws
- Maximal subgroups of odd index in finite groups with simple classical socle
- Some classic and nearly classic problems on varieties of groups
- Generalizations of the Sylow theorem
- Engel groups
- Lie methods in Engel groups
- On the degree of commutativity of p-groups of maximal class
- Class preserving automorphisms of finite p-groups: a survey
- Symmetric colorings of finite groups
- References
Summary
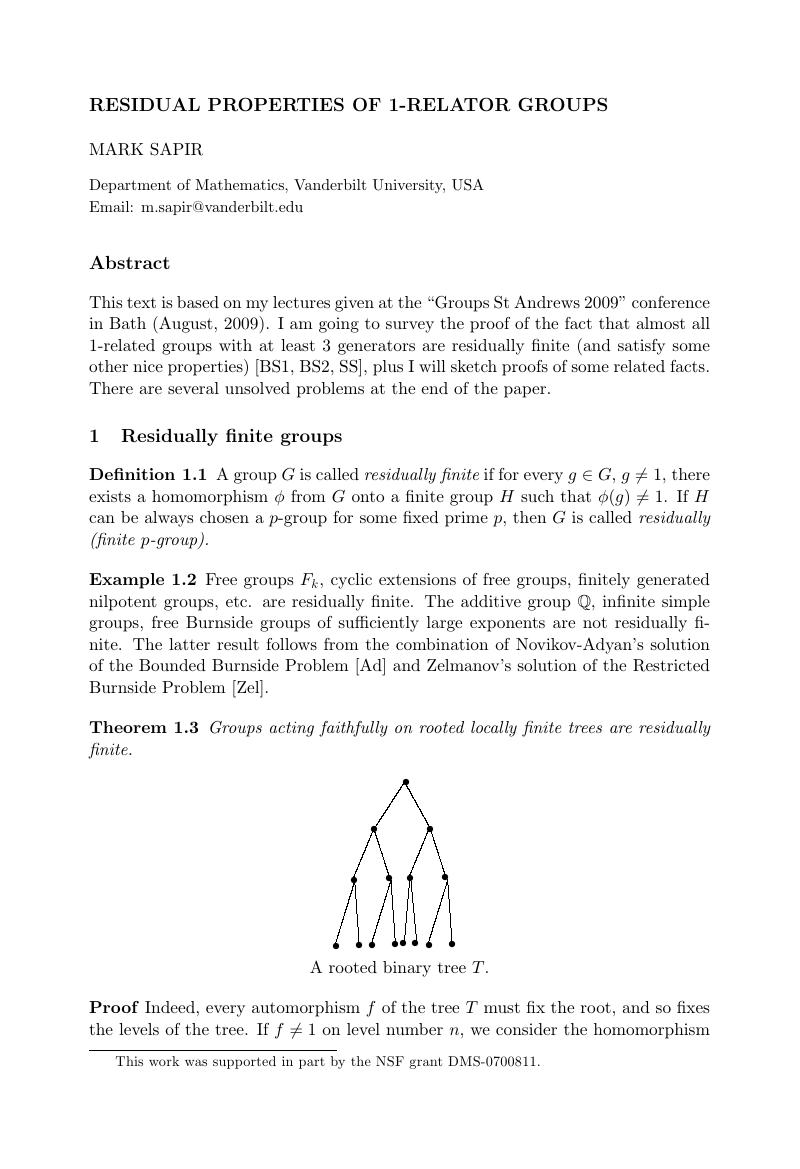
- Type
- Chapter
- Information
- Groups St Andrews 2009 in Bath , pp. 324 - 343Publisher: Cambridge University PressPrint publication year: 2011
References
- 2
- Cited by

