Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Boileau, Michel
2020.
Around 3-manifold groups.
Winter Braids Lecture Notes,
Vol. 5,
Issue. ,
p.
1.
de Jesus Nery, Genildo
2020.
Profinite genus of fundamental groups of torus bundles.
Communications in Algebra,
Vol. 48,
Issue. 4,
p.
1567.
Ueki, Jun
2021.
Profinite rigidity for twisted Alexander polynomials.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2021,
Issue. 771,
p.
171.
Chen, Haimiao
2022.
Alexander polynomial, Dijkgraaf-Witten invariant, and Seifert fibred surgery.
Topology and its Applications,
Vol. 322,
Issue. ,
p.
108303.
Liu, Yi
2023.
Finite Quotients, Arithmetic Invariants, and Hyperbolic Volume.
Peking Mathematical Journal,
Liu, Yi
2023.
Finite-volume hyperbolic 3-manifolds are almost determined by their finite quotient groups.
Inventiones mathematicae,
Vol. 231,
Issue. 2,
p.
741.
Liu, Yi
2023.
Mapping classes are almost determined by their finite quotient actions.
Duke Mathematical Journal,
Vol. 172,
Issue. 3,
Chagas, Sheila
del Rio, Ángel
and
Zalesskii, Pavel A.
2023.
Aritmethic lattices of SO(1,n) and units of group rings.
Journal of Pure and Applied Algebra,
Vol. 227,
Issue. 11,
p.
107405.
de Bessa, Vagner R.
Porto, Anderson L. P.
and
Zalesskii, Pavel A.
2023.
The profinite completion of accessible groups.
Monatshefte für Mathematik,
Vol. 202,
Issue. 2,
p.
217.
Rapoport, Paul
2024.
On the profinite distinguishability of hyperbolic
Dehn fillings of finite-volume 3–manifolds.
Algebraic & Geometric Topology,
Vol. 24,
Issue. 9,
p.
4779.
de Bessa, V.R.
Porto, A.L.P.
and
Zalesskii, P.A.
2024.
Profinite genus of free products with finite amalgamation.
Journal of Algebra,
Vol. 643,
Issue. ,
p.
11.
Morales, Ismael
2024.
Profinite properties of residually free groups.
Communications in Algebra,
p.
1.
Zalesskii, Pavel
2024.
The profinite completion of relatively hyperbolic virtually special groups.
Israel Journal of Mathematics,
Vol. 260,
Issue. 1,
p.
427.
Morales, Ismael
2024.
On the profinite rigidity of free and surface groups.
Mathematische Annalen,
Vol. 390,
Issue. 1,
p.
1507.
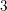 $3$-manifold determines the Kneser–Milnor decomposition. If
$3$-manifold determines the Kneser–Milnor decomposition. If  $M$ is irreducible, then the profinite completion determines the Jaco–Shalen–Johannson decomposition of
$M$ is irreducible, then the profinite completion determines the Jaco–Shalen–Johannson decomposition of  $M$.
$M$.

