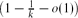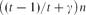Paper
Note on the Smallest Root of the Independence Polynomial
-
- Published online by Cambridge University Press:
- 18 July 2012, pp. 1-8
-
- Article
- Export citation
Hypergraph Independent Sets
-
- Published online by Cambridge University Press:
- 11 October 2012, pp. 9-20
-
- Article
- Export citation
Applications of the Semi-Definite Method to the Turán Density Problem for 3-Graphs
-
- Published online by Cambridge University Press:
- 07 December 2012, pp. 21-54
-
- Article
- Export citation
Turán and Ramsey Properties of Subcube Intersection Graphs
-
- Published online by Cambridge University Press:
- 03 October 2012, pp. 55-70
-
- Article
- Export citation
Embedding Spanning Bipartite Graphs of Small Bandwidth
-
- Published online by Cambridge University Press:
- 03 October 2012, pp. 71-96
-
- Article
- Export citation
A Multipartite Version of the Hajnal–Szemerédi Theorem for Graphs and Hypergraphs
-
- Published online by Cambridge University Press:
- 07 December 2012, pp. 97-111
-
- Article
- Export citation
The Largest Minimum Codegree of a 3-Graph Without a Generalized 4-Cycle
-
- Published online by Cambridge University Press:
- 19 October 2012, pp. 112-117
-
- Article
- Export citation
Improved Mixing Time Bounds for the Thorp Shuffle
-
- Published online by Cambridge University Press:
- 07 December 2012, pp. 118-132
-
- Article
- Export citation
Explosive Percolation in Erdős–Rényi-Like Random Graph Processes
-
- Published online by Cambridge University Press:
- 03 October 2012, pp. 133-145
-
- Article
- Export citation
Probably Intersecting Families are Not Nested
-
- Published online by Cambridge University Press:
- 09 October 2012, pp. 146-160
-
- Article
- Export citation
Front Cover (OFC, IFC) and matter
CPC volume 22 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 07 December 2012, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back Cover (IBC, OBC) and matter
CPC volume 22 issue 1 Cover and Back matter
-
- Published online by Cambridge University Press:
- 07 December 2012, pp. b1-b4
-
- Article
-
- You have access
- Export citation