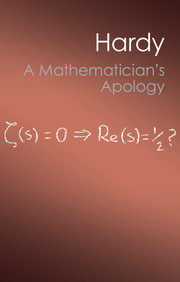15
Published online by Cambridge University Press: 05 August 2014
Summary
A ‘serious’ theorem is a theorem which contains ‘significant’ ideas, and I suppose that I ought to try to analyse a little more closely the qualities which make a mathematical idea significant. This is very difficult, and it is unlikely that any analysis which I can give will be very valuable. We can recognize a ‘significant’ idea when we see it, as we can those which occur in my two standard theorems; but this power of recognition requires a rather high degree of mathematical sophistication, and of that familiarity with mathematical ideas which comes only from many years spent in their company. So I must attempt some sort of analysis; and it should be possible to make one which, however inadequate, is sound and intelligible so far as it goes. There are two things at any rate which seem essential, a certain generality and a certain depth; but neither quality is easy to define at all precisely.
A significant mathematical idea, a serious mathematical theorem, should be ‘general’ in some such sense as this. The idea should be one which is a constituent in many mathematical constructs, which is used in the proof of theorems of many different kinds. The theorem should be one which, even if stated originally (like Pythagoras's theorem) in a quite special form, is capable of considerable extension and is typical of a whole class of theorems of its kind.
- Type
- Chapter
- Information
- A Mathematician's Apology , pp. 103 - 105Publisher: Cambridge University PressPrint publication year: 2012