Hostname: page-component-5cf477f64f-54txb Total loading time: 0 Render date: 2025-04-02T11:24:36.018Z Has data issue: false hasContentIssue false
  • English
  • Français

Pair correlation of sequences 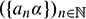$(\lbrace a_n \alpha \rbrace)_{n \in {\mathbb N}}$ with maximal additive energy

Published online by Cambridge University Press:  06 September 2018

GERHARD LARCHER  and
WOLFGANG STOCKINGER
GERHARD LARCHER
Affiliation:
Department of Financial Mathematics and Applied Number Theory, Johannes Kepler University, Linz, Altenbergerstr. 69, A-4040, Austria. e-mail: wolfgang.stockinger@jku.at, gerhard.larcher@jku.at
WOLFGANG STOCKINGER
Affiliation:
Department of Financial Mathematics and Applied Number Theory, Johannes Kepler University, Linz, Altenbergerstr. 69, A-4040, Austria. e-mail: wolfgang.stockinger@jku.at, gerhard.larcher@jku.at
Get access Rights & Permissions [Opens in a new window]

Abstract

We show for sequences 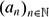$\left(a_{n}\right)_{n \in \mathbb N}$ of distinct positive integers with maximal order of additive energy, that the sequence $\left(\left\{a_{n} \alpha\right\}\right)_{n \in \mathbb N}$ does not have Poissonian pair correlations for any α. This result essentially sharpens a result obtained by J. Bourgain on this topic.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 168 , Issue 2 , March 2020 , pp. 287 - 293
Copyright
Copyright © Cambridge Philosophical Society 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Aichinger, I., Aistleitner, C. and Larcher, G.. On Quasi-Energy-Spectra, Pair Correlations of Sequences and Additive Combinatorics. Celebration of the 80th birthday of Ian Sloan (Dick, J., Kuo, F. Y., Woźniakowski, H., eds.). (Springer-Verlag, 2018).Google Scholar
[2] Aistleitner, C., Larcher, G. and Lewko, M.. Additive energy and the Hausdorff dimension of the exceptional set in metric pair correlation problems. With an appendix by Jean Bourgain. Israel J. Math. 222 (2017) No. 1, 463–485.Google Scholar
[3] Aistleitner, C., Lachmann, T. and Pausinger, F.. Pair correlations and equidistribution. J. Number Theory, to appear, available at https://arxiv.org/abs/1612.05495.Google Scholar
[4] Balog, A. and Szemerédi, E.. A statistical theorem of set addition. Combinatorica 14 (1994), 263–268.Google Scholar
[5] Freiman, G. R.. Foundations of a structural theory of set addition. Trans. Math. Monogr. 37 (Amer. Math. Soc., Providence, USA, 1973).Google Scholar
[6] Gowers, W. T.. A new proof of Szemerédi's theorem for arithmetic progressions of length four. Geom. Funct. Anal. 8 (1998), 529–551.Google Scholar
[7] Grepstad, S. and Larcher, G.. On pair correlation and discrepancy. Arch. Math. 109 (2017) 143–149.Google Scholar
[8] Lachmann, T. and Technau, N.. On exceptional sets in the metric poissonian pair correlations problem. Submitted (2017). arXiv:1708.08599.Google Scholar
[9] Larcher, G.. Remark on a result of Bourgain on Poissonian pair correlation. arXiv:1711.08663 (2017).Google Scholar
[10] Larcher, G. and Stockinger, W.. Some negative results related to Poissonian pair correlation problems. Submitted (2018). arXiv:1803.05236.Google Scholar
[11] Lev, V. F.. The (Gowers–)Balog–Szemerédi theorem: an exposition: http://people.math.gatech.edu/~ecroot/8803/baloszem.pdf.Google Scholar
[12] Marklof, J.. The Berry–Tabor conjecture. European Congress of Mathematics, Vol. II (Barcelona, 2000), 421–427. Progr. Math. 202 (Birkhäuser, Basel, 2001).Google Scholar
[13] Rudnick, Z. and Sarnak, P.. The pair correlation function of fractional parts of polynomials. Comm. Math. Phys. 194 (1) (1998), 61–70.Google Scholar
[14] Rudnick, Z., Sarnak, P. and Zaharescu, A.. The distribution of spacings between the fractional parts of n 2 α. Invent. Math. 145 (1) (2001), 37–57.Google Scholar
[15] Rudnick, Z. and Zaharescu, A.. A metric result on the pair correlation of fractional parts of sequences. Acta Arith. 89 (3) (1999), 283–293.Google Scholar
[16] Steinerberger, S.. Localized quantitative criteria for equidistribution. Acta Arith. 180 (2017), 183–199.Google Scholar
[17] Tao, T. and Vu, V.. Additive combinatorics. Camb. Stud. Adv. Math. vol. 105 (Cambridge University Press, Cambridge, 2006).Google Scholar
[18] Walker, A.. The primes are not Poissonian. arXiv:1702.07365 (2017).Google Scholar