Research Article
The Thirteenth Grade*
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 253-267
-
- Article
- Export citation
Division of Fractions: an Improved Technique
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 268-269
-
- Article
- Export citation
On Analytic Functions
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 270
-
- Article
- Export citation
Practical Experimentation in the Teaching of Basic Statistics
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 271-280
-
- Article
- Export citation
The S.H.M Equation
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 280-284
-
- Article
- Export citation
Two Properties of Integers
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 285-287
-
- Article
- Export citation
Approximation of Irrationals By Rationals
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 288-289
-
- Article
- Export citation
A Note on the Quadratic Form ax2 + 2bxy + cy2
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 290-291
-
- Article
- Export citation
Convergence of the Newton-Raphson Method For Arbitary Polynomials
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 291-295
-
- Article
- Export citation
The Theory of the Centralizer or Transverse Coupling in Electric Locomotives
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 296-304
-
- Article
- Export citation
Perspective Polygons
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 304-311
-
- Article
- Export citation
Other
Gleanings Far and Near
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 311-323
-
- Article
- Export citation
Classroom Notes
116. Heron's formula
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 312-313
-
- Article
- Export citation
117. Direct proofs of the formulae for tan (θ2 − θ1) and tan (θ2 + θ1) by geometrical methods
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 314-315
-
- Article
- Export citation
118. Linear differential equations of second order with constant coefficients (right-hand side zero)
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 315-316
-
- Article
- Export citation
119. Graphical test for a Poisson distribution
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 316
-
- Article
- Export citation
Mathematical Notes
3101. Two simple nets
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 317-318
-
- Article
- Export citation
3102. Curvature of a conic
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 318-320
-
- Article
- Export citation
3103. Extension of the Theorems of Menelaus and Ceva
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 321-322
-
- Article
- Export citation
3104. A problem in differential geometry
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 322-323
-
- Article
- Export citation

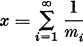 , where
, where  belongs to the set for all
belongs to the set for all  , it is then natural to denote the sides by
, it is then natural to denote the sides by 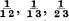 where
where 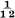 is the side containing
is the side containing 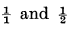 . Now bringing in the second triangle
. Now bringing in the second triangle 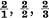 we get sides
we get sides 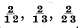 . Moreover, one pair of corresponding sides is
. Moreover, one pair of corresponding sides is 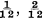 ; it is therefore natural to christen their intersection
; it is therefore natural to christen their intersection 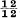 . The other two meets are
. The other two meets are 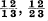 ; and, by Desargues' theorem, these three meets are collinear; we therefore christen the axis of perspective
; and, by Desargues' theorem, these three meets are collinear; we therefore christen the axis of perspective 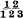 .
.