Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-17T21:14:08.076Z Has data issue: false hasContentIssue false
  • English
  • Français

Capturing Taylor–Görtler vortices in a streamwise-rotating channel at very high rotation numbers

Published online by Cambridge University Press:  22 January 2018

Zixuan Yang Open the ORCID record for Zixuan Yang [Opens in a new window]  and
Bing-Chen Wang Open the ORCID record for Bing-Chen Wang [Opens in a new window]
Zixuan Yang
Affiliation:
Department of Mechanical Engineering, University of Manitoba, Winnipeg, MB, R3T 5V6, Canada
Bing-Chen Wang*
Affiliation:
Department of Mechanical Engineering, University of Manitoba, Winnipeg, MB, R3T 5V6, Canada
*
†Email address for correspondence: BingChen.Wang@umanitoba.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

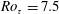In this paper, we study the scales and dynamics of Taylor–Görtler (TG) vortices in streamwise-rotating turbulent channel flows at moderate and high rotation numbers ($Ro_{\unicode[STIX]{x1D70F}}=7.5$, 15, 30, 75 and 150) with a fixed Reynolds number. In order to precisely capture TG vortices in the streamwise and spanwise directions, direct numerical simulations have been performed on 15 test cases of different domain sizes and rotation numbers. A two-layer pattern of TG vortices is identified, and the characteristic length scales of TG vortices are quantified using the premultiplied energy spectra. It is observed that as the rotation number increases, the spanwise scale of TG vortices remains stable but the streamwise scale increases rapidly. Three criteria have been used for judging a domain-size-independent solution in both physical and spectral spaces. The weakest criterion ensures accurate predictions of the first- and second-order statistical moments of the velocity, which requires a minimum streamwise domain size of 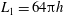$L_{1}=64\unicode[STIX]{x03C0}h$, where $h$ is one-half the channel height. However, the streamwise domain size needs to be stretched drastically to 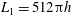$L_{1}=512\unicode[STIX]{x03C0}h$ if the most stringent criterion is considered, which demands that all energetic eddies be fully captured based on a predefined threshold value (i.e. 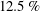$12.5\,\%$ of the peak value) of the premultiplied two-dimensional energy spectrum. The effects of streamwise system rotation on the scales and dynamics of TG vortices are investigated by comparing the statistical results of rotating and non-rotating channel flows, and through the analysis of two-point correlations, premultiplied energy spectra, and budget balance of turbulent stresses.

JFM classification

Turbulent Flows: Rotating turbulence Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 838 , 10 March 2018 , pp. 658 - 689
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

del Álamo, J. C. & Jiménez, J. 2003 Spectra of the very large anisotropic scales in turbulent channels. Phys. Fluids 15 (6), L41–L44.CrossRefGoogle Scholar
Alkishriwi, N., Meinke, M. & Schröder, M. 2008 Large-eddy simulation of streamwise-rotating turbulent channel flow. Comput. Fluids 37, 786–792.CrossRefGoogle Scholar
Avsarkisov, V., Hoyas, S., Oberlack, M. & García-Galache, J. P. 2014 Turbulent plane Couette flow at moderately high Reynolds number. J. Fluid Mech. 751, R1.CrossRefGoogle Scholar
Brethouwer, G., Schlatter, P., Duguet, Y., Henningson, D. S. & Johansson, A. V. 2014 Recurrent bursts via linear processes in turbulent environments. Phys. Rev. Lett. 112, 144502.CrossRefGoogle ScholarPubMed
Coleman, G. N., Ferziger, J. H. & Spalart, P. R. 1990 A numerical study of the turbulent Ekman layer. J. Fluid Mech. 213, 313–348.CrossRefGoogle Scholar
Deng, B.-Q., Huang, W.-X. & Xu, C.-X. 2016 Origin of effectiveness degradation in active drag reduction control of turbulent channel flow at Re 𝜏 = 1000. J. Turbul. 17 (8), 758–786.CrossRefGoogle Scholar
Deng, B.-Q. & Xu, C.-X. 2012 Influence of active control on STG-based generation of streamwise vortices in near-wall turbulence. J. Fluid Mech. 710, 234–259.CrossRefGoogle Scholar
Deng, B.-Q., Xu, C.-X., Huang, W.-X. & Cui, G.-X. 2014 Strengthened opposition control for skin-friction reduction in wall-bounded turbulent flows. J. Turbul. 15 (2), 122–143.CrossRefGoogle Scholar
Floryan, J. M. 1986 Görtler instability of boundary layers over concave and convex walls. Phys. Fluids 29, 2380–2387.CrossRefGoogle Scholar
Grundestam, O., Wallin, S. & Johansson, A. V. 2008 Direct numerical simulations of rotating turbulent channel flow. J. Fluid Mech. 598, 177–199.CrossRefGoogle Scholar
Hoyas, S. & Jiménez, J. 2006 Scaling of the velocity fluctuations in turbulent channels up to Re 𝜏 = 2003. Phys. Fluids 18 (1), 011702.CrossRefGoogle Scholar
Hoyas, S. & Jiménez, J. 2008 Reynolds number effects on the Reynolds-stress budgets in turbulent channels. Phys. Fluids 20 (10), 101511.CrossRefGoogle Scholar
Jiménez, J. & Moin, P. 1991 The minimal flow unit in near-wall turbulence. J. Fluid Mech. 225, 213–240.CrossRefGoogle Scholar
Johnston, J. P., Halleen, R. M. & Lezius, D. K. 1972 Effects of spanwise rotation on the structure of two-dimensional fully developed turbulent channel flow. J. Fluid Mech. 56, 533–557.CrossRefGoogle Scholar
Kim, J., Moin, P. & Moser, R. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.CrossRefGoogle Scholar
Kristoffersen, R. & Andersson, H. I. 1993 Direct simulations of low-Reynolds-number turbulent flow in a rotating channel. J. Fluid Mech. 256, 163–197.CrossRefGoogle Scholar
Masuda, S., Fukuda, S. & Nagata, M. 2008 Instabilities of plane Poiseuille flow with a streamwise system rotation. J. Fluid Mech. 603, 189–206.CrossRefGoogle Scholar
Moser, R. D., Kim, J. & Mansour, N. N. 1999 Direct numerical simulation of turbulent channel flow up to Re 𝜏 = 590. Phys. Fluids 11 (4), 943–945.CrossRefGoogle Scholar
Oberlack, M. 1999 Similarity in non-rotating and rotating turbulent pipe flows. J. Fluid Mech. 379, 1–22.CrossRefGoogle Scholar
Oberlack, M., Cabot, W., Reif, B. A. P. & Weller, T. 2006 Group analysis, direct numerical simulation and modelling of a turbulent channel flow with streamwise rotation. J. Fluid Mech. 562, 383–403.CrossRefGoogle Scholar
Orlandi, P. & Fatica, M. 1997 Direct simulations of turbulent flow in a pipe rotating about its axis. J. Fluid Mech. 343, 43–72.CrossRefGoogle Scholar
Recktenwald, I., Alkishriwi, N. & Schröder, W. 2009 PIV–LES analysis of channel flow rotating about the streamwise axis. Eur. J. Mech. (B Fluids) 28 (5), 677–688.CrossRefGoogle Scholar
Recktenwald, I., Weller, T., Schröder, W. & Oberlack, M. 2007 Comparison of direct numerical simulations and particle-image velocimetry data of turbulent channel flow rotating about the streamwise axis. Phys. Fluids 19 (8), 085114.CrossRefGoogle Scholar
Saric, W. S. 1994 Görtler vortices. Annu. Rev. Fluid Mech. 26, 379–409.CrossRefGoogle Scholar
Speziale, C. G. & Thangam, S. 1983 Numerical study of secondary flows and roll-cell instabilities in rotating channel flow. J. Fluid Mech. 130, 377–395.CrossRefGoogle Scholar
Tritton, D. J. 1992 Stabilization and destabilization of turbulent shear flow in a rotating fluid. J. Fluid Mech. 241, 503–523.CrossRefGoogle Scholar
Wall, D. P. & Nagata, M. 2006 Nonlinear secondary flow through a rotating channel. J. Fluid Mech. 564, 25–55.CrossRefGoogle Scholar
Weller, T. & Oberlack, M. 2006a DNS of a turbulent channel flow with streamwise rotation – investigation on the cross flow phenomena. In Direct and Large-Eddy Simulation VI (ed. Lamballais, E., Friedrich, R., Geurts, B. J. & Métais, O.), pp. 241–248. Springer.CrossRefGoogle Scholar
Weller, T. & Oberlack, M. 2006b DNS of a turbulent channel flow with streamwise rotation – study of the reverse effect of the cross flow. Proc. Appl. Maths. Mech. 6, 553–554.CrossRefGoogle Scholar
Wu, H. & Kasagi, N. 2004 Effects of arbitrary directional system rotation on turbulent channel flow. Phys. Fluids 16 (4), 979–990.CrossRefGoogle Scholar
Yang, Y.-T., Su, W.-D. & Wu, J.-Z. 2010 Helical-wave decomposition and applications to channel turbulence with streamwise rotation. J. Fluid Mech. 662, 91–122.CrossRefGoogle Scholar
Yang, Z., Cui, G., Xu, C. & Zhang, Z. 2012a Large eddy simulation of rotating turbulent channel flow with a new dynamic global-coefficient nonlinear subgrid stress model. J. Turbul. 13 (1), N48.CrossRefGoogle Scholar
Yang, Z. X., Cui, G. X., Zhang, Z. S. & Xu, C. X. 2012b A modified nonlinear sub-grid scale model for large eddy simulation with application to rotating turbulent channel flows. Phys. Fluids 24 (7), 075113.CrossRefGoogle Scholar