Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-01T20:12:49.171Z Has data issue: false hasContentIssue false
  • English
  • Français

Model for classical and ultimate regimes of radiatively driven turbulent convection

Published online by Cambridge University Press:  13 August 2020

M. Creyssels Open the ORCID record for M. Creyssels [Opens in a new window]
M. Creyssels*
Affiliation:
Laboratoire de Mécanique des Fluides et d'Acoustique, Ecole Centrale de Lyon, University of Lyon, CNRS, 69134Ecully, France
*
†Email address for correspondence: mathieu.creyssels@ec-lyon.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

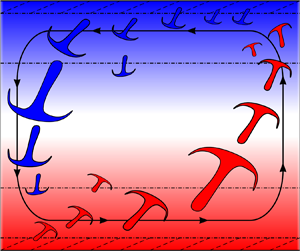
In a standard Rayleigh–Bénard experiment, a layer of fluid is confined between two horizontal plates and the convection regime is controlled by the temperature difference between the hot lower plate and the cold upper plate. The effect of direct heat injection into the fluid layer itself, for example by light absorption, is studied here theoretically. In this case, the Nusselt number ($Nu$) depends on three non-dimensional parameters: the Rayleigh ($Ra$) and Prandtl ($Pr$) numbers and the ratio between the spatial extension of the heat source ($l$) and the height of the fluid layer ($h$). For both the well-known classical and ultimate convection regimes, the theory developed here gives a formula for the variations of the Nusselt number as a function of these parameters. For the classical convection regime, by increasing $l/h$ from 0 to 1/2, $Nu$ gradually changes from the standard scaling $Nu \sim Ra^{1/3}$ to an asymptotic scaling $Nu \sim Ra^{{\theta }}$, with $\theta =2/3$ or $\theta =1$ by adopting, respectively, the Malkus (Proc. R. Soc. A, vol. 225, 1954, pp. 196–212) theory or the Grossmann & Lohse (J. Fluid Mech., vol. 407, 2000, pp. 27–56) theory. For the ultimate convection regime, $Nu$ gradually changes from $Nu \sim Ra^{1/2}$ scaling to an asymptotic behaviour seen only at very high $Ra$ for which $Nu \sim Ra^{2}$. This theory is validated by the recent experimental results given by Bouillaut et al. (J. Fluid Mech., vol. 861, 2019, R5) and also shows that for these experiments, $Ra$ and $Re$ numbers were too small to observe the ultimate regime. The predictions for the ultimate regime cannot be confirmed at this time due to the absence of experimental or numerical work on convection driven by internal sources and for very large $Ra$ numbers.

Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 900 , 10 October 2020 , A39
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh-Bénard convection. Rev. Mod. Phys. 81, 503–537.10.1103/RevModPhys.81.503CrossRefGoogle Scholar
Bouillaut, V., Lepot, S., Aumaître, S. & Gallet, B. 2019 Transition to the ultimate regime in a radiatively driven convection experiment. J. Fluid Mech. 861, R5.CrossRefGoogle Scholar
Chavanne, X., Chillà, F., Castaing, B., Hébral, B., Chabaud, B. & Chaussy, J. 1997 Observation of the ultimate regime in Rayleigh-Bénard convection. Phys. Rev. Lett. 79, 3648–3651.10.1103/PhysRevLett.79.3648CrossRefGoogle Scholar
Chillà, F & Schumacher, J. 2012 New perspectives in turbulent Rayleigh-Bénard convection. Eur. Phys. J. E 35 (7), 58.10.1140/epje/i2012-12058-1CrossRefGoogle ScholarPubMed
Doering, C. R. 2019 Thermal forcing and classical and ultimate regimes of Rayleigh-Bénard convection. J. Fluid Mech. 868, 1–4.10.1017/jfm.2019.118CrossRefGoogle Scholar
Goluskin, D. 2015 Internally Heated Convection and Rayleigh-Bénard Convection. Springer.Google Scholar
Goluskin, D. & van der Poel, E. P. 2016 Penetrative internally heated convection in two and three dimensions. J. Fluid Mech. 791, R6.10.1017/jfm.2016.69CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying view. J. Fluid Mech. 407, 27–56.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl numbers. Phys. Rev. Lett. 86, 3316–3319.10.1103/PhysRevLett.86.3316CrossRefGoogle ScholarPubMed
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23 (4), 045108.CrossRefGoogle Scholar
Kraichnan, R. H. 1962 Turbulent thermal convection at arbitrary Prandtl number. Phys. Fluids 5 (11), 1374–1389.10.1063/1.1706533CrossRefGoogle Scholar
Kulacki, F. A. & Goldstein, R. J. 1972 Thermal convection in a horizontal fluid layer with uniform volumetric energy sources. J. Fluid Mech. 55 (2), 271–287.10.1017/S0022112072001855CrossRefGoogle Scholar
Lepot, S., Aumaître, S. & Gallet, B. 2018 Radiative heating achieves the ultimate regime of thermal convection. Proc. Natl Acad. Sci. USA 115, 8937–8941.10.1073/pnas.1806823115CrossRefGoogle ScholarPubMed
Malkus, W. V. R. 1954 The heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225, 196–212.Google Scholar
Priestley, C. H. B. 1954 Convection from a large horizontal surface. Austral. J. Phys. 7, 176–201.10.1071/PH540176CrossRefGoogle Scholar
Qiu, X.-L., Xia, K.-Q. & Tong, P. 2005 Experimental study of velocity boundary layer near a rough conducting surface in turbulent natural convection. J. Turbul. 6, 30.CrossRefGoogle Scholar
Roche, P.-E., Castaing, B., Chabaud, B. & Hébral, B. 2001 Observation of the 1/2 power law in Rayleigh-Bénard convection. Phys. Rev. E 63 (4), 045303.CrossRefGoogle Scholar
Rusaouën, E., Liot, O., Castaing, B., Salort, J. & Chillà, F. 2018 Thermal transfer in Rayleigh-Bénard cell with smooth or rough boundaries. J. Fluid Mech. 837, 443–460.CrossRefGoogle Scholar
Shen, Y., Tong, P. & Xia, K.-Q. 1996 Turbulent convection over rough surfaces. Phys. Rev. Lett. 76, 908–911.10.1103/PhysRevLett.76.908CrossRefGoogle ScholarPubMed
Shraiman, B. I. & Siggia, E. D. 1990 Heat transport in high-Rayleigh number convection. Phys. Rev. A 42, 3650–3653.10.1103/PhysRevA.42.3650CrossRefGoogle ScholarPubMed
Siggia, E. D. 1994 High rayleigh number convection. Annu. Rev. Fluid Mech. 26 (1), 137–168.CrossRefGoogle Scholar
Spiegel, E. A. 1971 Convection in stars I. Basic Boussinesq convection. Annu. Rev. Astron. Astrophys. 9 (1), 323–352.10.1146/annurev.aa.09.090171.001543CrossRefGoogle Scholar
Stevens, R. J. A. M., van der Poel, E. P., Grossmann, S. & Lohse, D. 2013 The unifying theory of scaling in thermal convection: the updated prefactors. J. Fluid Mech. 730, 295–308.CrossRefGoogle Scholar
Stringano, G., Pascazio, G. & Verzicco, R. 2006 Turbulent thermal convection over grooved plates. J. Fluid Mech. 557, 307–336.CrossRefGoogle Scholar
Tisserand, J.-C., Creyssels, M., Gasteuil, Y., Pabiou, H., Gibert, M., Castaing, B. & Chillà, F. 2011 Comparison between rough and smooth plates within the same Rayleigh-Bénard cell. Phys. Fluids 23 (1), 015105.CrossRefGoogle Scholar
Zhu, X., Stevens, R. J. A. M., Shishkina, O., Verzicco, R. & Lohse, D. 2019 $Nu \sim Ra^{1/2}$ scaling enabled by multiscale wall roughness in Rayleigh-Bénard turbulence. J. Fluid Mech. 869, R4.CrossRefGoogle Scholar
Zhu, X., Stevens, R. J. A. M., Verzicco, R. & Lohse, D. 2017 Roughness-facilitated local $1/2$ scaling does not imply the onset of the ultimate regime of thermal convection. Phys. Rev. Lett. 119, 154501.CrossRefGoogle Scholar