Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-19T15:28:43.608Z Has data issue: false hasContentIssue false
  • English
  • Français

Acoustic feedback loops for screech tones of underexpanded free round jets at different modes

Published online by Cambridge University Press:  08 September 2020

Xiang-Ru Li Open the ORCID record for Xiang-Ru Li [Opens in a new window] ,
Xi-Wen Zhang ,
Peng-Fei Hao  and
Feng He
Xiang-Ru Li
Affiliation:
Department of Engineering Mechanics, School of Aerospace, Tsinghua University, Beijing100084, PR China
Xi-Wen Zhang
Affiliation:
Department of Engineering Mechanics, School of Aerospace, Tsinghua University, Beijing100084, PR China
Peng-Fei Hao
Affiliation:
Department of Engineering Mechanics, School of Aerospace, Tsinghua University, Beijing100084, PR China
Feng He*
Affiliation:
Department of Engineering Mechanics, School of Aerospace, Tsinghua University, Beijing100084, PR China
*
†Email address for correspondence: hefeng@tsinghua.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

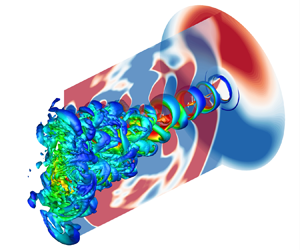
The flow structures and the acoustic feedback loops of underexpanded round jets are investigated by numerical simulations. The jets have a Mach number of 1 at the nozzle exit and a diameter-based Reynolds number of $2.5 \times {10^3}$. Three nozzle pressure ratios (NPRs) of 2.2, 2.4 and 2.6 are considered. The wavelengths of the screech tones are in good agreement with the experimental measurements on high-Reynolds-number jets in the literature. The screech tones are respectively at the A1 and B modes for the jets at NPRs of 2.2 and 2.6. Two screech tones at the A2 and B modes are identified in the jet at the NPR of 2.4 and the wavelet analyses conducted on the pressure fluctuations confirm that these two modes are contemporaneous. The amplitude and phase fields of fluctuating pressure at the screech frequencies are presented in the nozzle exit plane and azimuthal planes. The effective source locations of the screech tones are determined based on the distributions of the phase. The number of periods contained in the screech feedback loop is equal to the number of cells in the standing wave between the nozzle exit and the effective source. The screech frequencies estimated by the classical feedback model agree well with the numerical results at different modes. A modified model, in which the classical feedback model and the upstream-propagating acoustic wave mode of the jet are combined, shows that the screech feedback loops at the A1 and A2 modes are associated with the same acoustic wave mode. The modified model fails to estimate the screech frequencies at the B mode. Different feedback mechanisms lead to the coexistence of the A2 and B modes. The coherent structures corresponding to different screech modes are extracted by dynamic mode decomposition.

JFM classification

Wakes/Jets: Jets Acoustics: Jet noise Acoustics: Aeroacoustics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 902 , 10 November 2020 , A17
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andre, B., Castelain, T. & Bailly, C. 2011 Shock-tracking procedure for studying screech-induced oscillations. AIAA J., 49 (7), 1563–1566.CrossRefGoogle Scholar
Balsara, D. S. & Shu, C.-W. 2000 Monotonicity preserving weighted essentially non-oscillatory schemes with increasingly high order of accuracy. J. Comput. Phys. 160 (2), 405–452.CrossRefGoogle Scholar
Batchelor, G. K. 1953 The Theory of Homogeneous Turbulence. Cambridge University Press.Google Scholar
Berland, J., Bogey, C. & Bailly, C. 2007 Numerical study of screech generation in a planar supersonic jet. Phys. Fluids 19 (7), 075105.CrossRefGoogle Scholar
Bogey, C. & Bailly, C. 2010 Influence of nozzle-exit boundary-layer conditions on the flow and acoustic fields of initially laminar jets. J. Fluid Mech. 663, 507–538.CrossRefGoogle Scholar
Bogey, C. & Gojon, R. 2017 Feedback loop and upwind-propagating waves in ideally expanded supersonic impinging round jets. J. Fluid Mech. 823, 562–591.CrossRefGoogle Scholar
Davies, M. & Oldfield, D. 1962 Tones from a choked axisymmetric jet. I. Cell structure, eddy velocity and source locations. Acta Acust. 12 (4), 257–267.Google Scholar
Edgington-Mitchell, D. 2019 Aeroacoustic resonance and self-excitation in screeching and impinging supersonic jets – a review. Intl J. Aeroacoust. 18 (2–3), 118–188.CrossRefGoogle Scholar
Edgington-Mitchell, D., Jaunet, V., Jordan, P., Towne, A., Soria, J. & Honnery, D. 2018 Upstream-travelling acoustic jet modes as a closure mechanism for screech. J. Fluid Mech. 855, R1.CrossRefGoogle Scholar
Edgington-Mitchell, D., Oberleithner, K., Honnery, D. R. & Soria, J. 2014 Coherent structure and sound production in the helical mode of a screeching axisymmetric jet. J. Fluid Mech. 748, 822–847.Google Scholar
Gao, J. H. & Li, X. D. 2010 A multi-mode screech frequency prediction formula for circular supersonic jets. J. Acoust. Soc. Am. 127 (3), 1251–1257.CrossRefGoogle ScholarPubMed
Glass, D. R. 1968 Effects of acoustic feedback on the spread and decay of supersonic jets. AIAA J. 6 (10), 1890–1897.CrossRefGoogle Scholar
Gojon, R. & Bogey, C. 2017 Numerical study of the flow and the near acoustic fields of an underexpanded round free jet generating two screech tones. Intl J. Aeroacoust. 16 (7–8), 603–625.CrossRefGoogle Scholar
Gojon, R., Bogey, C. & Marsden, O. 2016 Investigation of tone generation in ideally expanded supersonic planar impinging jets using large-eddy simulation. J. Fluid Mech. 808, 90–115.CrossRefGoogle Scholar
Gojon, R., Bogey, C. & Mihaescu, M. 2018 Oscillation modes in screeching jets. AIAA J. 56 (7), 2918–2924.CrossRefGoogle Scholar
Heisenberg, W. 1985 Zur statistischen theorie der turbulenz. In Original Scientific Papers Wissenschaftliche Originalarbeiten, pp. 82–111. Springer.Google Scholar
Hu, T. F. & McLaughlin, D. K. 1990 Flow and acoustic properties of low Reynolds-number underexpanded supersonic jets. J. Sound Vib. 141 (3), 485–505.CrossRefGoogle Scholar
Li, X. D. & Gao, J. H. 2005 Numerical simulation of the generation mechanism of axisymmetric supersonic jet screech tones. Phys. Fluids 17 (8), 085105.CrossRefGoogle Scholar
Li, X. D. & Gao, J. H. 2008 Numerical simulation of the three-dimensional screech phenomenon from a circular jet. Phys. Fluids 20 (3), 035101.CrossRefGoogle Scholar
Lumley, J. L. 1967 The structure of inhomogeneous turbulent flows. In Atmospheric Turbulence and Radio Wave Propagation (ed. Yaglom, A. M. & Tatarski, V. I.), pp. 166–178. Nauka.Google Scholar
Mancinelli, M., Jaunet, V., Jordan, P. & Towne, A. 2019 Screech-tone prediction using upstream-travelling jet modes. Exp. Fluids 60, 22.CrossRefGoogle Scholar
Mancinelli, M., Pagliaroli, T., Di Marco, A., Camussi, R. & Castelain, T. 2017 Wavelet decomposition of hydrodynamic and acoustic pressures in the near field of the jet. J. Fluid Mech. 813, 716–749.CrossRefGoogle Scholar
Mercier, B., Castelain, T. & Bailly, C. 2017 Experimental characterisation of the screech feedback loop in underexpanded round jets. J. Fluid Mech. 824, 202–229.Google Scholar
Merle, M. 1956 Sur la frequence des ondes sonores emises par un jet dair a grande vitesse. C. R. Hebd. Seances Acad. Sci. 243 (5), 490–493.Google Scholar
Morinishi, Y., Vasilyev, O. V. & Ogi, T. 2004 Fully conservative finite difference scheme in cylindrical coordinates for incompressible flow simulations. J. Comput. Phys. 197 (2), 686–710.Google Scholar
Pack, D. C. 1950 A note on Prandtl formula for the wave-length of a supersonic gas jet. Q. J. Mech. Appl. Maths 3 (2), 173–181.CrossRefGoogle Scholar
Panda, J. 1998 Shock oscillation in underexpanded screeching jets. J. Fluid Mech. 363, 173–198.CrossRefGoogle Scholar
Panda, J. 1999 An experimental investigation of screech noise generation. J. Fluid Mech. 378, 71–96.CrossRefGoogle Scholar
Panda, J. & Seasholtz, R. G. 1999 Measurement of shock structure and shock–vortex interaction in underexpanded jets using Rayleigh scattering. Phys. Fluids 11 (12), 3761–3777.Google Scholar
Ponton, M. K. & Seiner, J. M. 1992 The effects of Nozzle exit lip thickness on plume resonance. J. Sound Vib. 154 (3), 531–549.Google Scholar
Ponton, M. K. & Seiner, J. M. 1995 Acoustic study of B helical mode for choked axisymmetric nozzle. AIAA J. 33 (3), 413–420.CrossRefGoogle Scholar
Powell, A. 1953 On the mechanism of choked jet noise. Proc. Phys. Soc. Lond. B 66 (408), 1039–1056.CrossRefGoogle Scholar
Powell, A., Umeda, Y. & Ishii, R. 1992 Observations of the oscillation modes of choked circular jets. J. Acoust. Soc. Am. 92 (5), 2823–2836.CrossRefGoogle Scholar
Raman, G. 1997 Screech tones from rectangular jets with spanwise oblique shock-cell structures. J. Fluid Mech. 330, 141–168.CrossRefGoogle Scholar
Raman, G. 1998 Advances in understanding supersonic jet screech: review and perspective. Prog. Aerosp. Sci. 34 (1–2), 45–106.CrossRefGoogle Scholar
Raman, G. 1999 Supersonic jet screech: half-century from Powell to the present. J. Sound Vib. 225 (3), 543–571.CrossRefGoogle Scholar
Ruuth, S. J. & Spiteri, R. J. 2004 High-order strong-stability-preserving Runge–Kutta methods with downwind-biased spatial discretizations. SIAM J. Numer. Anal. 42 (3), 974–996.Google Scholar
Schlichting, H. & Gersten, K. 2016 Boundary-Layer Theory. Springer.Google Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.CrossRefGoogle Scholar
Shen, H. & Tam, C. K. W. 2002 Three-dimensional numerical simulation of the jet screech phenomenon. AIAA J. 40 (1), 33–41.CrossRefGoogle Scholar
Sirovich, L. 1987 Turbulence and the dynamics of coherent structures. I. Coherent structures. Q. Appl. Maths 45 (3), 561–571.CrossRefGoogle Scholar
Steger, J. L. & Warming, R. F. 1981 Flux vector splitting of the inviscid gas-dynamic equations with application to finite-difference methods. J. Comput. Phys. 40 (2), 263–293.CrossRefGoogle Scholar
Suzuki, T. & Lele, S. K. 2003 Shock leakage through an unsteady vortex-laden mixing layer: application to jet screech. J. Fluid Mech. 490, 139–167.CrossRefGoogle Scholar
Taira, K., Brunton, S. L., Dawson, S. T. M., Rowley, C. W., Colonius, T., McKeon, B. J., Schmidt, O. T., Gordeyev, S., Theofilis, V. & Ukeiley, L. S. 2017 Modal analysis of fluid flows: an overview. AIAA J. 55 (12), 4013–4041.CrossRefGoogle Scholar
Tam, C. K. W. 1995 Supersonic jet noise. Annu. Rev. Fluid Mech. 27 (1), 17–43.CrossRefGoogle Scholar
Tam, C. K. W. & Ahuja, K. K. 1990 Theoretical-model of discrete tone generation by impinging jets. J. Fluid Mech. 214, 67–87.CrossRefGoogle Scholar
Tam, C. K. W. & Hu, F. Q. 1989 On the 3 families of instability waves of high-speed jets. J. Fluid Mech. 201, 447–483.CrossRefGoogle Scholar
Tam, C. K. W. & Norum, T. D. 1992 Impingement tones of large aspect ratio supersonic rectangular jets. AIAA J. 30 (2), 304–311.CrossRefGoogle Scholar
Thompson, K. W. 1987 Time-dependent boundary-conditions for hyperbolic systems. J. Comput. Phys. 68 (1), 1–24.CrossRefGoogle Scholar
Thompson, K. W. 1990 Time-dependent boundary-conditions for hyperbolic systems, II. J. Comput. Phys. 89 (2), 439–461.Google Scholar
Towne, A., Cavalieri, A. V. G., Jordan, P., Colonius, T., Schmidt, O., Jaunet, V. & Brès, G. A. 2017 Acoustic resonance in the potential core of subsonic jets. J. Fluid Mech. 825, 1113–1152.CrossRefGoogle Scholar
Umeda, Y. & Ishii, R. 2001 On the sound sources of screech tones radiated from choked circular jets. J. Acoust. Soc. Am. 110 (4), 1845–1858.Google ScholarPubMed
Walker, S. H., Gordeyev, S. V. & Thomas, F. O. 1997 A wavelet transform analysis applied to unsteady aspects of supersonic jet screech resonance. Exp. Fluids 22 (3), 229–238.Google Scholar
Wu, M. & Martin, M. P. 2007 Direct numerical simulation of supersonic turbulent boundary layer over a compression ramp. AIAA J. 45 (4), 879–889.CrossRefGoogle Scholar

Li et al. supplementary movie 1

The representation of the isosurfaces of the nondimensional Q-criterion=0.1, colored by the local Mach number, and of the pressure fluctuations in the planes of x=0, θ=π/2 and 3π/2 for the jet at the NPR of 2.2. The nozzle exit is located at x=0.

Download Li et al. supplementary movie 1(Video)
Video 8.3 MB

Li et al. supplementary movie 2

The representation of the isosurfaces of the nondimensional Q-criterion=0.1, colored by the local Mach number, and of the pressure fluctuations in the planes of x=0, θ=π/2 and 3π/2 for the jet at the NPR of 2.4. The nozzle exit is located at x=0.

Download Li et al. supplementary movie 2(Video)
Video 9.1 MB

Li et al. supplementary movie 3

The representation of the isosurfaces of the nondimensional Q-criterion=0.1, colored by the local Mach number, and of the pressure fluctuations in the planes of x=0, θ=π/2 and 3π/2 for the jet at the NPR of 2.6. The nozzle exit is located at x=0.

Download Li et al. supplementary movie 3(Video)
Video 9.2 MB