Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-19T20:47:34.421Z Has data issue: false hasContentIssue false
  • English
  • Français

Uniform Convexity and the Bishop–Phelps–Bollobás Property

Published online by Cambridge University Press:  20 November 2018

Sun Kwang Kim  and
Han Ju Lee
Sun Kwang Kim
Affiliation:
School of Mathematics, Korea Institute for Advanced Study (KIAS), 85 Hoegiro, Dongdaemun–gu, Seoul 130- 722, Republic of Korea. e-mail: lineksk@gmail.com
Han Ju Lee
Affiliation:
Department of Mathematics Education, Dongguk University–Seoul, 100-715 Seoul, Republic of Korea. e-mail: hanjulee@dongguk.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

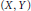A new characterization of the uniform convexity of Banach space is obtained in the sense of the Bishop–Phelps–Bollobás theorem. It is also proved that the couple of Banach spaces $\left( X,Y \right)$ has the Bishop–Phelps–Bollobás property for every Banach space $Y$ when 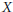$X$ is uniformly convex. 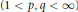As a corollary, we show that the Bishop–Phelps–Bollobás theorem holds for bilinear forms on ${{\ell }_{p}}\,\times \,{{\ell }_{q}}$$\left( 1\,<p,q\,<\,\infty \right)$.

Keywords

46B2046B22Bishop–Phelps–Bollobás propertyBishop–Phelps–Bollobás theoremnorm attaininguniformly convex
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 66 , Issue 2 , 01 April 2014 , pp. 373 - 386
Copyright
Copyright © Canadian Mathematical Society 2014

References

[1] Acosta, M. D., Alaminos, J.,García, D., and Maestre, M., On holomorphic functions attaining their norms. J. Math. Anal. Appl. 297(2004), no. 2, 625–644. http://dx.doi.org/10.1016/j.jmaa.2004.04.010 Google Scholar
[2] Acosta, M. D., Aron, R. M., García, D., and Maestre, M., The Bishop-Phelps-Bollobás theorem foroperators. J. Funct. Anal. 254(2008), no. 11, 2780–2799. http://dx.doi.org/10.1016/j.jfa.2008.02.014 Google Scholar
[3] Aron, R. M., Cascales, B., and Kozhushkina, O., The Bishop-Phelps-Bollobás theorem and Asplund operators. Proc. Amer. Math. Soc. 139(2011), no. 10, 3553–3560. http://dx.doi.org/10.1090/S0002-9939-2011-10755-X Google Scholar
[4] Aron, R. M., Choi, Y. S., García, D., and Maestre, M., The Bishop-Phelps-Bollobás theorem for L(L1(μ); L∞[0; 1]). Adv. Math. 228(2011), no. 1, 617–628. http://dx.doi.org/10.1016/j.aim.2011.05.023 Google Scholar
[5] Aron, R. M., Finet, C., and Werner, E., Some remarks on norm attaining N-linear forms. In: Functions spaces (Edwardsville, IL, 1994), Lecture Notes in Pure and Appl. Math., 172, Dekker, New York, 1995, pp. 19–28.Google Scholar
[6] Bishop, E. and Phelps, R. R., A proof that every Banach space is subreflexive. Bull. Amer. Math. Soc. 67(1961), 97–98. http://dx.doi.org/10.1090/S0002-9904-1961-10514-4 Google Scholar
[7] Bollobás, B., An extension to the theorem of Bishop and Phelps. Bull. London. Math. Soc. 2(1970), 181–182. http://dx.doi.org/10.1112/blms/2.2.181 Google Scholar
[8] Bourgain, J., Dentability and the Bishop-Phelps property. Israel J. Math. 28(1977), no. 4, 265–271. http://dx.doi.org/10.1007/BF02760634 Google Scholar
[9] Cheng, L. and Dai, D., The Bishop-Phelps-Bollobás Theorem for bilinear forms. Preprint.Google Scholar
[10] Choi, Y. S., Norm attaining bilinear forms on L1[0; 1]. J. Math. Anal. Appl. 211(1997), no. 1,295–300. http://dx.doi.org/10.1006/jmaa.1997.5461 Google Scholar
[11] Choi, Y. S. and Kim, S. G., Norm or numerical radius attaining multilinear mappings and polynomials. J. London Math. Soc. 54(1996), no. 1, 135–147. http://dx.doi.org/10.1112/jlms/54.1.135 Google Scholar
[12] Choi, Y. S. and Kim, S. K., The Bishop-Phelps-Bollobás theorem for operators from L1(μ) to Banach spaces with the Radon-Nikodým property. J. Funct. Anal. 261(2011), no. 6, 1446–1456. http://dx.doi.org/10.1016/j.jfa.2011.05.007 Google Scholar
[13] Choi, Y. S., The Bishop-Phelps-Bollobás property and lush spaces. J. Math. Anal. Appl. 390(2012), no. 2, 549–555. http://dx.doi.org/10.1016/j.jmaa.2012.01.053 Google Scholar
[14] Choi, Y. S., Lee, H. J., and Song, H. G., Denseness of norm-attaining mappings on Banach spaces. Publ. Res. Inst. Math. Sci. 46(2010), no. 1, 171–182. http://dx.doi.org/10.2977/PRIMS/4 Google Scholar
[15] Choi, Y. S., Bishop's theorem and differentiability of a subspace of Cb(K). Israel J. Math. 180(2010), 93–118. http://dx.doi.org/10.1007/s11856-010-0095-9 Google Scholar
[16] Choi, Y. S. and Song, H. G., The Bishop-Phelps-Bollobás theorem fails for bilinear forms on l1 × l1. J. Math. Anal. Appl. 360(2009), no. 2, 752–753. http://dx.doi.org/10.1016/j.jmaa.2009.07.008 Google Scholar
[17] Fabian, M., Habala, P., Hà jek, P., Montesinos, V., and Zizler, V., Banach space theory. The basis for linear and nonlinear analysis. CMS Books in Mathematics, Springer, New York, 2011.Google Scholar
[18] Finet, C. and Payà, R., Norm attaining operators from L1 into L∞. Israel J. Math. 108(1998), 139–143. http://dx.doi.org/10.1007/BF02783045 Google Scholar
[19] James, R. C., Weak compactness and reflexivity. Israel J. Math. 2(1964), 101–119. http://dx.doi.org/10.1007/BF02759950 Google Scholar
[20] Kim, J. and Lee, H. J., Strong peak points and strongly norm attaining points with applications to denseness and polynomial numerical indices. J. Funct. Anal. 257(2009), no. 4, 931–947. http://dx.doi.org/10.1016/j.jfa.2008.11.024 Google Scholar
[21] Kim, S. G. and Lee, H. J., Numerical peak holomorphic functions on Banach spaces. J. Math. Anal. Appl. 364(2010), no. 2, 437–452. http://dx.doi.org/10.1016/j.jmaa.2009.10.046 Google Scholar
[22] Kim, S. K., The Bishop-Phelps-Bollobás theorem for operators from c0 to uniformly convex spaces. Israel. J. Math., to appear. http://dx.doi.org/10.1007/s11856-012-0186-x Google Scholar
[23] Lindenstrauss, J., On operators which attain their norm. Israel J. Math. 1(1963), 139–148. http://dx.doi.org/10.1007/BF02759700 Google Scholar
[24] Payá, R.and Saleh, Y., Norm attaining operators from L1(μ) into L∞(ν). Arch.Math. 75(2000), no. 5, 380–388. http://dx.doi.org/10.1007/s000130050519 Google Scholar
[25] Schachermayer, W., Norm attaining operators on some classical Banach spaces. Pacific J. Math. 105(1983), no. 2, 427–438. http://dx.doi.org/10.2140/pjm.1983.105.427 Google Scholar
[26] Stegall, C., Optimization and differentiation in Banach spaces. Proceedings of the symposium on operator theory (Athens, 1985), Linear Algebra Appl. 84(1986), 191–211. http://dx.doi.org/10.1016/0024-3795(86)90314-9 Google Scholar
[27] Uhl, J. J., Jr, Norm attaining operators on L1[0; 1] and the Radon-Nykodým property. Pacific J. Math. 63(1976), no. 1, 293–300. http://dx.doi.org/10.2140/pjm.1976.63.293 Google Scholar