Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-18T04:21:47.382Z Has data issue: false hasContentIssue false
  • English
  • Français

The Absolute Arithmetic Continuum and the Unification Of all Numbers Great and Small

Published online by Cambridge University Press:  15 January 2014

Philip Ehrlich
Philip Ehrlich*
Affiliation:
Department of Philosophy, Ohio University, Athens, OH 45701, USAE-mail: ehrlich@ohio.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

In his monograph On Numbers and Games, J. H. Conway introduced a real-closed field containing the reals and the ordinals as well as a great many less familiar numbers including −ω, ω/2, 1/ω, 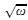and ω − π to name only a few. Indeed, this particular real-closed field, which Conway calls No, is so remarkably inclusive that, subject to the proviso that numbers—construed here as members of ordered fields—be individually definable in terms of sets of NBG (von Neumann–Bernays–Gödel set theory with global choice), it may be said to contain “All Numbers Great and Small.” In this respect, No bears much the same relation to ordered fields that the system ℝ of real numbers bears to Archimedean ordered fields.

In Part I of the present paper, we suggest that whereas ℝ should merely be regarded as constituting an arithmetic continuum (modulo the Archimedean axiom), No may be regarded as a sort of absolute arithmetic continuum (modulo NBG), and in Part II we draw attention to the unifying framework No provides not only for the reals and the ordinals but also for an array of non-Archimedean ordered number systems that have arisen in connection with the theories of non-Archimedean ordered algebraic and geometric systems, the theory of the rate of growth of real functions and nonstandard analysis.

In addition to its inclusive structure as an ordered field, the system No of surreal numbers has a rich algebraico-tree-theoretic structure—a simplicity hierarchical structure—that emerges from the recursive clauses in terms of which it is defined. In the development of No outlined in the present paper, in which the surreals emerge vis-à-vis a generalization of the von Neumann ordinal construction, the simplicity hierarchical features of No are brought to the fore and play central roles in the aforementioned unification of systems of numbers great and small and in some of the more revealing characterizations of No as an absolute continuum.

Type
Research Article
Information
Bulletin of Symbolic Logic , Volume 18 , Issue 1 , March 2012 , pp. 1 - 45
Copyright
Copyright © Association for Symbolic Logic 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ackermann, Wilhelm [1956], Zur Axiomatik der Mengenlehre, Mathematische Annalen, vol. 131, pp. 336–345.Google Scholar
Alling, Norman [1962], On the existence of real-closedfields that are ηα-sets of power ℵα , Transactions of the American Mathematical Society, vol. 103, pp. 341–352.Google Scholar
Alling, Norman [1985], Conway's field of surreal numbers, Transactions of the American Mathematical Society, vol. 287, pp. 365–386.Google Scholar
Alling, Norman [1987], Foundations of analysis over surreal number fields, North-Holland Publishing Company, Amsterdam.Google Scholar
Alling, Norman and Ehrlich, Philip [1986], An alternative construction of Conway's surreal numbers, Comptes Rendus Mathématiques de l'Académie des Sciences (Mathematical Reports), vol. VIII, pp. 241–246.Google Scholar
Alling, Norman and Ehrlich, Philip [1987], Sections 4.02 and 4.03 of [Alling 1987].Google Scholar
Artin, Emil and Schreier, Otto [1926], Algebraische Konstruktion reeller Körper, Abhandlungen aus dem Mathematischen Seminar der Hamburgischen Univeristät, vol. 5, pp. 85–99, reprinted in Serge Lang and John T. Tate (editors), The Collected Papers of Emil Artin, Addison-Wesley Publishing Company, Reeding, MA, 1965, pp. 258–272.Google Scholar
Aschenbrenner, Matthias and van den Dries, Lou [2000], Closed asymptotic couples, Journal of Algebra, vol. 225, pp. 309–358.Google Scholar
Boshernitzan, Michael [1981], An extension of Hardy's class L of “orders of infinity”, Journal d'Analyse Mathématique, vol. 39, pp. 235–255.Google Scholar
Bourbaki, Nicholas (pseudonym) [1951], Élements de mathématique. XII. Première partie: Les structures fondamentales de l'analyse. Livre IV: Fonctions d'une variable réelle, Hermann, Paris.Google Scholar
Cantor, Georg [1883], Grundlagen einer allgemeinen Mannigfaltigkeitslehre. Ein mathematisch-philosophischer Versuch in der Lehre des Unendlichen, B. G. Teubner, Leipzig, reprinted in [Cantor 1932, pp. 165–208]. English translation by W. D. Ewald in [Ewald 1996, pp. 881–920].Google Scholar
Cantor, Georg [1895], Sui numeri transfiniti, Rivista di Matematica, vol. 5, pp. 104–109.Google Scholar
Cantor, Georg [1932], Gesammelte Abhandlungen mathematischen und philosophischen Inhalts. Herausgegeben von Ernst Zermelo, nebst einem Lebenslauf Cantors von A. Fraenkel, J. Springer, Berlin, reprinted Hildesheim, Olms, 1966.Google Scholar
Carruth, Philip [1942], Arithmetic of ordinals with applications to the theory of ordered Abelian groups, Bulletin of the American Mathematical Society, vol. 48, pp. 262–271.Google Scholar
Chang, C. C. and Keisler, H. J. [1990], Model theory, third ed., North-Holland Publishing Company, Amsterdam.Google Scholar
Clifford, A. H. [1954], Note on Hahn's theorem on ordered Abelian groups, Proceedings of the American Mathematical Society, vol. 5, pp. 860–863.Google Scholar
Conrad, Paul [1954], On ordered division rings, Proceedings of the American Mathematical Society, vol. 5, pp. 323–328.Google Scholar
Conrad, Paul and Dauns, John [1969], An embedding theorem for lattice-ordered fields, Pacific Journal of Mathematics, vol. 30, pp. 385–397.Google Scholar
Conway, J. H. [1976], On numbers and games, Academic Press, London, Second edition, A K. Peters, Ltd., Natick, Massachusetts, 2001.Google Scholar
Dutari, Norberto Cuesta [1954], Algebra ordinal, Revista de la Real Academia de Ciencias Exactas. Físicas y Naturales, vol. 48, pp. 103–145.Google Scholar
Dutari, Norierto Cuesta [1958–1959], Matematica del orden, Revista de la Real Academia de Ciencias Exactas. Físicas y Naturales, vol. 52, pp. 147–321, 609–770; 53, pp. 33–190. These papers were reprinted in book form as Matematica del Orden, Madrid, 1959, and reissued in 1994 by the Universidad de Salamanca, Salamanca, Spain.Google Scholar
Dales, H. Garth and Wooden, W. Hugh [1996], Super-real ordered fields, Clarendon Press, Oxford.CrossRefGoogle Scholar
Dauben, Joseph [1979], Georg Cantor: His mathematics and philosophy of the infinite, Harvard University Press, Cambridge, Massachusetts.Google Scholar
Drake, Frank [1974], Set theory: An introduction to large cardinals, North-Holland Publishing Company, Amsterdam.Google Scholar
Du Bois-Reymond, Paul [1870–1871], Sur la grandeur relative des infinis des functions, Annali di matematica pura ed applicata, vol. 4, pp. 338–353.CrossRefGoogle Scholar
Bois-Reymond, Paul Du [1875], Ueber asymptotische Werthe, infinitäre Approximationen und infinitäre Auflösung von Gleichungen, Mathematische Annalen, vol. 8, pp. 363–414; (Nachträge zur Abhandlung: ueber asymptotische Werthe etc., pp. 574–576).Google Scholar
Du Bois-Reymond, Paul [1877], Ueber die Paradoxen des Infinitärcalcüls, Mathematische Annalen, vol. 11, pp. 149–167.Google Scholar
Du Bois-Reymond, Paul [1882], Die allgemeine Functionentheorie I, Verlag der H. Laupp'schen Buchhandlung, Tübingen.Google Scholar
Ehrlich, Philip [1987], The absolute arithmetic and geometric continua, PSA 1986 (Fine, Arthur and Machamer, Peter, editors), vol. 2, Philosophy of Science Association, Lansing, MI.Google Scholar
Ehrlich, Philip [1988], An alternative construction of Conway's ordered field No, Algebra Universalis, vol. 25, pp. 7–16, Errata, vol. 25 (1988), p. 233.Google Scholar
Ehrlich, Philip [1989], Absolutely saturated models, Fundamenta Mathematicae, vol. 133, no. 1, pp. 39–46.Google Scholar
Ehrlich, Philip [1989a], Universally extending continua, Abstracts of Papers Presented to the American Mathematical Society, vol. 10, no. 1, p. 15.Google Scholar
Ehrlich, Philip [1992], Universally extending arithmetic continua, Le labyrinthe du continu, colloque de Cerisy (Sinaceur, Hourya and Salanskis, Jean-Michel, editors), Springer-Verlag France, Paris.Google Scholar
Ehrlich, Philip (editor) [1994], Real numbers, generalizations of the reals, and theories of continua, Kluwer Academic Publishers, Dordrecht, Netherlands.Google Scholar
Ehrlich, Philip [1994a], All number great and small, Real numbers, generalizations of the reals, and theories of continua (Ehrlich, Philip, editor), Kluwer Academic Publishers, Dordrecht, Netherlands, pp. 239–258.Google Scholar
Ehrlich, Philip [1995], Hahn's Über die nichtarchimedischen Grössensysteme and the development of the modern theory of magnitudes and numbers to measure them, From Dedekind to Gödel: Essays on the development of the foundations of mathematics (Hintikka, Jaakko, editor), Kluwer Academic Publishers, Dordrecht, Netherlands.Google Scholar
Ehrlich, Philip [1997], Dedekind cuts of Archimedean complete ordered Abelian groups, Algebra Universalis, vol. 37, pp. 223–234.Google Scholar
Ehrlich, Philip [1997a], From completeness to Archimedean completeness: An essay in the foundations of Euclidean geometry, in A Symposium on David Hilbert edited by Tauber, Alfred and Kanamori, Akihiro, Synthese , vol. 110, pp. 57–76.Google Scholar
Ehrlich, Philip [2001], Number systems with simplicity hierarchies: A generalization of Conway's theory of surreal numbers, The Journal of Symbolic Logic, vol. 66, no. 3, pp. 1231–1258, Corrigendum, vol. 70 (2005), no. 3, p. 1022.Google Scholar
Ehrlich, Philip [2002], The absolute arithmetic continuum and the unification of all numbers great and small (abstract), Philosophical insights into logic and mathematics (abstracts), Archive Henri Poincaré, Université de Nancy 2, Laboratoire de Philosophie et d'Historie des Sciences, Nancy, France, pp. 41–43.Google Scholar
Ehrlich, Philip [2002a], Surreal numbers: An alternative construction (abstract), this Bulletin, vol. 8, no. 3, p. 448.Google Scholar
Ehrlich, Philip [2004], Surreal numbers and the unification of all numbers great and small (abstract), this Bulletin, vol. 10, no. 2, p. 253.Google Scholar
Ehrlich, Philip [2005], Continuity, Encyclopedia of philosophy, 2nd edition (Borchert, Donald M., editor), Macmillan Reference USA, Farmington Hills, MI, pp. 489–517. (The online version contains some slight improvements.).Google Scholar
Ehrlich, Philip [2006], The rise of non-Archimedean mathematics and the roots of a misconception I: the emergence ofnon-Archimedean systems of magnitudes, Archive for History of Exact Sciences, vol. 60, pp. 1–121.Google Scholar
Ehrlich, Philip [2007], Review of “The continuous and the infinitesimal in mathematics and philosophy” by John L. Bell, this Bulletin, vol. 13, no. 3, pp. 361–363.Google Scholar
Ehrlich, Philip [2010], The absolute arithmetic continuum and its Peircean counterpart, New essays on Peirce's mathematical philosophy (Moore, Matthew, editor), Open Court Press, pp. 235–282.Google Scholar
Ehrlich, Philip [2011], Conway names, the simplicity hierarchy and the surreal number tree, Journal of Logic and Analysis, vol. 3, pp. 1–26.Google Scholar
Ehrlich, Philip [forthcoming 1], The absolute arithmetic continuum, http://www.ohio.edu/people/ehrlich/.Google Scholar
Ehrlich, Philip [forthcoming 2], The rise of non-Archimedean mathematics and the roots of a misconception II: the emergence of non-Archimedean geometry and the theory of non-Archimedean ordered algebraic systems, Archive for History of Exact Sciences.Google Scholar
Enriques, Federigo [1911], Sui numeri non archimedei e su alcune loro interpretazioni, Bollettino della Mathesis, vol. IIIa, pp. 87–105.Google Scholar
Enriques, Federigo [1912], Inumeri reali, Questioni riguardanti le matematiche elementari (Enriques, Federigo and Zanichelli, Nicola, editors), vol. 1, Bologna, pp. 365–493, Second Edition 1924, pp. 231–389.Google Scholar
Erdös, Paul, Gillman, Leonard, and Henriksen, Melvin [1955], An isomorphism theorem for real-closed fields, Annals of Mathematics. Series 2, vol. 61, pp. 542–554.Google Scholar
Esterle, Jean [1977], Solution d'un problème d'Erdös, Gillman et Henriksen et application à l'étude des homomorphismes de C(K), Acta Mathematica Academiae Scientiarum Hungaricae, vol. 30, pp. 113–127.Google Scholar
Euler, Leonhard [1778], De infinities infinitis gradibus tam infinite magnorum quam infinite parvorum, Acta Academiae Scientiarum Petroplitanae (II) I, 1780, pp. 102–118. Reprinted in Opera Omnia (I) XV, pp. 298–313.Google Scholar
Ewald, William. B. (editor) [1996], From Kant to Hilbert: A source book in the foundations of mathematics, vol. II, Clarendon Press, Oxford.Google Scholar
Fisher, Gordon [1981], The infinite and infinitesimal quantities of du Bois-Reymond and their reception, Archive for History of Exact Sciences, vol. 24, pp. 101–164.Google Scholar
Fornasiero, Antongiulio [2004], Integration on surreal numbers, Ph.D. thesis, University of Edinburgh.Google Scholar
Fornasiero, Antongiulio [2006], Embedding Henselian fields into power series, Journal of Algebra, vol. 304, pp. 112–156.Google Scholar
Fraenkel, Airaham A., Bar-Hillel, Yehoshua, and Lévy, Azriel [1973], Foundations of set theory, second revised ed., North-Holland Publishing Company, Amsterdam.Google Scholar
Fuchs, Laszlo [1963], Partially ordered algebraic systems, Pergamon Press.Google Scholar
Gillman, Leonard and Jerison, Meyer [1961], Rings of continuous functions, Van Nostrand, 1976.Google Scholar
Goldblatt, Robert [1998], Lectures on the hyperreals. An introduction to nonstandard analysis, Springer, New York.Google Scholar
Gonshor, Harry [1986], An introduction to the theory of surreal numbers, Cambridge University Press, Cambridge.Google Scholar
Hahn, Hans [1907], Über die nichtarchimedischen Grössensysteme, Sitzungsberichte der Kaiserlichen Akademie der Wissenschaften, Wien, Mathematisch-Naturwissenschaftliche Klasse, vol. 116 (Abteilung IIa), pp. 601–655.Google Scholar
Hardy, G. H. [1910], Orders of infinity, the “Infinitärcalcül” of Paul Du Bois-Reymond, Cambridge University Press, Cambridge, Second edition, 1924; First edition reprinted by Hafner, New York, 1971.Google Scholar
Hardy, G. H. [1912], Properties of logarithmico-exponential functions, Proceedings of the London Mathematical Society. Second Series, vol. 10, pp. 54–90.Google Scholar
Harzheim, Egbert [2005], Ordered sets, Springer, New York.Google Scholar
Hausdorff, Felix [1907], Untersuchungen über Ordungtypen, Berichte über die Verhandlungen der königlich sächsischen Gesellschaft der Wissenschaften zu Leipzig, Matematisch-Physische Klasse, vol. 59, pp. 84–159, for English translation by J. M. Plotkin, see [Plotkin 2005].Google Scholar
Hausdorff, Felix [1909], Die Graduierung nach dem Endverlauf, Abhandlungen der königlich sächsischen Gesellschaft der Wissenschaften zu Leipzig, Matematisch-Physische Klasse, vol. 31, pp. 295–335, for English translation by J. M. Plotkin, see [Plotkin 2005].Google Scholar
Hausdorff, Felix [1914], Grundzüge der Mengenlehre, Leipzig.Google Scholar
Hausdorff, Felix [1927], Mengenlehre, W. de Gruyter, Berlin.Google Scholar
Hausdorff, Felix [1957], Set theory, Chelsea Publishing Company, New York.Google Scholar
Hessenberg, Gerhard [1906], Grundbegriffe der Mengenlehre, Abhandlungen der Friesschen Schule, (Neue Folge), Band I, Heft IV, Göttingen, reprinted by Vandenhoeck & Ruprecht, Göttingen, 1906.Google Scholar
Hewitt, Edwin [1948], Rings of real-valued continuous functions. I, Transactions of the American Mathematical Society, vol. 64, pp. 45–99.Google Scholar
Hilbert, David [1899], Grundlagen der Geometrie, Teubner, Leipzig.Google Scholar
Hilbert, David [1900], Über den Zahlbegriff, Jahresbericht der Deutschen Mathematiker–Vereinigung, vol. 8, pp. 180–184.Google Scholar
Hilbert, David [1971], Foundations of geometry, 10th edition of [Hilbert 1899] translated by Unger, Leo, Open Court, LaSalle, IL.Google Scholar
Jónsson, Bjarni [1960], Homogeneous universal relational structures, Mathematica Scandinavica, vol. 8, pp. 137–142.Google Scholar
Kanamori, Akihiro [2007], Gödel and set theory, this Bulletin, vol. 13, pp. 153–188.Google Scholar
Kaplansky, Irving [1942], Maximal fields with valuations, Duke Mathematical Journal, vol. 9, pp. 303–321.Google Scholar
Keisler, H. Jerome [1963], Limit ultrapowers, Transactions of the American Mathematical Society, vol. 107, pp. 383–408.Google Scholar
Keisler, H. Jerome [1976], Foundations of infinitesimal calculus, Prindle, Weber & Schmidt, Incorporated, 20 Newbury Street, Boston.Google Scholar
Keisler, H. Jerome [1976a], Elementary calculus, second 1986 ed., Prindle, Weber & Schmidt, Incorporated, 20 Newbury Street, Boston, now available free online at http://www.math.wisc.edu/~keisler/cal.html.Google Scholar
Keisler, H. Jerome [1994], The hyperreal line, Real numbers, generalizations of the reals, and theories of continua (Ehrlich, Philip, editor), Kluwer Academic Publishers, Dordrecht, Netherlands, pp. 207–237.Google Scholar
Klaua, Dieter [1994], Rational and real ordinal numbers, Real numbers, generalizations of the reals, and theories of continua (Ehrlich, Philip, editor), Kluwer Academic Publishers, Dordrecht, Netherlands, pp. 259–276.Google Scholar
Krull, Wolfgang [1932], Allgemeine Bewertungstheorie, Journal für die Reine und Angewandt Mathematik, vol. 167, pp. 160–196.Google Scholar
Kuhlmann, Salma [2000], Ordered exponential fields, Fields Institute Monographs, vol. 12, American Mathematical Society, Providence, RI.Google Scholar
Leibniz, Gottfried Wilhelm [1875–1890], Die philosophischen Schriften von Gottfried Wilhelm Leibniz, 7 volumes, edited by Gerhardt, C. I., Weidmann, Berlin. Reprinted by Olms, Hildesheim, 1960.Google Scholar
Levi-Civita, Tullio [1892–1893], Sugli infiniti ed infinitesimi attuali quali elementi analitici, Atti del R. Istituto Veneto di Scienze Lettre ed Arti, Venezia (Serie 7), vol. 4, pp. 1765–1815, reprinted in Tullio Levi-Civita, Opere Matematiche, Memorie e Note , Volume primo 1893–1900, Nicola Zanichelli, Bologna, 1954.Google Scholar
Levi-Civita, Tullio [1898], Sui numeri transfiniti, Atti della Reale Accademia dei Lincei, Classe di scienze fisiche, matematiche e naturali, Rendiconti, Roma serie Va, vol. 7, pp. 91–96, 113–121, reprinted in Tullio Levi-Civita, Opere Matematiche, Memorie e Note , Volume primo 1893–1900, Nicola Zanichelli, Bologna, 1954.Google Scholar
Lévy, Azriel [1959], On Ackermann's set theory, The Journal of Symbolic Logic, vol. 24, pp. 154–166.Google Scholar
Lévy, Azriel [1976], The role of classes in set theory, Sets and classes (Müller, G. H., editor), North-Holland Publishing Company, Amsterdam, pp. 173–215.Google Scholar
Lévy, Azriel and Vaught, Robert [1961], Principles of partial reflection in the set theories of Zermelo and Ackermann, Pacific Journal of Mathematics, vol. 11, pp. 1045–1062.Google Scholar
Meschkowski, Herbert and Nilson, Winfried (editors) [1991], Georg Cantor. Briefe, Springer-Verlag, Berlin.Google Scholar
Morley, Michael and Vaught, Robert [1962], Homogeneous universal models, Mathematica Scandinavica, vol. 11, pp. 37–57.Google Scholar
Mourgues, Marie-Hélène and Ressayre, Jean-Pierre [1991], A transfinite version of Puiseux's theorem with applications to real closed fields, Logic Colloquium'90 (Oikkonen, J. and Väänänen, J., editors), Springer-Verlag, Berlin, pp. 250–258.Google Scholar
Mourgues, Marie-Hélène and Ressayre, Jean-Pierre [1993], Every real closed field has an integer part, The Journal of Symbolic Logic, vol. 58, pp. 641–647.Google Scholar
Ostrowski, Alexander [1935], Untersuchungen zur arithmetischen Theorie der Körper, Mathematische Zeitschrift, vol. 39, pp. 269–404.Google Scholar
Peirce, Charles Sanders [circa 1897], Multitude and continuity, in The new elements of mathematics, vol. III.1 by Peirce, Charles S., (Eisele, C., editor), Moulton Publishers, The Hague, 1976, pp. 82–100.Google Scholar
Peirce, Charles Sanders [1898], The logic of relatives, Reasoning and the logic of things: The Cambridge conferences lectures of 1898 (Ketner, Kenneth Laine, editor), with an introduction by Ketner, Kenneth Laine and Putnam, Hilary. Cambridge, MA, Harvard University Press, 1992, pp. 146–164.Google Scholar
Peirce, Charles Sanders [1898a], The logic of continuity, Reasoning and the logic of things: The Cambridge conferences lectures of 1898 (Ketner, Kenneth Laine, editor), with an introduction by Ketner, Kenneth Laine and Putnam, Hilary. Cambridge, MA, Harvard University Press, 1992, pp. 242–268.Google Scholar
Peirce, Charles Sanders [1900], Infinitesimals, Science, vol. 2, pp. 430–433, reprinted in Collected papers of Charles Sanders Peirce , vol. III, (Charles Hartshone and Paul Weiss, editors), Cambridge, MA, Harvard University Press, 1935, pp. 360–365.Google Scholar
Pincherle, Salvatore [1884], Alcune osservazioni sugli ordini d'infinito delle funzioni, Memorie della R. Accademia delle scienze dell'istituto di Bologna, vol. 5, pp. 739–750.Google Scholar
Plotkin, J. M. (editor) [2005], Hausdorff on ordered sets, American Mathematical Society, Rhode Island.Google Scholar
Priess-Crampe, Sibylla [1973], Zum Hahnschen Einbettungssatz für Angeordnete Körper, Archiv der Mathematik, vol. 24, pp. 607–614.Google Scholar
Priess-Crampe, Sibylla [1983], Angeordnete Strukturen, Gruppen, Körper, projektive Ebenen, Springer-Verlag, Berlin.Google Scholar
Priess-Crampe, Sibylla and von Chossy, Reiner [1975], Ordungsverträgliche Bewertungen eines angeordneten Körpers, Archiv der Mathematik, vol. 26, pp. 373–387.Google Scholar
Rayner, Francis J. [1976], Ordered fields, Seminaire de Théorie des Nombres 1975–1976 (Univ. Bordeaux I. Talence), Exp. No. 1, pp. 1–8 Lab. Théorie des Nombres, Centre Nat. Recherche Sci., Talence.Google Scholar
Reinhardt, William [1970], Ackermann's set theory equals ZF, Annals of Mathematical Logic, vol. 2, pp. 189–259.Google Scholar
Robinson, Abraham [1961], Non-standard analysis, Proceedings of the Royal Academy of Sciences, Amsterdam (Series A), vol. 64, pp. 432–440, reprinted in Abraham Robinson selected papers , vol. 2, Nonstandard analysis and philosophy, Yale University Press, New Haven and London, 1979, pp. 3–11.Google Scholar
Robinson, Abraham [1966], Non-standard analysis, second (1974) ed., North-Holland Publishing Company, Amsterdam.Google Scholar
Robinson, Abraham [1972], On the real closure of a Hardy field, in Theory of sets and topology (in honour of Felix Hausdorff, 1868–1942), pp. 427–433. VEB Deutscher Verlag der Wissenschaften, Berlin, 1972, reprinted in Abraham Robinson selected papers , vol. 1, Model theory and algebra, Yale University Press, New Haven and London, 1979, pp. 607–613.Google Scholar
Rosenlicht, Maxwell [1983], Hardy fields, Journal of Mathematical Analysis and Applications, vol. 93, pp. 297–311.Google Scholar
Schmieden, Curt and Laugwitz, Detlef [1958], Eine Erweiterung der Infinitesimalrechnung, Mathematische Zeitschrift, vol. 69, pp. 1–39.Google Scholar
Sikorski, Roman [1948], On an ordered algebraic field, Comptes Rendus des Séances de la Classe III, Sciences Mathématiques et Physiques. La Société des Sciences et des Lettres de Varsovie, vol. 41, pp. 69–96.Google Scholar
Sjödin, Gunnar [1971], Hardy-fields, Arkiv für Matematik, vol. 8, pp. 217–237.Google Scholar
Skolem, Thoralf [1934], Über die Nichtcharakterisierbarkeit der Zahlenreihe mittels endlich oder abzaählbar unendlich vieler Aussagen mit ausschliesslich Zahlenvariablen, Fundamenta Mathematica, vol. 23, pp. 150–161, reprinted in Selected works in logic , by T. H. Skolem, (J. E. Fenstad, editor), Universitetsforlaget, Oslo, 1970, pp. 355–366.Google Scholar
Stolz, Otto [1879], Ueber die Grenzwerthe der Quotienten, Mathematische Annalen, vol. 14, pp. 231–240.Google Scholar
Stolz, Otto [1883], Zur Geometrie der Alten, insbesondere über ein Axiom des Archimedes, Mathematische Annalen, vol. 22, pp. 504–519.Google Scholar
Stolz, Otto [1885], Vorlesungen über Allgemeine Arithmetik, Erster Theil: Allgemeines und Arithmetik der Reelen Zahlen, Teubner, Leipzig.Google Scholar
van den Dries, Lou [1991], Truncation of power series (remarks on a manuscript by Ressayre), unpublished.Google Scholar
van den Dries, Lou and Ehrlich, Philip [2001], Fields of surreal numbers and exponentiation, Fundamenta Mathematicae, vol. 167, no. 2, pp. 173–188; erratum, Lou van den Dries and Philip Ehrlich [2001], Fields of surreal numbers and exponentiation, Fundamenta Mathematicae, vol. 168 (2001), no. 2, pp. 295–297.Google Scholar
van den Dries, Lou, Macintyre, Angus, and Marker, David [1994], The elementary theory of restricted analytic fields with exponentiation, Annals of Mathematics, vol. 140, pp. 183–205.Google Scholar
van den Dries, Lou, Macintyre, Angus, and Marker, David [1997], Logarithmic-exponential power series, Journal of the London Mathematical Society. Second Series, vol. 56, pp. 417–434.Google Scholar
Veronese, Giuseppe [1891], Fondamenti di geometria a più dimensioni e a più specie di unità rettilinee esposti in forma elementare, Tipografia del Seminario, Padova.Google Scholar
Veronese, Giuseppe [1894], Grundzüge der Geometrie von mehreren Dimensionen und mehreren Arten gradliniger Einheiten in elementarer Form entwickelt, Mit Genehmigung des Verfassers nach einer neuen Bearbeitung des Originals übersetzt von Adolf Schepp, Teubner, Leipzig.Google Scholar