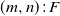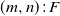444 results
Bivariate tempered space-fractional Poisson process and shock models
- Part of
-
- Journal:
- Journal of Applied Probability , First View
- Published online by Cambridge University Press:
- 23 May 2024, pp. 1-17
-
- Article
- Export citation
Replication studies in engineering design – a feasibility study
-
- Journal:
- Proceedings of the Design Society / Volume 4 / May 2024
- Published online by Cambridge University Press:
- 16 May 2024, pp. 115-124
-
- Article
-
- You have access
- Open access
- Export citation
Durability as a techno-socio-economic concept
-
- Journal:
- Proceedings of the Design Society / Volume 4 / May 2024
- Published online by Cambridge University Press:
- 16 May 2024, pp. 1219-1228
-
- Article
-
- You have access
- Open access
- Export citation
Validation of the Spanish translation Sheffield Profile for Assessment and Referral for Care (SPARC-Sp) at the Hospital Universitario San Jose of Popayan, Colombia
-
- Journal:
- Palliative & Supportive Care , First View
- Published online by Cambridge University Press:
- 27 March 2024, pp. 1-12
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
The Free-Riding Issue in Contemporary Organizations: Lessons from the Common Good Perspective
-
- Journal:
- Business Ethics Quarterly , First View
- Published online by Cambridge University Press:
- 25 March 2024, pp. 1-26
-
- Article
-
- You have access
- HTML
- Export citation
Development of Flood Preparedness Behavior Scale: A Methodological Validity and Reliability Study
-
- Journal:
- Prehospital and Disaster Medicine / Volume 39 / Issue 2 / April 2024
- Published online by Cambridge University Press:
- 25 March 2024, pp. 123-130
- Print publication:
- April 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Validation of the Japanese version of the Social Functioning in Dementia scale and COVID-19 pandemic’s impact on social function in mild cognitive impairment and mild dementia
-
- Journal:
- International Psychogeriatrics , First View
- Published online by Cambridge University Press:
- 11 March 2024, pp. 1-14
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Reliability assessment of off-policy deep reinforcement learning: A benchmark for aerodynamics
-
- Journal:
- Data-Centric Engineering / Volume 5 / 2024
- Published online by Cambridge University Press:
- 25 January 2024, e2
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Turkish adaptation, validation and reliability of the US Adult Food Security Survey Module in university students
-
- Journal:
- Public Health Nutrition / Volume 27 / Issue 1 / 2024
- Published online by Cambridge University Press:
- 22 January 2024, e45
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Chapter 1 - Introduction
-
- Book:
- Applying Benford's Law for Assessing the Validity of Social Science Data
- Published online:
- 09 November 2023
- Print publication:
- 23 November 2023, pp 1-10
-
- Chapter
-
- You have access
- HTML
- Export citation
Palliative care and spiritual care competency measurement among Turkish Nurses: A scale adaptation study
-
- Journal:
- Palliative & Supportive Care / Volume 21 / Issue 6 / December 2023
- Published online by Cambridge University Press:
- 06 November 2023, pp. 1048-1058
-
- Article
- Export citation
Postpartum family planning attitudes among Turkish women: development of a reliable and valid scale
-
- Journal:
- Primary Health Care Research & Development / Volume 24 / 2023
- Published online by Cambridge University Press:
- 18 October 2023, e59
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Safe and valid? A systematic review of the psychometric properties of culturally adapted depression scales for use among Indigenous populations
-
- Journal:
- Cambridge Prisms: Global Mental Health / Volume 10 / 2023
- Published online by Cambridge University Press:
- 14 September 2023, e60
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Reliability analyses of linear two-dimensional consecutive k-type systems
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 61 / Issue 2 / June 2024
- Published online by Cambridge University Press:
- 14 August 2023, pp. 439-464
- Print publication:
- June 2024
-
- Article
- Export citation
Asymptotic behaviors of aggregated Markov processes
-
- Journal:
- Probability in the Engineering and Informational Sciences / Volume 38 / Issue 2 / April 2024
- Published online by Cambridge University Press:
- 25 July 2023, pp. 299-317
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
The future of diagnosis in clinical neurosciences: Comparing multiple sclerosis and schizophrenia
-
- Journal:
- European Psychiatry / Volume 66 / Issue 1 / 2023
- Published online by Cambridge University Press:
- 21 July 2023, e58
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Darwin's doubt or Plantinga's conviction? Some failures in Plantinga's attempt to debunk naturalistic evolution
-
- Journal:
- Religious Studies , First View
- Published online by Cambridge University Press:
- 12 July 2023, pp. 1-16
-
- Article
- Export citation
8 - Moral Perception and Singular Moral Judgment
- from Part III - Moral Knowledge and Normative Realism
-
- Book:
- Of Moral Conduct
- Published online:
- 08 June 2023
- Print publication:
- 22 June 2023, pp 170-184
-
- Chapter
- Export citation
RELIABILITY ANALYSIS FOR SENSOR NETWORKS AND THEIR DATA ACQUISITION: A SYSTEMATIC LITERATURE REVIEW
-
- Journal:
- Proceedings of the Design Society / Volume 3 / July 2023
- Published online by Cambridge University Press:
- 19 June 2023, pp. 3065-3074
-
- Article
-
- You have access
- Open access
- Export citation
Chapter 2 - The History of Shaken Baby Syndrome
- from Section 1 - Prologue
-
-
- Book:
- Shaken Baby Syndrome
- Published online:
- 07 June 2023
- Print publication:
- 08 June 2023, pp 11-32
-
- Chapter
- Export citation