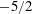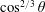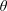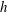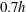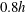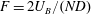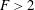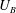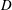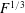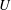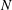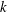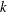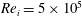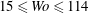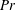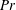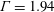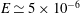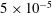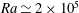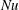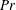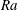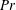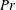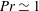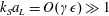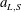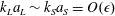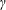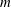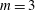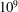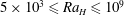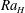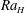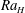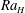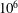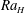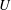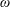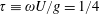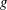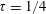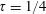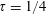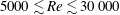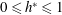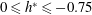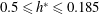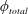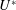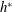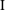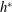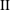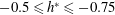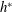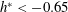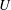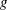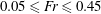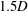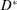Diving induces large pressure during water entry accompanied by the creation of cavity and water splash ejected from the free water surface. To minimize impact forces, divers streamline their shape at impact. Here, we investigate the impact forces and splash evolution of wedges entering water as a function of the wedge opening angle. A gradual transition from impactful to smooth entry is observed as the wedge angle decreases. After submersion, the wedge experiences significantly smaller drag forces (two-fold smaller) than immersed wedges. Our experimental findings compare favourably with existing force models upon the introduction of empirically based corrections. We experimentally characterize the shapes of the cavity and splash created by the wedge and find that they are independent of the entry velocity at short times, but that the splash exhibits distinct variations in shape at later times. We propose a one-dimensional model of the splash that takes into account gravity, surface tension and aerodynamic forces. The model shows, in conjunction with experimental data, that the splash shape is dominated by the interplay between a destabilizing Venturi-suction force due to air rushing between the splash and the water surface and a stabilizing force due to surface tension. Taken together, these findings could direct future research aimed at understanding and combining the mechanisms underlying all stages of water entry in application to engineering and bio-related problems, including naval engineering, disease spreading or platform diving.

We present an analysis that predicts the time to development of a singularity in the shape profile of a spatially periodic perturbed, planar shock wave for ideal gas dynamics. Beginning with a formulation in complex coordinates of Whitham’s approximate model geometrical shock dynamics (GSD), we apply a spectral treatment to derive the asymptotic form for the leading-order behaviour of the shock Fourier coefficients for large mode numbers and time. This is shown to determine a critical time at which the coefficients begin to decay, with respect to mode number, at an algebraic rate with an exponent of $-5/2$ , indicating loss of analyticity and the formation of a singularity in the shock geometry. The critical time is found to be inversely proportional to a representative measure of perturbation amplitude $\unicode[STIX]{x1D716}$ with an explicit analytic form for the constant of proportionality in terms of gas and shock parameters. To leading order, the time to singularity formation is dependent only on the first Fourier mode. Comparison with results of numerical solutions to the full GSD equations shows that the predicted critical time somewhat underestimates the time for shock–shock (triple-point) formation, where the latter is obtained by post-processing the numerical GSD results using an edge-detection algorithm. Aspects of the analysis suggest that the appearance of loss of analyticity in the shock surface may be a precursor to the first appearance of shock–shocks, which may account for part of the discrepancy. The frequency of oscillation of the shock perturbation is accurately predicted. In addition, the analysis is extended to the evolution of a perturbed planar, fast magnetohydrodynamic shock for the case when the external magnetic field is aligned parallel to the unperturbed shock. It is found that, for a strong shock, the presence of the magnetic field produces only a higher-order correction to the GSD equations with the result that the time to loss of analyticity is the same as for the gas-dynamic flow. Limitations and improvements for the analysis are discussed, as are comparisons with the analogous appearance of singularity formation in vortex-sheet evolution in an incompressible inviscid fluid.

We examine the dissolution of a sloping solid surface driven by turbulent compositional convection. The scaling analysis presented by Kerr & McConnochie (J. Fluid Mech., vol. 765, 2015, pp. 211–228) for the dissolution of a vertical wall is extended to the case of a sloping wall. The model has no free parameters and no dependence on height. It predicts that while the interfacial temperature and interfacial composition are independent of the slope, the dissolution velocity is proportional to $\cos ^{2/3}\unicode[STIX]{x1D703}$ , where $\unicode[STIX]{x1D703}$ is the angle of the sloping surface to the vertical. The analysis is tested by comparing it with laboratory measurements of the ablation of a sloping ice wall in contact with salty water. We apply the model to make predictions of the turbulent convective dissolution of a sloping ice shelf in the polar oceans.

The secondary instability in the wake of a two-dimensional blunt body with a chord to thickness ratio of 46.5 was experimentally investigated for Reynolds numbers of 3500, 5200 and 7000 based on the blunt trailing edge height $h$ . Planar, stereoscopic and high-speed particle image velocimetry (PIV) measurements were performed to characterise the wake and upstream boundary layer. The same mode B secondary instability that is found in the cylinder wake was found to be present in the wake of the elongated body studied here. The most probable wavelength of the secondary instability, defined as the spanwise distance between adjacent streamwise vortex pairs in the wake, was found to range from $0.7h$ to $0.8h$ by applying a spatial autocorrelation to the spanwise–wall-normal instantaneous fields of the $Q$ -criterion. The temporal evolution of the secondary wake vortices was investigated using time-resolved stereoscopic PIV measurements and it was shown that the vortices maintain both their directions of rotation and spanwise positions during the primary vortex shedding cycles. In agreement with previous literature, the secondary instability did not greatly change as the upstream boundary layer transitioned from laminar to turbulent. Moreover, any upstream boundary layer structures were found to rapidly evolve into wake structures just past the blunt trailing edge. The wavelength of the secondary instability was shown to match the spanwise distance between adjacent low-speed zones of streamwise velocity in the wake. These undulating velocity patterns proved to be a viable method for determining the secondary instability wavelength; however, this type of analysis is highly sensitive to the energy content used for data reconstruction when proper orthogonal decomposition is applied beforehand.

A combined experimental and numerical approach to the analysis of the secondary stability of realistic swept-wing boundary layers is presented. Global linear stability theory is applied to experimentally measured base flows. These base flows are three-dimensional laminar boundary layers subject to spanwise distortion due to the presence of primary stationary crossflow vortices. A full three-dimensional description of these flows is accessed through the use of tomographic particle image velocimetry (PIV). The stability analysis solves for the secondary high-frequency modes of type I and type II, ultimately responsible for turbulent breakdown. Several pertinent parameters arising from the application of the proposed methodology are investigated, including the mean flow ensemble size and the measurement domain extent. Extensive use is made of the decomposition of the eigensolutions into the terms of the Reynolds–Orr equation, allowing insight into the production and/or destruction of perturbations from various base flow features. Stability results demonstrate satisfactory convergence with respect to the mean flow ensemble size and are independent of the handling of the exterior of the measurement domain. The Reynolds–Orr analysis reveals a close relationship between the type I and type II instability modes with spanwise and wall-normal gradients of the base flow, respectively. The structural role of the in-plane velocity components in the perturbation growth, topology and sensitivity is identified. Using the developed framework, further insight is gained into the linear growth mechanisms and later stages of transition via the primary and secondary crossflow instabilities. Furthermore, the proposed methodology enables the extension and enhancement of the experimental measurement data to the pertinent instability eigenmodes. The present work is the first demonstration of the use of a measured base flow for stability analysis applied to the swept-wing boundary layer, directly avoiding the modelling of the primary vortices receptivity processes.

The steady speeds of the front of a gravity current and of an internal jump on a two-layer stratification are often sought in terms of the heights of the relatively dense fluid both up- and downstream from the front or jump, the height of the channel within which they flow, the densities of the two fluids and gravitational acceleration. In this study a unifying framework is presented for calculating the speeds by balancing mass and momentum fluxes across a control volume spanning the front or jump and by ensuring the assumed pressure field is single-valued, which is shown to be equivalent to forming a vorticity balance over the control volume. Previous models have assumed the velocity field is piecewise constant in each layer with a vortex sheet at their interface and invoked explicit or implicit closure assumptions about the dissipative effects to derive the speed. The new formulation yields all of the previously presented expressions and demonstrates that analysing the vorticity balance within the control volume is a useful means of constraining possible closure assumptions, which is arguably more effective than consideration of the flow energetics. However the new approach also reveals that a novel class of models may be developed in which there is shear in the velocity field in the wake downstream of the front or the jump, thus spreading the vorticity over a layer of non-vanishing thickness, rather than concentrating it into a vortex sheet. Mass, momentum and vorticity balances applied over the control volume allow the thickness of the wake and the speed of the front/jump to be evaluated. Results from this vortex-wake model are consistent with published numerical simulations and with data from laboratory experiments, and improve upon predictions from previous formulae. The results may be applied readily to Boussinesq and non-Boussinesq systems and because they arise as simple algebraic expressions, can be straightforwardly incorporated as jump conditions into spatially and temporally varying descriptions of the motion.

We use continuum conservation equations in conjunction with the entropy inequality to obtain a theoretical model that is accurate for hypersonic flows. This is accomplished by extending the constitutive equations to second order in strain rate and gradients of density and temperature. The accuracy of the extended theory is demonstrated by computing the shock structure in argon and nitrogen gases over a large range of Mach numbers. The solutions indicate that our constitutive equations are more accurate than the classical linear ones and are also more robust and accurate than equations obtained through expansions of the Boltzmann equation or equations arising from diffusion of volume arguments.

A hierarchy of models is formulated for the deflection of a thin two-dimensional liquid jet as it passes over a thin air-cushioning layer above a rigid flat impermeable substrate. We perform a systematic derivation of the leading-order equations of motion for the jet in the distinguished limit in which the air pressure jump, surface tension and gravity affect the displacement of the centreline of the jet, but not its thickness or velocity. We identify thereby the axial length scales for centreline deflection in regimes in which the air layer is dominated by viscous or inertial effects. The derived length scales and reduced equations aim to expand the suite of tools available for future analyses of the evolution of lamellae and ejecta in impact problems. Assuming that the jet is sufficiently long that tip and entry effects can be neglected, we demonstrate that the centreline of a constant-thickness jet moving with constant axial speed is destabilised by the air layer for sufficiently small surface tension. Expressions for the fastest-growing modes are obtained in both the viscous-dominated air and inertia-dominated air regimes. For a finite-length jet emanating from a nozzle, we show that, in one particular asymptotic limit, the evolution of the jet centreline is akin to the flapping of an unfurling flag above a thin air layer. We discuss the distinguished limit in which tip retraction can be neglected and perform numerical investigations into the resulting model. We show that the cushioning layer causes the jet centreline to bend, leading to rupture of the air layer. We discuss how our toolbox of models can be adapted and utilised in the context of recent experimental and numerical studies of splash dynamics.

This paper presents experimental and theoretical results on the internal waves emitted by a bluff body moving horizontally in a linearly stratified fluid. Three different bluff bodies (a sphere, a spheroid and a cylinder) have been used in order to study the effect of the shape of the bluff body, although most of the results are obtained for the sphere. Two types of internal waves have been observed experimentally: large wavelength lee waves generated by the bluff body itself and small wavelength coherent wake waves generated by the turbulent wake. First, the lee waves are separated from the wake waves by averaging the experimental measurements in the frame moving with the bluff body. The velocity amplitude of the lee waves scales as the inverse of the Froude number $F=2U_{B}/(ND)$ for $F>2$ (where $U_{B}$ is the towing velocity, $D$ the diameter and $N$ the buoyancy frequency). This scaling proves that the internal waves are related to the drag of the bluff body which is due to the separation of the flow behind the bluff body. This separation is usually not taken into account in the classical models which assume that the flow is dipolar. The drag can be modelled as a point force in the Navier–Stokes equations, which gives a correct prediction of the structure and the amplitude of the lee waves. Second, the wake waves have been separated from the lee waves by averaging the velocity fields in the frame moving at the phase velocity of the waves. The phase velocity and the wavelength scale as $F^{-2/3}$ and $F^{1/3}$ respectively which correspond to the velocity and distance between same sign vortices of the von Kármán vortex street. A simplified model is derived for the internal waves emitted by the double row of moving point vortices of the von Kármán street. The amplitude of the wake waves is measured experimentally and seems to depend on the Reynolds number.

Realistic flight vehicles for reentry into the Earth’s atmosphere are commonly similar to blunted cones. The main reason for blunting a cone is to mitigate high heat loads at the nose. Another reason for blunting the cone is to delay boundary-layer transition. It is commonly understood that the second mode is damped in flow over a cone as the nose radius is increased. This is thought to lead to the delay in transition. Here, a blunted cone at a realistic reentry trajectory point with significant real-gas effects is studied. It is shown, using linear stability theory and direct numerical simulation, that there exist multiple unstable modal instabilities in the boundary layer. One of these modal instabilities is called the supersonic mode, as its phase velocity is supersonic relative to the flow velocity at the edge of the boundary layer. Its growth rate is found to increase with increasing nose radius until a certain nose radius is reached. After this radius, any further increase in nose radius decreases its growth rate. There is adequate agreement between theory and direct numerical simulation for the growth rate, phase velocity and eigenfunction of the supersonic mode. At the reentry conditions tested, the supersonic mode is more likely the cause of boundary-layer transition than the second mode for blunted cones with a small wall-temperature ratio. Initial parametric studies confirm that a decrease in wall temperature amplifies the supersonic mode. Also, the supersonic mode’s growth rate is shown to be a maximum when its phase velocity is aligned with the flow velocity.

Strato-rotational instability (SRI) is normally interpreted as the resonant interactions between normal modes of the internal or Kelvin variety in three-dimensional settings in which the stratification and rotation are orthogonal to both the background flow and its shear. Using a combination of asymptotic analysis and numerical solution of the linear eigenvalue problem for plane Couette flow, it is shown that such resonant interactions can be destroyed by certain singular critical levels. These levels are not classical critical levels, where the phase speed $c$ of a normal mode matches the mean flow speed $U$ , but are a different type of singularity where $(c-U)$ matches a characteristic gravity-wave speed $\pm N/k$ , based on the buoyancy frequency $N$ and streamwise horizontal wavenumber $k$ . Instead, it is shown that a variant of SRI can occur due to the coupling of a Kelvin or internal wave to such ‘baroclinic’ critical levels. Two characteristic situations are identified and explored, and the conservation law for pseudo-momentum is used to rationalize the physical mechanism of instability. The critical level coupling removes the requirement for resonance near specific wavenumbers $k$ , resulting in an extensive continuous band of unstable modes.

We study periodically driven Taylor–Couette turbulence, i.e. the flow confined between two concentric, independently rotating cylinders. Here, the inner cylinder is driven sinusoidally while the outer cylinder is kept at rest (time-averaged Reynolds number is $Re_{i}=5\times 10^{5}$ ). Using particle image velocimetry, we measure the velocity over a wide range of modulation periods, corresponding to a change in Womersley number in the range $15\leqslant Wo\leqslant 114$ . To understand how the flow responds to a given modulation, we calculate the phase delay and amplitude response of the azimuthal velocity. In agreement with earlier theoretical and numerical work, we find that for large modulation periods the system follows the given modulation of the driving, i.e. the behaviour of the system is quasi-stationary. For smaller modulation periods, the flow cannot follow the modulation, and the flow velocity responds with a phase delay and a smaller amplitude response to the given modulation. If we compare our results with numerical and theoretical results for the laminar case, we find that the scalings of the phase delay and the amplitude response are similar. However, the local response in the bulk of the flow is independent of the distance to the modulated boundary. Apparently, the turbulent mixing is strong enough to prevent the flow from having radius-dependent responses to the given modulation.

Earth’s magnetic field is generated by convective motions in its liquid metal core. In this fluid, the heat diffuses significantly more than momentum, and thus the Prandtl number $Pr$ is well below unity. The thermally driven convective flow dynamics of liquid metals are very different from moderate- $Pr$ fluids, such as water and those used in current dynamo simulations. In order to characterise rapidly rotating thermal convection in low- $Pr$ number fluids, we have performed laboratory experiments in a cylinder of aspect ratio $\unicode[STIX]{x1D6E4}=1.94$ using liquid gallium ( $Pr\simeq 0.025$ ) as the working fluid. The Ekman number varies from $E\simeq 5\times 10^{-6}$ to $5\times 10^{-5}$ and the Rayleigh number varies from $Ra\simeq 2\times 10^{5}$ to $1.5\times 10^{7}$ . Using spectral analysis stemming from point-wise temperature measurements within the fluid and measurements of the Nusselt number $Nu$ , we characterise the different styles of low- $Pr$ rotating convective flow. The convection threshold is first overcome in the form of container-scale inertial oscillatory modes. At stronger forcing, sidewall-attached modes are identified for the first time in liquid metal laboratory experiments. These wall modes coexist with the bulk oscillatory modes. At $Ra$ well below the values where steady rotating columnar convection occurs, the bulk flow becomes turbulent. Our results imply that rotating convective flows in liquid metals do not develop in the form of quasisteady columns, as in moderate- $Pr$ fluids, but in the form of oscillatory convective motions. Thus, thermally driven flows in low- $Pr$ geophysical and astrophysical fluids can differ substantively from those occurring in $Pr\simeq 1$ models. Furthermore, our experimental results show that relatively low-frequency wall modes are an essential dynamical component of rapidly rotating convection in liquid metals.

We analyse the effect of surface viscoelasticity on the temporal stability of a free cylindrical liquid jet coated with insoluble surfactant, extending the results of Timmermans & Lister (J. Fluid Mech., vol. 459, 2002, pp. 289–306). Our development requires, in particular, deriving the correct expressions for the normal and tangential stress boundary conditions at a general axisymmetric interface when surface viscosity is modelled with the Boussinesq–Scriven constitutive equation. These stress conditions are applied to obtain a new dispersion relation for the liquid thread, which is solved to describe its temporal stability as a function of four governing parameters, namely the capillary Reynolds number, the elasticity parameter, and the shear and dilatational Boussinesq numbers. It is shown that both surface viscosities have a stabilising influence for all values of the capillary Reynolds number and elasticity parameter, the effect being more pronounced at low capillary Reynolds numbers. The wavenumber of maximum amplification depends non-monotonically on the Boussinesq numbers, especially for very viscous threads at low values of the elasticity parameter. Finally, two different lubrication approximations of the equations of motion are derived. While the validity of the leading-order model is limited to small enough values of the elasticity parameter and of the Boussinesq numbers, a higher-order parabolic model is able to accurately capture the linearised behaviour for the whole range of values of the four control parameters.

In high-order analysis and simulation of long–short surface wave interaction using mode decomposition, ‘divergent’ terms of the form $k_{S}a_{L}=O(\unicode[STIX]{x1D6FE}\unicode[STIX]{x1D716})\gg 1$ appear in the high-order expansions, where $k_{L,S}$ , $a_{L,S}$ are respectively the long, short modal wavenumbers and amplitudes, with $\unicode[STIX]{x1D6FE}\equiv k_{S}/k_{L}\gg 1$ and $k_{L}a_{L}\sim k_{S}a_{S}=O(\unicode[STIX]{x1D716})$ finite. We address the effect of these terms on the numerical scheme, showing numerical cancellation at all orders $m$ ; but increasing ill-conditioning of the numerics with $\unicode[STIX]{x1D6FE}$ and $m$ , which we quantify. In the context of mode decomposition, we show theoretical exact cancellation of the divergent terms up to $m=3$ , extending the existing result of Brueckner & West (J. Fluid Mech., vol. 196, 1988, pp. 585–592) and supporting the conjecture that this is obtained for all orders $m$ . We show the latter by developing a theoretical proof for any $m$ using a Dirichlet–Neumann operator and mathematical induction. The implication of the theoretical proof on the numerical simulation of long–short wave interaction is discussed.

Liquid jetting and fragmentation are important in many industrial and medical applications. Here, we study the jetting from the surface of single liquid droplets undergoing internal volume oscillations. This is accomplished by an explosively expanding and collapsing vapour bubble within the droplet. We observe jets emerging from the droplet surface, which pinch off into finer secondary droplets. The jetting is excited by the spherical Rayleigh–Taylor instability where the radial acceleration is due to the oscillation of an internal bubble. We study this jetting and the effect of fluid viscosity experimentally and numerically. Experiments are carried out by levitating the droplet in an acoustic trap and generating a laser-induced cavitation bubble near the centre of the droplet. On the simulation side, the volume of fluid method (OpenFOAM) solves the compressible Navier–Stokes equations while accounting for surface tension and viscosity. Both two-dimensional (2-D) axisymmetric and 3-D simulations are performed and show good agreement with each other and the experimental observation. While the axisymmetric simulation reveals how the bubble dynamics results destabilizes the interface, only the 3-D simulation computes the geometrically correct slender jets. Overall, experiments and simulations show good agreement for the bubble dynamics, the onset of disturbances and the rapid ejection of filaments after bubble collapse. Additionally, an analytic model for the droplet surface perturbation growth is developed based on the spherical Rayleigh–Taylor instability analysis, which allows us to evaluate the surface stability over a large parameter space. The analytic model predicts correctly the onset of jetting as a function of Reynolds number and normalized internal bubble energy.

The nonlinear dynamics of waves generated by the deformational oscillatory Marangoni instability in a two-layer film under the action of a two-dimensional temperature modulation on the solid substrate is considered. A system of long-wave equations governing the deformations of the upper surface and the interface between the liquids is presented. The long-wave approach is applied. The nonlinear simulations reveal the existence of different dynamic regimes, including stationary, time-periodic and quasi-periodic flows. The general diagrams of the flow regimes are constructed.

Motions in the solid mantle of silicate planets are predominantly driven by internal heat sources and occur in laminar regimes that have not been systematically investigated. Using high-resolution numerical simulations conducted in three dimensions for a large range of Rayleigh–Roberts numbers ( $5\times 10^{3}\leqslant Ra_{H}\leqslant 10^{9}$ ), we have determined the characteristics of flow in internally heated fluid layers with both rigid and free slip boundaries. Superficial planforms evolve with increasing $Ra_{H}$ from a steady-state tessellation of hexagonal cells with axial downwellings to time-dependent clusters of thin linear downwellings within large areas of nearly isothermal fluid. The transition between the two types of planforms occurs as a remarkable flow polarity reversal over a small $Ra_{H}$ range, such that downwellings go from isolated cylindrical structures encircled by upwellings to thin interconnected linear segments outlining polygonal cells. In time-dependent regimes at large values of $Ra_{H}$ , linear downwellings dominate the flow field at shallow depth but split and merge at intermediate depths into nearly cylindrical plume-like structures that go through the whole layer. With increasing $Ra_{H}$ , the number of plumes per unit area and their velocities increase whilst the amplitude of thermal anomalies decreases. Scaling laws for the main flow characteristics are derived for $Ra_{H}$ values in a $10^{6}$ – $10^{9}$ range. For given $Ra_{H}$ , plumes are significantly colder, narrower and wider apart beneath free boundaries than beneath rigid ones. From the perspective of planetary studies, these results alert to the dramatic changes of convective planform that can occur along secular cooling.

We study theoretically and numerically the nonlinear seakeeping problem of a submerged or floating body translating with constant forward speed $U$ parallel to the undisturbed free surface while at the same time undergoing a small oscillatory motion and/or encountering small-amplitude waves at frequency $\unicode[STIX]{x1D714}$ . It is known that at the critical frequency corresponding to $\unicode[STIX]{x1D70F}\equiv \unicode[STIX]{x1D714}U/g=1/4$ , where $g$ is the gravitational acceleration, the classical linear solution is unbounded for a single point source, and the inclusion of third-order free-surface nonlinearity due to cubic self-interactions of waves is necessary to remove the associated singularity. Although it has been shown that the linear solution is in fact bounded for a body with full geometry rather than a point source, the solution still varies sharply near the critical frequency. In this work, we show theoretically that for a submerged body, the nonlinear correction to the linear solution due to cubic self-interactions of resonant waves in the neighbourhood of $\unicode[STIX]{x1D70F}=1/4$ is of first order in the wave steepness (or body motion amplitude), which is the same order as the linear solution. With the inclusion of nonlinear effects in the dispersion relation, the wavenumbers of resonant waves become complex-valued and the resonant waves become evanescent, with their amplitudes vanishing with the distance away from the body. To assist in understanding the theory, we derive the analytic nonlinear solution for the case of a submerged two-dimensional circular cylinder in the neighbourhood of $\unicode[STIX]{x1D70F}=1/4$ . Independent numerical simulations confirm the analytic solution for the submerged circular cylinder. Finally, we also demonstrate by numerical simulations that similar significant nonlinear effects for a surface-piercing body exist in the neighbourhood of $\unicode[STIX]{x1D70F}=1/4$ .

Results are presented from an experimental investigation into the effects of proximity to a free surface on vortex-induced vibration (VIV) experienced by fully and semi-submerged spheres that are free to oscillate in the cross-flow direction. The VIV response is studied over a wide range of reduced velocities: $3\leqslant U^{\ast }\leqslant 20$ , covering the mode I, mode II and mode III resonant response branches and corresponding to the Reynolds number range of 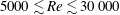$5000\lesssim Re\lesssim 30\,000$ . 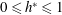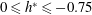The normalised immersion depth of the sphere is varied in small increments over the range $0\leqslant h^{\ast }\leqslant 1$ for the fully submerged case and $0\leqslant h^{\ast }\leqslant -0.75$ for the semi-submerged case. It is found that for a fully submerged sphere, the vibration amplitude decreases monotonically and gradually as the immersion ratio is decreased progressively, with a greater influence on the mode II and III parts of the response curve. The synchronisation regime becomes narrower as $h^{\ast }$  is decreased, with the peak saturation amplitude occurring at progressively lower reduced velocities. The peak response amplitude decreases almost linearly over the range of 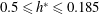$0.5\leqslant h^{\ast }\leqslant 0.185$ , beyond which the peak response starts increasing almost linearly. The trends in the total phase, 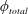$\unicode[STIX]{x1D719}_{total}$ , and the vortex phase, $\unicode[STIX]{x1D719}_{vortex}$ , reveal that the mode II response occurs for progressively lower 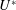$U^{\ast }$ values with decreasing 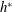$h^{\ast }$ . On the other hand, when the sphere pierces the free surface, there are two regimes with different characteristic responses. 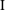In regime $\text{I}$ ( $-0.5<h^{\ast }<0$ ), the synchronisation region widens and the vibration amplitude increases, surprisingly becoming even higher than for the fully submerged case in some cases, as 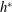$h^{\ast }$ decreases. However, in regime 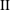$\text{II}$ ( 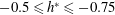$-0.5\leqslant h^{\ast }\leqslant -0.75$ ), the vibration amplitude decreases with a decrease in 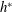$h^{\ast }$ , showing a very sharp reduction beyond 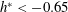$h^{\ast }<-0.65$ . The response in regime II is characterised by two distinct peaks in the amplitude response curve. Careful analysis of the force data and phase information reveals that the two peaks correspond to modes I and II seen for the fully submerged vibration response. This two-peak behaviour is different to the classic VIV response of a sphere under one degree of freedom (1-DOF). The response was found to be insensitive to the Froude number ( $Fr=U/\sqrt{gD}$ , where 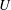$U$ is the free-stream velocity, $D$ is the sphere diameter and 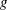$g$ is the acceleration due to gravity) in the current range of 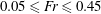$0.05\leqslant Fr\leqslant 0.45$ , although higher Froude numbers resulted in slightly lower peak response amplitudes. The wake measurements in the cross-plane 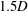$1.5D$ downstream of the rear of the sphere reveal a reduction in the vorticity of the upper vortex of the trailing vortex pair, presumably through diffusion of vorticity into the free surface. For the piercing sphere case, the near-surface vorticity completely diffuses into the free surface, with only the opposite-signed vortex visible in the cross-plane at this downstream position. Interestingly, this correlates with an even higher oscillation amplitude than the fully submerged case. Finally, the effects of immersion ratio and diameter ratio ( 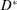$D^{\ast }$   $=$  sphere diameter/support-rod diameter) are quantified, showing care needs to be taken with these factors to avoid unduly influencing VIV predictions.