We construct cocompact lattices Γ'0 < Γ0 in the group G = PGLd$({\mathbb{F}_q(\!(t)\!)\!})$ which are type-preserving and act transitively on the set of vertices of each type in the building Δ associated to G. These lattices are commensurable with the lattices of Cartwright–Steger Isr. J. Math.103 (1998), 125–140. The stabiliser of each vertex in Γ'0 is a Singer cycle and the stabiliser of each vertex in Γ0 is isomorphic to the normaliser of a Singer cycle in PGLd(q). We show that the intersections of Γ'0 and Γ0 with PSLd$({\mathbb{F}_q(\!(t)\!)\!})$ are lattices in PSLd$({\mathbb{F}_q(\!(t)\!)\!})$, and identify the pairs (d, q) such that the entire lattice Γ'0 or Γ0 is contained in PSLd$({\mathbb{F}_q(\!(t)\!)\!})$. Finally we discuss minimality of covolumes of cocompact lattices in SL3$({\mathbb{F}_q(\!(t)\!)\!})$. Our proofs combine the construction of Cartwright–Steger Isr. J. Math.103 (1998), 125–140 with results about Singer cycles and their normalisers, and geometric arguments.

We define model structures on a triangulated category with respect to some proper classes of triangles and give a general study of triangulated model structures. We look at the relationship between these model structures and cotorsion pairs with respect to a proper class of triangles on the triangulated category. In particular, we get Hovey's one-to-one correspondence between triangulated model structures and complete cotorsion pairs with respect to a proper class of triangles. Some applications are given.

We present a construction of framed torsion free instanton sheaves on a projective variety containing a fixed line which further generalises the one on projective spaces. This is done by generalising the so called ADHM variety. We show that the moduli space of such objects is a quasi projective variety, which is fine in the case of projective spaces. We also give an ADHM categorical description of perverse instanton sheaves in the general case, along with a hypercohomological characterisation of these sheaves in the particular case of projective spaces.

A monoid S satisfies Condition (A) if every locally cyclic left S-act is cyclic. This condition first arose in Isbell's work on left perfect monoids, that is, monoids such that every left S-act has a projective cover. Isbell showed that S is left perfect if and only if every cyclic left S-act has a projective cover and Condition (A) holds. Fountain built on Isbell's work to show that S is left perfect if and only if it satisfies Condition (A) together with the descending chain condition on principal right ideals, MR. We note that a ring is left perfect (with an analogous definition) if and only if it satisfies MR. The appearance of Condition (A) in this context is, therefore, monoid specific. Condition (A) has a number of alternative characterisations, in particular, it is equivalent to the ascending chain condition on cyclic subacts of any left S-act. In spite of this, it remains somewhat esoteric. The first aim of this paper is to investigate the preservation of Condition (A) under basic semigroup-theoretic constructions. Recently, Khosravi, Ershad and Sedaghatjoo have shown that every left S-act has a strongly flat or Condition (P) cover if and only if every cyclic left S-act has such a cover and Condition (A) holds. Here we find a range of classes of S-acts 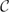$\mathcal{C}$ such that every left S-act has a cover from 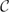$\mathcal{C}$ if and only if every cyclic left S-act does and Condition (A) holds. In doing so we find a further characterisation of Condition (A) purely in terms of the existence of covers of a certain kind. Finally, we make some observations concerning left perfect monoids and investigate a class of monoids close to being left perfect, which we name left$\mathcal{IP}$a-perfect.

Let Y be a compact nonsingular real algebraic variety of positive dimension. Then one can find a compact connected nonsingular real algebraic variety X, which admits a continuous map into Y that is not homotopic to any regular map. It is hard to determine the minimum dimension of such a variety X. In this paper, new upper bounds for dim X are obtained. The main role in the constructions is played by complex algebraic cycles on Y.

Meta-centralizers of non-locally compact group algebras are studied. Theorems about their representations with the help of families of generalized measures are proved. Isomorphisms of group algebras are investigated in relation with meta-centralizers.

We show that the Gromov-Lawson-Rosenberg conjecture for the Semi-Dihedral group of order 16 is true.

We show that two metacyclic groups of the following types are isomorphic if they have the same character tables: (i) split metacyclic groups, (ii) the metacyclic p-groups and (iii) the metacyclic {p, q}-groups, where p, q are odd primes.

We consider higher secant varieties to Veronese varieties. Most points on the rth secant variety are represented by a finite scheme of length r contained in the Veronese variety – in fact, for a general point, the scheme is just a union of r distinct points. A modern way to phrase it is: the smoothable rank is equal to the border rank for most polynomials. This property is very useful for studying secant varieties, especially, whenever the smoothable rank is equal to the border rank for all points of the secant variety in question. In this note, we investigate those special points for which the smoothable rank is not equal to the border rank. In particular, we show an explicit example of a cubic in five variables with border rank 5 and smoothable rank 6. We also prove that all cubics in at most four variables have the smoothable rank equal to the border rank.

Any singular level of a completely integrable system (c.i.s.) with non-degenerate singularities has a singular affine structure. We shall show how to construct a simple c.i.s. around the level, having the above affine structure. The cotangent bundle of the desingularized level is used to perform the construction, and the c.i.s. obtained looks like the simplest one associated to the affine structure. This method of construction is used to provide several examples of c.i.s. with different kinds of non-degenerate singularities.

As a special case of fully invariant subgroups, strongly invariant subgroups are introduced and studied for Abelian groups.

Let R be a complete intersection ring, and let M and N be R-modules. It is shown that the vanishing of ExtiR(M, N) for a certain number of consecutive values of i starting at n forces the complete intersection dimension of M to be at most n–1. We also estimate the complete intersection dimension of M*, the dual of M, in terms of vanishing of cohomology modules, ExtiR(M,N).

In this paper, we first deduce a formula of S-curvature of homogeneous Finsler spaces in terms of Killing vector fields. Then we prove that a homogeneous Finsler space has isotropic S-curvature if and only if it has vanishing S-curvature. In the special case that the homogeneous Finsler space is a Randers space, we give an explicit formula which coincides with the previous formula obtained by the second author using other methods.

Let p be a prime and K a number field of degree p. We determine the finiteness of the number of elliptic curves, up to K-isomorphism, having a prescribed property, where this property is either that the curve contains a fixed torsion group as a subgroup or that it has a cyclic isogeny of prescribed degree.

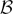We will characterize the boundedness and compactness of weighted composition operators on the closed subalgebra H∞ ∩ $\mathcal{B}$o between the disk algebra and the space of bounded analytic functions on the open unit disk.