Methane (CH4) production is a ubiquitous, apparently unavoidable side effect of fermentative fibre digestion by symbiotic microbiota in mammalian herbivores. Here, a data compilation is presented of in vivo CH4 measurements in individuals of 37 mammalian herbivore species fed forage-only diets, from the literature and from hitherto unpublished measurements. In contrast to previous claims, absolute CH4 emissions scaled linearly to DM intake, and CH4 yields (per DM or gross energy intake) did not vary significantly with body mass. CH4 physiology hence cannot be construed to represent an intrinsic ruminant or herbivore body size limitation. The dataset does not support traditional dichotomies of CH4 emission intensity between ruminants and nonruminants, or between foregut and hindgut fermenters. Several rodent hindgut fermenters and nonruminant foregut fermenters emit CH4 of a magnitude as high as ruminants of similar size, intake level, digesta retention or gut capacity. By contrast, equids, macropods (kangaroos) and rabbits produce few CH4 and have low CH4 : CO2 ratios for their size, intake level, digesta retention or gut capacity, ruling out these factors as explanation for interspecific variation. These findings lead to the conclusion that still unidentified host-specific factors other than digesta retention characteristics, or the presence of rumination or a foregut, influence CH4 production. Measurements of CH4 yield per digested fibre indicate that the amount of CH4 produced during fibre digestion varies not only across but also within species, possibly pointing towards variation in microbiota functionality. Recent findings on the genetic control of microbiome composition, including methanogens, raise the question about the benefits methanogens provide for many (but apparently not to the same extent for all) species, which possibly prevented the evolution of the hosting of low-methanogenic microbiota across mammals.

A semigroup is said to be completely regular if and only if each of its elements lies in a subgroup. It is shown that the algebra of a completely regular monoid (semigroup with identity) over a field of characteristic zero is directly finite.

A semigroup is said to be congruence-free if and only if its only congruences are the universal relation and the identical relation. Congruence-free inverse semigroups were studied by Baird [2], Trotter [19], Munn [15,16] and Reilly [18]. In addition, results on congruence-free regular semigroups have been obtained by Trotter [20], Hall [4] and Howie [7].

The purpose of this note is to extend the results of Reilly and Scheiblich (6) (see also Scheiblich (7) and Hall (2)) on the θ-class decomposition of the congruence lattice of a regular semigroup and, at the same time, to provide an alternative proof of these results.

A congruence ρ on a semigroup is said to be idempotent-separating if each ρ-class contains at most one idempotent. For any idempotent e of a semigroup S the set eSe is a subsemigroup of S with identity e and group of units He, the maximal subgroup of S containing e. The purpose of the present note is to show that if S is a regular O-bisimple semigroup and e is a non-zero idempotent of 5 then there is a one-to-one correspondence between the idempotentseparating congruences on 5 and the subgroups N of He with the property that aN ⊆ Na for all right units a of eSe and Nb ⊆ bN for all left units b of eSe. Some special cases of this result are discussed and, in the final section, an application is made to the principal factors of the full transformation semigroup on a set X.

In this paper the “hypercore” of a semigroup S is defined to be the subsemigroup generated by the union of all the subsemigroups of S without non-universal cancellative congruences, provided that at least one such subsemigroup exists: otherwise it is taken to be the empty set. It is shown first that if the hypercore of S is nonempty (which holds, for example, when S contains an idempotent) then it is the largest subsemigroup of S with no non-universal cancellative congruence, is full and unitary in S, and is contained in the identity class of every group congruence on S (Theorem 1).

Let S be a nontrivial monoid with zero and let F be a field. A sufficient condition, on the 0-simple principal factors of S and the characteristic of F, is given for the contracted monoid algebra of S over F to be directly finite.

It is shown that the complex semigroup algebra of a free monoid of rank at least two is *-primitive, where * denotes the involution on the algebra induced by word-reversal on the monoid.

Let S be a 0-bisimple inverse semigroup and let denote the contracted l1-algebra of S; that is, the Banach algebra l1 (S)/Z, where Z is the ideal spanned by the zero of S. It is shown that, if l1 (G) is primitive for some non-zero maximal subgroup G of S, then is primitive. The same result also holds with primitivity replaced by a stronger condition, here called *-primitivity

It is shown that the following conditions on a finite-dimensional algebra A over a real closed field or an algebraically closed field of characteristic zero are equivalent: (i) A admits a special involution, in the sense of Easdown and Munn, (ii) A admits a proper involution, (iii) A is semisimple.

A weak form of faithfulness, depending on Green’s equivalence $\mathcal{D}$, is introduced for a ring $R$ graded by a semigroup $S$. Suppose that $R$ satisfies this condition. It is shown that if $e$ and $f$ are $\mathcal{D}$-equivalent idempotents of $S$ and $R_e$ is semiprime (respectively, prime, semiprimitive, right primitive), then $R_f$ is semiprime (respectively, prime, semiprimitive, right primitive). In addition, it is shown that if $G$ and $H$ are maximal subgroups of $S$ lying in the same $\mathcal{D}$-class and $R_G$ is semiprime (respectively, prime, semiprimitive, right primitive), then $R_H$ is semiprime (respectively, prime, semiprimitive, right primitive).

AMS 2000 Mathematics subject classification: Primary 16W50. Secondary 20M25

Sufficient conditions are obtained for a ring R, faithfully graded by a bisimple inverse semigroup S, to be (a) prime and (b) right primitive, these conditions being on the subring RG consisting of all elements of R with support contained in G, a maximal subgroup of S. Earlier results on semigroup rings arise as special cases.

It is shown that every element of the complex contracted semigroup algebra of an inverse semigroup S = S0 has a Moore-Penrose inverse, with respect to the natural involution, if and only if S is locally finite. In particular, every element of a complex group algebra has such an inverse if and only if the group is locally finite.

The algebra consisting of those linear transformations of a complex inner product space that have a formal adjoint is shown to possess a special involution. Two earlier results concerning special involutions are then generalized.

The monoids considered are the free monoid Mx and the free monoid-with-involution MIx on a nonempty set X. In each case, relative to a simply-defined involution, an explicit construction is given for a separating family of continuous star matrix representations of the l1-algebra of the monoid and it is shown that this algebra admits a faithful trace. The results are based on earlier work by M. J. Crabb et al. concerning the complex semigroup algebras of Mx and MIx.

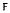Let denote a subring of the complex field that contains 1 and is closed under complex conjugation. It is shown that, with respect to the involution induced by word-reversal, the algebra over of a free monoid admits a trace and a separating family of star matrix representations. From the existence of a trace it is deduced that the aforementioned involution is special, in the sense of Easdown and Munn. Similar results hold for the algebra over of a free monoid with involution.

A construction is given for a trace function on the semigroup algebra of a certain type of E-unitary inverse semigroup over any subfield of the complex field that is closed under complex conjugation. In particular, the method applies to the semigroup algebras of free inverse semigroups of arbitrary rank.

Sufficient conditions are given for a contracted semigroup ring, in which the two-sided ideals have a certain property, to be (a) semiprime, (b) semiprimitive, (c) prime, (d) primitive. The results are applied to the contracted semigroup rings of inverse semigroups, where they provide new proofs of theorems of Domanov.