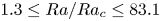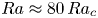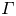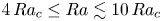147 results
Trauma diagnostic-related target proteins and their detection techniques
-
- Journal:
- Expert Reviews in Molecular Medicine / Volume 26 / 2024
- Published online by Cambridge University Press:
- 11 April 2024, e7
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Synthesis and Adsorption Desulfurization Performance of Modified Mesoporous Silica Materials M-MCM-41 (M = Fe, Co, Zn)
-
- Journal:
- Clays and Clay Minerals / Volume 67 / Issue 4 / August 2019
- Published online by Cambridge University Press:
- 01 January 2024, pp. 325-333
-
- Article
-
- You have access
- HTML
- Export citation
Photocatalytic Degradation of Methylene Blue Over Layered Double Hydroxides Using Various Divalent Metal Ions
-
- Journal:
- Clays and Clay Minerals / Volume 67 / Issue 4 / August 2019
- Published online by Cambridge University Press:
- 01 January 2024, pp. 340-347
-
- Article
-
- You have access
- HTML
- Export citation
Photocatalytic Activity of La-Containing Mixed- Metal Oxides Derived from Layered Double Hydroxides to Degrade Methylene Blue in the Presence of H2O2
-
- Journal:
- Clays and Clay Minerals / Volume 67 / Issue 4 / August 2019
- Published online by Cambridge University Press:
- 01 January 2024, pp. 253-264
-
- Article
-
- You have access
- HTML
- Export citation
Detecting Chlorite in the Chinese Loess Sequence by Diffuse Reflectance Spectroscopy
-
- Journal:
- Clays and Clay Minerals / Volume 54 / Issue 2 / April 2006
- Published online by Cambridge University Press:
- 01 January 2024, pp. 266-273
-
- Article
-
- You have access
- Export citation
Brain functional changes across mood states in bipolar disorder: from a large-scale network perspective
-
- Journal:
- Psychological Medicine / Volume 54 / Issue 4 / March 2024
- Published online by Cambridge University Press:
- 12 December 2023, pp. 763-774
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Subducted oceanic slab break-off in a post-collisional setting: Constraints from petrogenesis of Late Carboniferous dykes in central West Junggar, Xinjiang, NW China
-
- Journal:
- Geological Magazine / Volume 160 / Issue 9 / September 2023
- Published online by Cambridge University Press:
- 15 November 2023, pp. 1711-1741
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Changing trends in the global burden of mental disorders from 1990 to 2019 and predicted levels in 25 years
-
- Journal:
- Epidemiology and Psychiatric Sciences / Volume 32 / 2023
- Published online by Cambridge University Press:
- 07 November 2023, e63
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Vortex dynamics in rotating Rayleigh–Bénard convection
-
- Journal:
- Journal of Fluid Mechanics / Volume 974 / 10 November 2023
- Published online by Cambridge University Press:
- 06 November 2023, A43
-
- Article
-
- You have access
- HTML
- Export citation
Deep learning-based companion robot on senile dementia patients
-
- Journal:
- CNS Spectrums / Volume 28 / Issue S2 / October 2023
- Published online by Cambridge University Press:
- 27 October 2023, p. S3
-
- Article
-
- You have access
- Export citation
Treatment experience with and clinicopathological analysis of vocal fold leukoplakia per appearance classification guidance: a cohort of 1442 patients
-
- Journal:
- The Journal of Laryngology & Otology / Volume 138 / Issue 4 / April 2024
- Published online by Cambridge University Press:
- 15 September 2023, pp. 461-465
- Print publication:
- April 2024
-
- Article
-
- You have access
- HTML
- Export citation
Genetic and Environmental Influences on Blood Pressure and Serum Lipids Across Age-Groups
-
- Journal:
- Twin Research and Human Genetics / Volume 26 / Issue 3 / June 2023
- Published online by Cambridge University Press:
- 31 August 2023, pp. 223-230
-
- Article
-
- You have access
- HTML
- Export citation
Simple, stable and efficient nonlinear pulse compression through cascaded filamentation in air
-
- Journal:
- High Power Laser Science and Engineering / Volume 11 / 2023
- Published online by Cambridge University Press:
- 22 August 2023, e84
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Effects of monolaurin on intestinal barrier, blood biochemical profile, immunity and antioxidant function in porcine epidemic diarrhoea virus-infected piglets
-
- Journal:
- British Journal of Nutrition / Volume 131 / Issue 2 / 28 January 2024
- Published online by Cambridge University Press:
- 17 August 2023, pp. 185-192
- Print publication:
- 28 January 2024
-
- Article
-
- You have access
- HTML
- Export citation
Thulium-doped all-PM fiber chirped pulse amplifier delivering 314 W average power
-
- Journal:
- High Power Laser Science and Engineering / Volume 11 / 2023
- Published online by Cambridge University Press:
- 14 August 2023, e73
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
A novel approach for variable star classification based on imbalanced learning
-
- Journal:
- Publications of the Astronomical Society of Australia / Volume 40 / 2023
- Published online by Cambridge University Press:
- 09 August 2023, e037
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Efficacy of dynamic interpersonal therapy for major depressive disorder in China: results of a multicentered, three-arm, randomized, controlled trial
-
- Journal:
- Psychological Medicine / Volume 53 / Issue 15 / November 2023
- Published online by Cambridge University Press:
- 05 April 2023, pp. 7242-7254
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
High-power femtosecond laser generation from an all-fiber linearly polarized chirped pulse amplifier
-
- Journal:
- High Power Laser Science and Engineering / Volume 11 / 2023
- Published online by Cambridge University Press:
- 09 February 2023, e25
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Exploring the Genetic Association between Obesity and Serum Lipid Levels Using Bivariate Methods
-
- Journal:
- Twin Research and Human Genetics / Volume 25 / Issue 6 / December 2022
- Published online by Cambridge University Press:
- 06 January 2023, pp. 234-244
-
- Article
-
- You have access
- HTML
- Export citation
Communal breeding by women is associated with lower investment from husbands – ERRATUM
-
- Journal:
- Evolutionary Human Sciences / Volume 4 / 2022
- Published online by Cambridge University Press:
- 13 December 2022, e55
-
- Article
-
- You have access
- Open access
- HTML
- Export citation