1. Introduction
Most energy systems rely on fluids to transfer heat from one device to another to facilitate power generation, provision of heating or production of chemicals. Flows are often forced through channels or arrays of pipes taking heat away from the surfaces. In a nuclear reactor, for example, the reactions occur within the fuel pins, which are cooled by flow of coolant through the channels formed by arrays of fuel pins to maintain their temperature within a specific limit as well as transferring energy to the steam generators. In an isothermal flow, the volume flux is driven by an externally applied pressure gradient, and the flow is referred to as ‘forced’. In a vertical configuration, however, buoyancy resulting from the lightening of the fluid close to the heated wall can provide a force that partially or fully drives the flow, referred to as mixed or natural convection, respectively. When heat flux is very high, we can have a ‘supernatural’ state of flow, where the buoyancy is sufficiently strong that a reversed pressure gradient may be necessary to limit or maintain a constant volume flux. Under certain conditions (e.g. the Boussinesq approximation) an upward heated flow may be considered equivalent to a downward flow cooled at the boundary (Appendix A).
Mixed convection is of significant importance to engineering design and safety considerations and as such extensive research has been carried out to develop engineering correlations (Jackson, Cotton & Axcell Reference Jackson, Cotton and Axcell1989; Yoo Reference Yoo2013), turbulence models (Kim, He & Jackson Reference Kim, He and Jackson2008; Bae Reference Bae2016) and a better understanding of the physical flows (You, Yoo & Choi Reference You, Yoo and Choi2003). A particularly interesting physics is that the flow, at a Reynolds number where shear-driven turbulence is ordinarily observed, in the presence of buoyancy may be partially or fully laminarised, or becomes a convection-driven turbulent flow (i.e. natural convection, referred to above). Heat transfer may be significantly impaired under such conditions. He, He & Seddighi (Reference He, He and Seddighi2016) (hereinafter referred to as HHS) modelled the effect of buoyancy using a prescribed body force, with linear or step radial dependence, without solving the energy equation. They attributed the suppression of turbulence to a reduction in the apparent Reynolds number of the flow, as measured by the pressure gradient required to drive the flow. Thus, the forced flow is compared with the unforced ‘equivalent pressure gradient’ (EPG) reference flow.
Meanwhile, in ordinary (isothermal) pipe flow, Kühnen et al. (Reference Kühnen, Song, Scarselli, Budanur, Riedl, Willis, Avila and Hof2018), observed relaminarisation attributed to flattening of the base flow profile. The idea of flattening was first suggested by Hof et al. (Reference Hof, De Lozar, Avila, Tu and Schneider2010) who showed that when two puffs were triggered too close to each other, the downstream puff would collapse due to the flattened streamwise velocity profile induced by the upstream puff. In the experiments of Kühnen et al. (Reference Kühnen, Song, Scarselli, Budanur, Riedl, Willis, Avila and Hof2018) the flattening was introduced by a range of internal and boundary flow manipulations and a full collapse of turbulence was obtained for Reynolds numbers up to 40 000. Marensi, Willis & Kerswell (Reference Marensi, Willis and Kerswell2019) showed the complement effect, i.e. the enhanced nonlinear stability of the laminar flow. They found that the minimal seed (smallest amplitude disturbance) for transition is ‘pushed out’ from the laminar state to larger amplitudes when the base flow is flattened, thus implying that a flattened base profile is more stable than the parabolic profile. Here, buoyancy forces also have a flattening effect and turbulence may be partially or fully suppressed. Furthermore, early experimental observations (Hanratty, Rosen & Kabel Reference Hanratty, Rosen and Kabel1958; Kemeny & Somers Reference Kemeny and Somers1962; Scheele & Hanratty Reference Scheele and Hanratty1962) and subsequent linear (Yao Reference Yao1987a,Reference Yaob; Yao & Rogers Reference Yao and Rogers1989; Chen & Chung Reference Chen and Chung1996; Su & Chung Reference Su and Chung2000) and weakly nonlinear (Rogers & Yao Reference Rogers and Yao1993; Khandelwal & Bera Reference Khandelwal and Bera2015) stability analyses suggested that, for sufficiently large heating, the flow becomes unstable and transitions to a new non-isothermal equilibrium state characterised by large-scale regular motions. In agreement with the experiments, the linear theory showed that this instability can occur at low Reynolds number (below 100) and for ![]() $Re>50$ the critical value of the Rayleigh number is almost independent of
$Re>50$ the critical value of the Rayleigh number is almost independent of ![]() $Re$ (Yao Reference Yao1987a). The first azimuthal mode was found to be the least stable (Yao Reference Yao1987a; Su & Chung Reference Su and Chung2000), consistent with the double-spiral patterns observed experimentally (Hanratty et al. Reference Hanratty, Rosen and Kabel1958) and the instability was linked to the inflectional velocity profile in the buoyancy-assisted case. As suggested by Su & Chung (Reference Su and Chung2000), a competition between different mechanisms – driven by either shear or convection – thus exists, and understanding its effect on the nature of the flow is the object of our study.
$Re$ (Yao Reference Yao1987a). The first azimuthal mode was found to be the least stable (Yao Reference Yao1987a; Su & Chung Reference Su and Chung2000), consistent with the double-spiral patterns observed experimentally (Hanratty et al. Reference Hanratty, Rosen and Kabel1958) and the instability was linked to the inflectional velocity profile in the buoyancy-assisted case. As suggested by Su & Chung (Reference Su and Chung2000), a competition between different mechanisms – driven by either shear or convection – thus exists, and understanding its effect on the nature of the flow is the object of our study.
In particular, in this work, we are interested in whether a flow is turbulent or laminar under certain heating conditions and when a turbulent flow may be laminarised or vice versa under the influence of buoyancy. We address this question for a vertically heated pipe, initially in the dynamical systems context through linear stability and by investigating how travelling wave solutions are affected by the buoyancy force. Next, the nature of the laminarisation is considered. In isothermal flow at transitional Reynolds numbers, the shear-driven state is known to be metastable – the probability of laminarisation follows a Poisson process with a laminarisation rate that depends on the Reynolds number. In any practical setting, where a pipe is of finite length, its length affects the probability of turbulence surviving to the end of a pipe. Hence a range of Reynolds numbers for transition are quoted, typically between 2000 and 2300. Therefore, we do not attempt to quantify the full statistical nature of the transition in the heated case, but instead we focus on the phenomenological-based EPG analysis of HHS. Through the above approaches, i.e. linear stability, nonlinear travelling wave (TW) and EPG analyses, we aim to elucidate the physical mechanisms underlying the buoyancy-suppression of turbulence, illustrating the bistability nature of such flows.
1.1. Nonlinear dynamical systems view
In subcritical wall-bounded shear flows, turbulence arises despite the linear stability of the laminar state (Schmid & Henningson Reference Schmid and Henningson2001; Drazin & Reid Reference Drazin and Reid2004). The implication is that the observed transition scenario can only be triggered by finite amplitude disturbances. In the last 30 years our understanding of transition to turbulence in such flows has greatly benefited from a fully nonlinear geometrical approach which adopts concepts from the dynamical systems theory. In this view, the flow is analysed as a huge (formally infinite-dimensional) dynamical system in which the flow state evolves along a trajectory in a phase space populated by various invariant solutions, TWs and periodic orbits (POs). Nonlinear TW solutions were first discovered numerically in the early 1990s for plane Couette flows (Nagata Reference Nagata1990) and in the 2000s for pipe flows (Faisst & Eckhardt Reference Faisst and Eckhardt2003; Wedin & Kerswell Reference Wedin and Kerswell2004; Pringle & Kerswell Reference Pringle and Kerswell2007). Since then, partly thanks to the advances in our computational and experimental capabilities, a growing amount of evidence has been collected for their dynamical importance (see reviews : Kerswell (Reference Kerswell2005), Eckhardt et al. (Reference Eckhardt, Schneider, Hof and Westerweel2007), Kawahara, Uhlmann & van Veen (Reference Kawahara, Uhlmann and van Veen2012) and Graham & Floryan (Reference Graham and Floryan2021)). These solutions, often referred to as ‘exact coherent states’ (ECSs), are believed to act as organising centres (Waleffe Reference Waleffe2001) in phase space, in the sense that, when the flow state approaches them, spatio-temporally organised patterns (streaks and streamwise rolls) are observed (Hof et al. Reference Hof, van Doorne, Westerweel, Nieuwstadt, Faisst, Eckhardt, Wedin, Kerswell and Waleffe2004; Kerswell & Tutty Reference Kerswell and Tutty2007).
ECSs are finite-amplitude non-trivial solutions disconnected from the laminar state and enter via saddle-node bifurcations as the flow rate is increased. Some solutions, typically those of higher spatial symmetry, exist at flow rates much below that at which transition is usually observed (Pringle, Duguet & Kerswell Reference Pringle, Duguet and Kerswell2009). ECSs are linearly unstable, although with only a few unstable directions. They may be divided into ‘upper-branch’ and ‘lower-branch’ states, depending on whether they are associated with a high or low friction factor. Lower-branch solutions are representative of the laminar–turbulent boundary – the so called ‘edge of chaos’ (Itano & Toh Reference Itano and Toh2001; Schneider & Eckhardt Reference Schneider and Eckhardt2006) – which separates initial conditions that lead to turbulence from those that decay and relaminarise. The edge comes closest to the laminar equilibrium at the ‘minimal seed’ for transition (Kerswell Reference Kerswell2018). Lower-branch solutions are believed to mediate the transition to turbulence (Duguet, Willis & Kerswell Reference Duguet, Willis and Kerswell2008; Schneider, Eckhardt & Yorke Reference Schneider, Eckhardt and Yorke2007), while some upper-branch solutions are embedded in the turbulent attractor and are representative of the turbulent dynamics (Avila et al. Reference Avila, Mellibovsky, Roland and Hof2013; Budanur et al. Reference Budanur, Short, Farazmand, Willis and Cvitanović2017).
Here, we are interested in studying how TW solutions are affected by the buoyancy force in a vertical heated pipe, and, in analysing their dynamics, we aim to elucidate the physical mechanisms underlying the buoyancy-suppression of turbulence. The transition between regimes is first investigated using linear stability in § 3.2, followed by analysis of TWs in § 3.3.
1.2. Equivalent pressure gradient analysis of HHS
Rather than simulating a temperature field, to reduce complexity HHS considered a fixed radially dependent axial body force that models the buoyancy force, and applied this to isothermal flow. Conventionally, heated flows are compared with the isothermal (unforced) flow at equivalent flow rate (EFR), but HHS observed better comparison with flows at the equivalent pressure gradient. In particular, after careful analysis, they observed that adding the radially dependent force does not alter the turbulent viscosity of an unforced flow driven by the same pressure gradient (see figure 10 therein). The unforced EPG flow is therefore a reference flow for cases with the extra radially dependent forcing.
Note that in a fixed mass-flux calculation, the pressure gradient reduces in response to driving from the buoyancy. Given a heated flow at a particular Reynolds number ![]() $Re$ (defined in terms of the mass flux), to determine the Reynolds number of the EPG flow, one must split the mass flux into contributions from the pressure gradient and from the buoyancy. The former component determines the ‘apparent Reynolds number’
$Re$ (defined in terms of the mass flux), to determine the Reynolds number of the EPG flow, one must split the mass flux into contributions from the pressure gradient and from the buoyancy. The former component determines the ‘apparent Reynolds number’ ![]() $Re_{app}$ of the EPG flow. Laminarisation of the body forced flow is observed to occur when its
$Re_{app}$ of the EPG flow. Laminarisation of the body forced flow is observed to occur when its ![]() $Re_{app}$ is consistent with the
$Re_{app}$ is consistent with the ![]() $Re$ at which laminarisation occurs in isothermal flow.
$Re$ at which laminarisation occurs in isothermal flow.
Further details of the analysis are provided in § 3.4 and HHS prediction is compared with a suite of simulations in § 3.5.
2. Formulation
Consider a vertically aligned circular pipe of diameter ![]() $D$, with the flow of fluid upwards. We model a short pipe section of length
$D$, with the flow of fluid upwards. We model a short pipe section of length ![]() $L$ (figure 1a) and let
$L$ (figure 1a) and let ![]() $\{\boldsymbol {u}(\boldsymbol {x},t),p(\boldsymbol {x},t),T(\boldsymbol {x},t) \}$ be the velocity, pressure and temperature fields, respectively. The fluid has kinematic viscosity
$\{\boldsymbol {u}(\boldsymbol {x},t),p(\boldsymbol {x},t),T(\boldsymbol {x},t) \}$ be the velocity, pressure and temperature fields, respectively. The fluid has kinematic viscosity ![]() $\nu$, density
$\nu$, density ![]() $\rho$, volume expansion coefficient
$\rho$, volume expansion coefficient ![]() $\gamma$ and thermal diffusivity
$\gamma$ and thermal diffusivity ![]() $\kappa$. Under the Boussinesq approximation, density variations are ignored except where they appear in terms multiplied by the acceleration due to gravity,
$\kappa$. Under the Boussinesq approximation, density variations are ignored except where they appear in terms multiplied by the acceleration due to gravity, ![]() $g\,\hat {\boldsymbol {z}}$, leading to the governing equations
$g\,\hat {\boldsymbol {z}}$, leading to the governing equations
where ![]() $T_{ref}$ is a reference temperature, defined in the following subsection, and
$T_{ref}$ is a reference temperature, defined in the following subsection, and ![]() $\mathrm {d}_zP$ is the pressure gradient for laminar flow with bulk velocity
$\mathrm {d}_zP$ is the pressure gradient for laminar flow with bulk velocity ![]() $U_b$. We suppose that
$U_b$. We suppose that ![]() $U_b$ is fixed, in which case
$U_b$ is fixed, in which case ![]() $\beta (\boldsymbol {u})$ adjusts to maintain fixed bulk velocity. We also suppose that the temperature of the wall,
$\beta (\boldsymbol {u})$ adjusts to maintain fixed bulk velocity. We also suppose that the temperature of the wall, ![]() $T_w$, and the bulk temperature,
$T_w$, and the bulk temperature, ![]() $T_b$, are fixed. The latter is achieved by including a uniform heat sink
$T_b$, are fixed. The latter is achieved by including a uniform heat sink ![]() $\epsilon (t)$ which adjusts to maintain the fixed bulk value,
$\epsilon (t)$ which adjusts to maintain the fixed bulk value, ![]() $T_b$. For such a flow, we can introduce axial periodicity, so that
$T_b$. For such a flow, we can introduce axial periodicity, so that ![]() $\epsilon (t)$ may be considered equivalent to the rate at which heat absorbed by the fluid would otherwise be carried out of the section of pipe. (Spatial periodicity limits the domain over which wall friction is averaged, which can lead to unrealistic fluctuations (mean-square variations from the time average) in the bulk velocity. We therefore assume constant flux.)
$\epsilon (t)$ may be considered equivalent to the rate at which heat absorbed by the fluid would otherwise be carried out of the section of pipe. (Spatial periodicity limits the domain over which wall friction is averaged, which can lead to unrealistic fluctuations (mean-square variations from the time average) in the bulk velocity. We therefore assume constant flux.)

Figure 1. (a) Schematic of the flow configuration. A pipe section of length ![]() $L$ and radius
$L$ and radius ![]() $R$ is considered. The pipe is vertically aligned in the gravity field
$R$ is considered. The pipe is vertically aligned in the gravity field ![]() $\boldsymbol {g}$ and the fluid inside it is driven upwards by an externally applied pressure gradient and by buoyancy. The latter results from the lightening of the fluid close to the heated wall. We assume that the temperature at the wall
$\boldsymbol {g}$ and the fluid inside it is driven upwards by an externally applied pressure gradient and by buoyancy. The latter results from the lightening of the fluid close to the heated wall. We assume that the temperature at the wall ![]() $T_w$ remains constant in the pipe section. (b) Laminar velocity profiles (2.11a,b) for increasing values of
$T_w$ remains constant in the pipe section. (b) Laminar velocity profiles (2.11a,b) for increasing values of ![]() $C$, as indicated by the arrows. Red dashed line,
$C$, as indicated by the arrows. Red dashed line, ![]() $C=0$ (isothermal profile); light grey to black lines,
$C=0$ (isothermal profile); light grey to black lines, ![]() $C=3$, 5, 7.5, 10.
$C=3$, 5, 7.5, 10.
For laminar flow, the flow is purely axial so that radial heat transport is purely conductive. If ![]() $\epsilon _0$ is the heating rate for the laminar case, then the observed quantity
$\epsilon _0$ is the heating rate for the laminar case, then the observed quantity ![]() $Nu:=\bar {\epsilon }/\epsilon _0$ is the Nusselt Number, where the overbar
$Nu:=\bar {\epsilon }/\epsilon _0$ is the Nusselt Number, where the overbar ![]() $\overline {(\bullet )}$ denotes time average.
$\overline {(\bullet )}$ denotes time average.
2.1. Non-dimensionalisation
Given the temperature at the wall ![]() $T_w$ and the bulk temperature
$T_w$ and the bulk temperature ![]() $T_b$, we put
$T_b$, we put ![]() $\Delta T=2(T_w-T_b)$ and take a reference temperature
$\Delta T=2(T_w-T_b)$ and take a reference temperature ![]() $T_{ref}=T_w-\Delta T=2T_b-T_w=T_c$, where
$T_{ref}=T_w-\Delta T=2T_b-T_w=T_c$, where ![]() $T_c$ is the centreline temperature for the case of laminar flow. (The choice for
$T_c$ is the centreline temperature for the case of laminar flow. (The choice for ![]() $T_{ref}$ does not influence the flow, since the constant
$T_{ref}$ does not influence the flow, since the constant ![]() $\gamma gT_{ref}$ could be absorbed into the pressure gradient.) We introduce the dimensionless temperature
$\gamma gT_{ref}$ could be absorbed into the pressure gradient.) We introduce the dimensionless temperature ![]() $\varTheta =(T-T_c)/\Delta T$. Let the pipe radius
$\varTheta =(T-T_c)/\Delta T$. Let the pipe radius ![]() $R=D/2$ be the length scale and the isothermal laminar centreline velocity
$R=D/2$ be the length scale and the isothermal laminar centreline velocity ![]() $2U_b$ be the velocity scale. The corresponding time scale is thus
$2U_b$ be the velocity scale. The corresponding time scale is thus ![]() $R/(2U_b)$. Hereafter, all variables are dimensionless except
$R/(2U_b)$. Hereafter, all variables are dimensionless except ![]() $\epsilon (t)$ which always appears in the dimensionless ratio
$\epsilon (t)$ which always appears in the dimensionless ratio ![]() $\epsilon /\epsilon _0$, i.e. the instantaneous Nusselt number. Non-dimensionalising with these scales, for the temperature equation we find
$\epsilon /\epsilon _0$, i.e. the instantaneous Nusselt number. Non-dimensionalising with these scales, for the temperature equation we find
For the laminar case, ![]() $\varTheta =\varTheta _{lam} = r^2$, we find
$\varTheta =\varTheta _{lam} = r^2$, we find
Plugging this ![]() $\Delta T$ back into (2.4), we obtain the dimensionless temperature equation
$\Delta T$ back into (2.4), we obtain the dimensionless temperature equation
where ![]() $Re:=U_bD/\nu$ is the Reynolds number and
$Re:=U_bD/\nu$ is the Reynolds number and ![]() $Pr:=\nu /\kappa$ is the Prandtl number. For the momentum equation we find
$Pr:=\nu /\kappa$ is the Prandtl number. For the momentum equation we find
The coefficient of the buoyancy term can be written
where ![]() $Gr:=\gamma g(T_w-T_b)D^3/\nu ^2$ is the Grashof number. Although the Grashof number is in common use, from
$Gr:=\gamma g(T_w-T_b)D^3/\nu ^2$ is the Grashof number. Although the Grashof number is in common use, from ![]() $Gr$ it is difficult to judge the magnitude of the buoyancy force relative to the pressure gradient of the laminar flow for this particular configuration. We therefore write the dimensionless momentum equation as
$Gr$ it is difficult to judge the magnitude of the buoyancy force relative to the pressure gradient of the laminar flow for this particular configuration. We therefore write the dimensionless momentum equation as
where ![]() $C$ measures the buoyancy force relative to the force that drives the laminar isothermal shear flow,
$C$ measures the buoyancy force relative to the force that drives the laminar isothermal shear flow,
The laminar velocity and laminar temperature profiles for this configuration are
and the no-slip and fixed-temperature boundary conditions at ![]() $r=1$ are
$r=1$ are
respectively, while periodic boundary conditions are applied in the streamwise direction. The laminar velocity profiles for different ![]() $C$ are shown in figure 1(b). The isothermal pipe flow is recovered for
$C$ are shown in figure 1(b). The isothermal pipe flow is recovered for ![]() $C=0$ (no buoyancy force) and
$C=0$ (no buoyancy force) and ![]() $Pr=0$ (temperature diffuses immediately), with the parabolic laminar profile
$Pr=0$ (temperature diffuses immediately), with the parabolic laminar profile ![]() $U_0=1-r^2$.
$U_0=1-r^2$.
For a statistically steady flow, Reynolds averaging is both time averaging and cylindrical surface averaging, where the latter is denoted as
Turbulent fluctuations are calculated as deviations from the mean components of the flow, i.e. ![]() $\{\boldsymbol {u}'(\boldsymbol {x},t), \varTheta '(\boldsymbol {x},t)\}:= \{ \boldsymbol {u}(\boldsymbol {x},t), \varTheta (\boldsymbol {x},t) \} - \{\langle \bar {\boldsymbol {u}}\rangle (r), \langle \bar {\varTheta }\rangle (r)\}$.
$\{\boldsymbol {u}'(\boldsymbol {x},t), \varTheta '(\boldsymbol {x},t)\}:= \{ \boldsymbol {u}(\boldsymbol {x},t), \varTheta (\boldsymbol {x},t) \} - \{\langle \bar {\boldsymbol {u}}\rangle (r), \langle \bar {\varTheta }\rangle (r)\}$.
2.2. Numerics
Simulations were carried out using the Openpipeflow solver (Willis Reference Willis2017), modified to include timestepping of the temperature field and the buoyancy term in the momentum equation. A variable ![]() $q(r,\theta , z)$ is discretised using a non-uniform grid in the radial direction with points clustered near the wall and Fourier decompositions in the azimuthal and streamwise directions, namely
$q(r,\theta , z)$ is discretised using a non-uniform grid in the radial direction with points clustered near the wall and Fourier decompositions in the azimuthal and streamwise directions, namely
where ![]() $\alpha =2{\rm \pi} /L$ is the streamwise wavenumber and
$\alpha =2{\rm \pi} /L$ is the streamwise wavenumber and ![]() $m_p$ determines the azimuthal periodicity (
$m_p$ determines the azimuthal periodicity (![]() $m_p=1$ for no discrete rotational symmetry). Radial derivatives are evaluated using central finite differences with a nine-point stencil. At
$m_p=1$ for no discrete rotational symmetry). Radial derivatives are evaluated using central finite differences with a nine-point stencil. At ![]() $Re=5300$, in an
$Re=5300$, in an ![]() $L=5D$ long pipe we use a spatial resolution of
$L=5D$ long pipe we use a spatial resolution of ![]() $(N \times M \times K) = (64 \times 96 \times 96)$, which ensures a drop of at least four orders of magnitude in the amplitude spectra and provides the correct value for the friction factor, as reported in the literature (Eggels et al. Reference Eggels, Unger, Weiss, Westerweel, Adrian, Freidrich and Nieuwstadt1994). Following the 3/2 dealiasing rule, variables are evaluated on an
$(N \times M \times K) = (64 \times 96 \times 96)$, which ensures a drop of at least four orders of magnitude in the amplitude spectra and provides the correct value for the friction factor, as reported in the literature (Eggels et al. Reference Eggels, Unger, Weiss, Westerweel, Adrian, Freidrich and Nieuwstadt1994). Following the 3/2 dealiasing rule, variables are evaluated on an ![]() $N \times 3M \times 3K$ grid in physical space. A second-order predictor–corrector scheme is employed for temporal discretisation, and a fixed timestep of 0.01 is used. This is sufficient to ensure that the time discretisation error is no larger than the spatial discretisation error (measured by the corrector and spectra, respectively) and corresponds to a Courant–Friedrichs–Lewy (known as CFL) number of approximately 0.2–0.25.
$N \times 3M \times 3K$ grid in physical space. A second-order predictor–corrector scheme is employed for temporal discretisation, and a fixed timestep of 0.01 is used. This is sufficient to ensure that the time discretisation error is no larger than the spatial discretisation error (measured by the corrector and spectra, respectively) and corresponds to a Courant–Friedrichs–Lewy (known as CFL) number of approximately 0.2–0.25.
Data for simulations for various ![]() $Gr=16\,Re\,C$ and constant
$Gr=16\,Re\,C$ and constant ![]() $Re=5300$,
$Re=5300$, ![]() $Pr=0.7$ are shown in figure 2. There is good agreement with numerical data (You et al. Reference You, Yoo and Choi2003) and experimental data (Steiner Reference Steiner1971; Carr, Connor & Buhr Reference Carr, Connor and Buhr1973; Parlatan, Todreas & Driscoll Reference Parlatan, Todreas and Driscoll1996).
$Pr=0.7$ are shown in figure 2. There is good agreement with numerical data (You et al. Reference You, Yoo and Choi2003) and experimental data (Steiner Reference Steiner1971; Carr, Connor & Buhr Reference Carr, Connor and Buhr1973; Parlatan, Todreas & Driscoll Reference Parlatan, Todreas and Driscoll1996).

Figure 2. Change in ![]() $Nu$ flux, normalised by that for turbulent ‘forced convection’ (
$Nu$ flux, normalised by that for turbulent ‘forced convection’ (![]() $C \to 0$), as a function of
$C \to 0$), as a function of ![]() $Bo=8\times 10^4$
$Bo=8\times 10^4$ ![]() $(8 Nu\, Gr) / (Re^{3.425} Pr^{0.8})$. Data from simulations at
$(8 Nu\, Gr) / (Re^{3.425} Pr^{0.8})$. Data from simulations at ![]() $Re=5300$,
$Re=5300$, ![]() $Pr=0.7$ and various
$Pr=0.7$ and various ![]() $Gr=16\,Re\,C$. The upper and lower branches correspond to flow in shear-driven and convection-driven states, respectively.
$Gr=16\,Re\,C$. The upper and lower branches correspond to flow in shear-driven and convection-driven states, respectively.
2.3. Travelling wave solutions
In order to apply dynamical systems theory, the discretised momentum and temperature equations are formulated as an autonomous dynamical system (Viswanath Reference Viswanath2007; Willis, Cvitanović & Avila Reference Willis, Cvitanović and Avila2013),
where ![]() $\boldsymbol {X}$ is the vector of dependent variables, here
$\boldsymbol {X}$ is the vector of dependent variables, here ![]() $\boldsymbol {X}=(\boldsymbol {u},\varTheta )$, and
$\boldsymbol {X}=(\boldsymbol {u},\varTheta )$, and ![]() $\boldsymbol {p}$ is the vector of parameters of the system,
$\boldsymbol {p}$ is the vector of parameters of the system, ![]() $\boldsymbol {p}=(Re,C)$. The simplest solution is an equilibrium, which satisfies
$\boldsymbol {p}=(Re,C)$. The simplest solution is an equilibrium, which satisfies ![]() $\boldsymbol {F}(\boldsymbol {X};\boldsymbol {p})=0$. For pipe flow, the only equilibrium solution is the laminar solution. Travelling wave solutions satisfy
$\boldsymbol {F}(\boldsymbol {X};\boldsymbol {p})=0$. For pipe flow, the only equilibrium solution is the laminar solution. Travelling wave solutions satisfy ![]() $\boldsymbol {X}(\boldsymbol {x},t)=g(ct)\,\boldsymbol {X}(\boldsymbol {x},0)$, where here
$\boldsymbol {X}(\boldsymbol {x},t)=g(ct)\,\boldsymbol {X}(\boldsymbol {x},0)$, where here ![]() $g(l)$ applies a streamwise shift by
$g(l)$ applies a streamwise shift by ![]() $l$, and
$l$, and ![]() $c$ is the phase speed. Travelling waves are also known as ‘relative’ equilibrium solutions, as they are steady in a comoving frame. They therefore satisfy
$c$ is the phase speed. Travelling waves are also known as ‘relative’ equilibrium solutions, as they are steady in a comoving frame. They therefore satisfy
for some vector ![]() $(\boldsymbol {X},l,T)$, and hence can be calculated via a root solving method. The most popular method at present is the Newton–Krylov method. (Note that in addition to (2.16), two extra constraints are required to match the extra unknowns
$(\boldsymbol {X},l,T)$, and hence can be calculated via a root solving method. The most popular method at present is the Newton–Krylov method. (Note that in addition to (2.16), two extra constraints are required to match the extra unknowns ![]() $l$,
$l$, ![]() $T$ – see Viswanath (Reference Viswanath2007).) Time-dependent POs may also be calculated by this method. Typically POs originate via a Hopf bifurcation off a TW, but are not discussed further in this work. Stability of the solutions is calculated using the Arnoldi method to solve the eigenvalue problem
$T$ – see Viswanath (Reference Viswanath2007).) Time-dependent POs may also be calculated by this method. Typically POs originate via a Hopf bifurcation off a TW, but are not discussed further in this work. Stability of the solutions is calculated using the Arnoldi method to solve the eigenvalue problem
where ![]() $\sigma$ is the growth rate and the operator on the right-hand side is linearised about the TW
$\sigma$ is the growth rate and the operator on the right-hand side is linearised about the TW ![]() $\boldsymbol {X}_0$ by taking
$\boldsymbol {X}_0$ by taking ![]() $||\boldsymbol {dX}||\ll ||\boldsymbol {X}_0||$. (Numerical performance is improved by replacing
$||\boldsymbol {dX}||\ll ||\boldsymbol {X}_0||$. (Numerical performance is improved by replacing ![]() $\boldsymbol {X}_0(0)$ with
$\boldsymbol {X}_0(0)$ with ![]() $g(-l)\boldsymbol {X}_0(T)$ in (2.17).)
$g(-l)\boldsymbol {X}_0(T)$ in (2.17).)
The Newton–Krylov and Arnoldi solver, already available as a utility of Openpipeflow (Willis Reference Willis2017), were integrated with the timestepping code described in § 2.2 for heated pipe flow.
3. Results and discussion
All results presented herein pertain to the case ![]() $Pr=0.7$ and constant volume flux. This relatively low Prandtl number is a reasonable starting choice for the applications we are interested in, where most gasses have
$Pr=0.7$ and constant volume flux. This relatively low Prandtl number is a reasonable starting choice for the applications we are interested in, where most gasses have ![]() $Pr\approx 0.7$, e.g.
$Pr\approx 0.7$, e.g. ![]() $\textrm {CO}_2$. In large-scale cooling applications using liquid metal,
$\textrm {CO}_2$. In large-scale cooling applications using liquid metal, ![]() $Pr$ is much smaller. Cases where
$Pr$ is much smaller. Cases where ![]() $Pr>1$ (e.g.
$Pr>1$ (e.g. ![]() $Pr=7$ for water) are more expensive numerically due to a need for higher resolution for the temperature field.
$Pr=7$ for water) are more expensive numerically due to a need for higher resolution for the temperature field.
3.1. Direct numerical simulations
Simulations were performed in a pipe of length ![]() $L=5D$ for a range of Reynolds numbers to study the effect of the buoyancy parameter
$L=5D$ for a range of Reynolds numbers to study the effect of the buoyancy parameter ![]() $C$. Results are first shown for a relatively low Reynolds number,
$C$. Results are first shown for a relatively low Reynolds number, ![]() $Re=2500$. Figure 3 shows complete relaminarisation of transitional turbulence in response to the introduction of buoyancy for intermediate values of
$Re=2500$. Figure 3 shows complete relaminarisation of transitional turbulence in response to the introduction of buoyancy for intermediate values of ![]() $C=O(10^{-1} )-O(1)$. Relaminarisation events are revealed by monitoring the energy of the streamwise-dependent component of the flow, denoted
$C=O(10^{-1} )-O(1)$. Relaminarisation events are revealed by monitoring the energy of the streamwise-dependent component of the flow, denoted ![]() $E_{3D}$, which shows a rapid decay when the flow relaminarises,
$E_{3D}$, which shows a rapid decay when the flow relaminarises, ![]() $E_{3D}\to 0$ and
$E_{3D}\to 0$ and ![]() $\varepsilon (t)/\varepsilon _0\to 1$. At larger
$\varepsilon (t)/\varepsilon _0\to 1$. At larger ![]() $C\geqslant O(10)$, turbulent fluctuations are not completely suppressed. Instead a convection-driven flow is set up, which becomes stronger as
$C\geqslant O(10)$, turbulent fluctuations are not completely suppressed. Instead a convection-driven flow is set up, which becomes stronger as ![]() $C$ is increased.
$C$ is increased.

Figure 3. Energy of the streamwise-dependent component of the flow. Here ![]() $Re=2500$,
$Re=2500$, ![]() $L=5D$,
$L=5D$, ![]() $Pr=0.7$ for a range of
$Pr=0.7$ for a range of ![]() $C$ (values reported in the legend). Intermediate values of
$C$ (values reported in the legend). Intermediate values of ![]() $C$ destabilise the turbulence, or even cause immediate relaminarisation.
$C$ destabilise the turbulence, or even cause immediate relaminarisation.
At ![]() $Re=5300$ the effect of buoyancy is found to be slightly different – turbulence is not observed to undergo complete relaminarisation, but instead transitions directly to a weak convection-driven state. Figure 4 shows simulations with
$Re=5300$ the effect of buoyancy is found to be slightly different – turbulence is not observed to undergo complete relaminarisation, but instead transitions directly to a weak convection-driven state. Figure 4 shows simulations with ![]() $C=O(1)\text {--}O(10)$. The buoyancy causes suppression of the turbulence and therefore a drop in
$C=O(1)\text {--}O(10)$. The buoyancy causes suppression of the turbulence and therefore a drop in ![]() $\varepsilon (t)/\varepsilon _0$, so that the Nusselt number
$\varepsilon (t)/\varepsilon _0$, so that the Nusselt number ![]() $Nu=\bar {\varepsilon }/\varepsilon _0$ reduces substantially. The corresponding velocity and temperature mean profiles,
$Nu=\bar {\varepsilon }/\varepsilon _0$ reduces substantially. The corresponding velocity and temperature mean profiles, ![]() $\langle u_z\rangle (r)$ and
$\langle u_z\rangle (r)$ and ![]() $\langle \varTheta \rangle (r)$, are shown in figure 4(b,c) together with the laminar profiles at
$\langle \varTheta \rangle (r)$, are shown in figure 4(b,c) together with the laminar profiles at ![]() $C=0$ for comparison. Cases where turbulence is suppressed exhibit a flattened base velocity profile.
$C=0$ for comparison. Cases where turbulence is suppressed exhibit a flattened base velocity profile.

Figure 4. Here ![]() $Re=5300$,
$Re=5300$, ![]() $L=5\,D$,
$L=5\,D$, ![]() $Pr=0.7$, resolution
$Pr=0.7$, resolution ![]() $64\times 96\times 96$. (a) Non-dimensional instantaneous heat flux,
$64\times 96\times 96$. (a) Non-dimensional instantaneous heat flux, ![]() $Nu=\epsilon /\epsilon _0$ for different values of
$Nu=\epsilon /\epsilon _0$ for different values of ![]() $C$, as indicated in the legend. (b,c) Snapshots of mean streamwise velocity
$C$, as indicated in the legend. (b,c) Snapshots of mean streamwise velocity ![]() $\langle u_z\rangle (r)$ and temperature
$\langle u_z\rangle (r)$ and temperature ![]() $\langle \varTheta \rangle (r)$ profiles at
$\langle \varTheta \rangle (r)$ profiles at ![]() $t=1000$ for the same values of
$t=1000$ for the same values of ![]() $C$ shown at the top. The thick light-grey lines correspond to the laminar profiles (2.11a,b) with
$C$ shown at the top. The thick light-grey lines correspond to the laminar profiles (2.11a,b) with ![]() $C=0$.
$C=0$.
The case for ![]() $C=7.5$ is shown for longer time in figure 5(a). The shear-driven turbulent state is metastable only, and around
$C=7.5$ is shown for longer time in figure 5(a). The shear-driven turbulent state is metastable only, and around ![]() $t \approx 2000$ turbulence is more suppressed as there is a switch to the more quiescent convection-driven state. As
$t \approx 2000$ turbulence is more suppressed as there is a switch to the more quiescent convection-driven state. As ![]() $C$ is increased further the buoyancy starts to drive a more turbulent convection-driven state. For these cases the velocity profile is more ‘M-shaped’ as seen in figure 5(b).
$C$ is increased further the buoyancy starts to drive a more turbulent convection-driven state. For these cases the velocity profile is more ‘M-shaped’ as seen in figure 5(b).

Figure 5. Parameters as in figure 4 but for larger ![]() $C$ (values reported in the legend). (a) Non-dimensional instantaneous heat flux,
$C$ (values reported in the legend). (a) Non-dimensional instantaneous heat flux, ![]() $Nu=\epsilon /\epsilon _0$. The initial transients (
$Nu=\epsilon /\epsilon _0$. The initial transients (![]() $t \approx 100\text {--}200$) are omitted for all trajectories and the curves corresponding to
$t \approx 100\text {--}200$) are omitted for all trajectories and the curves corresponding to ![]() $C \geqslant 12.5$ are shifted in time by an arbitrary offset, for clarity only. (b) Snapshots of the mean streamwise velocity profiles
$C \geqslant 12.5$ are shifted in time by an arbitrary offset, for clarity only. (b) Snapshots of the mean streamwise velocity profiles ![]() $\langle u_z\rangle (r)$ for the same values of
$\langle u_z\rangle (r)$ for the same values of ![]() $C$ shown in panel (a). All the snapshots are taken at
$C$ shown in panel (a). All the snapshots are taken at ![]() $t=1000$. For
$t=1000$. For ![]() $C=7.5$ an additional snapshot (solid grey line with dots) is shown corresponding to
$C=7.5$ an additional snapshot (solid grey line with dots) is shown corresponding to ![]() $t=7500$ (marked with a grey dot on the corresponding trajectory in panel (a)). The thick light-grey line in panel (b) corresponds to the laminar streamwise velocity profile (2.11a) with
$t=7500$ (marked with a grey dot on the corresponding trajectory in panel (a)). The thick light-grey line in panel (b) corresponds to the laminar streamwise velocity profile (2.11a) with ![]() $C=0$.
$C=0$.
The convective state at ![]() $Re=5300$ and
$Re=5300$ and ![]() $C=7.5$ is visualised in figure 6 together with the metastable shear-driven turbulent state. When comparing the deviations from the isothermal laminar profile (see figure 6a–d), both the shear and convective states show a deceleration in the core and acceleration close to the wall, with the convective states showing very smooth and almost
$C=7.5$ is visualised in figure 6 together with the metastable shear-driven turbulent state. When comparing the deviations from the isothermal laminar profile (see figure 6a–d), both the shear and convective states show a deceleration in the core and acceleration close to the wall, with the convective states showing very smooth and almost ![]() $z$- and
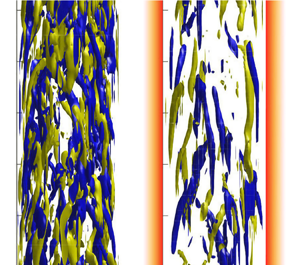
$z$- and ![]() $\theta$-independent contour levels. Deviations from the mean profile (see figure 6e–h), however, reveal that the convective state has larger and more elongated flow structures compared with the shear-driven turbulence. In both types of visualisation it is clear that the small-scale turbulent eddies are strongly suppressed in the convection-driven flow.
$\theta$-independent contour levels. Deviations from the mean profile (see figure 6e–h), however, reveal that the convective state has larger and more elongated flow structures compared with the shear-driven turbulence. In both types of visualisation it is clear that the small-scale turbulent eddies are strongly suppressed in the convection-driven flow.

Figure 6. Isolevels of streamwise velocity perturbation for (a,c,e,g) the shear-driven turbulence and (b,d,f,h) the convective state at ![]() $Re=5300$,
$Re=5300$, ![]() $C=7.5$ and
$C=7.5$ and ![]() $t=1000$,
$t=1000$, ![]() $t=7500$, respectively. (The corresponding streamwise velocity profiles at these times were shown in 5b.) Plots in panels (a–d) show deviations from the isothermal laminar profile
$t=7500$, respectively. (The corresponding streamwise velocity profiles at these times were shown in 5b.) Plots in panels (a–d) show deviations from the isothermal laminar profile ![]() $U_0=1-r^2$, while plots in panels (e–h) show deviations from the mean profile
$U_0=1-r^2$, while plots in panels (e–h) show deviations from the mean profile ![]() $\langle u_z\rangle (r)$. Dark/light regions correspond to slow/fast streaks. Ten contours are used between the maximum and minimum values, corresponding to (a–d)
$\langle u_z\rangle (r)$. Dark/light regions correspond to slow/fast streaks. Ten contours are used between the maximum and minimum values, corresponding to (a–d) ![]() $u-U_0 \in [-0.4, 0.3]$, (e,g)
$u-U_0 \in [-0.4, 0.3]$, (e,g) ![]() $u' \in [-0.2, 0.1]$ and (f,h)
$u' \in [-0.2, 0.1]$ and (f,h) ![]() $u'\in [-0.1, 0.08]$. The arrows in the
$u'\in [-0.1, 0.08]$. The arrows in the ![]() $r-\theta$ cross-sections (c,d,g,h) indicate the cross-sectional velocity components, multiplied by a factor of two for the shear turbulence (c,g) and five for the convective state (d,h), for visualisation reasons only. The
$r-\theta$ cross-sections (c,d,g,h) indicate the cross-sectional velocity components, multiplied by a factor of two for the shear turbulence (c,g) and five for the convective state (d,h), for visualisation reasons only. The ![]() $r-\theta$ cross-sections (c,d,g,h) are taken at
$r-\theta$ cross-sections (c,d,g,h) are taken at ![]() $z=0$ while the
$z=0$ while the ![]() $r-z$ sections are taken at
$r-z$ sections are taken at ![]() $\theta ={\rm \pi} /2$.
$\theta ={\rm \pi} /2$.
Figure 7 shows the type of state seen in simulations: laminar flow (L), shear-driven turbulence (S) and convection-driven flow (C), for a range of ![]() $Re$ and
$Re$ and ![]() $C$. The initial condition for each simulation was a previously calculated shear-driven state at similar
$C$. The initial condition for each simulation was a previously calculated shear-driven state at similar ![]() $Re$. (This is except for
$Re$. (This is except for ![]() $Re\leqslant 2000$ and
$Re\leqslant 2000$ and ![]() $C>3$, where it is clear that the shear-driven state decays immediately, i.e. only the convective state could be supported, and hence the initial condition was of convection type.) For each simulation it is relatively easy to distinguish between the shear- and convection-type flows, since the former shows far more chaotic time series and higher heat flux. The case for
$C>3$, where it is clear that the shear-driven state decays immediately, i.e. only the convective state could be supported, and hence the initial condition was of convection type.) For each simulation it is relatively easy to distinguish between the shear- and convection-type flows, since the former shows far more chaotic time series and higher heat flux. The case for ![]() $C=7.5$ in figure 5(a) shows this difference, and also that multiple behaviours are possible at the same parameters for significant periods of time. The shear-driven state is marked if observed for
$C=7.5$ in figure 5(a) shows this difference, and also that multiple behaviours are possible at the same parameters for significant periods of time. The shear-driven state is marked if observed for ![]() ${\gtrsim }1000$ time units. (It is stable or at least metastable with a long expected lifetime.) A relaminarisation is marked if the energy of the streamwise component of the flow drops below
${\gtrsim }1000$ time units. (It is stable or at least metastable with a long expected lifetime.) A relaminarisation is marked if the energy of the streamwise component of the flow drops below ![]() $10^{-5}$. Overall, figure 7 indicates that as
$10^{-5}$. Overall, figure 7 indicates that as ![]() $C$ (or
$C$ (or ![]() $Gr$) increases, a larger
$Gr$) increases, a larger ![]() $Re$ is needed in order to drive shear turbulence, or, equivalently, as
$Re$ is needed in order to drive shear turbulence, or, equivalently, as ![]() $Re$ increases, shear-driven states persist to larger
$Re$ increases, shear-driven states persist to larger ![]() $C$. For
$C$. For ![]() $C \geqslant 4$ simulations suggest that a convective instability kicks in, roughly independently of the Reynolds number over this range. In between, it is possible to completely relaminarise flow up to
$C \geqslant 4$ simulations suggest that a convective instability kicks in, roughly independently of the Reynolds number over this range. In between, it is possible to completely relaminarise flow up to ![]() $Re\approx 3500$, but at larger
$Re\approx 3500$, but at larger ![]() $Re$ the progression is as in figure 5 – from a shear-driven turbulent state to a weak convection-driven state, then to a more turbulent convection-driven state as
$Re$ the progression is as in figure 5 – from a shear-driven turbulent state to a weak convection-driven state, then to a more turbulent convection-driven state as ![]() $C$ is increased.
$C$ is increased.

Figure 7. Regions of laminar (L) flow, shear-driven (S) turbulence and convection-driven (C) flow. Points where multiple behaviours are observed are marked with a slight offset in ![]() $Re$. Simulations are initiated with a previously calculated shear-driven state at similar
$Re$. Simulations are initiated with a previously calculated shear-driven state at similar ![]() $Re$, except for the region
$Re$, except for the region ![]() $Re\leqslant 2000$ and
$Re\leqslant 2000$ and ![]() $C>3$ where the shear-driven state decays immediately and hence simulations are started with a convection-driven state.
$C>3$ where the shear-driven state decays immediately and hence simulations are started with a convection-driven state.
In the following sections we determine whether the boundaries of stability observed in figure 7 are consistent with linear stability of the laminar flow, analysis of TW solutions and the viewpoint of HHS.
3.2. Linear stability analysis
As the transition to shear-driven turbulence in isothermal flow occurs in the absence of a linear instability, this section relates to the transition to convection-driven flow states, in particular with respect to loss of stability of the modified laminar base profile (2.11a,b) for non-zero ![]() $C$. Linear stability of mixed-convection pipe flow has been studied by Yao (Reference Yao1987a) and Su & Chung (Reference Su and Chung2000), where the model differs slightly in the boundary condition and form of the heat sink. Our figure 2 suggests these differences make little difference to transition, however, we check for consistency with the nonlinear results of § 3.1.
$C$. Linear stability of mixed-convection pipe flow has been studied by Yao (Reference Yao1987a) and Su & Chung (Reference Su and Chung2000), where the model differs slightly in the boundary condition and form of the heat sink. Our figure 2 suggests these differences make little difference to transition, however, we check for consistency with the nonlinear results of § 3.1.
As our code uses Fourier expansions in the periodic dimensions, to calculate the eigenfunctions and stability of the base flow (2.11a,b) we need simulate only using a few Fourier modes. The Arnoldi method is employed to accelerate convergence and to access eigenvalues beyond the leading one. Linear stability analysis is performed for azimuthal wavenumbers ![]() $m=0,1,2$ and two streamwise wavenumbers
$m=0,1,2$ and two streamwise wavenumbers ![]() $\alpha =0.628$ and
$\alpha =0.628$ and ![]() $\alpha =1.7$ (commensurate with the pipe lengths
$\alpha =1.7$ (commensurate with the pipe lengths ![]() $L=5D$ and
$L=5D$ and ![]() $L=1.85D$ used in our direct numerical simulations (DNS) study of § 3.1 and in the TW analysis of § 3.3).
$L=1.85D$ used in our direct numerical simulations (DNS) study of § 3.1 and in the TW analysis of § 3.3).
The neutral curves, where the growth rate ![]() $\textrm {Re}(\sigma )=0$, are shown in figure 8. As expected (and as also reported by Yao (Reference Yao1987a) and Su & Chung (Reference Su and Chung2000)), the first azimuthal mode is found to be the least stable, it corresponds to the spatially largest mode and is the only mode that can exhibit flow across the axis. (The axisymmetric mode
$\textrm {Re}(\sigma )=0$, are shown in figure 8. As expected (and as also reported by Yao (Reference Yao1987a) and Su & Chung (Reference Su and Chung2000)), the first azimuthal mode is found to be the least stable, it corresponds to the spatially largest mode and is the only mode that can exhibit flow across the axis. (The axisymmetric mode ![]() $m=0$ is included in the numerical calculations for stability of the
$m=0$ is included in the numerical calculations for stability of the ![]() $m=1$ mode, but we have not observed instability of
$m=1$ mode, but we have not observed instability of ![]() $m=0$ type.) As shown in figure 8, the
$m=0$ type.) As shown in figure 8, the ![]() $m=1$ mode exhibits a fairly complex dependence on
$m=1$ mode exhibits a fairly complex dependence on ![]() $C$, while it is only weakly affected by the axial wavenumber. Indeed, the first branch for
$C$, while it is only weakly affected by the axial wavenumber. Indeed, the first branch for ![]() $\alpha =0.628$ almost coincides with that for
$\alpha =0.628$ almost coincides with that for ![]() $\alpha =1.7$ and the other two branches (not shown) are slightly shifted to the right. Consistent with the linear stability of isothermal pipe flow, the critical Reynolds number approaches infinity as
$\alpha =1.7$ and the other two branches (not shown) are slightly shifted to the right. Consistent with the linear stability of isothermal pipe flow, the critical Reynolds number approaches infinity as ![]() $C\to 0$ for any
$C\to 0$ for any ![]() $m$.
$m$.

Figure 8. Linear stability analysis for ![]() $\alpha =1.7$,
$\alpha =1.7$, ![]() $k=1$ (
$k=1$ (![]() $L=1.85D$) (solid lines). In the main figure,
$L=1.85D$) (solid lines). In the main figure, ![]() $m=1$; in the inset,
$m=1$; in the inset, ![]() $m=2$. The axisymmetric mode is included in the
$m=2$. The axisymmetric mode is included in the ![]() $m=1$ analysis (i.e.
$m=1$ analysis (i.e. ![]() $m=0$ and
$m=0$ and ![]() ${\pm }1$), but instability of this mode is not observed. The first branch (for
${\pm }1$), but instability of this mode is not observed. The first branch (for ![]() $m=1$) is also shown for the case
$m=1$) is also shown for the case ![]() $\alpha =0.628$ (dashed line). The neutral curves delimit regions where the flow is linearly stable (S) or unstable (U). The dotted vertical line indicates the value of
$\alpha =0.628$ (dashed line). The neutral curves delimit regions where the flow is linearly stable (S) or unstable (U). The dotted vertical line indicates the value of ![]() $C$ (
$C$ (![]() $C=5$) at which the growth rate is shown in figure 9 as a function of
$C=5$) at which the growth rate is shown in figure 9 as a function of ![]() $Re$.
$Re$.
Consistent with the appearance of the convective state found in simulation (figure 7), at ![]() $C \approx 4$ a linear instability appears, roughly independent of
$C \approx 4$ a linear instability appears, roughly independent of ![]() $Re$ for most of the range considered. The corresponding laminar profiles for
$Re$ for most of the range considered. The corresponding laminar profiles for ![]() $C = 3 \text {--} 10$ are shown in figure 1(b). For
$C = 3 \text {--} 10$ are shown in figure 1(b). For ![]() $C> 4$ the profiles present an ‘M-shape’ (independent of
$C> 4$ the profiles present an ‘M-shape’ (independent of ![]() $Re$, see (2.11a,b)), which becomes increasingly more pronounced as
$Re$, see (2.11a,b)), which becomes increasingly more pronounced as ![]() $C$ increases. The difference at the centreline is more than 80 % for
$C$ increases. The difference at the centreline is more than 80 % for ![]() $C=10$. The profile at
$C=10$. The profile at ![]() $C=3$ is flatter than the parabolic (isothermal) profile, with a centreline difference of almost 30 %, but does not have any inflection point. Therefore, in agreement with previous experimental and theoretical studies (Scheele & Hanratty Reference Scheele and Hanratty1962; Yao Reference Yao1987a; Su & Chung Reference Su and Chung2000), our analysis suggests that the linear instability of buoyancy-assisted pipe flow is linked to the inflectional velocity profiles occurring at sufficiently large heating and it is almost independent of
$C=3$ is flatter than the parabolic (isothermal) profile, with a centreline difference of almost 30 %, but does not have any inflection point. Therefore, in agreement with previous experimental and theoretical studies (Scheele & Hanratty Reference Scheele and Hanratty1962; Yao Reference Yao1987a; Su & Chung Reference Su and Chung2000), our analysis suggests that the linear instability of buoyancy-assisted pipe flow is linked to the inflectional velocity profiles occurring at sufficiently large heating and it is almost independent of ![]() $Re$.
$Re$.
Figure 8 also shows that, for ![]() $C \gtrsim 4$, a region of restabilisation is observed as
$C \gtrsim 4$, a region of restabilisation is observed as ![]() $Re$ is increased. This is also evidenced in figure 9, which shows a region of negative
$Re$ is increased. This is also evidenced in figure 9, which shows a region of negative ![]() $\textrm {Re}(\sigma )$ for
$\textrm {Re}(\sigma )$ for ![]() $1450< Re<6200$ at
$1450< Re<6200$ at ![]() $C=5$. Isosurfaces of streamwise vorticity for the eigenfunctions corresponding to the two neutral points where
$C=5$. Isosurfaces of streamwise vorticity for the eigenfunctions corresponding to the two neutral points where ![]() $\textrm {Re}(\sigma )$ becomes positive (
$\textrm {Re}(\sigma )$ becomes positive (![]() $Re \approx 400$ and 6200) are also shown in the insets of figure 9. For the larger Reynolds number,
$Re \approx 400$ and 6200) are also shown in the insets of figure 9. For the larger Reynolds number, ![]() $Re \approx 6200$, the eigenfunction looks like it is spiralling in the centre and resembles the ‘spiral’ solution found by Senoo, Deguchi & Nagata (Reference Senoo, Deguchi and Nagata2012), although their visualised solutions are nonlinear.
$Re \approx 6200$, the eigenfunction looks like it is spiralling in the centre and resembles the ‘spiral’ solution found by Senoo, Deguchi & Nagata (Reference Senoo, Deguchi and Nagata2012), although their visualised solutions are nonlinear.

Figure 9. Growth rate versus Reynolds number from linear stability analysis at ![]() $\alpha =1.7$,
$\alpha =1.7$, ![]() $k=1$ (
$k=1$ (![]() $L=1.85D$),
$L=1.85D$), ![]() $m=1$ and
$m=1$ and ![]() $C=5$ (corresponding to the dotted vertical line in figure 8). Insets: streamwise vorticity (blue/yellow are 30 % of the min/max value) close to the two neutral points (
$C=5$ (corresponding to the dotted vertical line in figure 8). Insets: streamwise vorticity (blue/yellow are 30 % of the min/max value) close to the two neutral points (![]() $Re \approx 400$ and 6200).
$Re \approx 400$ and 6200).
3.3. Continuation from  $\mathrm {TW}_{\mathrm {N4L}}$
$\mathrm {TW}_{\mathrm {N4L}}$
To better understand the effect of buoyancy, we perform a nonlinear analysis, starting from a known TW in isothermal pipe flow (![]() $C=Gr=0$) and continuing the solution to larger values. A vast repertoire of TWs has now been compiled in isothermal pipe flows (Budanur et al. Reference Budanur, Short, Farazmand, Willis and Cvitanović2017). For our purpose, we decided to focus on a fundamental solution, labelled
$C=Gr=0$) and continuing the solution to larger values. A vast repertoire of TWs has now been compiled in isothermal pipe flows (Budanur et al. Reference Budanur, Short, Farazmand, Willis and Cvitanović2017). For our purpose, we decided to focus on a fundamental solution, labelled ![]() $\text {TW}_{\text {N4L}}$ (Pringle et al. Reference Pringle, Duguet and Kerswell2009), which is highly symmetric (satisfying both shift-reflect and shift-rotate symmetries) and characterised by relatively smooth continuation branches in order to aid the numerical continuation. In Willis et al. (Reference Willis, Cvitanović and Avila2013), the lower branch of this solution was found to lie on the boundary between the laminar state and turbulence in a ‘minimal flow unit’. Localised solutions bifurcate off this class of solutions (Chantry, Willis & Kerswell Reference Chantry, Willis and Kerswell2014) and are found to mediate transition in extended domains (Avila et al. Reference Avila, Mellibovsky, Roland and Hof2013; Budanur & Hof Reference Budanur and Hof2017).
$\text {TW}_{\text {N4L}}$ (Pringle et al. Reference Pringle, Duguet and Kerswell2009), which is highly symmetric (satisfying both shift-reflect and shift-rotate symmetries) and characterised by relatively smooth continuation branches in order to aid the numerical continuation. In Willis et al. (Reference Willis, Cvitanović and Avila2013), the lower branch of this solution was found to lie on the boundary between the laminar state and turbulence in a ‘minimal flow unit’. Localised solutions bifurcate off this class of solutions (Chantry, Willis & Kerswell Reference Chantry, Willis and Kerswell2014) and are found to mediate transition in extended domains (Avila et al. Reference Avila, Mellibovsky, Roland and Hof2013; Budanur & Hof Reference Budanur and Hof2017).
Following Willis, Short & Cvitanović (Reference Willis, Short and Cvitanović2016) we start with the ‘minimal flow unit’ at Reynolds number ![]() $Re=2500$ with domain
$Re=2500$ with domain ![]() $(r, \theta , z) = [0,1] \times [0, {\rm \pi}/2] \times [0, 2{\rm \pi} /1.7]$, i.e.
$(r, \theta , z) = [0,1] \times [0, {\rm \pi}/2] \times [0, 2{\rm \pi} /1.7]$, i.e. ![]() $m_p=4$ and
$m_p=4$ and ![]() $\alpha =1.7$ in (2.14). For isothermal flow (
$\alpha =1.7$ in (2.14). For isothermal flow (![]() $C=Gr=0$), the phase speed of
$C=Gr=0$), the phase speed of ![]() $\text {TW}_{\text {N4L}}$ is
$\text {TW}_{\text {N4L}}$ is ![]() $c=0.61925$. The isothermal TW was first reconverged at
$c=0.61925$. The isothermal TW was first reconverged at ![]() $Pr=0.7$ using the Newton solver. A parametric continuation in
$Pr=0.7$ using the Newton solver. A parametric continuation in ![]() $C$ to non-zero values was then performed (figure 10) for fixed
$C$ to non-zero values was then performed (figure 10) for fixed ![]() $Re$,
$Re$, ![]() $Pr$ and
$Pr$ and ![]() $\alpha$. We were able to continue the isothermal solution from
$\alpha$. We were able to continue the isothermal solution from ![]() $C=0$ around positive
$C=0$ around positive ![]() $C$ and find that it connects with the upper branch at
$C$ and find that it connects with the upper branch at ![]() $C=0$, then beyond to
$C=0$, then beyond to ![]() $C\approx -40$. (Negative
$C\approx -40$. (Negative ![]() $C$ corresponds to a downward cooled flow – see Appendix A.) As a check, we verified that the values of
$C$ corresponds to a downward cooled flow – see Appendix A.) As a check, we verified that the values of ![]() $c=0.52575$ and
$c=0.52575$ and ![]() $Nu=2.378$ at
$Nu=2.378$ at ![]() $C=0$ on the upper branch, as well as the mean profiles, matched those of the previously known upper-branch isothermal solution
$C=0$ on the upper branch, as well as the mean profiles, matched those of the previously known upper-branch isothermal solution ![]() $\text {TW}_{\text {N4U}}$ with
$\text {TW}_{\text {N4U}}$ with ![]() $Pr=0.7$.
$Pr=0.7$.

Figure 10. Continuation in ![]() $C$ (or
$C$ (or ![]() $Gr$) from N4L at
$Gr$) from N4L at ![]() $Re=2500$. (a) Phase speed
$Re=2500$. (a) Phase speed ![]() $c$ versus
$c$ versus ![]() $C$ (or
$C$ (or ![]() $Gr$), (b)
$Gr$), (b) ![]() ${Nu}$ versus
${Nu}$ versus ![]() $C$ (or
$C$ (or ![]() $Gr$). Filled circles indicate the points along the continuation at which the mean streamwise velocity and temperature profiles are shown in figure 11.
$Gr$). Filled circles indicate the points along the continuation at which the mean streamwise velocity and temperature profiles are shown in figure 11.
In figure 10(b) it is seen that from ![]() $C=0$ to
$C=0$ to ![]() $C=6$ the Nusselt number
$C=6$ the Nusselt number ![]() ${Nu}$ increases by approx 0.75. By comparison, along the upper branch, over the large range
${Nu}$ increases by approx 0.75. By comparison, along the upper branch, over the large range ![]() $C=6$ to
$C=6$ to ![]() $C=-40$, it increases by only a further 1.25. Relatively speaking, the lower branch is rapidly pushed back towards the upper branch over the increase in
$C=-40$, it increases by only a further 1.25. Relatively speaking, the lower branch is rapidly pushed back towards the upper branch over the increase in ![]() $C$ and is suppressed altogether for
$C$ and is suppressed altogether for ![]() $C>7.5$. The mean velocity and temperature profiles at different points along the continuation are shown in figure 11. Observe that the profile in the near-wall region, where rolls and streaks occur, is similar at the saddle-node point to that of the isothermal upper branch solution. Figure 12(a) shows these rolls (arrows) and streaks (contours) in cross-sections of the velocity perturbation at the saddle-node point. The corresponding temperature perturbation field (‘thermal streaks’) is shown on the right. Similar to its isothermal counterpart, the TW is characterised by fast streaks located near the pipe wall and slow streaks in the interior. The core shows a strongly decelerated region relative to the laminar (isothermal) profile and thus the profile must become steeper at the wall to preserve the mass flux. The difference from the isothermal
$C>7.5$. The mean velocity and temperature profiles at different points along the continuation are shown in figure 11. Observe that the profile in the near-wall region, where rolls and streaks occur, is similar at the saddle-node point to that of the isothermal upper branch solution. Figure 12(a) shows these rolls (arrows) and streaks (contours) in cross-sections of the velocity perturbation at the saddle-node point. The corresponding temperature perturbation field (‘thermal streaks’) is shown on the right. Similar to its isothermal counterpart, the TW is characterised by fast streaks located near the pipe wall and slow streaks in the interior. The core shows a strongly decelerated region relative to the laminar (isothermal) profile and thus the profile must become steeper at the wall to preserve the mass flux. The difference from the isothermal ![]() $\mathrm {TW}_{\mathrm {N4L}}$, however, is less marked in the near-wall region than it is in the core.
$\mathrm {TW}_{\mathrm {N4L}}$, however, is less marked in the near-wall region than it is in the core.

Figure 11. Mean streamwise velocity (a) and temperature (b) profiles at the points along the continuation from N4L (![]() $Re=2500$) marked in figure 10 (SN: saddle node, LB/UB: lower/upper branch). The temperature profiles for
$Re=2500$) marked in figure 10 (SN: saddle node, LB/UB: lower/upper branch). The temperature profiles for ![]() $C=0$ and
$C=0$ and ![]() $C=2$ on the lower branch are indistinguishable.
$C=2$ on the lower branch are indistinguishable.

Figure 12. Cross-sections of streamwise velocity (a) and temperature (b) perturbations (deviations from the isothermal laminar flow) for the N4L TW at ![]() $Re=2500$ and
$Re=2500$ and ![]() $C=7.4$ (saddle node). Ten contours are used between the maximum and minimum. The arrows in the left graph indicate the cross-sectional velocities.
$C=7.4$ (saddle node). Ten contours are used between the maximum and minimum. The arrows in the left graph indicate the cross-sectional velocities.
Continuations were also performed at ![]() $Re=2000$ and
$Re=2000$ and ![]() $3000$, after reconverging the isothermal
$3000$, after reconverging the isothermal ![]() $\text {TW}_{\text {N4L}}$ at these Reynolds numbers. Results are shown in figure 13. The TW survives to larger
$\text {TW}_{\text {N4L}}$ at these Reynolds numbers. Results are shown in figure 13. The TW survives to larger ![]() $C$ as the Reynolds number increases (the saddle-node point of each curve moves to larger
$C$ as the Reynolds number increases (the saddle-node point of each curve moves to larger ![]() $C$ as
$C$ as ![]() $Re$ increases). This is consistent with the shear turbulence region in figure 7 persisting to larger
$Re$ increases). This is consistent with the shear turbulence region in figure 7 persisting to larger ![]() $C$ as
$C$ as ![]() $Re$ is increased. The saddle-node bifurcations at each
$Re$ is increased. The saddle-node bifurcations at each ![]() $Re$ occur at much larger values of
$Re$ occur at much larger values of ![]() $C$ than those at which suppression of turbulence was observed in the DNS. For example, at
$C$ than those at which suppression of turbulence was observed in the DNS. For example, at ![]() $Re=2500$ the saddle-node bifurcation occurs at
$Re=2500$ the saddle-node bifurcation occurs at ![]() $C\approx 7.5$, while in figure 7 shear-turbulence survives only for
$C\approx 7.5$, while in figure 7 shear-turbulence survives only for ![]() $C \lesssim 1$. This is not so surprising, considering that in isothermal pipe flows the lowest
$C \lesssim 1$. This is not so surprising, considering that in isothermal pipe flows the lowest ![]() $Re$ at which the N4L TW solution is found, i.e.
$Re$ at which the N4L TW solution is found, i.e. ![]() $Re=1290$ (Pringle et al. Reference Pringle, Duguet and Kerswell2009), is much below the commonly observed value for transition in experiments (
$Re=1290$ (Pringle et al. Reference Pringle, Duguet and Kerswell2009), is much below the commonly observed value for transition in experiments (![]() $Re \approx 1800 \text {--} 2300$). Furthermore, it should be taken into account that only one TW solution is analysed here – it cannot capture the entire phenomenon of turbulence suppression in a heated pipe flow, although is found to capture some of the fundamental characteristics.
$Re \approx 1800 \text {--} 2300$). Furthermore, it should be taken into account that only one TW solution is analysed here – it cannot capture the entire phenomenon of turbulence suppression in a heated pipe flow, although is found to capture some of the fundamental characteristics.

Figure 13. Continuation in ![]() $C$ from N4L for increasing values of
$C$ from N4L for increasing values of ![]() $Re$. The curve for
$Re$. The curve for ![]() $Re=2500$ is the same as that shown in figure 10(b).
$Re=2500$ is the same as that shown in figure 10(b).
Figure 14 shows that, while the lower branch solution for ![]() $Re=3000$ is on the edge of an attractor for shear-driven turbulence at
$Re=3000$ is on the edge of an attractor for shear-driven turbulence at ![]() $C=0$, this is no longer the case for
$C=0$, this is no longer the case for ![]() $C=4$. Shear-driven turbulence does not survive in the heated case, although shooting in the upper direction for
$C=4$. Shear-driven turbulence does not survive in the heated case, although shooting in the upper direction for ![]() $C=4$ does still produce a short turbulent transient. In particular, large amplification of the initial disturbance still occurs in the heated case, but the self-sustaining mechanism appears to be disrupted.
$C=4$ does still produce a short turbulent transient. In particular, large amplification of the initial disturbance still occurs in the heated case, but the self-sustaining mechanism appears to be disrupted.

Figure 14. Times series of (a) total dissipation ![]() $\mathcal {D}_{tot}$ (normalised by the laminar isothermal value
$\mathcal {D}_{tot}$ (normalised by the laminar isothermal value ![]() $\mathcal {D}_0=2{\rm \pi} L_z |-2|=4{\rm \pi} L_z$) and (b) energy of the streamwise-dependent modes
$\mathcal {D}_0=2{\rm \pi} L_z |-2|=4{\rm \pi} L_z$) and (b) energy of the streamwise-dependent modes ![]() $E_{3d}$ for simulations started from the lower-branch TW solutions at
$E_{3d}$ for simulations started from the lower-branch TW solutions at ![]() $Re=3000$,
$Re=3000$, ![]() $\alpha =1.7$ with
$\alpha =1.7$ with ![]() $C=0$ and
$C=0$ and ![]() $C=4$. The TW is perturbed by adding
$C=4$. The TW is perturbed by adding ![]() ${\mp } 0.001\,(\boldsymbol {w}_1 +0.01 \boldsymbol {w}_2)$ (denoted as ‘upper’ and ‘opposite’ directions) where
${\mp } 0.001\,(\boldsymbol {w}_1 +0.01 \boldsymbol {w}_2)$ (denoted as ‘upper’ and ‘opposite’ directions) where ![]() $\boldsymbol {w_1}$ and
$\boldsymbol {w_1}$ and ![]() $\boldsymbol {w_2}$ are the first (leading) and second eigenvectors. Shooting in the ‘upper’ direction leads to turbulence for
$\boldsymbol {w_2}$ are the first (leading) and second eigenvectors. Shooting in the ‘upper’ direction leads to turbulence for ![]() $C=0$, while the flow goes back to laminar when perturbed in the opposite direction. For
$C=0$, while the flow goes back to laminar when perturbed in the opposite direction. For ![]() $C=4$ both directions end up at the laminar point.
$C=4$ both directions end up at the laminar point.
To summarise this section, we have observed that a known TW solution of the isothermal pipe flow is suppressed by buoyancy and that it is connected to the transition to turbulence. The observations are consistent with destabilisation of the shear-driven turbulent state, but at this stage another approach is required to forge an approximate quantitative link with the transition from turbulence.
3.4. Calculation of the apparent Reynolds number of HHS
In § 1.2, where we gave a brief overview of HHS, the (isothermal) EPG flow was identified as a useful reference case for heated flows. To calculate the apparent Reynolds number of the EPG reference flow, one must determine the contribution to the mass flux from the buoyancy force that would have been induced in a fixed pressure-gradient flow. Here we summarise the key points of the analysis of HHS and apply them to a selected example case from our data. (The interested reader is referred to §§ 3.3 and 3.5 of HHS for a detailed derivation.) In the following section we relate HHS analysis to the phase diagram determined from the simulations of § 3.1.
The analysis starts by decomposing the body-force influenced flow (i.e. the total flow) into a pressure-driven flow of equivalent pressure gradient (the EPG reference flow) and a perturbation flow due to the body force,
where the superscripts ![]() ${{\dagger} }$ and
${{\dagger} }$ and ![]() $f$ denote the EPG and the body-force perturbation driven flows, respectively. In contrast to the conventional view, HHS observe that adding a non-uniform (radially dependent) streamwise body force to a flow initially driven only by a pressure gradient, does not alter its turbulent mixing characteristics and in particular the turbulent viscosity remains approximately the same. From this point of view, the body-force influenced flow behaves in the same way as the EPG flow and relaminarisation occurs when the Reynolds number
$f$ denote the EPG and the body-force perturbation driven flows, respectively. In contrast to the conventional view, HHS observe that adding a non-uniform (radially dependent) streamwise body force to a flow initially driven only by a pressure gradient, does not alter its turbulent mixing characteristics and in particular the turbulent viscosity remains approximately the same. From this point of view, the body-force influenced flow behaves in the same way as the EPG flow and relaminarisation occurs when the Reynolds number ![]() $Re_{app}$ of this ‘apparent’ flow drops below a certain threshold where turbulence cannot be sustained any more. Given the difficulties discussed in § 1 to uniquely define a critical Reynolds number for transition, we decided to follow HHS and select a nominal value of 2300, as quoted in many engineering textbooks (see e.g. White Reference White1979). By writing the bulk velocity
$Re_{app}$ of this ‘apparent’ flow drops below a certain threshold where turbulence cannot be sustained any more. Given the difficulties discussed in § 1 to uniquely define a critical Reynolds number for transition, we decided to follow HHS and select a nominal value of 2300, as quoted in many engineering textbooks (see e.g. White Reference White1979). By writing the bulk velocity ![]() $U_b$ of the EPG flow as the difference between that of the total flow and of the body-force perturbation driven flow, i.e.
$U_b$ of the EPG flow as the difference between that of the total flow and of the body-force perturbation driven flow, i.e. ![]() $U_b^{{\dagger} }=0.5-U_b^{f}$, the above relaminarisation criterion can be expressed as
$U_b^{{\dagger} }=0.5-U_b^{f}$, the above relaminarisation criterion can be expressed as
To determine ![]() $U_b^f$, the following expression was derived by integrating three times the Reynolds-averaged
$U_b^f$, the following expression was derived by integrating three times the Reynolds-averaged ![]() $z$-momentum equation of the body-forced perturbation flow:
$z$-momentum equation of the body-forced perturbation flow:
 \begin{equation} U_b^f:=Re\left[ \underbrace{\frac{1}{2}\int_0^1 (1-r^2)f(r)\,r\,\mathrm{d}r}_{\mathcal{I}_1} + \underbrace{\int_0^1 r\mathscr{R}_{uv}^f(r)\, r\,\mathrm{d}r}_{\mathcal{I}_2}\right] ,\end{equation}
\begin{equation} U_b^f:=Re\left[ \underbrace{\frac{1}{2}\int_0^1 (1-r^2)f(r)\,r\,\mathrm{d}r}_{\mathcal{I}_1} + \underbrace{\int_0^1 r\mathscr{R}_{uv}^f(r)\, r\,\mathrm{d}r}_{\mathcal{I}_2}\right] ,\end{equation}
where ![]() $\mathscr {R}_{uv}^f(r):=\langle \overline {(u_z'u_r')^f}\rangle$ is the Reynolds shear stress due to the perturbation flow induced by the body force
$\mathscr {R}_{uv}^f(r):=\langle \overline {(u_z'u_r')^f}\rangle$ is the Reynolds shear stress due to the perturbation flow induced by the body force ![]() $f(r)$. The first integral of (3.3),
$f(r)$. The first integral of (3.3), ![]() $\mathcal {I}_1:=\frac {1}{2}\int _0^1 (1-r^2)f(r)\,r\,\mathrm {d}r$, represents the direct contribution of the body force (which is assisting the flow), while the second integral,
$\mathcal {I}_1:=\frac {1}{2}\int _0^1 (1-r^2)f(r)\,r\,\mathrm {d}r$, represents the direct contribution of the body force (which is assisting the flow), while the second integral, ![]() $\mathcal {I}_2:=\int _0^1 r\mathscr {R}_{uv}^f(r) \,r\,\mathrm {d}r$, corresponds to the turbulent contribution related to the body-force perturbed flow. The Reynolds stress term
$\mathcal {I}_2:=\int _0^1 r\mathscr {R}_{uv}^f(r) \,r\,\mathrm {d}r$, corresponds to the turbulent contribution related to the body-force perturbed flow. The Reynolds stress term ![]() $\mathscr {R}_{uv}^f$ of the body-force perturbed flow is related to that of the total (
$\mathscr {R}_{uv}^f$ of the body-force perturbed flow is related to that of the total (![]() $\mathscr {R}_{uv}$) and EPG (
$\mathscr {R}_{uv}$) and EPG (![]() $\mathscr {R}_{uv}^{{\dagger} }$) flows by using the decomposition (3.1) and is approximated by introducing the eddy viscosity concept,
$\mathscr {R}_{uv}^{{\dagger} }$) flows by using the decomposition (3.1) and is approximated by introducing the eddy viscosity concept,
where ![]() $\mathscr {U}_{z}(r):= \langle \overline {({u}_z)}\rangle$,
$\mathscr {U}_{z}(r):= \langle \overline {({u}_z)}\rangle$, ![]() $\mathscr {U}_{z}^{{\dagger} }(r):= \langle \overline {({u}_z)^{{\dagger} }}\rangle$ and
$\mathscr {U}_{z}^{{\dagger} }(r):= \langle \overline {({u}_z)^{{\dagger} }}\rangle$ and ![]() $\nu _t$ and
$\nu _t$ and ![]() $\nu _{t}^{{\dagger} }$ are the eddy viscosities of the total and EPG flows, respectively. Under the assumption that
$\nu _{t}^{{\dagger} }$ are the eddy viscosities of the total and EPG flows, respectively. Under the assumption that ![]() $\nu _t = \nu _{t}^{{\dagger} }$, we obtain
$\nu _t = \nu _{t}^{{\dagger} }$, we obtain
where the perturbation flow ![]() $\mathscr {U}_{z}^f(r):= \langle \overline {({u}_z)^{f}}\rangle$ due to the imposed body force is obtained by integrating the Reynolds-averaged
$\mathscr {U}_{z}^f(r):= \langle \overline {({u}_z)^{f}}\rangle$ due to the imposed body force is obtained by integrating the Reynolds-averaged ![]() $z$-momentum equation
$z$-momentum equation
provided that the EPG flow (and hence ![]() $\nu _t^{{\dagger} }$) is known. Equations (3.5) and (3.6) correspond to (3.6) and (3.7) of HHS and the reader is referred their §§ 3.3 and 3.5 for a detailed derivation.
$\nu _t^{{\dagger} }$) is known. Equations (3.5) and (3.6) correspond to (3.6) and (3.7) of HHS and the reader is referred their §§ 3.3 and 3.5 for a detailed derivation.
Here, we apply the criterion for relaminarisation (3.2) proposed by HHS to our model for a vertical heated pipe. The radially dependent body force is ![]() $f_0=(4C/Re) \langle \bar {\varTheta }\rangle (r)$. Since the body force in HHS is zero at the axis, we shift the temperature profile by its value at the axis
$f_0=(4C/Re) \langle \bar {\varTheta }\rangle (r)$. Since the body force in HHS is zero at the axis, we shift the temperature profile by its value at the axis ![]() $\left .\langle \bar {\varTheta }\rangle \right |_{r=0}$ and absorb this constant into the pressure gradient (see figure 15). This leads to the body force
$\left .\langle \bar {\varTheta }\rangle \right |_{r=0}$ and absorb this constant into the pressure gradient (see figure 15). This leads to the body force
and a fixed-pressure Reynolds number

Figure 15. Application of HHS's relaminarisation criterion (3.3) in the case ![]() $C=2$ and
$C=2$ and ![]() $Re=3000$. (a) Temperature profile shifted by
$Re=3000$. (a) Temperature profile shifted by ![]() $\left .\langle \bar {\varTheta }\rangle \right |_{r=0}$; (b) the corresponding pressure gradient.
$\left .\langle \bar {\varTheta }\rangle \right |_{r=0}$; (b) the corresponding pressure gradient.
Initially, we consider the simulation with ![]() $C=2$ and
$C=2$ and ![]() $Re=3000$ for which it is observed that
$Re=3000$ for which it is observed that ![]() $Re_p=4252.71$. By inserting
$Re_p=4252.71$. By inserting ![]() $f=f_1$ in
$f=f_1$ in ![]() $\mathcal {I}_1$ we obtain
$\mathcal {I}_1$ we obtain ![]() $Re\,\mathcal {I}_1=0.12$. To calculate
$Re\,\mathcal {I}_1=0.12$. To calculate ![]() $\mathcal {I}_2$ we need to evaluate the EPG flow in order to obtain
$\mathcal {I}_2$ we need to evaluate the EPG flow in order to obtain ![]() $\nu _{t}^{{\dagger} }(r)$ and hence the Reynolds stress term
$\nu _{t}^{{\dagger} }(r)$ and hence the Reynolds stress term ![]() $\mathscr {R}_{uv}^f(r)$ via (3.5) and (3.6). By definition,
$\mathscr {R}_{uv}^f(r)$ via (3.5) and (3.6). By definition, ![]() $Re_p^{{\dagger} }=Re_p$. In an approach similar to Willis, Hwang & Cossu (Reference Willis, Hwang and Cossu2010), summarised in Appendix B, the eddy viscosity
$Re_p^{{\dagger} }=Re_p$. In an approach similar to Willis, Hwang & Cossu (Reference Willis, Hwang and Cossu2010), summarised in Appendix B, the eddy viscosity ![]() $\nu _{t}^{{\dagger} }(r)$ of the EPG reference flow is calculated using an expression originally suggested by Cess (Reference Cess1958), see (B2). The resulting eddy viscosity is shown in figure 16(a). By substituting
$\nu _{t}^{{\dagger} }(r)$ of the EPG reference flow is calculated using an expression originally suggested by Cess (Reference Cess1958), see (B2). The resulting eddy viscosity is shown in figure 16(a). By substituting ![]() $\nu _{t}^{{\dagger} }$ in (3.6) we can invert for
$\nu _{t}^{{\dagger} }$ in (3.6) we can invert for ![]() $\mathrm {d} \mathscr {U}_z^f/\mathrm {d}r$ which plugged into (3.5) gives us the Reynolds stress
$\mathrm {d} \mathscr {U}_z^f/\mathrm {d}r$ which plugged into (3.5) gives us the Reynolds stress ![]() $\mathscr {R}_{uv}^f(r)$ (see figure 16b). Finally, by inserting the latter in the second integral of (3.3) we obtain
$\mathscr {R}_{uv}^f(r)$ (see figure 16b). Finally, by inserting the latter in the second integral of (3.3) we obtain ![]() $Re\,\mathcal {I}_2=0.0405$. Putting everything together, (3.3) gives
$Re\,\mathcal {I}_2=0.0405$. Putting everything together, (3.3) gives ![]() $U_b^f=Re\,\mathcal {I}_1+Re\,\mathcal {I}_2=0.12+0.0405\approx 0.16$. Then, using (3.2),
$U_b^f=Re\,\mathcal {I}_1+Re\,\mathcal {I}_2=0.12+0.0405\approx 0.16$. Then, using (3.2), ![]() $Re_{app} = Re(1-2U_b^f)=2040 < 2300$, i.e. the flow is expected to relaminarise. This value obtained for the apparent Reynolds number is reasonable, since relaminarisation occurs after approximately 400 time units (see figure 15b).
$Re_{app} = Re(1-2U_b^f)=2040 < 2300$, i.e. the flow is expected to relaminarise. This value obtained for the apparent Reynolds number is reasonable, since relaminarisation occurs after approximately 400 time units (see figure 15b).

Figure 16. Eddy viscosity (a) of the EPG flow and Reynolds shear stress (b) of the body-force perturbed flow in the case ![]() $C=2$ and
$C=2$ and ![]() $Re=3000$. The eddy viscosity is calculated following an approach similar to Willis et al. (Reference Willis, Hwang and Cossu2010), as summarised in Appendix B. Once
$Re=3000$. The eddy viscosity is calculated following an approach similar to Willis et al. (Reference Willis, Hwang and Cossu2010), as summarised in Appendix B. Once ![]() $\nu _t^{{\dagger} }$ is known,
$\nu _t^{{\dagger} }$ is known, ![]() $\mathscr {R}_{uv}^f(r)$ is calculated using (3.5), together with (3.6).
$\mathscr {R}_{uv}^f(r)$ is calculated using (3.5), together with (3.6).
3.5. HHS prediction of phase diagram and nonlinear dynamics
We now consider the general case of a flow at ![]() $Re$ with heating
$Re$ with heating ![]() $C$, while introducing a number of approximations to simplify the analysis.
$C$, while introducing a number of approximations to simplify the analysis.
Firstly, the case discussed in § 3.4 (![]() $C=2$ and
$C=2$ and ![]() $Re=3000$) suggests that
$Re=3000$) suggests that ![]() $Re\,\mathcal {I}_1$ has a significantly greater contribution than
$Re\,\mathcal {I}_1$ has a significantly greater contribution than ![]() $Re\,\mathcal {I}_2$ in determining the body-force perturbation flow. This is found to be generally true for the cases considered herein, as well as those discussed in HHS, and hence we omit the term
$Re\,\mathcal {I}_2$ in determining the body-force perturbation flow. This is found to be generally true for the cases considered herein, as well as those discussed in HHS, and hence we omit the term ![]() $Re\,\mathcal {I}_2$ for simplicity below. The perturbation flow due to the body force can thus be evaluated as
$Re\,\mathcal {I}_2$ for simplicity below. The perturbation flow due to the body force can thus be evaluated as
where (3.7) has been used for ![]() $f(r)$.
$f(r)$.
Secondly, figure 4(c) shows that the temperature mean profiles are remarkably similar in all turbulent shear-driven flows (i.e. ignoring the laminar or convection driven flow states), as far as the integral part of the right-hand side of (3.9) is concerned, despite that the values of the ![]() $Nu$ (proportional to the gradient at the wall) are necessarily quite different for different cases. For the case
$Nu$ (proportional to the gradient at the wall) are necessarily quite different for different cases. For the case ![]() $Re=5300$,
$Re=5300$, ![]() $C=3.75$, for the left-hand side of (3.9) we obtain
$C=3.75$, for the left-hand side of (3.9) we obtain ![]() $Re\,\mathcal {I}_1 = 0.164$. By applying the above assumption,
$Re\,\mathcal {I}_1 = 0.164$. By applying the above assumption,
Let ![]() $Re_{app}$=2300 to find the critical
$Re_{app}$=2300 to find the critical ![]() $C$ for flow laminarisation, that is,
$C$ for flow laminarisation, that is,
or
For ![]() $C\gtrsim C_{cr,1}$ we expect to see rapid transition from the shear-driven turbulent state to the convective state. Noting
$C\gtrsim C_{cr,1}$ we expect to see rapid transition from the shear-driven turbulent state to the convective state. Noting ![]() $C=Gr/(16Re)$, the above can be expressed as a critical Grashof number
$C=Gr/(16Re)$, the above can be expressed as a critical Grashof number
Let us now consider the opposite scenario in which the flow under heating ![]() $C$ is either laminar or convection driven. Figure 4(c) shows that the temperature profiles in such flows are significantly different from those in a turbulent shear-driven flow, and generally with a much thicker thermal boundary layer, and hence a greater buoyancy force. Consider the extreme case when the radial heat transfer is purely due to conduction and the temperature distribution is given by
$C$ is either laminar or convection driven. Figure 4(c) shows that the temperature profiles in such flows are significantly different from those in a turbulent shear-driven flow, and generally with a much thicker thermal boundary layer, and hence a greater buoyancy force. Consider the extreme case when the radial heat transfer is purely due to conduction and the temperature distribution is given by ![]() $\langle \bar {\varTheta }\rangle =r^2$. The buoyancy-driven perturbation flow is therefore
$\langle \bar {\varTheta }\rangle =r^2$. The buoyancy-driven perturbation flow is therefore
Then a second critical ![]() $C=C_{cr,2}$ can be evaluated,
$C=C_{cr,2}$ can be evaluated,
below which the flow is expected to transition to the shear-driven turbulent flow. To put it another way, it is predicted that metastability of the shear-driven turbulent state should not be observed for ![]() $C\lesssim C_{cr,2}$, so that the turbulent state is stable. Between
$C\lesssim C_{cr,2}$, so that the turbulent state is stable. Between ![]() $C_{cr,1}$ and
$C_{cr,1}$ and ![]() $C_{cr,2}$ the shear-driven state is expected to be metastable, so that this or a convective state may be observed. In terms of the Grashof number,
$C_{cr,2}$ the shear-driven state is expected to be metastable, so that this or a convective state may be observed. In terms of the Grashof number,
Equations (3.12) and (3.15) are plotted on the ![]() $Re$–
$Re$–![]() $C$ graph in figure 17 together with all DNS results already presented in figure 7. The data of figure 7 was obtained starting from shear-driven turbulent states. Some additional simulations were performed at
$C$ graph in figure 17 together with all DNS results already presented in figure 7. The data of figure 7 was obtained starting from shear-driven turbulent states. Some additional simulations were performed at ![]() $Re=5300$ starting from convection-driven states and are reported in figure 17 using hollow symbols, with a slight offset in
$Re=5300$ starting from convection-driven states and are reported in figure 17 using hollow symbols, with a slight offset in ![]() $Re$ for visualisation reasons. Note that in an
$Re$ for visualisation reasons. Note that in an ![]() $Re$–
$Re$–![]() $Gr$ graph, (3.13) and (3.16) are straight lines (see the inset in figure 17).
$Gr$ graph, (3.13) and (3.16) are straight lines (see the inset in figure 17).

Figure 17. Regions of laminar (L) flow, shear-driven (S) turbulence and convection-driven (C) flow, as in figure 7, together with (3.12) and (3.15) and the linear stability stability curve (dashed red curve in figure 8). Initial conditions are a shear-driven turbulent state, except for the hollow symbols at ![]() $Re=5300$ which are started with a convection driven state, and similarly cases towards the bottom-right, where it is clear that the shear-driven state decays immediately.
$Re=5300$ which are started with a convection driven state, and similarly cases towards the bottom-right, where it is clear that the shear-driven state decays immediately.
Considering a series of DNS runs for a fixed ![]() $Re$, for example
$Re$, for example ![]() $Re=5300$, but increasing
$Re=5300$, but increasing ![]() $C$ values (heating) starting from
$C$ values (heating) starting from ![]() $C=0$, (3.12) gives the critical
$C=0$, (3.12) gives the critical ![]() $C=C_{cr,1}$ above which the flow will be laminarised or switch to convection driven. On the other hand, starting from a large
$C=C_{cr,1}$ above which the flow will be laminarised or switch to convection driven. On the other hand, starting from a large ![]() $C$ when the flow is laminarised or convective, (3.15) predicts a critical
$C$ when the flow is laminarised or convective, (3.15) predicts a critical ![]() $C=C_{cr,2}$ below which the flow will be turbulent when sufficient disturbances are provided in the DNS. As
$C=C_{cr,2}$ below which the flow will be turbulent when sufficient disturbances are provided in the DNS. As ![]() $C_{cr,1}$ is larger than
$C_{cr,1}$ is larger than ![]() $C_{cr,2}$ for a given
$C_{cr,2}$ for a given ![]() $Re$, there is an overlap in the possible state of flow, and consequently there is a hysteresis region in which the flow may or may not be laminarised, depending on the initial flow of the simulation (or experiment). As a result, the
$Re$, there is an overlap in the possible state of flow, and consequently there is a hysteresis region in which the flow may or may not be laminarised, depending on the initial flow of the simulation (or experiment). As a result, the ![]() $Re$–
$Re$–![]() $C$ plane can be divided into three regimes by the curves representing the two equations, i.e. turbulent shear-driven flow (regime I), convection-driven or laminar flow (regime III) and regime II in which either of the above may happen dependent on the initial flow. Note that for the Reynolds number range considered here, the linear stability curve (showed as a dashed grey line in figure 7) is always to the right of
$C$ plane can be divided into three regimes by the curves representing the two equations, i.e. turbulent shear-driven flow (regime I), convection-driven or laminar flow (regime III) and regime II in which either of the above may happen dependent on the initial flow. Note that for the Reynolds number range considered here, the linear stability curve (showed as a dashed grey line in figure 7) is always to the right of ![]() $C_{cr,2}$, i.e.
$C_{cr,2}$, i.e. ![]() $C_{cr,2}< C_{LS}$. The two curves cross at
$C_{cr,2}< C_{LS}$. The two curves cross at ![]() $Re\approx 6000$ (not shown), which means that, for
$Re\approx 6000$ (not shown), which means that, for ![]() $Re<6000$ the convective flow is always linearly stable if
$Re<6000$ the convective flow is always linearly stable if ![]() $C < C_{cr,2}$. Hence, below
$C < C_{cr,2}$. Hence, below ![]() $Re\approx 6000$, shear driven turbulence may be observed for
$Re\approx 6000$, shear driven turbulence may be observed for ![]() $C< C_{LS}$.
$C< C_{LS}$.
A plot showing the phase transitions for the fixed Reynolds number ![]() $Re=5300$ is provided in figure 18, where the Nusselt number is displayed as a function of
$Re=5300$ is provided in figure 18, where the Nusselt number is displayed as a function of ![]() $C$ for simulations started with either shear-driven or convection-driven states. The two critical
$C$ for simulations started with either shear-driven or convection-driven states. The two critical ![]() $C$ at this Reynolds number,
$C$ at this Reynolds number, ![]() $C_{cr,1}=7.1$ and
$C_{cr,1}=7.1$ and ![]() $C_{cr,2}=3.4$, are indicated with vertical lines in figure 18. Starting from an unheated (
$C_{cr,2}=3.4$, are indicated with vertical lines in figure 18. Starting from an unheated (![]() $C=0$) turbulent flow, applying a low heating (
$C=0$) turbulent flow, applying a low heating (![]() $C \lessapprox 7$), we observe that the flow remains turbulent over the entire period of simulation (
$C \lessapprox 7$), we observe that the flow remains turbulent over the entire period of simulation (![]() $t = 2000$). The dynamics thus sits on the upper branch shown in figure 18. As
$t = 2000$). The dynamics thus sits on the upper branch shown in figure 18. As ![]() $C$ is increased, the lifetime of shear-turbulence drops below 2000 time units for
$C$ is increased, the lifetime of shear-turbulence drops below 2000 time units for ![]() $C \gtrapprox 7.5$ and turbulence only survives for less than 500 time units at
$C \gtrapprox 7.5$ and turbulence only survives for less than 500 time units at ![]() $C = 10$. It then switches to the convection-type flow. This behaviour is marked in figure 18 by plotting the upper-branch curve with a dashed line for
$C = 10$. It then switches to the convection-type flow. This behaviour is marked in figure 18 by plotting the upper-branch curve with a dashed line for ![]() $C \geqslant 7.5$ until it crosses the lower-branch at
$C \geqslant 7.5$ until it crosses the lower-branch at ![]() $C = 12.5$. At this value of
$C = 12.5$. At this value of ![]() $C$, indeed, the switch to the convective flow appears to be immediate. Now, starting from this convection-driven flow and applying a lower
$C$, indeed, the switch to the convective flow appears to be immediate. Now, starting from this convection-driven flow and applying a lower ![]() $C$, the flow remains convection-driven turbulent for
$C$, the flow remains convection-driven turbulent for ![]() $C \geqslant 3.8$, or relaminarises for
$C \geqslant 3.8$, or relaminarises for ![]() $C \lessapprox 3.8$. This value of
$C \lessapprox 3.8$. This value of ![]() $C$ corresponds to the onset of the linear instability, which is responsible for the kink in
$C$ corresponds to the onset of the linear instability, which is responsible for the kink in ![]() $Nu$ as
$Nu$ as ![]() $C$ is decreased. Our previous analysis predicts that for flows on the left of (3.15), their
$C$ is decreased. Our previous analysis predicts that for flows on the left of (3.15), their ![]() $Re_{app}$ is greater than 2300, hence they may be prone to transition to turbulence subject to sufficient disturbances. Correspondingly, the lower-branch curve in figure 18 is plotted with a dashed line for
$Re_{app}$ is greater than 2300, hence they may be prone to transition to turbulence subject to sufficient disturbances. Correspondingly, the lower-branch curve in figure 18 is plotted with a dashed line for ![]() $C< C_{cr,2} = 3.4$ to indicate that in practice (e.g. in a laboratory experiment) the flow would become shear-driven turbulent again. However, as previously discussed, at this Reynolds number,
$C< C_{cr,2} = 3.4$ to indicate that in practice (e.g. in a laboratory experiment) the flow would become shear-driven turbulent again. However, as previously discussed, at this Reynolds number, ![]() $C_{cr,2}< C_{LS}$. Bistability (between shear or convection driven states) is thus observed for
$C_{cr,2}< C_{LS}$. Bistability (between shear or convection driven states) is thus observed for ![]() $3.8 \lessapprox C \lessapprox 7.5$. The latter value is in very good agreement with the threshold
$3.8 \lessapprox C \lessapprox 7.5$. The latter value is in very good agreement with the threshold ![]() $C_{cr,1}=7.1$ predicted above.
$C_{cr,1}=7.1$ predicted above.

Figure 18. Nusselt number versus ![]() $C$ for simulations started with shear and convection initial conditions (ICs) at
$C$ for simulations started with shear and convection initial conditions (ICs) at ![]() $Re=5300$. The magenta and cyan vertical lines correspond to the critical buoyancy parameters
$Re=5300$. The magenta and cyan vertical lines correspond to the critical buoyancy parameters ![]() $C_{cr,1}$ and
$C_{cr,1}$ and ![]() $C_{cr,2}$ given by (3.12) and (3.15), respectively. For values of
$C_{cr,2}$ given by (3.12) and (3.15), respectively. For values of ![]() $C \gtrapprox C_{cr_1}$ (
$C \gtrapprox C_{cr_1}$ (![]() $C \lessapprox C_{cr_2}$) the shear-driven (convection-driven) state is not supported and correspondingly the upper (lower) branch is plotted with a dashed semitransparent line.
$C \lessapprox C_{cr_2}$) the shear-driven (convection-driven) state is not supported and correspondingly the upper (lower) branch is plotted with a dashed semitransparent line.
In figures 19 and 20 the turbulent structures of the isothermal and heated flows at ![]() $Re=5300$,
$Re=5300$, ![]() $C=0$ and
$C=0$ and ![]() $5$, are compared with those of the EPG reference flow. The latter was computed by performing a DNS with fixed pressure gradient such that
$5$, are compared with those of the EPG reference flow. The latter was computed by performing a DNS with fixed pressure gradient such that ![]() $Re_p^{{\dagger} }=Re_p=10898.7$. The flow structures – streaks and vortices – are visualised as isosurfaces of streamwise velocity and streamwise vorticity fluctuations, normalised by the apparent friction velocity based on the pressure gradient component of the wall shear stress only,
$Re_p^{{\dagger} }=Re_p=10898.7$. The flow structures – streaks and vortices – are visualised as isosurfaces of streamwise velocity and streamwise vorticity fluctuations, normalised by the apparent friction velocity based on the pressure gradient component of the wall shear stress only, ![]() $u_{\tau p}^*$, where the asterisk
$u_{\tau p}^*$, where the asterisk ![]() $^*$ denotes a dimensional quantity here. The resulting apparent friction Reynolds number is
$^*$ denotes a dimensional quantity here. The resulting apparent friction Reynolds number is ![]() $Re_{\tau p}:=u_{\tau p}^* R^*/\nu ^* = Re_{\tau }^{{\dagger} }=147.6$.
$Re_{\tau p}:=u_{\tau p}^* R^*/\nu ^* = Re_{\tau }^{{\dagger} }=147.6$.

Figure 19. Three-dimensional visualisations of low (blue) and high (yellow) speed streaks in the isothermal (a), heated (b) and EPG (c) flows. Isosurfaces of turbulent streamwise velocity normalised by the corresponding apparent friction velocity ![]() $u_z'/u_{\tau p}=\pm 4$.
$u_z'/u_{\tau p}=\pm 4$.

Figure 20. Three-dimensional visualisations of vortical structures in the isothermal (a), heated (b) and EPG (c) flows. Isosurfaces of streamwise vorticity fluctuations normalised by the corresponding apparent friction velocity ![]() $\omega _z'/u_{\tau p}=\pm 35$.
$\omega _z'/u_{\tau p}=\pm 35$.
Comparison between the isothermal and heated flows show that the streaks are relatively unaffected, while vortices are significantly weakened. Our interpretation is that while the streaks are responsible for the saturation of the nonlinearity of the flow, via nonlinear normality of the mean flow (Waleffe Reference Waleffe1995), it is relatively ‘easy’ to produce streaks. Note that the mean axial flow for these cases is almost identical (figure 4), and at the end of § 3.3 large initial amplifications of disturbances remains possible in the heated case. It is observed that weaker vortices in the heated case are sufficient to produce saturated streaks of the same amplitude. Thus, vortices are more important in the sense that criticality for transition appears to occur when the vortices are too weak. Comparing now the heated flow with the EPG flow, consistent with the observations of HHS (see their figure 19), it can be seen that the streaks in the heated flow are typically stronger than in the EPG flow, while the vortices are of similar strength. In figure 21 we plot root mean square (r.m.s.) velocity fluctuations. Axial perturbations (figure 21a) are not strongly affected by the heating, while the cross-flow components (figure 21b) are significantly suppressed. (The plot for ![]() $u'_r$ is very similar to that shown for
$u'_r$ is very similar to that shown for ![]() $u'_\theta$.) In figure 21(c) it is seen that the heated and EPG flow have very similar cross-components, while axial perturbations in the heated case are slightly stronger than in the EPG flow. These results are consistent with observations from the three-dimensional visualisations of figures 19 and 20, and likewise suggest that it is the weakening of rolls rather than streaks that appear to be responsible for laminarisation.
$u'_\theta$.) In figure 21(c) it is seen that the heated and EPG flow have very similar cross-components, while axial perturbations in the heated case are slightly stronger than in the EPG flow. These results are consistent with observations from the three-dimensional visualisations of figures 19 and 20, and likewise suggest that it is the weakening of rolls rather than streaks that appear to be responsible for laminarisation.

Figure 21. The r.m.s. velocity fluctuations as a function of wall-normal distance ![]() $y=1-r$. (a) Here
$y=1-r$. (a) Here ![]() $u'_\theta$, a measure of ‘rolls’, are suppressed as
$u'_\theta$, a measure of ‘rolls’, are suppressed as ![]() $C$ increases, while (b)
$C$ increases, while (b) ![]() $u'_z$ a measure of ‘streaks’, are little changed. (c) Rolls for the
$u'_z$ a measure of ‘streaks’, are little changed. (c) Rolls for the ![]() $C=5$ case correspond closely to its EPG counterpart, while the heated case has slightly stronger streaks.
$C=5$ case correspond closely to its EPG counterpart, while the heated case has slightly stronger streaks.
4. Conclusions
In this paper we have studied the flow of fluid through a vertically aligned heated pipe using DNS, linear stability and nonlinear TW solution analyses. The flow is driven by an externally applied pressure gradient and aided by the buoyancy resulting from the lightening of the fluid close to the heated wall. Direct numerical simulations were performed for a range of Reynolds numbers ![]() $Re$ and buoyancy parameters
$Re$ and buoyancy parameters ![]() $C$, where the latter measures the magnitude of the buoyancy force relative to the pressure gradient of the laminar isothermal shear flow, At relatively low
$C$, where the latter measures the magnitude of the buoyancy force relative to the pressure gradient of the laminar isothermal shear flow, At relatively low ![]() $Re \lesssim 3500$ turbulence is completely suppressed (relaminarised) by buoyancy and as
$Re \lesssim 3500$ turbulence is completely suppressed (relaminarised) by buoyancy and as ![]() $C$ is increased convection starts driving a relatively quiescent flow. For larger
$C$ is increased convection starts driving a relatively quiescent flow. For larger ![]() $Re$, instead, the shear-driven turbulent flow transitions directly to the convection-driven state. Consistent with the appearance of the convective state observed in simulations, a linear instability was found at
$Re$, instead, the shear-driven turbulent flow transitions directly to the convection-driven state. Consistent with the appearance of the convective state observed in simulations, a linear instability was found at ![]() $C \approx 4$, roughly independent of
$C \approx 4$, roughly independent of ![]() $Re$ for most of the range considered. The result of increasing
$Re$ for most of the range considered. The result of increasing ![]() $C$ can be compared with that of increasing polymer concentration, or Weissenberg number
$C$ can be compared with that of increasing polymer concentration, or Weissenberg number ![]() $Wi$, which is known to have a drag reducing effect on turbulent flows (Virk et al. Reference Virk, Merrill, Mickley, Smith and Mollo-Christensen1967). Similar to our phase diagram (figure 7), a region of relatively quiescent flow has been reported for a certain range of
$Wi$, which is known to have a drag reducing effect on turbulent flows (Virk et al. Reference Virk, Merrill, Mickley, Smith and Mollo-Christensen1967). Similar to our phase diagram (figure 7), a region of relatively quiescent flow has been reported for a certain range of ![]() $Re$ and
$Re$ and ![]() $Wi$ (Choueiri, Lopez & Hof Reference Choueiri, Lopez and Hof2018; Lopez, Choueiri & Hof Reference Lopez, Choueiri and Hof2019), although the underlying physical mechanism (elastoinertial instability) is clearly very different from the one studied here (convection driven).
$Wi$ (Choueiri, Lopez & Hof Reference Choueiri, Lopez and Hof2018; Lopez, Choueiri & Hof Reference Lopez, Choueiri and Hof2019), although the underlying physical mechanism (elastoinertial instability) is clearly very different from the one studied here (convection driven).
Cases where turbulence is suppressed exhibit a flattened mean streamwise velocity profile. In agreement with recent observations by Kühnen et al. (Reference Kühnen, Song, Scarselli, Budanur, Riedl, Willis, Avila and Hof2018) and Marensi et al. (Reference Marensi, Willis and Kerswell2019) on the effect of flattening, we found that states that mediate turbulence (lower-branch TW solutions) are ‘pushed out’ from the laminar state, i.e. as ![]() $C$ increases, a larger perturbation amplitude or larger
$C$ increases, a larger perturbation amplitude or larger ![]() $Re$ are required to drive shear turbulence until, for sufficiently large
$Re$ are required to drive shear turbulence until, for sufficiently large ![]() $C$, the TW is suppressed altogether.
$C$, the TW is suppressed altogether.
Finally, we used the relaminarisation criterion recently proposed by HHS, based on an ‘apparent Reynolds number’ of the flow, to predict the critical ![]() $C=C_{cr,1}(Re)$ above which the flow will be laminarised or switch to the convection-driven type. This apparent Reynolds number is based on an apparent friction velocity associated with only the pressure force of the flow (i.e. excluding the contribution of the body force/buoyancy). Bistability between shear or convection-driven states was found to occur in the region
$C=C_{cr,1}(Re)$ above which the flow will be laminarised or switch to the convection-driven type. This apparent Reynolds number is based on an apparent friction velocity associated with only the pressure force of the flow (i.e. excluding the contribution of the body force/buoyancy). Bistability between shear or convection-driven states was found to occur in the region ![]() $4 \lesssim C \lesssim C_{cr,1}$ where the flow may or may not be laminarised depending on the initial flow of the simulation or experiment.
$4 \lesssim C \lesssim C_{cr,1}$ where the flow may or may not be laminarised depending on the initial flow of the simulation or experiment.
Comparison of the turbulent flow structures (rolls and streaks) with those of two reference flows – the flow of equivalent pressure gradient and that of equivalent mass flux – suggests that near criticality for relaminarisation the vortices, rather than the streaks, are more important in the sense that criticality for transition occurs when the vortices are too weak. This picture is not straightforward to reconcile with the interpretation of Kühnen et al. (Reference Kühnen, Song, Scarselli, Budanur, Riedl, Willis, Avila and Hof2018), where relaminarisation is attributed to reduced ability to produce streaks in the presence of the flattened base profile. In the heated case, the base velocity profile does not appear to change significantly while shear-driven turbulence is present. Thus it appears unlikely that transient growth of streaks is affected by the heating. Indeed, laminarisation occurs despite little suppression of the streaks. The experiments of Kühnen et al. (Reference Kühnen, Song, Scarselli, Budanur, Riedl, Willis, Avila and Hof2018) are slightly different, however, in that the various flow manipulations they introduce do change the base profile of the flow. In that case it is correct that transient growth will be affected, although we conjecture that it is the suppression of the vortices due to suppression of the streaks that is responsible for laminarisation in that case. Their numerical experiments in the presence of a force are very similar to the calculations here and of HHS. In that case we expect the mechanism we have described to be more clearly responsible for the laminarisation.
Acknowledgements
The anonymous referees are kindly acknowledged for their useful suggestions and comments.
Funding
This work was funded by EPSRC grant EP/P000959/1.
Declaration of interests
The authors report no conflict of interest.
Appendix A. Link between upward-heated and downward-cooled cases
Consider the axial force from the pressure gradient and buoyancy terms in (2.9). Ignoring the factor ![]() $4/Re$ that multiplies all terms, let
$4/Re$ that multiplies all terms, let
with ![]() $C>0$ for the upward heated case on the left-hand side. Let the right-hand side represent the downward cooled case, taking
$C>0$ for the upward heated case on the left-hand side. Let the right-hand side represent the downward cooled case, taking ![]() $\tilde {\varTheta }=1-\varTheta$ so that
$\tilde {\varTheta }=1-\varTheta$ so that ![]() $\tilde {\varTheta }$ is coolest on the boundary (
$\tilde {\varTheta }$ is coolest on the boundary (![]() $\tilde {\varTheta }=1-r^2$ for the laminar case). Put
$\tilde {\varTheta }=1-r^2$ for the laminar case). Put ![]() $\tilde {C}=-C<0$, as buoyancy due to positive temperature variations oppose the pressure gradient. (Cooling, however, aids the downward flow.) Substituting in (A1) we find
$\tilde {C}=-C<0$, as buoyancy due to positive temperature variations oppose the pressure gradient. (Cooling, however, aids the downward flow.) Substituting in (A1) we find ![]() $\tilde {\beta }=\beta +C$, i.e. the systems differ only by a known offset in the pressure gradient required to maintain volume flux.
$\tilde {\beta }=\beta +C$, i.e. the systems differ only by a known offset in the pressure gradient required to maintain volume flux.
Appendix B. Turbulent base flow and eddy viscosity
The turbulent mean flow profile for a pipe may be written ![]() $\boldsymbol {U}=U(y)\hat {\boldsymbol {z}}$, where
$\boldsymbol {U}=U(y)\hat {\boldsymbol {z}}$, where ![]() $y=1-r$ is the dimensionless distance from the boundary wall and
$y=1-r$ is the dimensionless distance from the boundary wall and ![]() $r$ is the radial coordinate. Applying the Boussinesq eddy viscosity to model for the turbulent Reynolds-stresses, the streamwise component of the Reynolds-averaged momentum conservation reads
$r$ is the radial coordinate. Applying the Boussinesq eddy viscosity to model for the turbulent Reynolds-stresses, the streamwise component of the Reynolds-averaged momentum conservation reads
where the total effective viscosity is ![]() $\nu _T(y)=1+\nu _{t}(y)$ and
$\nu _T(y)=1+\nu _{t}(y)$ and ![]() $\nu _{t}$ is the eddy-viscosity, normalised such that
$\nu _{t}$ is the eddy-viscosity, normalised such that ![]() $\nu _T(0)=1$, i.e. the kinematic value is attained at the wall.
$\nu _T(0)=1$, i.e. the kinematic value is attained at the wall.
To calculate ![]() $\nu _{t}$ it is convenient to use the expression originally suggested for pipe flow by Cess (Reference Cess1958), later used for channel flows by Reynolds & Tiederman (Reference Reynolds and Tiederman1967) and then by many others (Butler & Farrell Reference Butler and Farrell1993; Del Alamo & Jimenez Reference Del Alamo and Jimenez2006; Pujals et al. Reference Pujals, García-Villalba, Cossu and Depardon2009),
$\nu _{t}$ it is convenient to use the expression originally suggested for pipe flow by Cess (Reference Cess1958), later used for channel flows by Reynolds & Tiederman (Reference Reynolds and Tiederman1967) and then by many others (Butler & Farrell Reference Butler and Farrell1993; Del Alamo & Jimenez Reference Del Alamo and Jimenez2006; Pujals et al. Reference Pujals, García-Villalba, Cossu and Depardon2009),
 \begin{align} \nu_{t}(y) = \frac{1}{2} \left\{ 1 + \frac{\kappa^2 \hat{R}^2 \hat{B}}{9} \left(2y-y^2\right)^2 \left(3-4y+2y^2\right)^2 \left[ 1 - \exp\left({\frac{-y \hat{R} \sqrt{\hat{B}}}{A^+}}\right) \right]^2\right\} ^{{1}/{2}} - \frac{1}{2} . \end{align}
\begin{align} \nu_{t}(y) = \frac{1}{2} \left\{ 1 + \frac{\kappa^2 \hat{R}^2 \hat{B}}{9} \left(2y-y^2\right)^2 \left(3-4y+2y^2\right)^2 \left[ 1 - \exp\left({\frac{-y \hat{R} \sqrt{\hat{B}}}{A^+}}\right) \right]^2\right\} ^{{1}/{2}} - \frac{1}{2} . \end{align}
Here, ![]() $\hat {R}=Re/2$,
$\hat {R}=Re/2$, ![]() $\hat {B}=2B$, with
$\hat {B}=2B$, with ![]() $B = -\partial _{z}P$ being the averaged streamwise pressure gradient. The parameters
$B = -\partial _{z}P$ being the averaged streamwise pressure gradient. The parameters ![]() $A^+=27$ and
$A^+=27$ and ![]() $\kappa =0.42$ have been chosen to fit the more recent observations of McKeon, Zagarola & Smits (Reference McKeon, Zagarola and Smits2005).
$\kappa =0.42$ have been chosen to fit the more recent observations of McKeon, Zagarola & Smits (Reference McKeon, Zagarola and Smits2005).
For the calculation of § 3.4, the (apparent) pressure gradient ![]() $B$ and (apparent)
$B$ and (apparent) ![]() $Re_p$ are known. The mass flux
$Re_p$ are known. The mass flux ![]() $Re$ of (B1) is not yet known, and we wish to determine
$Re$ of (B1) is not yet known, and we wish to determine ![]() $\nu _t$. An initial estimate for
$\nu _t$. An initial estimate for ![]() $Re$ is obtained from the approximation of Blasius (Reference Blasius1913), which may be written
$Re$ is obtained from the approximation of Blasius (Reference Blasius1913), which may be written
Then, (B2) can be used to calculate ![]() $\nu _t(r)$, but we must check consistency with (B1). The latter equation can be inverted for
$\nu _t(r)$, but we must check consistency with (B1). The latter equation can be inverted for ![]() $U(r)$, and, as it has been non-dimensionalised with the same scales of § 2.1, the mean velocity
$U(r)$, and, as it has been non-dimensionalised with the same scales of § 2.1, the mean velocity ![]() $U_b=2\int _0^1 U(r)\,r\,\mathrm {d}r$ should be
$U_b=2\int _0^1 U(r)\,r\,\mathrm {d}r$ should be ![]() $0.5$. It will not be exactly so, as
$0.5$. It will not be exactly so, as ![]() $Re$ (for the given
$Re$ (for the given ![]() $\partial _zP$) has only been estimated. A better estimate is given by
$\partial _zP$) has only been estimated. A better estimate is given by ![]() $Re := (0.5/U_b)\,Re$, so that
$Re := (0.5/U_b)\,Re$, so that ![]() $\nu _t$ can be recalculated and iteratively improved.
$\nu _t$ can be recalculated and iteratively improved.