Article contents
WARING’S PROBLEM WITH SHIFTS
Published online by Cambridge University Press: 06 March 2015
Abstract
Let 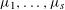 ${\it\mu}_{1},\ldots ,{\it\mu}_{s}$ be real numbers, with
${\it\mu}_{1},\ldots ,{\it\mu}_{s}$ be real numbers, with 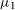 ${\it\mu}_{1}$ irrational. We investigate sums of shifted
${\it\mu}_{1}$ irrational. We investigate sums of shifted  $k$th powers
$k$th powers 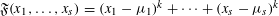 $\mathfrak{F}(x_{1},\ldots ,x_{s})=(x_{1}-{\it\mu}_{1})^{k}+\cdots +(x_{s}-{\it\mu}_{s})^{k}$. For
$\mathfrak{F}(x_{1},\ldots ,x_{s})=(x_{1}-{\it\mu}_{1})^{k}+\cdots +(x_{s}-{\it\mu}_{s})^{k}$. For 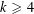 $k\geqslant 4$, we bound the number of variables needed to ensure that if
$k\geqslant 4$, we bound the number of variables needed to ensure that if  ${\it\eta}$ is real and
${\it\eta}$ is real and 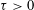 ${\it\tau}>0$ is sufficiently large then there exist integers
${\it\tau}>0$ is sufficiently large then there exist integers  $x_{1}>{\it\mu}_{1},\ldots ,x_{s}>{\it\mu}_{s}$ such that
$x_{1}>{\it\mu}_{1},\ldots ,x_{s}>{\it\mu}_{s}$ such that 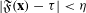 $|\mathfrak{F}(\mathbf{x})-{\it\tau}|<{\it\eta}$. This is a real analogue to Waring’s problem. When
$|\mathfrak{F}(\mathbf{x})-{\it\tau}|<{\it\eta}$. This is a real analogue to Waring’s problem. When 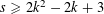 $s\geqslant 2k^{2}-2k+3$, we provide an asymptotic formula. We prove similar results for sums of general univariate degree-
$s\geqslant 2k^{2}-2k+3$, we provide an asymptotic formula. We prove similar results for sums of general univariate degree-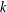 $k$ polynomials.
$k$ polynomials.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2015
References
- 4
- Cited by




