Hostname: page-component-76fb5796d-2lccl Total loading time: 0 Render date: 2024-04-27T16:09:52.429Z Has data issue: false hasContentIssue false
  • English
  • Français

On an algebra of sets of finite sequences 1

Published online by Cambridge University Press:  12 March 2014

J. Donald Monk
J. Donald Monk*
Affiliation:
University of Colorado
Get access Rights & Permissions [Opens in a new window]

Extract

The algebras studied in this paper were suggested to the author by William Craig as a possible substitute for cylindric algebras. Both kinds of algebras may be considered as algebraic versions of first-order logic. Cylindric algebras can be introduced as follows. Let ℒ be a first-order language, and let 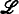be an ℒ-structure. We assume that ℒ has a simple infinite sequence ν 0, ν 1, … of individual variables, and we take as known what it means for a sequence ν 0, ν 1, … of individual variables, and we take as known what it means for a sequence x = 〈x 0, x 1, …〉 of elements of 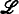to satisfy a formula ϕ of ℒ in 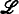. Let ϕ 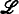be the collection of all sequences x which satisfy ϕ in 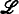. We can perform certain natural operations on the sets ϕ 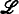, of basic model-theoretic significance: Boolean operations =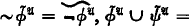 cylindrifications 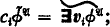diagonal elements (0-ary operations) . In this way we make the class of all sets ϕ 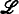into an algebra; a natural abstraction gives the class 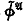of all cylindric set algebras (of dimension ω). Thus this method of constructing an algebraic counterpart of first-order logic is based upon the notion of satisfaction of a formula by an infinite sequence of elements. Since, however, a formula has only finitely many variables occurring in it, it may seem more natural to consider satisfaction by a finite sequence of elements; then ϕ 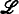becomes a collection of finite sequences of varying ranks (cf. Tarski [10]). In forming an algebra of sets of finite sequences it turns out to be possible to get by with only finitely many operations instead of the infinitely many ci 's and dij 's of cylindric algebras. Let 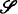be the class of all algebras of sets of finite sequences (an exact definition is given in §1).

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 35 , Issue 1 , March 1970 , pp. 19 - 28
Copyright
Copyright © Association for Symbolic Logic 1970

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

1

Research supported in part by NSF grants GP7387 and GP6232-x.

References

[1] Bernays, P., Über eine natürliche Erweiterung des Relationenkalkuh, Constructivity in mathematics, North-Holland, Amsterdam, 1957, pp. 1–14.Google Scholar
[2] Copeland, A. H. Sr., A note on cylindric and polyadic algebras, The Michigan mathematical journal, vol. 3 (1955), pp. 155–157.CrossRefGoogle Scholar
[3] Craig, W., Boolean notions extended to higher dimensions, Theory of models, North-Holland, Amsterdam, 1965, pp. 55–69.Google Scholar
[4] Frayne, T., Morel, A. and Scott, D., Reduced direct products, Fundamenta mathematicae, vol. 51 (1962), pp. 195–228.CrossRefGoogle Scholar
[5] Henkin, L. and Tarski, A., Cylindric algebras, Lattice theory, Proceedings of the Symposium in Pure Mathematics, vol. 2, Amer. Math. Soc., 1961, 83–113.CrossRefGoogle Scholar
[6] Henkin, L., Monk, J. D. and Tarski, A., Cylindric algebras. Part I, North-Holland, Amsterdam (to appear).CrossRefGoogle Scholar
[7] Johnson, J., Nonfinitizability of classes of representable polyadic algebras, this Journal, vol. 34 (1969), pp. 344–352.Google Scholar
[8] Monk, J. D., On representable relation algebras, The Michigan mathematical journal, vol. 11 (1964), pp. 207–210.CrossRefGoogle Scholar
[9] Monk, J. D., Nonfinitizability of classes of representable cylindric algebras, this Journal, vol. 34 (1969), pp. 331–343.Google Scholar
[10] Tarski, A., Der Wahrheitsbegriff in den formatierten Sprachen, Studia phil., vol. 1 (1935), pp. 261–405.Google Scholar