Hostname: page-component-8448b6f56d-tj2md Total loading time: 0 Render date: 2024-04-24T08:21:59.454Z Has data issue: false hasContentIssue false
  • English
  • Français

Trace Class Elements and Cross-Sections in Kac-Moody Groups

Published online by Cambridge University Press:  20 November 2018

Gerd Brüchert
Gerd Brüchert*
Affiliation:
Mathematisches Seminar, Universität Hamburg, Bundesstrasse, 55, 20146 Hamburg, Germany email: bruechert@math.uni-hamburg.de
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

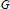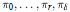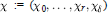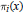Let $G$ be an affine Kac-Moody group, ${{\pi }_{0}},...,{{\pi }_{r}},{{\pi }_{\delta }}$ its fundamental irreducible representations and ${{\chi }_{0}},...,{{\chi }_{r}},{{\chi }_{\delta }}$ their characters. We determine the set of all group elements $x$ such that all ${{\pi }_{i}}(x)$ act as trace class operators, i.e., such that 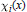${{\chi }_{i}}(x)$ exists, then prove that the ${{\chi }_{i}}$ are class functions. Thus, (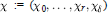$\chi \,:=\,({{\chi }_{0}},...,{{\chi }_{r}},\,{{\chi }_{\delta }})$)factors to an adjoint quotient $\bar{\chi }$ for 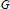$G$. In a second part, following Steinberg, we define a cross-section $C$ for the potential regular classes in 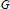$G$. We prove that the restriction 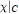$\chi \text{ }\!\!|\!\!\text{ }c$ behaves well algebraically. Moreover, we obtain an action of 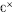${{\mathbb{C}}^{\times }}$ on $C$, which leads to a functional identity for 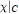$\text{ }\chi |\text{ c}$ which shows that 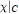$\text{ }\chi |\text{ c}$ is quasi-homogeneous.

Keywords

22E6517B65
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 50 , Issue 5 , 01 October 1998 , pp. 972 - 1006
Copyright
Copyright © Canadian Mathematical Society 1998

References

[B] Brüchert, G., Steinberg-Querschnitte und Spurklassenelemente in Kac-Moody-Gruppen. Ph.D. thesis, University of Hamburg, Germany, 1995.Google Scholar
[BLM] Berman, S., Lee, Y.S. and Moody, R.V., The Spectrum of a Coxeter Transformation, Affine Coxeter Transformations and the Defect Map. J. Algebra 121(1989), 339–357.Google Scholar
[C] Carter, R.W., Simple Groups of Lie Type. Wiley and Sons, NY, 1971.Google Scholar
[Co] Coleman, A.J., Killing and the Coxeter Transformation of Kac-Moody Algebras. Invent.Math. 95(1989), 447–477.Google Scholar
[Con] Conway, J.B., A Course in Functional Analysis. GTM 96, Springer Verlag, 1985.Google Scholar
[FLM] Frenkel, I., Lepowsky, J. and Meurman, A., Vertex Operator Algebras and the Monster. Academic Press, 1988.Google Scholar
[G] Garland, H., The Arithmetic Theory of Loop Groups. Inst. Hautes Études Sci. Publ. Math. 52(1980), 5–136.Google Scholar
[Go] Goldberg, S., Unbounded Linear Operators. Dover, 1985.Google Scholar
[H] Humphreys, J.E., Reflection Groups and Coxeter Groups. Cambridge University Press, 1990.Google Scholar
[K] Kac, V.G., Infinite dimensional Lie Algebras. 3rd edn, Cambridge University Press, 1990.Google Scholar
[K2] Kac, V.G., Constructing Groups Associated to Infinite-dimensional Lie Algebras. In: Infinite dimensional groups with applications, Math. Sci. Res. Inst. Publ. 4, Springer, 1985.Google Scholar
[KP] Kac, V.G. and Peterson, D.H., Defining relations of certain infinite dimensional groups. In: Elie Cartan et les Mathématiques d’aujourd’hui, Astérisque, Numéro hors série, 1985. 165–208.Google Scholar
[MP] Moody, R.V. and Pianzola, A., Lie Algebras with Triangular Decompositions. Canadian Mathematical Society Series of Monographs and Advanced Texts, J. Wiley, NY, 1995.Google Scholar
[PK] Peterson, D.H. and Kac, V.G., Infinite flag varieties and conjugacy theorems. Proc.Natl. Acad. Sci. USA 80(1983), 1778.ndash;1782.Google Scholar
[S1] Slodowy, P., Simple Singularities and Simple Algebraic Groups. Lecture Notes in Math. 815, Springer, 1980.Google Scholar
[S2] Slodowy, P., Chevalley Groups over C((t)) and deformations of simply elliptic singularities. In: Algebraic Geometry (Eds. Aroca, Buchweitz, Giusti and Merle), Lecture Notes in Math. 961, Springer, 1982.Google Scholar
[S3] Slodowy, P., Singularitäten, Kac-Moody-Algebren, assoziierte Gruppen und Verallgemeinerungen. Habilitationsschrift, Universität Bonn, 1984.Google Scholar
[S4] Slodowy, P., An Adjoint Quotient for Certain Groups Attached to Kac-Moody Algebras. In: Infinite dimensional groups with applications, Math. Sci. Res. Inst. Publ. 4, Springer, 1985. 307–334.Google Scholar
[Sle] Slebarski, S. and Slodowy, P., Informal notes on the SERC project GR/D98655. University of Liverpool, July 1987–Oct. 1989.Google Scholar
[St1] Steinberg, R., Conjugacy Classes in Algebraic Groups. Lecture Notes in Math. 366, Springer, 1974.Google Scholar
[St2] Steinberg, R., Regular Elements of Semisimple Algebraic Groups. Inst. Hautes Études Sci. Publ. Math. 25(1965), 49–80.Google Scholar
[St3] Steinberg, R., Finite Subgroups of SU(2), Dynkin Diagrams and Affine Coxeter Elements. Pacific J. Math. 118(1985), 587–598.Google Scholar