Hostname: page-component-76fb5796d-5g6vh Total loading time: 0 Render date: 2024-04-26T16:47:07.868Z Has data issue: false hasContentIssue false
  • English
  • Français

On some generalisations of the Erdős distance problem over finite fields

Published online by Cambridge University Press:  17 April 2009

Igor E. Shparlinski
Igor E. Shparlinski
Affiliation:
Department of Computing, Macquarie University, Sydney, NSW 2109, Australia e-mail igor@ics.mq.edu.au
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We use exponential sums to obtain new lower bounds on the number of distinct distances defined by all pairs of points (a, b) ∈ A × B for two given sets 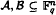where 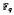is a finite field of q elements and n ≥ 1 is an integer.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 73 , Issue 2 , April 2006 , pp. 285 - 292
Copyright
Copyright © Australian Mathematical Society 2006

References

[1]Erdogan, M.B., ‘A bilinear Fourier extension theorem and applications to the distance set problem’, Intern. Math. Res. Notices 23 (2005), 1411–1425.CrossRefGoogle Scholar
[2]Hofmann, S. and Iosevich, A., ‘Circular averages and Falconer/Erdős distance conjecture in the plane for random metrics’, Proc. Amer. Mat. Soc. 133 (2005), 133–143.Google Scholar
[3]Iosevich, A. and Laba, I., ‘Distance sets of well-distributed planar sets’, Discrete Comput. Geom. 31 (2004), 243–250.Google Scholar
[4]Iosevich, A. and Rudnev, M., ‘Spherical averages, distance sets, and lattice points on convex surfaces’, (preprint, 2005).Google Scholar
[5]Iosevich, A. and Rudnev, M., ‘Erdős distance problem in vector spaces over finite fields’, Trans. Amer. Math. Soc. (to appear).Google Scholar
[6]Katz, N. and Tardos, G., ‘A new entropy inequality for the Erdős distance problem’, in Towards a theory of geometric graphs, Contemp. Math., 342 (Amer. Math. Soc., Providence, RI, 2004), pp. 119–126.Google Scholar
[7]Lidl, R. and Niederreiter, H., Finite fields (Cambridge University Press, Cambridge, 1997).Google Scholar