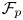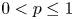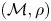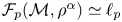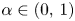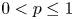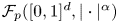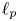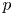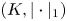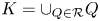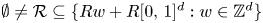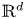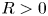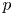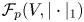1. Introduction
Given a pointed metric space $\mathcal {M}$![]() , there exists a Banach space $\mathcal {F}(\mathcal {M})$
, there exists a Banach space $\mathcal {F}(\mathcal {M})$![]() , called the Lipschitz-free space over $\mathcal {M}$
, called the Lipschitz-free space over $\mathcal {M}$![]() , such that $\mathcal {M}$
, such that $\mathcal {M}$![]() embeds isometrically into $\mathcal {F}(\mathcal {M})$
embeds isometrically into $\mathcal {F}(\mathcal {M})$![]() via a map $\delta :\mathcal {M} \to \mathcal {F}(\mathcal {M})$
via a map $\delta :\mathcal {M} \to \mathcal {F}(\mathcal {M})$![]() , and for every Banach space $Y$
, and for every Banach space $Y$![]() and a Lipschitz map $f:\mathcal {M}\to Y$
and a Lipschitz map $f:\mathcal {M}\to Y$![]() which vanishes at the origin, $f$
which vanishes at the origin, $f$![]() extends uniquely to a linear operator $T_f : \mathcal {F}(\mathcal {M})\to Y$
extends uniquely to a linear operator $T_f : \mathcal {F}(\mathcal {M})\to Y$![]() such that $\operatorname {Lip} f = \lVert T_f\rVert$
such that $\operatorname {Lip} f = \lVert T_f\rVert$![]() .
.
Lipschitz-free spaces are distinguished by their ability to relate the classical linear theory to the non-linear geometry of Banach spaces. This line of research can be traced back to the seminal paper by Godefroy and Kalton [Reference Godefroy and Kalton8], where Lipschitz-free spaces were identified as a natural class of objects for studying the deep classical problem of whether two Lipschitz isomorphic Banach spaces are linearly isomorphic.
To give an application of the theory, we note the authors were able to establish that whenever $X$![]() is a separable Banach space and $X$
is a separable Banach space and $X$![]() embeds into a Banach space $Y$
embeds into a Banach space $Y$![]() isometrically, then there exists a linear isometric embedding of $X$
isometrically, then there exists a linear isometric embedding of $X$![]() into $Y$
into $Y$![]() . Similarly, they used the universal extension property of Lipschitz-free spaces to show that a bounded approximation property of a Banach space is preserved merely by Lipschitz isomorphisms. Let us remark that the study of approximation properties and the non-linear geometry of Banach spaces is an ongoing topic (see [Reference Albiac, Ansorena, Cúth and Doucha4, Reference Borel-Mathurin7, Reference Godefroy9, Reference Hájek and Medina10, Reference Kalton13, Reference Pernecká and Smith16]).
. Similarly, they used the universal extension property of Lipschitz-free spaces to show that a bounded approximation property of a Banach space is preserved merely by Lipschitz isomorphisms. Let us remark that the study of approximation properties and the non-linear geometry of Banach spaces is an ongoing topic (see [Reference Albiac, Ansorena, Cúth and Doucha4, Reference Borel-Mathurin7, Reference Godefroy9, Reference Hájek and Medina10, Reference Kalton13, Reference Pernecká and Smith16]).
In the context of the Lipschitz isomorphism problem, Albiac and Kalton [Reference Albiac and Kalton1] later came with an example of two separable $p$![]() -Banach spaces, for each $0< p<1$
-Banach spaces, for each $0< p<1$![]() , which are Lipschitz isomorphic but fail to be linearly isomorphic. As it turns out, the counterexample to the generalized variant of the Lipschitz isomorphism problem could be developed in the setting of generalized Lipschitz-free spaces, coined the Lipschitz-free $p$
, which are Lipschitz isomorphic but fail to be linearly isomorphic. As it turns out, the counterexample to the generalized variant of the Lipschitz isomorphism problem could be developed in the setting of generalized Lipschitz-free spaces, coined the Lipschitz-free $p$![]() -spaces.
-spaces.
For each $0< p\leq 1$![]() , the Lipschitz-free $p$
, the Lipschitz-free $p$![]() -space $\mathcal {F}_p (\mathcal {M})$
-space $\mathcal {F}_p (\mathcal {M})$![]() over a metric space $\mathcal {M}$
over a metric space $\mathcal {M}$![]() is a $p$
is a $p$![]() -Banach space into which $\mathcal {M}$
-Banach space into which $\mathcal {M}$![]() isometrically embeds, and such that for every $p$
isometrically embeds, and such that for every $p$![]() -Banach space $Y$
-Banach space $Y$![]() and a Lipschitz map $f:\mathcal {M}\to Y$
and a Lipschitz map $f:\mathcal {M}\to Y$![]() which vanishes at the origin, $f$
which vanishes at the origin, $f$![]() extends uniquely to a linear operator $T_f : \mathcal {F}_p(\mathcal {M})\to Y$
extends uniquely to a linear operator $T_f : \mathcal {F}_p(\mathcal {M})\to Y$![]() with $\operatorname {Lip} f = \lVert T_f\rVert$
with $\operatorname {Lip} f = \lVert T_f\rVert$![]() . We note that a thorough study of Lipschitz-free $p$
. We note that a thorough study of Lipschitz-free $p$![]() -space was recently initiated in [Reference Albiac, Ansorena, Cúth and Doucha2].
-space was recently initiated in [Reference Albiac, Ansorena, Cúth and Doucha2].
The locally non-convex geometry of Lipschitz-free $p$![]() -spaces is rather challenging to grasp. As evidence, we note that for any subspace $\mathcal {N}$
-spaces is rather challenging to grasp. As evidence, we note that for any subspace $\mathcal {N}$![]() of a metric space $\mathcal {M}$
of a metric space $\mathcal {M}$![]() , it is straightforward to show that $\mathcal {F}_1 (\mathcal {N})$
, it is straightforward to show that $\mathcal {F}_1 (\mathcal {N})$![]() embeds isometrically into $\mathcal {F}_1 (\mathcal {M})$
embeds isometrically into $\mathcal {F}_1 (\mathcal {M})$![]() via a canonical linearization of the inclusion map $i: \mathcal {N} \to \mathcal {M}$
via a canonical linearization of the inclusion map $i: \mathcal {N} \to \mathcal {M}$![]() . However, this is not the case for $p<1$
. However, this is not the case for $p<1$![]() , and it remains an open question whether the inclusion in general is an isomorphic embedding, see [Reference Albiac, Ansorena, Cúth and Doucha2, Theorem 6.1 and Question 6.2], respectively.
, and it remains an open question whether the inclusion in general is an isomorphic embedding, see [Reference Albiac, Ansorena, Cúth and Doucha2, Theorem 6.1 and Question 6.2], respectively.
A distinctive feature of the $p<1$![]() theory is that a duality argument is no longer at our disposal, and we instead have to proceed by a direct geometrical construction in the Lipschitz-free $p$
theory is that a duality argument is no longer at our disposal, and we instead have to proceed by a direct geometrical construction in the Lipschitz-free $p$![]() -space itself. Moreover, strict concavity of a $p$
-space itself. Moreover, strict concavity of a $p$![]() -norm for $p<1$
-norm for $p<1$![]() typically introduces a dimensionality factor into the proof work; typically, this would render many of the techniques developed within the vast literature dedicated to approximation properties of Lipschitz-free spaces hardly adaptable.
typically introduces a dimensionality factor into the proof work; typically, this would render many of the techniques developed within the vast literature dedicated to approximation properties of Lipschitz-free spaces hardly adaptable.
Here we consider two open questions on the structure of Lipschitz-free $p$![]() -spaces over subsets of finite-dimensional normed spaces. In particular, we expound the extent to which selected results from the classical $p=1$
-spaces over subsets of finite-dimensional normed spaces. In particular, we expound the extent to which selected results from the classical $p=1$![]() theory generalize to the $0< p\leq 1$
theory generalize to the $0< p\leq 1$![]() scale.
scale.
Theorem 1 cf. Theorem 4.9
Let $(\mathcal {M}, \rho )$![]() be an infinite doubling metric space (e.g. an infinite subset of an Euclidean space) and $0 < \alpha < 1,$
be an infinite doubling metric space (e.g. an infinite subset of an Euclidean space) and $0 < \alpha < 1,$![]() $0< p\leq 1$
$0< p\leq 1$![]() . Then $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha )$
. Then $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha )$![]() is isomorphic to the space $\ell _p$
is isomorphic to the space $\ell _p$![]() .
.
A classical result in the theory of Lipschitz-free spaces states that if $|\cdot |$![]() is a norm on $\mathbb {R}^d$
is a norm on $\mathbb {R}^d$![]() and $\mathcal {M}$
and $\mathcal {M}$![]() is an infinite bounded subset of $\mathbb {R}^d$
is an infinite bounded subset of $\mathbb {R}^d$![]() endowed with the snowflake metric $|\cdot |^\alpha$
endowed with the snowflake metric $|\cdot |^\alpha$![]() , where $0<\alpha <1$
, where $0<\alpha <1$![]() , then $\mathcal {F}_1 (\mathcal {M}, |\cdot |^\alpha )\simeq \ell _1$
, then $\mathcal {F}_1 (\mathcal {M}, |\cdot |^\alpha )\simeq \ell _1$![]() .
.
The standard approach (consider e.g. [Reference Weaver18]) is to identify an isometric predual of $\mathcal {F}_1 (\mathcal {M}, |\cdot |^\alpha )$![]() as the subspace $\operatorname {lip}_0 (\mathcal {M}, |\cdot |^\alpha )$
as the subspace $\operatorname {lip}_0 (\mathcal {M}, |\cdot |^\alpha )$![]() consisting of little Lipschitz functions in the Lipschitz dual $\operatorname {Lip}_0 (\mathcal {M}, |\cdot |^\alpha )\simeq \mathcal {F}_1^* (\mathcal {M}, |\cdot |^\alpha )$
consisting of little Lipschitz functions in the Lipschitz dual $\operatorname {Lip}_0 (\mathcal {M}, |\cdot |^\alpha )\simeq \mathcal {F}_1^* (\mathcal {M}, |\cdot |^\alpha )$![]() . The proof then proceeds by constructing an isomorphism between $\operatorname {lip}_0 (\mathcal {M}, |\cdot |^\alpha )$
. The proof then proceeds by constructing an isomorphism between $\operatorname {lip}_0 (\mathcal {M}, |\cdot |^\alpha )$![]() and the space $c_0$
and the space $c_0$![]() ; this is an earlier result which traces back to, e.g. [Reference Bonic, Frampton and Tromba6].
; this is an earlier result which traces back to, e.g. [Reference Bonic, Frampton and Tromba6].
More recently, the result was generalized in [Reference Albiac, Ansorena, Cúth and Doucha3] to infinite subsets of $\mathbb {R}^d$![]() . In particular, an observation was made showing that for any $0< p\leq 1$
. In particular, an observation was made showing that for any $0< p\leq 1$![]() , if $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )$
, if $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )$![]() is isomorphic to the space $\ell _p$
is isomorphic to the space $\ell _p$![]() , then $\mathcal {F}_p (\mathcal {M}, |\cdot |^\alpha )\simeq \ell _p$
, then $\mathcal {F}_p (\mathcal {M}, |\cdot |^\alpha )\simeq \ell _p$![]() for any infinite subset $\mathcal {M}$
for any infinite subset $\mathcal {M}$![]() of $\mathbb {R}^d$
of $\mathbb {R}^d$![]() (and, by the Assouad embedding theorem, to snowflake distortions of infinite doubling metric spaces). The authors claimed that the ideas from the standard $p=1$
(and, by the Assouad embedding theorem, to snowflake distortions of infinite doubling metric spaces). The authors claimed that the ideas from the standard $p=1$![]() argument adapt to yield the isomorphism $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )\simeq \ell _p$
argument adapt to yield the isomorphism $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )\simeq \ell _p$![]() for $d=1$
for $d=1$![]() and $0< p\leq 1$
and $0< p\leq 1$![]() . However, for $d\geq 2$
. However, for $d\geq 2$![]() the available techniques turned insufficient and the problem remained open, see [Reference Albiac, Ansorena, Cúth and Doucha3, Question 6.8].
the available techniques turned insufficient and the problem remained open, see [Reference Albiac, Ansorena, Cúth and Doucha3, Question 6.8].
Here we tackle the multidimensional structure of $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )$![]() , and unlike the standard proof for $p=1$
, and unlike the standard proof for $p=1$![]() , we set up an explicit linear bijection between $p$
, we set up an explicit linear bijection between $p$![]() -norming sets in the respective spaces. As it turns out, the basis shares the form with the Schauder basis of $\mathcal {F}_p ([0,\,1]^d)$
-norming sets in the respective spaces. As it turns out, the basis shares the form with the Schauder basis of $\mathcal {F}_p ([0,\,1]^d)$![]() , see [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8].
, see [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8].
It is also interesting to note that our approach gives an estimate on the Banach–Mazur distance between $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )$![]() and $\ell _p$
and $\ell _p$![]() whenever $|\cdot |$
whenever $|\cdot |$![]() is identified as the $\ell _1$
is identified as the $\ell _1$![]() -norm, which is a new detail even for the case $p=1$
-norm, which is a new detail even for the case $p=1$![]() . An interested reader may want to compare the upper bound of $(4 d^{2-\alpha } c(\alpha ) )^d$
. An interested reader may want to compare the upper bound of $(4 d^{2-\alpha } c(\alpha ) )^d$![]() with a lower bound of $c'(\alpha )d^{\alpha (1-\alpha )}(\log (2n))^{-\alpha /2}$
with a lower bound of $c'(\alpha )d^{\alpha (1-\alpha )}(\log (2n))^{-\alpha /2}$![]() whenever $\alpha \in [1/2, 1)$
whenever $\alpha \in [1/2, 1)$![]() and $c'(\alpha )d^{\alpha /2}(\log (2n))^{-\alpha /2}$
and $c'(\alpha )d^{\alpha /2}(\log (2n))^{-\alpha /2}$![]() otherwise, where $p=1$
otherwise, where $p=1$![]() and $c(\alpha )$
and $c(\alpha )$![]() , $c'(\alpha )$
, $c'(\alpha )$![]() are universal constants, see [Reference Kalton12, Proposition 8.6].
are universal constants, see [Reference Kalton12, Proposition 8.6].
As an introduction to the proof of theorem 1, it will be instructive to better investigate a canonical, locally coordinatewise affine retraction from $(K, |\cdot |_1)$![]() , where $K=\cup _{Q\in \mathcal {R}} Q$
, where $K=\cup _{Q\in \mathcal {R}} Q$![]() is a union of a collection $\emptyset \neq \mathcal {R} \subseteq \{ Rw + R[0,\,1]^d: w\in \mathbb {Z}^d\}$
is a union of a collection $\emptyset \neq \mathcal {R} \subseteq \{ Rw + R[0,\,1]^d: w\in \mathbb {Z}^d\}$![]() of cubes in $\mathbb {R}^d$
of cubes in $\mathbb {R}^d$![]() with side length $R>0$
with side length $R>0$![]() , into the Lipschitz-free $p$
, into the Lipschitz-free $p$![]() -space $\mathcal {F}_p (V, |\cdot |_1)$
-space $\mathcal {F}_p (V, |\cdot |_1)$![]() over their vertices.
over their vertices.
From [Reference Lancien and Pernecká14] we know that for $p=1$![]() , the retraction is Lipschitz continuous with the Lipschitz constant equal to one. More recently, it was established in [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 5.1] that the retraction is Lipschitz continuous for any $0< p\leq 1$
, the retraction is Lipschitz continuous with the Lipschitz constant equal to one. More recently, it was established in [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 5.1] that the retraction is Lipschitz continuous for any $0< p\leq 1$![]() . However, the roles of $p$
. However, the roles of $p$![]() and $d$
and $d$![]() in the estimate of the Lipschitz constant were unclear, and the method led to a suboptimal estimate even for the classical $p=1$
in the estimate of the Lipschitz constant were unclear, and the method led to a suboptimal estimate even for the classical $p=1$![]() case.
case.
Here we present an alternative approach which generically refines the estimate from [Reference Albiac, Ansorena, Cúth and Doucha4], and we apply a double counting argument to derive a lower bound on the Lipschitz constant of the retraction. That is, we obtain the following result, which answers [Reference Albiac, Ansorena, Cúth and Doucha4, Question 4.6] in the negative.
Theorem 2 cf. Theorem 3.2
There is a unique map $r_{K, V}:(K, |\cdot |_1)\to \mathcal {F}_p (V, |\cdot |_1)$![]() such that $r_{K, V}(v) = \delta _V (v)$
such that $r_{K, V}(v) = \delta _V (v)$![]() , where $v\in V$
, where $v\in V$![]() , and $r_{K, V}$
, and $r_{K, V}$![]() is coordinatewise affine on each of the cubes in $\mathcal {R}$
is coordinatewise affine on each of the cubes in $\mathcal {R}$![]() . If we denote $C(p, n)= n^{1/p -1}$
. If we denote $C(p, n)= n^{1/p -1}$![]() , where $n\in \mathbb {N}$
, where $n\in \mathbb {N}$![]() , then
, then
The article is organized as follows. In $\S$![]() 2, we recall the notion of a $p$
2, we recall the notion of a $p$![]() -Banach space and include several foundational properties of Lipschitz-free $p$
-Banach space and include several foundational properties of Lipschitz-free $p$![]() -spaces. We also introduce the canonical, locally coordinatewise affine retraction in a cube. Section 3 is devoted to the proof of theorem 2. In $\S$
-spaces. We also introduce the canonical, locally coordinatewise affine retraction in a cube. Section 3 is devoted to the proof of theorem 2. In $\S$![]() 4, we develop a series of results leading up to the proof of theorem 1 for the particular case $\mathcal {M}=[0,\,1]^d$
4, we develop a series of results leading up to the proof of theorem 1 for the particular case $\mathcal {M}=[0,\,1]^d$![]() , and then we deduce the general conclusion for snowflakes of infinite doubling metric spaces.
, and then we deduce the general conclusion for snowflakes of infinite doubling metric spaces.
2. Preliminaries
2.1 $p$ -normed spaces
-normed spaces
Definition 2.1 Let $X$![]() be a vector space. We say that a map $\lVert \cdot \rVert : X\to [0, \infty )$
be a vector space. We say that a map $\lVert \cdot \rVert : X\to [0, \infty )$![]() is a quasi-norm on $X$
is a quasi-norm on $X$![]() if there exists $\kappa \geq 1$
if there exists $\kappa \geq 1$![]() such that
such that
(i) $\lVert x \rVert > 0$
 for any $x\neq 0$
for any $x\neq 0$ ,
,(ii) $\lVert \alpha x \rVert = |\alpha |\lVert x \rVert$
 for any scalar $\alpha$
for any scalar $\alpha$ and $x\in X$
and $x\in X$ ,
,(iii) $\lVert x+y\rVert \leq \kappa (\lVert x\rVert + \lVert y\rVert )$
 for any $x,\,y\in X$
for any $x,\,y\in X$ .
.
We then call $(X, \lVert \cdot \rVert )$![]() a quasi-normed space.
a quasi-normed space.
Replacing (iii) with the assumption that for some $0< p\leq 1$![]() ,
,
(iii’) $\lVert x+y\rVert ^p \leq \lVert x\rVert ^p + \lVert y\rVert ^p$
 for any $x,\,y\in X$
for any $x,\,y\in X$ ,
,
we obtain the notion of a $p$![]() -norm and a $p$
-norm and a $p$![]() -normed space. Moreover, if $X$
-normed space. Moreover, if $X$![]() is complete with respect to the metric $d(x,\,y)=\lVert x-y\rVert ^p$
is complete with respect to the metric $d(x,\,y)=\lVert x-y\rVert ^p$![]() ($x,\,y\in X$
($x,\,y\in X$![]() ), we say $(X, \lVert \cdot \rVert )$
), we say $(X, \lVert \cdot \rVert )$![]() is a $p$
is a $p$![]() -Banach space.
-Banach space.
Definition 2.2 For $0< p\leq 1$![]() , we say that a subset $Z$
, we say that a subset $Z$![]() of a vector space $X$
of a vector space $X$![]() is absolutely $p$
is absolutely $p$![]() -convex if for any $x, y\in Z$
-convex if for any $x, y\in Z$![]() and scalars $\alpha,\,\beta$
and scalars $\alpha,\,\beta$![]() , where $|\alpha |^p+|\beta |^p\leq 1$
, where $|\alpha |^p+|\beta |^p\leq 1$![]() , we have $\alpha x + \beta y\in Z$
, we have $\alpha x + \beta y\in Z$![]() .
.
The smallest absolutely $p$![]() -convex set containing $Z$
-convex set containing $Z$![]() is denoted by $\operatorname {aconv}_p Z$
is denoted by $\operatorname {aconv}_p Z$![]() .
.
We shall write $B_{X} = \{ x\in X : \lVert x \rVert \leq 1\}$![]() for the unit ball of a quasi-normed space $(X, \lVert \cdot \rVert )$
for the unit ball of a quasi-normed space $(X, \lVert \cdot \rVert )$![]() .
.
Definition 2.3 For $0< p\leq 1$![]() , we say that a subset $Z$
, we say that a subset $Z$![]() of a quasi-normed space $X$
of a quasi-normed space $X$![]() is $p$
is $p$![]() -norming with constants $\alpha, \,\beta > 0$
-norming with constants $\alpha, \,\beta > 0$![]() whenever
whenever
If $\alpha =\beta =1$![]() , we say $Z$
, we say $Z$![]() is isometrically $p$
is isometrically $p$![]() -norming.
-norming.
If $X_1$![]() and $X_2$
and $X_2$![]() are two quasi-normed spaces, we let $B(X_1, X_2)$
are two quasi-normed spaces, we let $B(X_1, X_2)$![]() denote the space of bounded linear operators from $X_1$
denote the space of bounded linear operators from $X_1$![]() into $X_2$
into $X_2$![]() .
.
The following fact is an easy linear variant of an extension theorem for Lipschitz continuous maps.
Lemma 2.4 Let $0< p\leq 1$![]() . Assume that $Y_1$
. Assume that $Y_1$![]() and $Y_2$
and $Y_2$![]() are $p$
are $p$![]() -norming in $p$
-norming in $p$![]() -Banach spaces $X_1$
-Banach spaces $X_1$![]() and $X_2$
and $X_2$![]() , respectively, and that $\operatorname {aconv}_p Y_1$
, respectively, and that $\operatorname {aconv}_p Y_1$![]() and $\operatorname {aconv}_p Y_2$
and $\operatorname {aconv}_p Y_2$![]() contain neighbourhoods of zero in $\operatorname {span} Y_1$
contain neighbourhoods of zero in $\operatorname {span} Y_1$![]() and $\operatorname {span} Y_2$
and $\operatorname {span} Y_2$![]() , respectively. That is, we have $\operatorname {aconv}_p Y_i \supseteq c_i B_{X_i} \cap \operatorname {span} Y_i$
, respectively. That is, we have $\operatorname {aconv}_p Y_i \supseteq c_i B_{X_i} \cap \operatorname {span} Y_i$![]() for some $c_i > 0$
for some $c_i > 0$![]() , for each $i\in \{1, 2\}$
, for each $i\in \{1, 2\}$![]() .
.
If $T$![]() is a one-to-one linear map from $\operatorname {span} Y_1$
is a one-to-one linear map from $\operatorname {span} Y_1$![]() into $X_2$
into $X_2$![]() such that $T(Y_1)=Y_2$
such that $T(Y_1)=Y_2$![]() , then $T$
, then $T$![]() extends to an onto isomorphism $\widetilde T : X_1 \to X_2$
extends to an onto isomorphism $\widetilde T : X_1 \to X_2$![]() .
.
Quantitatively, if $Y_1$![]() , $Y_2$
, $Y_2$![]() are $p$
are $p$![]() -norming in $X_1$
-norming in $X_1$![]() , $X_2$
, $X_2$![]() with constants $\alpha, \,\beta$
with constants $\alpha, \,\beta$![]() and $\alpha ', \,\beta '$
and $\alpha ', \,\beta '$![]() , respectively, then $\lVert \widetilde T \rVert \leq \beta /\alpha '$
, respectively, then $\lVert \widetilde T \rVert \leq \beta /\alpha '$![]() and $\lVert {\widetilde T}^{-1} \rVert \leq \beta '/\alpha$
and $\lVert {\widetilde T}^{-1} \rVert \leq \beta '/\alpha$![]() .
.
We remark that [Reference Albiac, Ansorena, Cúth and Doucha2, Lemma 2.6] states a stronger, alleged variant of the extension result, leaving out the assumption that $\operatorname {aconv}_p Y_i$![]() contains a neighbourhood of zero in $\operatorname {span} Y_i$
contains a neighbourhood of zero in $\operatorname {span} Y_i$![]() , for each $i\in \{1,\,2\}$
, for each $i\in \{1,\,2\}$![]() . This claim, however, is not true. Nevertheless, it turns out that the assumptions of lemma 2.4 are satisfied in the applications of [Reference Albiac, Ansorena, Cúth and Doucha2, Lemma 2.6] in [Reference Albiac, Ansorena, Cúth and Doucha2]; hence, the derived results remain valid.
. This claim, however, is not true. Nevertheless, it turns out that the assumptions of lemma 2.4 are satisfied in the applications of [Reference Albiac, Ansorena, Cúth and Doucha2, Lemma 2.6] in [Reference Albiac, Ansorena, Cúth and Doucha2]; hence, the derived results remain valid.
The following counterexample to [Reference Albiac, Ansorena, Cúth and Doucha2, Lemma 2.6] was suggested by Ansorena.
Counter example 2.5 Let $X$![]() be a $p$
be a $p$![]() -Banach space, where $0< p\leq 1$
-Banach space, where $0< p\leq 1$![]() , and let $M$
, and let $M$![]() be a closed subspace of $X$
be a closed subspace of $X$![]() . Let $T:X\to X/M$
. Let $T:X\to X/M$![]() be the quotient map.
be the quotient map.
Pick a dense subspace $V$![]() of $X$
of $X$![]() such that $V\cap M = \{ 0 \}$
such that $V\cap M = \{ 0 \}$![]() , and set $K = B_X \cap V$
, and set $K = B_X \cap V$![]() . It is easy to see that $K$
. It is easy to see that $K$![]() is absolutely $p$
is absolutely $p$![]() -convex as well as isometrically $p$
-convex as well as isometrically $p$![]() -norming in $X$
-norming in $X$![]() . If $U_X$
. If $U_X$![]() denotes the open unit neighbourhood of zero in $X$
denotes the open unit neighbourhood of zero in $X$![]() , we verify that
, we verify that
For instance, for any $0< p\leq 1$![]() we may take $X=\ell _p$
we may take $X=\ell _p$![]() , $M=\operatorname {span} \{ e_1 \}$
, $M=\operatorname {span} \{ e_1 \}$![]() , and $V = \operatorname {span} \left \{\{ e_n: n\geq 2\} \cup \{e_1 + \sum _{n=2}^\infty 2^{-n} e_n \}\right \}$
, and $V = \operatorname {span} \left \{\{ e_n: n\geq 2\} \cup \{e_1 + \sum _{n=2}^\infty 2^{-n} e_n \}\right \}$![]() .
.
If we denote $X_1=X$![]() , $X_2=X/M$
, $X_2=X/M$![]() and $Y_1 = K$
and $Y_1 = K$![]() , $Y_2 = T(K)$
, $Y_2 = T(K)$![]() , then $Y_1$
, then $Y_1$![]() and $Y_2$
and $Y_2$![]() are absolutely $p$
are absolutely $p$![]() -norming in $X_1$
-norming in $X_1$![]() and $X_2$
and $X_2$![]() , respectively, and $T$
, respectively, and $T$![]() is a linear bijection from $\operatorname {span} Y_1$
is a linear bijection from $\operatorname {span} Y_1$![]() onto $\operatorname {span} Y_2$
onto $\operatorname {span} Y_2$![]() . However, $T$
. However, $T$![]() does not extend to an isomorphism from $X_1$
does not extend to an isomorphism from $X_1$![]() into $X_2$
into $X_2$![]() .
.
A particular class of coefficients
We introduce a coefficient $C(p, n)$![]() which has the role of $\kappa$
which has the role of $\kappa$![]() in (iii) for sums of $n$
in (iii) for sums of $n$![]() elements, i.e. if $(X, \lVert \cdot \rVert )$
elements, i.e. if $(X, \lVert \cdot \rVert )$![]() is a quasi-normed space and both $0< p\leq 1$
is a quasi-normed space and both $0< p\leq 1$![]() and $n\in \mathbb {N}$
and $n\in \mathbb {N}$![]() are given, then $\lVert \sum _{i=1}^n x_i\rVert \leq C(p, n)\sum _{i=1}^n \lVert x_i\rVert$
are given, then $\lVert \sum _{i=1}^n x_i\rVert \leq C(p, n)\sum _{i=1}^n \lVert x_i\rVert$![]() for any $x_1, \ldots, x_n \in X$
for any $x_1, \ldots, x_n \in X$![]() .
.
Definition 2.6 For any $n \in \mathbb {N}$![]() and $0< p \leq 1$
and $0< p \leq 1$![]() , let us denote
, let us denote

Note that $(\sum _{i=1}^n |w_i|^p)^{1/p} \leq C(p, n)|w|_1$![]() for any $n\in \mathbb {N}$
for any $n\in \mathbb {N}$![]() , $0< p \leq 1$
, $0< p \leq 1$![]() , and $w=(w_i)_{i=1}^n \in \mathbb {R}^n$
, and $w=(w_i)_{i=1}^n \in \mathbb {R}^n$![]() .
.
An explicit formula for $C(p, n)$![]() follows easily from Hölder's inequality.
follows easily from Hölder's inequality.
Fact 2.7 Let $n \in \mathbb {N}$![]() , $0< p \leq 1$
, $0< p \leq 1$![]() . It holds that $C(p, n) = n^{1/p -1}$
. It holds that $C(p, n) = n^{1/p -1}$![]() .
.
2.2 Lipschitz-free $p$ -spaces
-spaces
If $(\mathcal {M}, \rho )$![]() is a pointed metric space with $0_{\mathcal {M}}$
is a pointed metric space with $0_{\mathcal {M}}$![]() as its base point, we consider $\delta : \mathcal {M} \to \operatorname {Lip}_0 (\mathcal {M})^*$
as its base point, we consider $\delta : \mathcal {M} \to \operatorname {Lip}_0 (\mathcal {M})^*$![]() that maps $x\in \mathcal {M}$
that maps $x\in \mathcal {M}$![]() to the canonical evaluation functional $\delta (x)\in \operatorname {Lip}_0 (\mathcal {M})^*$
to the canonical evaluation functional $\delta (x)\in \operatorname {Lip}_0 (\mathcal {M})^*$![]() , i.e. $\langle \delta (x), f \rangle = f(x)$
, i.e. $\langle \delta (x), f \rangle = f(x)$![]() for each $f \in \operatorname {Lip}_0 (\mathcal {M})$
for each $f \in \operatorname {Lip}_0 (\mathcal {M})$![]() .
.
We recall that the Lipschitz-free space $\mathcal {F} (\mathcal {M})$![]() over $\mathcal {M}$
over $\mathcal {M}$![]() can be identified as the closed span of $\delta (\mathcal {M})$
can be identified as the closed span of $\delta (\mathcal {M})$![]() in $\operatorname {Lip}_0 (\mathcal {M})^*$
in $\operatorname {Lip}_0 (\mathcal {M})^*$![]() ,
,
Furthermore, $\mathcal {F}(\mathcal {M})^*$![]() is linearly isometric to $\operatorname {Lip}_0 (\mathcal {M})$
is linearly isometric to $\operatorname {Lip}_0 (\mathcal {M})$![]() . In fact, if $\operatorname {Mol} (\mathcal {M})$
. In fact, if $\operatorname {Mol} (\mathcal {M})$![]() denotes the set of elementary molecules in $\mathcal {F}(\mathcal {M})$
denotes the set of elementary molecules in $\mathcal {F}(\mathcal {M})$![]() ,
,
it follows from the Hahn–Banach theorem that $B_{\mathcal {F}(\mathcal {M})} = \overline{\operatorname {conv}}\operatorname {Mol} (\mathcal {M})$![]() .
.
Let $0< p\leq 1$![]() and denote $\mathcal {P} (\mathcal {M}) = \operatorname {span} \{ \delta (x) : x\in \mathcal {M}\}$
and denote $\mathcal {P} (\mathcal {M}) = \operatorname {span} \{ \delta (x) : x\in \mathcal {M}\}$![]() . Drawing from the outlined construction, we set for each $m\in \mathcal {P}(\mathcal {M})$
. Drawing from the outlined construction, we set for each $m\in \mathcal {P}(\mathcal {M})$![]()

the infimum being taken over all $n\in \mathbb {N}_0$![]() and $\mu _i\in \operatorname {Mol} (\mathcal {M})$
and $\mu _i\in \operatorname {Mol} (\mathcal {M})$![]() , $a_i\in \mathbb {R}$
, $a_i\in \mathbb {R}$![]() , for each $i\in \{1, \ldots, n\}$
, for each $i\in \{1, \ldots, n\}$![]() , such that $m = \sum _{i=1}^n a_i\mu _i$
, such that $m = \sum _{i=1}^n a_i\mu _i$![]() .
.
It turns out that $(\mathcal {P}(\mathcal {M}), \lVert \cdot \rVert )$![]() is a $p$
is a $p$![]() -normed space and $\delta$
-normed space and $\delta$![]() is an isometric embedding. The completion yields the Lipschitz-free $p$
is an isometric embedding. The completion yields the Lipschitz-free $p$![]() -space $\mathcal {F}_p(\mathcal {M})$
-space $\mathcal {F}_p(\mathcal {M})$![]() .
.
Theorem 2.8 cf. [Reference Albiac, Ansorena, Cúth and Doucha2, Theorem 4.5]
Let $(\mathcal {M}, \rho )$![]() be a pointed metric space. Given $0< p\leq 1$
be a pointed metric space. Given $0< p\leq 1$![]() , there exists a $p$
, there exists a $p$![]() -Banach space $(\mathcal {F}_p (\mathcal {M}), \lVert \cdot \rVert )$
-Banach space $(\mathcal {F}_p (\mathcal {M}), \lVert \cdot \rVert )$![]() , called the Lipschitz-free $p$
, called the Lipschitz-free $p$![]() -space over $\mathcal {M}$
-space over $\mathcal {M}$![]() , and a map $\delta : \mathcal {M} \to \mathcal {F}_p (\mathcal {M})$
, and a map $\delta : \mathcal {M} \to \mathcal {F}_p (\mathcal {M})$![]() such that
such that
(i) $\delta$
 is an isometric embedding with $\delta (0_{\mathcal {M}})=0_{\mathcal {F}_p(\mathcal {M})}$
is an isometric embedding with $\delta (0_{\mathcal {M}})=0_{\mathcal {F}_p(\mathcal {M})}$ ,
,(ii) $\mathcal {F}_p (\mathcal {M}) = \overline {\operatorname {span}} \{ \delta (x) : x\in \mathcal {M} \}$
 ,
,(iii) if $(Y, \lVert \cdot \rVert _Y)$
 is a $p$
is a $p$ -Banach space, then $B(\mathcal {F}_p(\mathcal {M}), Y)$
-Banach space, then $B(\mathcal {F}_p(\mathcal {M}), Y)$ is linearly isometric to $\operatorname {Lip}_0 (\mathcal {M}, Y)$
is linearly isometric to $\operatorname {Lip}_0 (\mathcal {M}, Y)$ via the map $f^* \mapsto f^* \circ \delta$
via the map $f^* \mapsto f^* \circ \delta$ for each $f^*\in B(\mathcal {F}_p(\mathcal {M}), Y)$
for each $f^*\in B(\mathcal {F}_p(\mathcal {M}), Y)$ .
.
Fact 2.9 cf. [Reference Albiac, Ansorena, Cúth and Doucha2, Corollary 4.11]
Let $(\mathcal {M}, \rho )$![]() be a pointed metric space. For each $0< p\leq 1$
be a pointed metric space. For each $0< p\leq 1$![]() , the set $\operatorname {Mol} (\mathcal {M})$
, the set $\operatorname {Mol} (\mathcal {M})$![]() is isometrically $p$
is isometrically $p$![]() -norming in $\mathcal {F}_p (\mathcal {M})$
-norming in $\mathcal {F}_p (\mathcal {M})$![]() . That is, $B_{\mathcal {F}_p (\mathcal {M})} = \overline{\operatorname {aconv}}_p\operatorname {Mol} (\mathcal {M})$
. That is, $B_{\mathcal {F}_p (\mathcal {M})} = \overline{\operatorname {aconv}}_p\operatorname {Mol} (\mathcal {M})$![]() , and for each $m\in \mathcal {P}(\mathcal {M})$
, and for each $m\in \mathcal {P}(\mathcal {M})$![]() we have
we have

the infimum being taken over all $n\in \mathbb {N}_0$![]() and $\mu _i\in \operatorname {Mol} (\mathcal {M})$
and $\mu _i\in \operatorname {Mol} (\mathcal {M})$![]() , $a_i\in \mathbb {R}$
, $a_i\in \mathbb {R}$![]() , where $i\in \{1, \ldots, n\}$
, where $i\in \{1, \ldots, n\}$![]() , such that $m = \sum _{i=1}^n a_i\mu _i$
, such that $m = \sum _{i=1}^n a_i\mu _i$![]() .
.
We show that for any dense subset $\mathcal {N}$![]() of $\mathcal {M}$
of $\mathcal {M}$![]() and any $m\in \mathcal {P} (\mathcal {N})$
and any $m\in \mathcal {P} (\mathcal {N})$![]() , the above formula is still valid if we consider decompositions of $m$
, the above formula is still valid if we consider decompositions of $m$![]() merely into molecules over $\mathcal {N}$
merely into molecules over $\mathcal {N}$![]() .
.
Lemma 2.10 Let $\mathcal {N}$![]() be a dense subset of a pointed metric space $(\mathcal {M}, \rho )$
be a dense subset of a pointed metric space $(\mathcal {M}, \rho )$![]() . Then for each $0< p\leq 1$
. Then for each $0< p\leq 1$![]() and $m\in \mathcal {P}(\mathcal {N})$
and $m\in \mathcal {P}(\mathcal {N})$![]() , we have $\left \Vert m \right \Vert _{\mathcal {F}_p (\mathcal {M})} = \inf ( \sum _{i=1}^n |a_i|^p )^{1/p}$
, we have $\left \Vert m \right \Vert _{\mathcal {F}_p (\mathcal {M})} = \inf ( \sum _{i=1}^n |a_i|^p )^{1/p}$![]() , the infimum being taken over all $n\in \mathbb {N}_0$
, the infimum being taken over all $n\in \mathbb {N}_0$![]() and $\mu _i\in \operatorname {Mol} (\mathcal {N})$
and $\mu _i\in \operatorname {Mol} (\mathcal {N})$![]() , $a_i\in \mathbb {R}$
, $a_i\in \mathbb {R}$![]() , where $i\in \{1, \ldots, n\}$
, where $i\in \{1, \ldots, n\}$![]() , such that $m = \sum _{i=1}^n a_i\mu _i$
, such that $m = \sum _{i=1}^n a_i\mu _i$![]() .
.
In particular, $\operatorname {aconv}_p \operatorname {Mol} (\mathcal {N})$![]() contains the open unit neighbourhood of zero in $\mathcal {P} (\mathcal {N})$
contains the open unit neighbourhood of zero in $\mathcal {P} (\mathcal {N})$![]() , with respect to the ambient space $\mathcal {F}_p (\mathcal {M})$
, with respect to the ambient space $\mathcal {F}_p (\mathcal {M})$![]() . That is, we have $\{ m \in \mathcal {P} (\mathcal {N}): \lVert m \rVert _{\mathcal {F}_p (\mathcal {M})} < 1 \} \subseteq \operatorname {aconv}_p \operatorname {Mol} (\mathcal {N})$
. That is, we have $\{ m \in \mathcal {P} (\mathcal {N}): \lVert m \rVert _{\mathcal {F}_p (\mathcal {M})} < 1 \} \subseteq \operatorname {aconv}_p \operatorname {Mol} (\mathcal {N})$![]() .
.
Proof. Let $m\in \mathcal {P} (\mathcal {N})$![]() and pick $\epsilon > 0$
and pick $\epsilon > 0$![]() . It follows from fact 2.9 that there exist $n\in \mathbb {N}_0$
. It follows from fact 2.9 that there exist $n\in \mathbb {N}_0$![]() and $\mu _i\in \operatorname {Mol} (\mathcal {M})$
and $\mu _i\in \operatorname {Mol} (\mathcal {M})$![]() , $a_i\in \mathbb {R}$
, $a_i\in \mathbb {R}$![]() , where $i\in \{1, \ldots, n\}$
, where $i\in \{1, \ldots, n\}$![]() , for which $m = \sum _{i=1}^n a_i\mu _i$
, for which $m = \sum _{i=1}^n a_i\mu _i$![]() , and $( \sum _{i=1}^n |a_i|^p )^{1/p} < (1+\epsilon )\lVert m \rVert _{\mathcal {F}_p (\mathcal {M})}$
, and $( \sum _{i=1}^n |a_i|^p )^{1/p} < (1+\epsilon )\lVert m \rVert _{\mathcal {F}_p (\mathcal {M})}$![]() . We further consider $u_i,\,v_i \in \mathcal {M}$
. We further consider $u_i,\,v_i \in \mathcal {M}$![]() , $u_i\neq v_i$
, $u_i\neq v_i$![]() , such that $\mu _i = {\delta (u_i)-\delta (v_i)}/{|u_i-v_i|^\alpha }$
, such that $\mu _i = {\delta (u_i)-\delta (v_i)}/{|u_i-v_i|^\alpha }$![]() , where $i\in \{1, \ldots, n\}$
, where $i\in \{1, \ldots, n\}$![]() .
.
Let us denote $\mathcal {B} = \{u_i\}_{i\in \{1, \ldots, n\}} \cup \{ v_i\}_{i\in \{1, \ldots, n\}}$![]() . By density of $\mathcal {N}$
. By density of $\mathcal {N}$![]() in $\mathcal {M}$
in $\mathcal {M}$![]() , it is easy to construct a mapping $r: \mathcal {B} \mapsto \mathcal {N}$
, it is easy to construct a mapping $r: \mathcal {B} \mapsto \mathcal {N}$![]() such that $r(b)=b$
such that $r(b)=b$![]() for any $b\in \mathcal {N}$
for any $b\in \mathcal {N}$![]() , and ${|r(u_i)-r(v_i)|^{\alpha }}/{|u_i-v_i|^{\alpha }}<1+\epsilon$
, and ${|r(u_i)-r(v_i)|^{\alpha }}/{|u_i-v_i|^{\alpha }}<1+\epsilon$![]() but $r(u_i)\neq r(v_i)$
but $r(u_i)\neq r(v_i)$![]() , for any $i\in \{1,\,\ldots, n\}$
, for any $i\in \{1,\,\ldots, n\}$![]() .
.
We consider the unique linear mapping $r':\mathcal {P} (\mathcal {B}) \mapsto \mathcal {P} (\mathcal {N})$![]() which satisfies that $r'(\delta (b)) = \delta (r(b))$
which satisfies that $r'(\delta (b)) = \delta (r(b))$![]() for each $b\in \mathcal {B}$
for each $b\in \mathcal {B}$![]() . It is easy to see that $r'(m) = m$
. It is easy to see that $r'(m) = m$![]() , as $r'$
, as $r'$![]() agrees with the identity on $\mathcal {P} (\mathcal {N})$
agrees with the identity on $\mathcal {P} (\mathcal {N})$![]() .
.
Let us rewrite $m=r'(m)=\sum _{i=1}^n a_i r'(\mu _i)$![]() , where
, where

For each $i\in \{ 1, \ldots, n\}$![]() , we denote $\mu '_i = {\delta (r(u_i))-\delta (r(v_i))}/{|r(u_i)-(v_i)|^\alpha } \in \operatorname {Mol} (\mathcal {N})$
, we denote $\mu '_i = {\delta (r(u_i))-\delta (r(v_i))}/{|r(u_i)-(v_i)|^\alpha } \in \operatorname {Mol} (\mathcal {N})$![]() and set $a'_i = a_i {|r(u_i)-r(v_i)|^\alpha }/{|u_i-v_i|^\alpha }$
and set $a'_i = a_i {|r(u_i)-r(v_i)|^\alpha }/{|u_i-v_i|^\alpha }$![]() . It follows from the construction that $m = \sum _{i=1}^n a'_i\mu '_i$
. It follows from the construction that $m = \sum _{i=1}^n a'_i\mu '_i$![]() and $( \sum _{i=1}^n |a'_i|^p )^{1/p} < (1+\epsilon )( \sum _{i=1}^n |a_i|^p )^{1/p}<(1+\epsilon )^2\lVert m \rVert _{\mathcal {F}_p (\mathcal {M})}$
and $( \sum _{i=1}^n |a'_i|^p )^{1/p} < (1+\epsilon )( \sum _{i=1}^n |a_i|^p )^{1/p}<(1+\epsilon )^2\lVert m \rVert _{\mathcal {F}_p (\mathcal {M})}$![]() . We take $\epsilon \to 0$
. We take $\epsilon \to 0$![]() , and the first claim follows.
, and the first claim follows.
Note that, in particular, the already proven part shows that $\{ m \in \mathcal {P} (\mathcal {N}): \lVert m \rVert _{\mathcal {F}_p (\mathcal {M})} < 1 \} \subseteq \operatorname {aconv}_p \operatorname {Mol} (\mathcal {N})$![]() . This establishes the second claim.
. This establishes the second claim.
We note that any Lipschitz map between pointed metric spaces which vanishes at the base point has an extension to a bounded operator between the respective Lipschitz-free $p$![]() -spaces, for every $0< p\leq 1$
-spaces, for every $0< p\leq 1$![]() .
.
Fact 2.11 cf. [Reference Albiac, Ansorena, Cúth and Doucha2, Lemma 4.8]
Let $\mathcal {M}$![]() , $\mathcal {N}$
, $\mathcal {N}$![]() be pointed metric spaces. For every $0< p\leq 1$
be pointed metric spaces. For every $0< p\leq 1$![]() , there is a linear isometry $L: \operatorname {Lip}_0 (\mathcal {M}, \mathcal {N}) \to B (\mathcal {F}_p(\mathcal {M}), \mathcal {F}_p(\mathcal {N}))$
, there is a linear isometry $L: \operatorname {Lip}_0 (\mathcal {M}, \mathcal {N}) \to B (\mathcal {F}_p(\mathcal {M}), \mathcal {F}_p(\mathcal {N}))$![]() , called the canonical linearization operator, such that $\delta _{\mathcal {N}} \circ f = L(f) \circ \delta _{\mathcal {M}}$
, called the canonical linearization operator, such that $\delta _{\mathcal {N}} \circ f = L(f) \circ \delta _{\mathcal {M}}$![]() , for every $f\in \operatorname {Lip}_0 (\mathcal {M}, \mathcal {N})$
, for every $f\in \operatorname {Lip}_0 (\mathcal {M}, \mathcal {N})$![]() .
.
2.3 A projective construction in $[0, 1]^d$
We overview a canonical, locally coordinatewise affine projective construction in $[0, 1]^d$![]() ; hereby we set ground for basis expansions in $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )$
; hereby we set ground for basis expansions in $\mathcal {F}_p ([0,\,1]^d, |\cdot |^\alpha )$![]() considered within § 4. Let us remark that this particular choice has appeared in various contexts, e.g. [Reference Albiac, Ansorena, Cúth and Doucha4, Reference Lancien and Pernecká14, Reference Weaver18]. We adopt the notation from [Reference Albiac, Ansorena, Cúth and Doucha4, Section 3].
considered within § 4. Let us remark that this particular choice has appeared in various contexts, e.g. [Reference Albiac, Ansorena, Cúth and Doucha4, Reference Lancien and Pernecká14, Reference Weaver18]. We adopt the notation from [Reference Albiac, Ansorena, Cúth and Doucha4, Section 3].
Let $d\in \mathbb {N}$![]() , $R>0$
, $R>0$![]() . If $w=(w_i)_{i=1}^d \in \mathbb {Z}^d$
. If $w=(w_i)_{i=1}^d \in \mathbb {Z}^d$![]() , we set $V^d_{w, R} = Rw+R\{0, 1\}^d$
, we set $V^d_{w, R} = Rw+R\{0, 1\}^d$![]() and define a cube $Q^d_{w, R}$
and define a cube $Q^d_{w, R}$![]() as the convex hull of the set $V^d_{w, R}$
as the convex hull of the set $V^d_{w, R}$![]() , i.e. $Q^d_{w, R} = Rw+R[0, 1]^d$
, i.e. $Q^d_{w, R} = Rw+R[0, 1]^d$![]() . We denote $\mathcal {Q}^d_{R} = \left \{Q^d_{w, R}: w\in \mathbb {Z}^d \right \}$
. We denote $\mathcal {Q}^d_{R} = \left \{Q^d_{w, R}: w\in \mathbb {Z}^d \right \}$![]() and $\mathcal {V}^d_{R} = \left \{V^d_{w, R}: w\in \mathbb {Z}^d \right \}$
and $\mathcal {V}^d_{R} = \left \{V^d_{w, R}: w\in \mathbb {Z}^d \right \}$![]() . Let us further introduce a map $\mathcal {V} : \mathcal {Q}^d_{R} \to \mathcal {V}^d_{R}$
. Let us further introduce a map $\mathcal {V} : \mathcal {Q}^d_{R} \to \mathcal {V}^d_{R}$![]() by $\mathcal {V}(Q^d_{w, R})= V^d_{w, R}$
by $\mathcal {V}(Q^d_{w, R})= V^d_{w, R}$![]() , where $w\in \mathbb {Z}^d$
, where $w\in \mathbb {Z}^d$![]() .
.
We define projective coefficients from $\mathbb {R}^d$![]() to the vertex set $\mathcal {V}^d_{R}$
to the vertex set $\mathcal {V}^d_{R}$![]() . For any $x\in [0, 1]$
. For any $x\in [0, 1]$![]() and $w\in \mathbb {Z}$
and $w\in \mathbb {Z}$![]() , we first put
, we first put

and write, whenever $x=(x_i)_{i=1}^d \in [0, 1]^d$![]() and $w=(w_i)_{i=1}^d \in \mathbb {Z}^d$
and $w=(w_i)_{i=1}^d \in \mathbb {Z}^d$![]() ,
,

We find that this construction admits a lift to $\mathbb {R}^d$![]() in $x$
in $x$![]() .
.
Lemma 2.12 cf. [Reference Albiac, Ansorena, Cúth and Doucha4, Lemma 3.1]
Let $d\in \mathbb {N}$![]() , $R>0$
, $R>0$![]() . There exists a map
. There exists a map
such that $\Lambda _R^d(Ru, Rw+Rx)=x^{(u-w)}$![]() , for every $x\in [0, 1]^d$
, for every $x\in [0, 1]^d$![]() and $u,\,w\in \mathbb {Z}^d$
and $u,\,w\in \mathbb {Z}^d$![]() .
.
Moreover, we list properties of $\Lambda ^d_R$![]() which we shall refer to in the sequel.
which we shall refer to in the sequel.
Lemma 2.13 cf. [Reference Albiac, Ansorena, Cúth and Doucha4, Lemma 3.4]
Let $d\in \mathbb {N}$![]() , $R>0$
, $R>0$![]() . It holds that
. It holds that
(i) $\Lambda ^d_R (v, x) =0$
 whenever $x\in Q \in \mathcal {Q}^d_{R}$
whenever $x\in Q \in \mathcal {Q}^d_{R}$ and $v\notin \mathcal {V}(Q)$
and $v\notin \mathcal {V}(Q)$ ,
,(ii) $\Lambda ^d_R (v, u) = \delta _{v, u}$
 for any $u,\,v\in \mathcal {V}^d_R$
for any $u,\,v\in \mathcal {V}^d_R$ ,
,(iii) $\sum _{v\in \mathcal {V}^d_R} \Lambda ^d_R (v, x)=1$
 for any $x\in \mathbb {R}^d$
for any $x\in \mathbb {R}^d$ ,
,(iv) $\Lambda ^d_R (v, x) = \prod _{i=1}^d \Lambda ^1_R (v_i, x_i)$
 for any $x=(x_i)_{i=1}^d\in \mathbb {R}^d$
for any $x=(x_i)_{i=1}^d\in \mathbb {R}^d$ , $v=(v_i)_{i=1}^d\in \mathcal {V}^d_R$
, $v=(v_i)_{i=1}^d\in \mathcal {V}^d_R$ .
.
3. A retraction in $\mathcal {F}_p (\mathbb [0, 1]^d, |\cdot |_1)$
Drawing from the projective construction introduced in § 2.3, we consider a retraction from $(K, |\cdot |_1)$![]() , where $K = \cup _{Q\in \mathcal {R}} Q$
, where $K = \cup _{Q\in \mathcal {R}} Q$![]() is a union of a collection $\mathcal {R}$
is a union of a collection $\mathcal {R}$![]() of regularly spaced cubes in $\mathbb {R}^d$
of regularly spaced cubes in $\mathbb {R}^d$![]() with equal side length, into the Lipschitz-free $p$
with equal side length, into the Lipschitz-free $p$![]() -space $\mathcal {F}_p (V, |\cdot |_1)$
-space $\mathcal {F}_p (V, |\cdot |_1)$![]() over their vertices. We provide bounds on the Lipschitz constant thereof and analyse locally coordinatewise affine extensions of Lipschitz maps from a vertex set ranging into $p$
over their vertices. We provide bounds on the Lipschitz constant thereof and analyse locally coordinatewise affine extensions of Lipschitz maps from a vertex set ranging into $p$![]() -Banach spaces.
-Banach spaces.
We pick $d\in \mathbb {N}$![]() and endow $\mathbb {R}^d$
and endow $\mathbb {R}^d$![]() with the $\ell _1$
with the $\ell _1$![]() -norm hereinafter.
-norm hereinafter.
Adopting the notation of § 2.3, let $\mathcal {R} \subseteq \mathcal {Q}^d_R$![]() , $K = \cup _{Q\in \mathcal {R}} Q$
, $K = \cup _{Q\in \mathcal {R}} Q$![]() , $V = \cup _{Q\in \mathcal {Q}} \mathcal {V}(Q)$
, $V = \cup _{Q\in \mathcal {Q}} \mathcal {V}(Q)$![]() , and fix a point of $V$
, and fix a point of $V$![]() as the base point of both $K$
as the base point of both $K$![]() , $V$
, $V$![]() . As a consequence of lemma 2.13 (i), for any $x\in K$
. As a consequence of lemma 2.13 (i), for any $x\in K$![]() the coefficients $\Lambda ^d_R (\cdot, x)$
the coefficients $\Lambda ^d_R (\cdot, x)$![]() are finitely supported, and hence we are justified to introduce $r=r_{K, V}:K\to \mathcal {F}_p(V)$
are finitely supported, and hence we are justified to introduce $r=r_{K, V}:K\to \mathcal {F}_p(V)$![]() as
as
We can easily see that $r_{K,V}=\delta _V$![]() on $V$
on $V$![]() . Moreover, in [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.5], it was established that the map $r_{K, V}$
. Moreover, in [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.5], it was established that the map $r_{K, V}$![]() is Lipschitz with an upper bound depending on both $p$
is Lipschitz with an upper bound depending on both $p$![]() and $d$
and $d$![]() , under the assumption of the $\ell _\infty$
, under the assumption of the $\ell _\infty$![]() -norm on $\mathbb {R}^d$
-norm on $\mathbb {R}^d$![]() . Applying their method to the $p=1$
. Applying their method to the $p=1$![]() and $\ell _1$
and $\ell _1$![]() -norm case, the obtained estimate still retained a term depending on $d$
-norm case, the obtained estimate still retained a term depending on $d$![]() , thus contrasting a positive result due to [Reference Lancien and Pernecká14] which shows the Lipschitz constant to equal 1.
, thus contrasting a positive result due to [Reference Lancien and Pernecká14] which shows the Lipschitz constant to equal 1.
Question 3.1 [Reference Albiac, Ansorena, Cúth and Doucha4, Question 4.6]
Is there a constant $C$![]() depending on $p$
depending on $p$![]() but not on $d$
but not on $d$![]() , $K$
, $K$![]() or $V$
or $V$![]() , such that $\operatorname {Lip}(r_{K, V})\leq C$
, such that $\operatorname {Lip}(r_{K, V})\leq C$![]() ?
?
We refine the estimate of [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.5] but answer the above question in negative.
Theorem 3.2 Let $d\in \mathbb {N}$![]() , $R>0$
, $R>0$![]() , $\mathcal {R} \subseteq \mathcal {Q}^d_R$
, $\mathcal {R} \subseteq \mathcal {Q}^d_R$![]() , where $\mathcal {R} \neq \emptyset$
, where $\mathcal {R} \neq \emptyset$![]() , $K = \cup _{Q\in \mathcal {R}} Q$
, $K = \cup _{Q\in \mathcal {R}} Q$![]() , $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$
, $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$![]() , and consider $V$
, and consider $V$![]() as a pointed metric space with the subspace $\ell _1$
as a pointed metric space with the subspace $\ell _1$![]() metric. We let $0< p\leq 1$
metric. We let $0< p\leq 1$![]() and $r=r_{K, V}:K\to \mathcal {F}_p(V)$
and $r=r_{K, V}:K\to \mathcal {F}_p(V)$![]() be as above.
be as above.
For any $x, y \in K$![]() we have
we have
Conversely, there exist $x, y \in K$![]() such that
such that
We develop a series of preliminary results which outline properties of the present construction under dilation and translation.
Lemma 3.3 Let $V \subseteq \mathbb {Z}^d$![]() , $0_V \in V$
, $0_V \in V$![]() , $R>0$
, $R>0$![]() and $x\in \mathbb {Z}$
and $x\in \mathbb {Z}$![]() . Denote $V^\prime = RV+Rx$
. Denote $V^\prime = RV+Rx$![]() , $0_{V'}=R0_V+Rx$
, $0_{V'}=R0_V+Rx$![]() , and consider $0_V$
, and consider $0_V$![]() , $0_{V'}$
, $0_{V'}$![]() as the base points of $V$
as the base points of $V$![]() , $V^\prime$
, $V^\prime$![]() , respectively.
, respectively.
For any $0< p\leq 1$![]() , the map $\tau ^\prime : \delta _{V}(V) \subseteq \mathcal {F}_p (V) \to \mathcal {F}_p (V^\prime )$
, the map $\tau ^\prime : \delta _{V}(V) \subseteq \mathcal {F}_p (V) \to \mathcal {F}_p (V^\prime )$![]() , $\delta _{V}(v) \mapsto \delta _{V^\prime }(Rv+Rx)$
, $\delta _{V}(v) \mapsto \delta _{V^\prime }(Rv+Rx)$![]() , where $v\in V$
, where $v\in V$![]() , extends to an isomorphism $\tau$
, extends to an isomorphism $\tau$![]() of $\mathcal {F}_p (V)$
of $\mathcal {F}_p (V)$![]() and $\mathcal {F}_p (V^\prime )$
and $\mathcal {F}_p (V^\prime )$![]() , such that $\left \Vert \tau (x)\right \Vert _{\mathcal {F}_p (V^\prime )} = R\left \Vert x \right \Vert _{\mathcal {F}_p (V)}$
, such that $\left \Vert \tau (x)\right \Vert _{\mathcal {F}_p (V^\prime )} = R\left \Vert x \right \Vert _{\mathcal {F}_p (V)}$![]() for any $x\in \mathcal {F}_p (V)$
for any $x\in \mathcal {F}_p (V)$![]() .
.
Proof. We note the map $\sigma : V \to V^\prime$![]() , $v \mapsto Rv+Rx$
, $v \mapsto Rv+Rx$![]() , where $v\in V$
, where $v\in V$![]() , is a bi-Lipschitz bijection of $V$
, is a bi-Lipschitz bijection of $V$![]() , $V^\prime$
, $V^\prime$![]() , such that $|\sigma (v)-\sigma (u)|_1=R|v-u|_1$
, such that $|\sigma (v)-\sigma (u)|_1=R|v-u|_1$![]() , where $v,\,u\in V$
, where $v,\,u\in V$![]() .
.
By fact 2.11, there exists an isomorphism $\tau$![]() of spaces $\mathcal {F}_p (V)$
of spaces $\mathcal {F}_p (V)$![]() and $\mathcal {F}_p (V^\prime )$
and $\mathcal {F}_p (V^\prime )$![]() satisfying $\tau \circ \delta _V = \delta _{V^\prime } \circ \sigma$
satisfying $\tau \circ \delta _V = \delta _{V^\prime } \circ \sigma$![]() and $\left \Vert \tau (x)\right \Vert _{\mathcal {F}_p (V^\prime )} = R\left \Vert x \right \Vert _{\mathcal {F}_p (V)}$
and $\left \Vert \tau (x)\right \Vert _{\mathcal {F}_p (V^\prime )} = R\left \Vert x \right \Vert _{\mathcal {F}_p (V)}$![]() , where $x\in \mathcal {F}_p (V)$
, where $x\in \mathcal {F}_p (V)$![]() . Since $\tau \restriction _{\delta _{V}(V)} = \tau ^\prime$
. Since $\tau \restriction _{\delta _{V}(V)} = \tau ^\prime$![]() by the construction, the conclusion follows.
by the construction, the conclusion follows.
Lemma 3.4 Let $d\in \mathbb {N}$![]() , $R>0$
, $R>0$![]() , $\mathcal {R} \subseteq \mathcal {Q}^d_R$
, $\mathcal {R} \subseteq \mathcal {Q}^d_R$![]() , $K = \cup _{Q\in \mathcal {R}} Q$
, $K = \cup _{Q\in \mathcal {R}} Q$![]() and $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$
and $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$![]() . Whenever $x,\,y \in K$
. Whenever $x,\,y \in K$![]() satisfy the condition $y-x\in R\mathbb {Z}^d$
satisfy the condition $y-x\in R\mathbb {Z}^d$![]() , we have that
, we have that
(i) $\Lambda _R^d (v, x)=\Lambda _R^d (v+(y-x), y)$
 , where $v\in R\mathbb {Z}^d$
, where $v\in R\mathbb {Z}^d$ ,
,(ii) $v+(y-x)\in V$
 for any $v\in V$
for any $v\in V$ , $\Lambda ^d_R(v, x)\neq 0$
, $\Lambda ^d_R(v, x)\neq 0$ ,
,(iii) $r(y) = \sum _{v\in V,\;\Lambda ^d_R(v, x)\neq 0} \Lambda ^d_R(v, x)\delta _V(v+(y-x))$
 .
.
Proof. Pick $w,\,u\in \mathbb {Z}^d$![]() such that $x\in Q^d_{w, R} \in \mathcal {R}$
such that $x\in Q^d_{w, R} \in \mathcal {R}$![]() and $y\in Q^d_{u, R} \in \mathcal {R}$
and $y\in Q^d_{u, R} \in \mathcal {R}$![]() .
.
Appealing to the definition of $\Lambda _R^d$![]() , we establish for any $v \in R\mathbb {Z}^d$
, we establish for any $v \in R\mathbb {Z}^d$![]()

where $v+(y-x)\in R\mathbb {Z}^d$![]() by the assumption. This verifies the first claim.
by the assumption. This verifies the first claim.
As $\Lambda ^d_R(v, y) = 0$![]() whenever $v\in \mathcal {V}^d_R$
whenever $v\in \mathcal {V}^d_R$![]() and $v\notin \mathcal {V}(Q^d_{u, R}) \subseteq V$
and $v\notin \mathcal {V}(Q^d_{u, R}) \subseteq V$![]() , we deduce $\{ v+(y-x) : v\in V, \Lambda ^d_R(v, x)\neq 0\} \subseteq \{ v : v\in V,\,\Lambda ^d_R(v, y)\neq 0 \}$
, we deduce $\{ v+(y-x) : v\in V, \Lambda ^d_R(v, x)\neq 0\} \subseteq \{ v : v\in V,\,\Lambda ^d_R(v, y)\neq 0 \}$![]() . This proves the second claim.
. This proves the second claim.
In fact, since the inclusion similarly holds for the role of $x$![]() , $y$
, $y$![]() interchanged, we conclude
interchanged, we conclude
We assert that

Indeed, the first equality follows from the definition of $r$![]() . The second and third equalities rely on equations (3.3) and (3.2), respectively. The proof is now complete.
. The second and third equalities rely on equations (3.3) and (3.2), respectively. The proof is now complete.
We proceed to the proof of the main result. To that end, let us first introduce the following notation.
Notation 3.5 For any $j\in \{1,\,\ldots, d\}$![]() , we define $\pi _j : \mathbb {R}^d \to \mathbb {R}^{d-1}$
, we define $\pi _j : \mathbb {R}^d \to \mathbb {R}^{d-1}$![]() , $(x_i)_{i=1}^d \mapsto (x_1, \ldots, x_{j-1}, x_{j+1} \ldots, x_d)$
, $(x_i)_{i=1}^d \mapsto (x_1, \ldots, x_{j-1}, x_{j+1} \ldots, x_d)$![]() , where $(x_i)_{i=1}^d \in \mathbb {R}^d$
, where $(x_i)_{i=1}^d \in \mathbb {R}^d$![]() .
.
Given $v=(v_i)_{i=1}^{d-1} \in \{ 0, 1\}^{d-1}$![]() , we shall write $v^0=(v^0_i)_{i=1}^{d}$
, we shall write $v^0=(v^0_i)_{i=1}^{d}$![]() and $v^1=(v^1_i)_{i=1}^{d}$
and $v^1=(v^1_i)_{i=1}^{d}$![]() for the elements of $\mathbb \{0, 1\}^{d}$
for the elements of $\mathbb \{0, 1\}^{d}$![]() satisfying $v^0_i=v^1_i=v_i$
satisfying $v^0_i=v^1_i=v_i$![]() , where $i \in \{1, \ldots, d-1\}$
, where $i \in \{1, \ldots, d-1\}$![]() , and $v^0_d=0$
, and $v^0_d=0$![]() , $v^1_d=1$
, $v^1_d=1$![]() , respectively.
, respectively.
Proof. Proof of theorem 3.2
We claim that up to a dilation and a translation, it suffices to consider the case $R=1$![]() and $Q_{0, 1} \in \mathcal {R}$
and $Q_{0, 1} \in \mathcal {R}$![]() . Indeed, if $\mathcal {R}^\prime \subseteq \mathcal {Q}^d_R$
. Indeed, if $\mathcal {R}^\prime \subseteq \mathcal {Q}^d_R$![]() , $K^\prime = \cup _{Q\in \mathcal {R}^\prime } Q$
, $K^\prime = \cup _{Q\in \mathcal {R}^\prime } Q$![]() , $V^\prime = \cup _{Q\in \mathcal {R}^\prime } \mathcal {V}(Q)$
, $V^\prime = \cup _{Q\in \mathcal {R}^\prime } \mathcal {V}(Q)$![]() and $0_{V'}$
and $0_{V'}$![]() is the base point of $V'$
is the base point of $V'$![]() , we may find $\mathcal {R} \subseteq \mathcal {Q}^d_1$
, we may find $\mathcal {R} \subseteq \mathcal {Q}^d_1$![]() , $Q_{0, 1} \in \mathcal {R}$
, $Q_{0, 1} \in \mathcal {R}$![]() , $K = \cup _{Q\in \mathcal {R}} Q$
, $K = \cup _{Q\in \mathcal {R}} Q$![]() , $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$
, $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$![]() , $0_V\in V$
, $0_V\in V$![]() and $w\in \mathbb {Z}^d$
and $w\in \mathbb {Z}^d$![]() , such that $K^\prime =RK+Rw$
, such that $K^\prime =RK+Rw$![]() , $V^\prime =RV+Rw$
, $V^\prime =RV+Rw$![]() and $0_{V'}=R0_V+Rw$
and $0_{V'}=R0_V+Rw$![]() . We define $\sigma : K \to K'$
. We define $\sigma : K \to K'$![]() by $y \mapsto Ry+Rw$
by $y \mapsto Ry+Rw$![]() for $y\in K$
for $y\in K$![]() , and consider $0_V$
, and consider $0_V$![]() as the base point of $V$
as the base point of $V$![]() .
.
We let $\tau : \mathcal {F}_p (V) \to \mathcal {F}_p (V^\prime )$![]() denote the isomorphism from lemma 3.3. Pick $y\in K$
denote the isomorphism from lemma 3.3. Pick $y\in K$![]() . For any $v\in \mathbb {Z}^d$
. For any $v\in \mathbb {Z}^d$![]() , we note that $\Lambda _R^d (Rv+Rw, Ry+Rw)=\Lambda _1^d (v+w, y+w)$
, we note that $\Lambda _R^d (Rv+Rw, Ry+Rw)=\Lambda _1^d (v+w, y+w)$![]() and $\Lambda _1^d (v+w, y+w)=\Lambda _1^d (v, y)$
and $\Lambda _1^d (v+w, y+w)=\Lambda _1^d (v, y)$![]() by the defining property of $\Lambda ^d_R$
by the defining property of $\Lambda ^d_R$![]() and lemma 3.4 (i), respectively. We may thus write
and lemma 3.4 (i), respectively. We may thus write

Since $y\in K$![]() was arbitrary, and using the fact that $\left \Vert \tau (x)\right \Vert _{\mathcal {F}_p (V^\prime )} = R\left \Vert x \right \Vert _{\mathcal {F}_p (V)}$
was arbitrary, and using the fact that $\left \Vert \tau (x)\right \Vert _{\mathcal {F}_p (V^\prime )} = R\left \Vert x \right \Vert _{\mathcal {F}_p (V)}$![]() , where $x\in \mathcal {F}_p (V)$
, where $x\in \mathcal {F}_p (V)$![]() , we deduce for any $y,\,z\in K$
, we deduce for any $y,\,z\in K$![]()
Similarly, we note that $|\sigma (y)-\sigma (z)|_1 = R|y-z|_1$![]() for any $y,\,z\in K$
for any $y,\,z\in K$![]() . As $\sigma$
. As $\sigma$![]() is a bijection of $K$
is a bijection of $K$![]() and $K^\prime$
and $K^\prime$![]() , the claim follows.
, the claim follows.
To establish an upper bound on the Lipschitz constant of $r$![]() , we begin with the case when $x, y\in Q$
, we begin with the case when $x, y\in Q$![]() for some $Q\in \mathcal {R}$
for some $Q\in \mathcal {R}$![]() . To that end, let us first note that for $x=(x_i)_{i=1}^d \in Q^d_{w, 1}\in \mathcal {R}$
. To that end, let us first note that for $x=(x_i)_{i=1}^d \in Q^d_{w, 1}\in \mathcal {R}$![]() , where $w=(w_i)_{i=1}^d\in \mathbb {Z}^d$
, where $w=(w_i)_{i=1}^d\in \mathbb {Z}^d$![]() , it holds by lemma 2.13(i) and (iv)
, it holds by lemma 2.13(i) and (iv)

Pick $x=(x_i)_{i=1}^d, y=(y_i)_{i=1}^d \in Q^d_{w, 1}\in \mathcal {R}$![]() , where $w=(w_i)_{i=1}^d\in \mathbb {Z}^d$
, where $w=(w_i)_{i=1}^d\in \mathbb {Z}^d$![]() . We shall further assume that $x$
. We shall further assume that $x$![]() , $y$
, $y$![]() differ in at most one coordinate; without loss of generality, let $x_i=y_i$
differ in at most one coordinate; without loss of generality, let $x_i=y_i$![]() for any $i\in \{1, \ldots, d-1\}$
for any $i\in \{1, \ldots, d-1\}$![]() . It follows from (3.4) that
. It follows from (3.4) that

We have $\sum _{u\in \mathcal {V}^{d-1} (\pi _d(Q^d_{w, 1}))}\Lambda ^{d-1}_1(u, \pi _d(x))=1$![]() by lemma 2.13 (iii). Since also $\left \Vert \delta _V(w+u^1)-\delta _V(w+u^0)\right \Vert _p=1$
by lemma 2.13 (iii). Since also $\left \Vert \delta _V(w+u^1)-\delta _V(w+u^0)\right \Vert _p=1$![]() for any $u\in \{0,\,1\}^{d-1}$
for any $u\in \{0,\,1\}^{d-1}$![]() , we deduce the inequality
, we deduce the inequality
For any $x=(x_i)_{i=1}^d, y=(y_i)_{i=1}^d \in Q^d_{w, 1}\in \mathcal {R}$![]() we may now take a sequence $(z^i)_{i=0}^d = ((z^i_j)_{j=1}^d)_{i=0}^d \in (Q^d_{w, 1})^{d+1}$
we may now take a sequence $(z^i)_{i=0}^d = ((z^i_j)_{j=1}^d)_{i=0}^d \in (Q^d_{w, 1})^{d+1}$![]() such that for any $i\in \{0, \ldots, d\}$
such that for any $i\in \{0, \ldots, d\}$![]() and $j\in \{1, \ldots, d\}$
and $j\in \{1, \ldots, d\}$![]() , it holds that $z^i_j=x_j$
, it holds that $z^i_j=x_j$![]() if and only if $i\geq j$
if and only if $i\geq j$![]() and $z^i_j=y_j$
and $z^i_j=y_j$![]() otherwise. Any two consecutive elements of the sequence $(z^i)_{i=1}^d$
otherwise. Any two consecutive elements of the sequence $(z^i)_{i=1}^d$![]() differ in at most one coordinate, and using the inequality (3.6) we establish
differ in at most one coordinate, and using the inequality (3.6) we establish

hence $\left \Vert r(x)-r(y) \right \Vert _p \leq C(p, 2^{d-1})C(p, d)|x-y|_1$![]() .
.
As for the general case, we consider $x=(x_i)_{i=1}^d \in Q^d_{w, 1}\in \mathcal {R}$![]() and $y=(y_i)_{i=1}^d \in Q^d_{u, 1}\in \mathcal {R}$
and $y=(y_i)_{i=1}^d \in Q^d_{u, 1}\in \mathcal {R}$![]() , where $w, u \in \mathbb {Z}^d$
, where $w, u \in \mathbb {Z}^d$![]() . Let us denote $I = \{ i \in \{1, \ldots, d\} : w_i \neq u_i\}$
. Let us denote $I = \{ i \in \{1, \ldots, d\} : w_i \neq u_i\}$![]() .
.
Given $i \in I$![]() , we may find $n_i, m_i \in \mathbb {Z}$
, we may find $n_i, m_i \in \mathbb {Z}$![]() such that $n_i\in \{w_i, w_i+1\}$
such that $n_i\in \{w_i, w_i+1\}$![]() , $m_i\in \{u_i, u_i+1\}$
, $m_i\in \{u_i, u_i+1\}$![]() and $|x_i-y_i|=|x_i-n_i|+|n_i-m_i|+|m_i-y_i|$
and $|x_i-y_i|=|x_i-n_i|+|n_i-m_i|+|m_i-y_i|$![]() . Let us also pick $x^\prime = (x^\prime _i)_{i=1}^d \in \mathbb {R}^d$
. Let us also pick $x^\prime = (x^\prime _i)_{i=1}^d \in \mathbb {R}^d$![]() , $y^\prime = (y^\prime _i)_{i=1}^d \in \mathbb {R}^d$
, $y^\prime = (y^\prime _i)_{i=1}^d \in \mathbb {R}^d$![]() such that $x^\prime _i=y^\prime _i=x_i$
such that $x^\prime _i=y^\prime _i=x_i$![]() if and only if $i\in \{1, \ldots, d\} \setminus I$
if and only if $i\in \{1, \ldots, d\} \setminus I$![]() and $x^\prime _i=n_i$
and $x^\prime _i=n_i$![]() , $y^\prime _i=m_i$
, $y^\prime _i=m_i$![]() otherwise, respectively. It follows after short thought that $x^\prime \in Q^d_{w, 1}$
otherwise, respectively. It follows after short thought that $x^\prime \in Q^d_{w, 1}$![]() , $y^\prime \in Q^d_{u, 1}$
, $y^\prime \in Q^d_{u, 1}$![]() and $|x-y|_1=|x-x^\prime |_1+|x^\prime -y^\prime |_1+|y^\prime -y|_1$
and $|x-y|_1=|x-x^\prime |_1+|x^\prime -y^\prime |_1+|y^\prime -y|_1$![]() .
.
We note that $y^\prime -x^\prime \in \mathbb {Z}^d$![]() ; hence lemma 3.4 applies and
; hence lemma 3.4 applies and

If $I$![]() was empty, then necessarily $r(x^\prime )=r(y^\prime )$
was empty, then necessarily $r(x^\prime )=r(y^\prime )$![]() . Otherwise at least one coordinate of $x^\prime$
. Otherwise at least one coordinate of $x^\prime$![]() assumes value in $\mathbb {Z}$
assumes value in $\mathbb {Z}$![]() ; we claim $|\{ v\in \mathcal {V}(Q): \Lambda ^d_1(v, x^\prime ) \neq 0 \}| \leq 2^{d-1}$
; we claim $|\{ v\in \mathcal {V}(Q): \Lambda ^d_1(v, x^\prime ) \neq 0 \}| \leq 2^{d-1}$![]() in this case. To that end, let $x'_j \in \mathbb {Z}$
in this case. To that end, let $x'_j \in \mathbb {Z}$![]() for some $j\in \{1, \ldots, d\}$
for some $j\in \{1, \ldots, d\}$![]() , and define $w'_j=w_j+1$
, and define $w'_j=w_j+1$![]() if and only if $x'_j=w_j+1$
if and only if $x'_j=w_j+1$![]() and $w'_j=w_j-1$
and $w'_j=w_j-1$![]() otherwise. If we now denote $w' = (w_1, \ldots, w_{j-1}, w'_j, w_{j+1}, \ldots, w_d)\in \mathbb {Z}^d$
otherwise. If we now denote $w' = (w_1, \ldots, w_{j-1}, w'_j, w_{j+1}, \ldots, w_d)\in \mathbb {Z}^d$![]() , it follows that $w\neq w'$
, it follows that $w\neq w'$![]() and $x^\prime \in Q_{w, 1} \cap Q_{w', 1}$
and $x^\prime \in Q_{w, 1} \cap Q_{w', 1}$![]() . We have that $\Lambda ^d_1 (v, x') = 0$
. We have that $\Lambda ^d_1 (v, x') = 0$![]() whenever $v\notin \mathcal {V}(Q_{w, 1}) \cap \mathcal {V}(Q_{w', 1})$
whenever $v\notin \mathcal {V}(Q_{w, 1}) \cap \mathcal {V}(Q_{w', 1})$![]() by lemma 2.13 (i). Since $|\mathcal {V}(Q_{w, 1}) \cap \mathcal {V}(Q_{w', 1})|\leq 2^{d-1}$
by lemma 2.13 (i). Since $|\mathcal {V}(Q_{w, 1}) \cap \mathcal {V}(Q_{w', 1})|\leq 2^{d-1}$![]() , the claim follows.
, the claim follows.
As $\left \Vert \delta _V(v)-\delta _V(v+(y^\prime -x^\prime ))\right \Vert _p=|x^\prime -y^\prime |_1$![]() for any $v\in \mathcal {V}(Q)$
for any $v\in \mathcal {V}(Q)$![]() , $\Lambda ^d_1(v, x^\prime )\neq 0$
, $\Lambda ^d_1(v, x^\prime )\neq 0$![]() and $\sum _{v\in V} \Lambda ^d_1(v, x^\prime ) = 1$
and $\sum _{v\in V} \Lambda ^d_1(v, x^\prime ) = 1$![]() by lemma 2.13 (iii), in either case we are thus justified to establish the inequality
by lemma 2.13 (iii), in either case we are thus justified to establish the inequality
Using the already proven parts and the fact that $x,\,x^\prime \in Q^d_{w, 1}$![]() , $y,\,y^\prime \in Q^d_{u, 1}$
, $y,\,y^\prime \in Q^d_{u, 1}$![]() , we deduce
, we deduce

and altogether we obtain $\left \Vert r(x)-r(y) \right \Vert _p \leq C(p, 2^{d-1})C(p, d)C(p, 3)|x-y|_1$![]() .
.
Regarding the converse part, we let $\mathcal {R} \subseteq \mathcal {Q}^d_R$![]() , where $\mathcal {R} \neq \emptyset$
, where $\mathcal {R} \neq \emptyset$![]() , $K = \cup _{Q\in \mathcal {R}} Q$
, $K = \cup _{Q\in \mathcal {R}} Q$![]() , $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$
, $V = \cup _{Q\in \mathcal {R}} \mathcal {V}(Q)$![]() , and consider $0_V$
, and consider $0_V$![]() as the base point of $V$
as the base point of $V$![]() . By the initial remark, it suffices to consider the case $R=1$
. By the initial remark, it suffices to consider the case $R=1$![]() and $Q_{0, 1} \in \mathcal {R}$
and $Q_{0, 1} \in \mathcal {R}$![]() .
.
We let $x = (x_i)_{i=1}^d$![]() , $y = (y_i)_{i=1}^d$
, $y = (y_i)_{i=1}^d$![]() be such that $x_i=y_i=1/2$
be such that $x_i=y_i=1/2$![]() , for each $i\in \{1, \ldots, d-1\}$
, for each $i\in \{1, \ldots, d-1\}$![]() , and $x_d=0$
, and $x_d=0$![]() , $y_d=1$
, $y_d=1$![]() . Recall that using (3.5), we have $r(y)-r(x) = \sum _{u\in \mathcal \{0, 1\}^{d-1}} 2^{-d+1}(\delta _V(u^1)-\delta _V(u^0))$
. Recall that using (3.5), we have $r(y)-r(x) = \sum _{u\in \mathcal \{0, 1\}^{d-1}} 2^{-d+1}(\delta _V(u^1)-\delta _V(u^0))$![]() .
.
Denote $\mathcal {M} = \left \{ {\delta (z)-\delta (z')}/{|z-z'|_1}: z,\,z' \in V, z\neq z' \right \}$![]() ; by fact 2.9, we have
; by fact 2.9, we have

the infimum being taken over all $n\in \mathbb {N}_0$![]() and $\mu _i\in \mathcal {M}$
and $\mu _i\in \mathcal {M}$![]() , $a_i\in \mathbb {R}$
, $a_i\in \mathbb {R}$![]() , where $i\in \{1, \ldots, n\}$
, where $i\in \{1, \ldots, n\}$![]() , such that $r(y)-r(x) = \sum _{i=1}^n a_i\mu _i$
, such that $r(y)-r(x) = \sum _{i=1}^n a_i\mu _i$![]() .
.
We shall see that $\left \Vert r(x) - r(y) \right \Vert _p \geq C(p, 2^{d-1})|x-y|_1 = C(p, 2^{d-1})$![]() . To that end, pick $n\in \mathbb {N}_0$
. To that end, pick $n\in \mathbb {N}_0$![]() and $a_i\in \mathbb {R}$
and $a_i\in \mathbb {R}$![]() , $\mu _i\in \mathcal {M}$
, $\mu _i\in \mathcal {M}$![]() , where $i \in \{1, \ldots, n\}$
, where $i \in \{1, \ldots, n\}$![]() , as above.
, as above.
We introduce $\mathcal {N} : \mathcal {V}(Q_{0, 1}) \to \mathcal {P} (\{1, \ldots, n\})$![]() as
as
It follows that for any $i \in \{1, \ldots, n\}$![]() , there exist at most two distinct elements $u, v \in \mathcal {V}(Q_{0, 1})$
, there exist at most two distinct elements $u, v \in \mathcal {V}(Q_{0, 1})$![]() such that $i \in \mathcal {N}(u) \cap \mathcal {N}(v)$
such that $i \in \mathcal {N}(u) \cap \mathcal {N}(v)$![]() ; consequently, $\sum _{i=1}^n |a_i|^p \geq 1/2 \sum _{u\in \mathcal {V}(Q_{0, 1})} \sum _{i\in \mathcal {N}(u)} |a_i|^p$
; consequently, $\sum _{i=1}^n |a_i|^p \geq 1/2 \sum _{u\in \mathcal {V}(Q_{0, 1})} \sum _{i\in \mathcal {N}(u)} |a_i|^p$![]() .
.
We claim that $\sum _{i\in \mathcal {N}(u)} |a_i| \geq 2^{-d+1}$![]() for any $u \in \mathcal {V}(Q_{0, 1})$
for any $u \in \mathcal {V}(Q_{0, 1})$![]() .
.
To that end, we pick $u \in \mathcal {V}(Q_{0, 1})$![]() and construct $\varphi : V\to \mathbb {R}$
and construct $\varphi : V\to \mathbb {R}$![]() as follows: If $\chi _{A}$
as follows: If $\chi _{A}$![]() denotes the indicator function of a set $A\subseteq V$
denotes the indicator function of a set $A\subseteq V$![]() , we define $\varphi = \chi _{\{u\}}$
, we define $\varphi = \chi _{\{u\}}$![]() if $u\neq 0_V$
if $u\neq 0_V$![]() and $\varphi =\chi _{V\setminus \{ u \}}$
and $\varphi =\chi _{V\setminus \{ u \}}$![]() otherwise. We note that $\varphi \in \operatorname {Lip}_0 (V)$
otherwise. We note that $\varphi \in \operatorname {Lip}_0 (V)$![]() , $\operatorname {Lip} \varphi \leq 1$
, $\operatorname {Lip} \varphi \leq 1$![]() , as $\varphi (0_V)=0$
, as $\varphi (0_V)=0$![]() and $|\varphi (z)-\varphi (z)|\leq 1$
and $|\varphi (z)-\varphi (z)|\leq 1$![]() , $|z-z'|_1 \geq 1$
, $|z-z'|_1 \geq 1$![]() for any two $z, z' \in V$
for any two $z, z' \in V$![]() , $z\neq z'$
, $z\neq z'$![]() . By (iii) of $\mathcal {F}_p (V)$
. By (iii) of $\mathcal {F}_p (V)$![]() , we let $\varphi ^*\in \mathcal {F}_p (V)^*$
, we let $\varphi ^*\in \mathcal {F}_p (V)^*$![]() be such that $\varphi =\varphi ^*\circ \delta _V$
be such that $\varphi =\varphi ^*\circ \delta _V$![]() on the set $V$
on the set $V$![]() .
.
We observe that $\left |\left \langle \varphi ^*, \mu _i \right \rangle \right | \leq 1$![]() for any $i \in \{1, \ldots, n\}$
for any $i \in \{1, \ldots, n\}$![]() , where $\left \langle \varphi ^*, \mu _i \right \rangle = 0$
, where $\left \langle \varphi ^*, \mu _i \right \rangle = 0$![]() for each $i \in \{1, \ldots, n\} \setminus \mathcal {N} (u)$
for each $i \in \{1, \ldots, n\} \setminus \mathcal {N} (u)$![]() . Hence, we may write
. Hence, we may write

and

and the intermediate claim is established.
We note that $x\mapsto x^p$![]() is subadditive on $[0, \infty )$
is subadditive on $[0, \infty )$![]() , and thus $\sum _{i\in \mathcal {N}(u)} |a_i|^p \geq 2^{p(-d+1)}$
, and thus $\sum _{i\in \mathcal {N}(u)} |a_i|^p \geq 2^{p(-d+1)}$![]() for any $u \in \mathcal {V}(Q_{0, 1})$
for any $u \in \mathcal {V}(Q_{0, 1})$![]() . Altogether, we are justified to establish
. Altogether, we are justified to establish

which concludes the proof.
Let us recall a closely related result which shows that $\mathcal {F}_p ([0, 1]^d)$![]() has a Schauder basis, consider [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8]. In fact, the associated canonical projections are the retractions $r$
has a Schauder basis, consider [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8]. In fact, the associated canonical projections are the retractions $r$![]() ; hence, theorem 3.2 gives a refined estimate on the basis constant.
; hence, theorem 3.2 gives a refined estimate on the basis constant.
4. On the geometry of $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha )$ , where $(\mathcal {M}, \rho )$
, where $(\mathcal {M}, \rho )$ is infinite doubling
is infinite doubling
We recall that a metric space $(\mathcal {M}, \rho )$![]() is doubling if there exists $\lambda _{\mathcal {M}} \in \mathbb {N}$
is doubling if there exists $\lambda _{\mathcal {M}} \in \mathbb {N}$![]() , called the doubling constant, such that any closed ball in $\mathcal {M}$
, called the doubling constant, such that any closed ball in $\mathcal {M}$![]() of radius $2r>0$
of radius $2r>0$![]() can be covered by $\lambda _{\mathcal {M}}$
can be covered by $\lambda _{\mathcal {M}}$![]() -many closed balls of radius $r$
-many closed balls of radius $r$![]() . As an example, any subspace of a finite-dimensional Banach space is doubling.
. As an example, any subspace of a finite-dimensional Banach space is doubling.
A classical result due to Assouad [Reference Assouad5] implies that for any $\alpha \in (0, 1)$![]() , the metric snowflake $(\mathcal {M}, \rho ^\alpha )$
, the metric snowflake $(\mathcal {M}, \rho ^\alpha )$![]() is bi-Lipschitz equivalent to $(\mathcal {M}', |\cdot |^\beta )$
is bi-Lipschitz equivalent to $(\mathcal {M}', |\cdot |^\beta )$![]() for some $\mathcal {M}' \subseteq \mathbb {R}^d$
for some $\mathcal {M}' \subseteq \mathbb {R}^d$![]() , where $d\in \mathbb {N}$
, where $d\in \mathbb {N}$![]() and $\beta \in (0,\,1)$
and $\beta \in (0,\,1)$![]() . As a consequence, it follows that $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha ) \simeq \mathcal {F}_p (\mathcal {M}', | \cdot |^\beta )$
. As a consequence, it follows that $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha ) \simeq \mathcal {F}_p (\mathcal {M}', | \cdot |^\beta )$![]() for any $0< p\leq 1$
for any $0< p\leq 1$![]() , see fact 2.11.
, see fact 2.11.
Moreover, it was observed in [Reference Albiac, Ansorena, Cúth and Doucha3] that, for infinite subsets $\mathcal {M}$![]() of $\mathbb {R}^d$
of $\mathbb {R}^d$![]() , the isomorphism theorem reduces to the case where $\mathcal {M}= [0, 1]^d$
, the isomorphism theorem reduces to the case where $\mathcal {M}= [0, 1]^d$![]() . Hence, the central topic of this section is to fill in the missing part and establish that $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )\simeq \ell _p$
. Hence, the central topic of this section is to fill in the missing part and establish that $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )\simeq \ell _p$![]() , for any $0 < \alpha < 1$
, for any $0 < \alpha < 1$![]() and $0< p\leq 1$
and $0< p\leq 1$![]() .
.
4.1 The isomorphism $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )\simeq \ell _p$
It is easy to see that for any fixed $0<\alpha < 1$![]() , the associated snowflake distortion of any two norms $|\cdot |$
, the associated snowflake distortion of any two norms $|\cdot |$![]() , $|\cdot |_*$
, $|\cdot |_*$![]() on $\mathbb {R}^d$
on $\mathbb {R}^d$![]() are Lipschitz equivalent; thus, $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ) \simeq \mathcal {F}_p ([0, 1]^d, |\cdot |_*^\alpha )$
are Lipschitz equivalent; thus, $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ) \simeq \mathcal {F}_p ([0, 1]^d, |\cdot |_*^\alpha )$![]() for any $0< p\leq 1$
for any $0< p\leq 1$![]() , again by fact 2.11. It will be convenient to identify $|\cdot |$
, again by fact 2.11. It will be convenient to identify $|\cdot |$![]() as the $\ell _1$
as the $\ell _1$![]() -norm in the sequel.
-norm in the sequel.
Theorem 4.1 Let $d\in \mathbb {N}$![]() and $0 < \alpha < 1$
and $0 < \alpha < 1$![]() , $0< p\leq 1$
, $0< p\leq 1$![]() . Then $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
. Then $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() is isomorphic to the space $\ell _p$
is isomorphic to the space $\ell _p$![]() .
.
Quantitatively, if we consider the Banach–Mazur distance (see, e.g. [Reference Jaegermann11, p. 277]) of $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() and $\ell _p (V)$
and $\ell _p (V)$![]() defined by $d(\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ), \ell _p (V)) = \inf \, \lVert T \rVert \lVert T^{-1}\rVert$
defined by $d(\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ), \ell _p (V)) = \inf \, \lVert T \rVert \lVert T^{-1}\rVert$![]() , where $T$
, where $T$![]() ranges over onto isomorphisms $T: \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ) \to \ell _p (V)$
ranges over onto isomorphisms $T: \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ) \to \ell _p (V)$![]() , we show that $d(\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ), \ell _p (V)) \leq C(p, 2^d)\rho ^d\tau ^d$
, we show that $d(\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ), \ell _p (V)) \leq C(p, 2^d)\rho ^d\tau ^d$![]() , where $\rho$
, where $\rho$![]() and $\tau$
and $\tau$![]() are as in lemmas 4.8 and 4.4, respectively.
are as in lemmas 4.8 and 4.4, respectively.
Pick $0 < \alpha < 1$![]() and $0< p\leq 1$
and $0< p\leq 1$![]() . Whenever $d\in \mathbb {N}$
. Whenever $d\in \mathbb {N}$![]() is fixed and known from the context, we shall denote $V_{-1} = \{ 0 \}$
is fixed and known from the context, we shall denote $V_{-1} = \{ 0 \}$![]() , $V_k = [0, 1]^d \cap 2^{-k}\mathbb {Z}^d$
, $V_k = [0, 1]^d \cap 2^{-k}\mathbb {Z}^d$![]() , where $k\in \mathbb {N}_0$
, where $k\in \mathbb {N}_0$![]() , and $V = \cup _{k\in \mathbb {N}_0} V_k\setminus V_{k-1}$
, and $V = \cup _{k\in \mathbb {N}_0} V_k\setminus V_{k-1}$![]() . We take $0$
. We take $0$![]() as the base point of $[0, 1]^d$
as the base point of $[0, 1]^d$![]() and write $\delta : [0, 1]^d \to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
and write $\delta : [0, 1]^d \to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() for the canonical isometric embedding.
for the canonical isometric embedding.
The proof proceeds by considering the map $\iota : \{ e_v : v\in V \} \subset \ell _p (V) \to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() ,
,

A brief consideration shows that $\{ e_v : v\in V\}$![]() is $p$
is $p$![]() -norming (see definition 2.3) in $\ell _p (V)$
-norming (see definition 2.3) in $\ell _p (V)$![]() and that $\iota$
and that $\iota$![]() extends to a one-to-one linear map from $\operatorname {span} \{ e_v : v\in V\}$
extends to a one-to-one linear map from $\operatorname {span} \{ e_v : v\in V\}$![]() into $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
into $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() . Hence, by lemma 2.4, $\iota$
. Hence, by lemma 2.4, $\iota$![]() shall provide us with an onto isomorphism $\tilde \iota : \ell _p(V)\to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
shall provide us with an onto isomorphism $\tilde \iota : \ell _p(V)\to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() once we show that $\iota (\{e_v : v\in V\})$
once we show that $\iota (\{e_v : v\in V\})$![]() is $p$
is $p$![]() -norming in $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
-norming in $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() .
.
Let us note this particular choice of $\iota (e_v)$![]() , where $v\in V$
, where $v\in V$![]() , is frequent in the theory of Lipschitz-free spaces over an Euclidean space. Indeed, a dual variant thereof is used in the standard proof of the $p=1$
, is frequent in the theory of Lipschitz-free spaces over an Euclidean space. Indeed, a dual variant thereof is used in the standard proof of the $p=1$![]() case (see, e.g. [Reference Weaver18, Theorem 8.44]), and it is known that $\iota (e_v)$
case (see, e.g. [Reference Weaver18, Theorem 8.44]), and it is known that $\iota (e_v)$![]() , where $v\in V$
, where $v\in V$![]() , forms a Schauder basis of $\mathcal {F}_p ([0, 1]^d, |\cdot |)$
, forms a Schauder basis of $\mathcal {F}_p ([0, 1]^d, |\cdot |)$![]() , see [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8].
, see [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8].
Notation 4.2 If $d\in \mathbb {N}$![]() is fixed, $x=(x_i)_{i=1}^d \in \mathbb {R}^d$
is fixed, $x=(x_i)_{i=1}^d \in \mathbb {R}^d$![]() , $j\in \{1, \ldots, d\}$
, $j\in \{1, \ldots, d\}$![]() , and $\epsilon \in \mathbb {R}$
, and $\epsilon \in \mathbb {R}$![]() , let us set $x^j_\epsilon =(x_1, \ldots, x_j+\epsilon, \ldots, x_d)\in \mathbb {R}^d$
, let us set $x^j_\epsilon =(x_1, \ldots, x_j+\epsilon, \ldots, x_d)\in \mathbb {R}^d$![]() . If $x\in [0, 1]$
. If $x\in [0, 1]$![]() , $x\in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$
, $x\in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$![]() , for some $n\in \mathbb {N}$
, for some $n\in \mathbb {N}$![]() , we will write $x^+ = x+2^{-n}$
, we will write $x^+ = x+2^{-n}$![]() , $x^- = x-2^{-n}$
, $x^- = x-2^{-n}$![]() .
.
For $d\in \mathbb {N}$![]() , $j\in \{1, \ldots, d\}$
, $j\in \{1, \ldots, d\}$![]() , and $(x_i)_{i=1}^{d-1}\in [0, 1]^{d-1}$
, and $(x_i)_{i=1}^{d-1}\in [0, 1]^{d-1}$![]() , we furthermore adopt the notation $\delta ^j_{(x_i)_{i=1}^{d-1}} (x) = \delta (x_1, \ldots, x_{j-1}, x, x_j, \ldots, x_{d-1})$
, we furthermore adopt the notation $\delta ^j_{(x_i)_{i=1}^{d-1}} (x) = \delta (x_1, \ldots, x_{j-1}, x, x_j, \ldots, x_{d-1})$![]() .
.
4.1.1 One linearization result
We expand the element $\delta (v)$![]() , where $v\in V$
, where $v\in V$![]() , in a way that partially recovers the candidate basis geometry. This will have a great importance for the construction considered in lemma 4.4.
, in a way that partially recovers the candidate basis geometry. This will have a great importance for the construction considered in lemma 4.4.
Lemma 4.3 Let $u_1, u_2 \in [0, 1]\cap 2^{-n}\mathbb {Z}$![]() for some $n\in \mathbb {N}_0$
for some $n\in \mathbb {N}_0$![]() , $u_1< u_2$
, $u_1< u_2$![]() and $|u_1-u_2|=2^{-n}$
and $|u_1-u_2|=2^{-n}$![]() . Let $v\in [u_1, u_2]$
. Let $v\in [u_1, u_2]$![]() satisfy $v\in 2^{-k}\mathbb {Z}$
satisfy $v\in 2^{-k}\mathbb {Z}$![]() , where $k\in \mathbb {N}$
, where $k\in \mathbb {N}$![]() , $k\geq n$
, $k\geq n$![]() .
.
There exist $\mu _1, \mu _2 \geq 0$![]() , $\mu _1+\mu _2=1$
, $\mu _1+\mu _2=1$![]() , $l \in \mathbb {N}_0$
, $l \in \mathbb {N}_0$![]() , $\nu _i > 0$
, $\nu _i > 0$![]() , $n_i \in \mathbb {N}$
, $n_i \in \mathbb {N}$![]() , $n_i>n$
, $n_i>n$![]() , and $v_i \in 2^{-n_i}\mathbb {Z} \setminus 2^{-n_i+1}\mathbb {Z}$
, and $v_i \in 2^{-n_i}\mathbb {Z} \setminus 2^{-n_i+1}\mathbb {Z}$![]() , where $i \in \{1, \ldots, l\}$
, where $i \in \{1, \ldots, l\}$![]() , such that
, such that

for any $d\in \mathbb {N}$![]() , $j\in \{1, \ldots, d\}$
, $j\in \{1, \ldots, d\}$![]() , and $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$
, and $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$![]() .
.
Moreover, we have $(\sum _{i=1}^l |\nu _i|^p)^{1/p} \leq 2^{-\alpha }({1}/{1-2^{-p\alpha }})^{1/p}$![]() .
.
Proof. We shall establish that for any $k\in \mathbb {N}$![]() , $k\geq n$
, $k\geq n$![]() and $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$
and $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$![]() , we can choose the coefficients $\mu ^v_1, \mu ^v_2 \geq 0$
, we can choose the coefficients $\mu ^v_1, \mu ^v_2 \geq 0$![]() , $l^v \in \mathbb {N}_0$
, $l^v \in \mathbb {N}_0$![]() , $\nu ^v_i \in [0, 1]$
, $\nu ^v_i \in [0, 1]$![]() , $v^v_i \in [u_1, u_2]$
, $v^v_i \in [u_1, u_2]$![]() and $n^v_i \in \mathbb {N}$
and $n^v_i \in \mathbb {N}$![]() , where $i\in \{1, \ldots, l^v\}$
, where $i\in \{1, \ldots, l^v\}$![]() , so that for any $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$
, so that for any $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$![]() , it holds
, it holds
(i) $\mu ^v_1, \, \mu ^v_2 \geq 0$
 , $\mu ^v_1+\mu ^v_2=1$
, $\mu ^v_1+\mu ^v_2=1$ and $k\geq n^v_i > n$
and $k\geq n^v_i > n$ for every $i \in \{1, \ldots, l^v\}$
for every $i \in \{1, \ldots, l^v\}$ ,
,(ii) if $v^\prime \in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$
 , there exists at most one index $i\in \{1, \ldots, l^v\}$
, there exists at most one index $i\in \{1, \ldots, l^v\}$ such that $v^v_i=v^\prime$
such that $v^v_i=v^\prime$ ,
,(iii) $v^v_i \in [u_1, u_2] \cap 2^{-n^v_i}\mathbb {Z} \setminus 2^{-n^v_i+1}\mathbb {Z}$
 for every $i \in \{1, \ldots, l^v\}$
for every $i \in \{1, \ldots, l^v\}$ ,
,(iv) for any $j\in \{1, \ldots, d\}$
 and $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$
and $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$ , we have
\begin{align*} 2^{n\alpha}\delta^j_x(v) & = \mu^v_1 2^{n\alpha}\delta^j_x(u_1)+\mu^v_2 2^{n\alpha}\delta^j_x(u_2)\\ & \phantom={+}\sum_{i=1}^l \nu^v_i 2^{n^v_i\alpha}\left(\delta^j_x (v^v_i) - \frac 1 2 (\delta^j_x ((v^v_i)^-)+\delta^j_x ((v^v_i)^+))\right), \end{align*}
, we have
\begin{align*} 2^{n\alpha}\delta^j_x(v) & = \mu^v_1 2^{n\alpha}\delta^j_x(u_1)+\mu^v_2 2^{n\alpha}\delta^j_x(u_2)\\ & \phantom={+}\sum_{i=1}^l \nu^v_i 2^{n^v_i\alpha}\left(\delta^j_x (v^v_i) - \frac 1 2 (\delta^j_x ((v^v_i)^-)+\delta^j_x ((v^v_i)^+))\right), \end{align*}
(v) $0<\nu ^v_i\leq 2^{(n-n^v_i)\alpha }$
 for every $i \in \{1, \ldots, l^v\}$
for every $i \in \{1, \ldots, l^v\}$ ,
,(vi) if $v^\prime \in [u_1, u_2] \cap 2^{-k+1}\mathbb {Z}$
 is such that $|v-v^\prime |=2^{-k}$
is such that $|v-v^\prime |=2^{-k}$ , then $\{ v^{v^\prime }_i : i\in \{1, \ldots, l^{v^\prime }\}\} \subseteq \{ v^{v}_i : i\in \{1, \ldots, l^{v}\}\}$
, then $\{ v^{v^\prime }_i : i\in \{1, \ldots, l^{v^\prime }\}\} \subseteq \{ v^{v}_i : i\in \{1, \ldots, l^{v}\}\}$ ,
,(vii) $|\{ v^{v}_i : i\in \{1, \ldots, l^{v}\}\} \cap 2^{-m}\mathbb {Z} \setminus 2^{-m+1}\mathbb {Z}\} | \leq 1$
 for any $m \in \mathbb {N}$
for any $m \in \mathbb {N}$ , $m>n$
, $m>n$ .
.
We proceed by induction on $k$![]() . To that end, note that for $k=n$
. To that end, note that for $k=n$![]() , we may take $l^v=0$
, we may take $l^v=0$![]() . We also set either $\mu ^v_1=1$
. We also set either $\mu ^v_1=1$![]() , $\mu ^v_2=0$
, $\mu ^v_2=0$![]() or $\mu ^v_1=0$
or $\mu ^v_1=0$![]() , $\mu ^v_2=1$
, $\mu ^v_2=1$![]() when $v$
when $v$![]() equals $u_1$
equals $u_1$![]() or $u_2$
or $u_2$![]() , respectively.
, respectively.
Let $k\in \mathbb {N}$![]() , $k>n$
, $k>n$![]() , be such that the claim holds for $k-1$
, be such that the claim holds for $k-1$![]() .
.
For any $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$![]() , we define the coefficients $\mu ^v_1,\,\mu ^v_2 \in \mathbb {R}$
, we define the coefficients $\mu ^v_1,\,\mu ^v_2 \in \mathbb {R}$![]() , $l^v \in \mathbb {N}_0$
, $l^v \in \mathbb {N}_0$![]() , $\nu ^v_i \in [0, 1]$
, $\nu ^v_i \in [0, 1]$![]() , $n^v_i \in \mathbb {N}$
, $n^v_i \in \mathbb {N}$![]() , $k\geq n^v_i>n$
, $k\geq n^v_i>n$![]() , $i \in \{1, \ldots, l^v\}$
, $i \in \{1, \ldots, l^v\}$![]() , as follows. If $v\in 2^{-k+1}\mathbb {Z}$
, as follows. If $v\in 2^{-k+1}\mathbb {Z}$![]() , we take the coefficients from the induction hypothesis. Otherwise $v\in 2^{-k} \mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$
, we take the coefficients from the induction hypothesis. Otherwise $v\in 2^{-k} \mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$![]() , and hence, both $v^-$
, and hence, both $v^-$![]() and $v^+$
and $v^+$![]() belong to $[u_1, u_2] \cap 2^{-k+1}\mathbb {Z}$
belong to $[u_1, u_2] \cap 2^{-k+1}\mathbb {Z}$![]() . For any $d\in \mathbb {N}$
. For any $d\in \mathbb {N}$![]() , $j\in \{1, \ldots, d\}$
, $j\in \{1, \ldots, d\}$![]() , and $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$
, and $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$![]() , we may write
, we may write

Let now $\mu ^{v^+}_1$![]() , $\mu ^{v^+}_2$
, $\mu ^{v^+}_2$![]() , $l^{v^+}$
, $l^{v^+}$![]() , $\nu ^{v^+}_i$
, $\nu ^{v^+}_i$![]() , $n^{v^+}_i$
, $n^{v^+}_i$![]() , $v^{v^+}_i$
, $v^{v^+}_i$![]() , $i \in \{1, \ldots, l^{v^+}\}$
, $i \in \{1, \ldots, l^{v^+}\}$![]() , and $\mu ^{v^-}_1, \mu ^{v^-}_2$
, and $\mu ^{v^-}_1, \mu ^{v^-}_2$![]() , $l^{v^-}$
, $l^{v^-}$![]() , $\nu ^{v^-}_i$
, $\nu ^{v^-}_i$![]() , $n^{v^-}_i$
, $n^{v^-}_i$![]() , $v^{v^+}_i$
, $v^{v^+}_i$![]() , $i \in \{1, \ldots, l^{v^-}\}$
, $i \in \{1, \ldots, l^{v^-}\}$![]() , be the coefficients corresponding to $v^+$
, be the coefficients corresponding to $v^+$![]() and $v^-$
and $v^-$![]() , respectively. We denote $\mathcal {V} = \{ v^{v^+}_i : i\in \{1, \ldots, l^{v^+}\}\} \cup \{ v^{v^-}_i : i\in \{1, \ldots, l^{v^-}\}\}$
, respectively. We denote $\mathcal {V} = \{ v^{v^+}_i : i\in \{1, \ldots, l^{v^+}\}\} \cup \{ v^{v^-}_i : i\in \{1, \ldots, l^{v^-}\}\}$![]() . Finally, set $l^v = |\mathcal {V}|+1$
. Finally, set $l^v = |\mathcal {V}|+1$![]() .
.
Let $v^v_i$![]() , $i\in \{1, \ldots, l^v-1\}$
, $i\in \{1, \ldots, l^v-1\}$![]() , be such that $\{ v^v_i : i\in \{1, \ldots, l^v-1\}\}=\mathcal {V}$
, be such that $\{ v^v_i : i\in \{1, \ldots, l^v-1\}\}=\mathcal {V}$![]() ; such an arrangement of the finite set $\mathcal {V}$
; such an arrangement of the finite set $\mathcal {V}$![]() necessarily exists. By the choice of $\mathcal {V}$
necessarily exists. By the choice of $\mathcal {V}$![]() and the induction hypothesis, for any $i\in \{1, \ldots, l^v-1\}$
and the induction hypothesis, for any $i\in \{1, \ldots, l^v-1\}$![]() we may further find $n^v_i \in \mathbb {N}$
we may further find $n^v_i \in \mathbb {N}$![]() , $k-1\geq n^v_i > n$
, $k-1\geq n^v_i > n$![]() , such that $v^v_i\in 2^{-n^v_i}\mathbb {Z} \setminus 2^{-n^v_i+1}\mathbb {Z}$
, such that $v^v_i\in 2^{-n^v_i}\mathbb {Z} \setminus 2^{-n^v_i+1}\mathbb {Z}$![]() .
.
Given $i\in \{1, \ldots, l^v-1\}$![]() , let us denote $\nu ^v_i = 1/2\sum _{j\in \{1, \ldots, l^{v^+}\},\,v^{v^+}_j=v^v_i} \nu ^{v^+}_j +1/ 2\sum _{j\in \{1, \ldots, l^{v^-}\}, \,v^{v^-}_j=v^v_i} \nu ^{v^-}_j$
, let us denote $\nu ^v_i = 1/2\sum _{j\in \{1, \ldots, l^{v^+}\},\,v^{v^+}_j=v^v_i} \nu ^{v^+}_j +1/ 2\sum _{j\in \{1, \ldots, l^{v^-}\}, \,v^{v^-}_j=v^v_i} \nu ^{v^-}_j$![]() . It follows from the induction hypothesis that there is at most one index $j\in \{1, \ldots, l^{v^+}\}$
. It follows from the induction hypothesis that there is at most one index $j\in \{1, \ldots, l^{v^+}\}$![]() such that $v^{v^+}_j=v^v_i$
such that $v^{v^+}_j=v^v_i$![]() (similarly for $v^-$
(similarly for $v^-$![]() ). Hence, we obtain $0<\nu ^v_i \leq 2^{\alpha (n-n^v_i)}$
). Hence, we obtain $0<\nu ^v_i \leq 2^{\alpha (n-n^v_i)}$![]() .
.
We set $n^v_{l^v}=k$![]() , $v^v_{l^v}=v$
, $v^v_{l^v}=v$![]() , $\nu ^v_{l^v}=2^{(n-k)\alpha }$
, $\nu ^v_{l^v}=2^{(n-k)\alpha }$![]() , and $\mu ^v_1=1/2 (\mu ^{v^+}_1+\mu ^{v^-}_1)$
, and $\mu ^v_1=1/2 (\mu ^{v^+}_1+\mu ^{v^-}_1)$![]() , $\mu ^v_2=1/2 (\mu ^{v^+}_2+\mu ^{v^-}_2)$
, $\mu ^v_2=1/2 (\mu ^{v^+}_2+\mu ^{v^-}_2)$![]() . Let us remark that $\mu ^v_1, \mu ^v_2 \geq 0$
. Let us remark that $\mu ^v_1, \mu ^v_2 \geq 0$![]() and $\mu ^v_1+\mu ^v_2=1/2 (\mu ^{v^+}_1+\mu ^{v^-}_1)+1/2 (\mu ^{v^+}_2+\mu ^{v^-}_2)= 1$
and $\mu ^v_1+\mu ^v_2=1/2 (\mu ^{v^+}_1+\mu ^{v^-}_1)+1/2 (\mu ^{v^+}_2+\mu ^{v^-}_2)= 1$![]() since $\mu ^{v^+}_1+\mu ^{v^+}_2 = \mu ^{v^-}_1+\mu ^{v^-}_2=1$
since $\mu ^{v^+}_1+\mu ^{v^+}_2 = \mu ^{v^-}_1+\mu ^{v^-}_2=1$![]() , by the induction hypothesis.
, by the induction hypothesis.
Continuing (4.1), let us rewrite the above coefficients

We note that for any $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$![]() and for the elements $\mu ^v_1$
and for the elements $\mu ^v_1$![]() , $\mu ^v_2$
, $\mu ^v_2$![]() , $l^v$
, $l^v$![]() , $n^v_i$
, $n^v_i$![]() , $v^v_i$
, $v^v_i$![]() and $\nu ^v_i$
and $\nu ^v_i$![]() , where $i \in \{1, \ldots, l^v\}$
, where $i \in \{1, \ldots, l^v\}$![]() , the (i) to (vi) now follow at once if $v\in 2^{-k+1}\mathbb {Z}$
, the (i) to (vi) now follow at once if $v\in 2^{-k+1}\mathbb {Z}$![]() by the induction hypothesis and were otherwise established during the construction whenever $v\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$
by the induction hypothesis and were otherwise established during the construction whenever $v\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$![]() .
.
To establish (vii), we first remark the conclusion is satisfied for any $v\in 2^{-k+1}\mathbb {Z}$![]() by the construction and the induction hypothesis. Let us pick $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$
by the construction and the induction hypothesis. Let us pick $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$![]() .
.
Since we have $v^-,\,v^+ \in 2^{-k+1}\mathbb {Z}$![]() , $|v^+-v^-|=2^{-k+1}$
, $|v^+-v^-|=2^{-k+1}$![]() , it follows that $\{v^-, v^+\} \cap 2^{-k+2}\mathbb {Z} \neq \emptyset$
, it follows that $\{v^-, v^+\} \cap 2^{-k+2}\mathbb {Z} \neq \emptyset$![]() . Hence, there exist $u$
. Hence, there exist $u$![]() , $u^\prime$
, $u^\prime$![]() such that $u \in \{v^-, v^+\} \cap 2^{-k+1}\mathbb {Z} \setminus 2^{-k+2}\mathbb {Z}$
such that $u \in \{v^-, v^+\} \cap 2^{-k+1}\mathbb {Z} \setminus 2^{-k+2}\mathbb {Z}$![]() , $u^\prime \in \{v^-, v^+\} \cap 2^{-k+2}\mathbb {Z}$
, $u^\prime \in \{v^-, v^+\} \cap 2^{-k+2}\mathbb {Z}$![]() . By the induction hypothesis and (vi) for $u$
. By the induction hypothesis and (vi) for $u$![]() and $u^\prime$
and $u^\prime$![]() , we get that $\{ v^{u^\prime }_i : i\in \{1, \ldots, l^{u^\prime }\}\} \subseteq \{ v^{u}_i : i\in \{1, \ldots, l^{u}\}\}$
, we get that $\{ v^{u^\prime }_i : i\in \{1, \ldots, l^{u^\prime }\}\} \subseteq \{ v^{u}_i : i\in \{1, \ldots, l^{u}\}\}$![]() . Since $\{ v^{v}_i : i\in \{1, \ldots, l^{v}\}\} \cap 2^{-k+1}\mathbb {Z} = \{ v^{v^+}_i : i\in \{1, \ldots, l^{v^+}\}\} \cup \{ v^{v^-}_i : i\in \{1, \ldots, l^{v^-}\}\}$
. Since $\{ v^{v}_i : i\in \{1, \ldots, l^{v}\}\} \cap 2^{-k+1}\mathbb {Z} = \{ v^{v^+}_i : i\in \{1, \ldots, l^{v^+}\}\} \cup \{ v^{v^-}_i : i\in \{1, \ldots, l^{v^-}\}\}$![]() by the construction, we deduce that
by the construction, we deduce that
The conclusion of (vii) now follows from the induction hypothesis for $u$![]() whenever $m \in \mathbb {N}$
whenever $m \in \mathbb {N}$![]() , $k-1\geq m>n$
, $k-1\geq m>n$![]() . Additionally, since $\{v^{v}_i : i\in \{1, \ldots, l^{v}\}\} \setminus 2^{-k+1}\mathbb {Z} = \{v\}$
. Additionally, since $\{v^{v}_i : i\in \{1, \ldots, l^{v}\}\} \setminus 2^{-k+1}\mathbb {Z} = \{v\}$![]() , (vii) is satisfied. The proof of the induction step is now complete.
, (vii) is satisfied. The proof of the induction step is now complete.
Pick $k\in \mathbb {N}$![]() , $k\geq n$
, $k\geq n$![]() and $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$
and $v\in [u_1, u_2] \cap 2^{-k}\mathbb {Z}$![]() . We note that $n^v_i > n$
. We note that $n^v_i > n$![]() , where $i \in \{ 1, \ldots, l^v\}$
, where $i \in \{ 1, \ldots, l^v\}$![]() , by (i), and $|\{ i \in \{ 1, \ldots, l^v\} : n^v_i=j \}|\leq 1$
, by (i), and $|\{ i \in \{ 1, \ldots, l^v\} : n^v_i=j \}|\leq 1$![]() , where $j\in \mathbb {N}$
, where $j\in \mathbb {N}$![]() , $j>n$
, $j>n$![]() , by (ii), (iii) and (vii). Likewise, (v) shows that $0<\nu ^v_i\leq 2^{(n-n^v_i)\alpha }$
, by (ii), (iii) and (vii). Likewise, (v) shows that $0<\nu ^v_i\leq 2^{(n-n^v_i)\alpha }$![]() for every $i \in \{1, \ldots, l^v\}$
for every $i \in \{1, \ldots, l^v\}$![]() . Hence,
. Hence,

The claim is established.
Lemma 4.4 Let $d\in \mathbb {N}$![]() and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() be such that for any $v\in V_k$
be such that for any $v\in V_k$![]() , where $k\in \mathbb {N}_0$
, where $k\in \mathbb {N}_0$![]() , we have $2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u)) \in \mathcal {M}$
, we have $2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u)) \in \mathcal {M}$![]() .
.
There exists a constant $\rho ^\prime >0$![]() for which the following holds true. Consider $v=(v_i)_{i=1}^d\in V$
for which the following holds true. Consider $v=(v_i)_{i=1}^d\in V$![]() and $i\in \{1, \ldots, d\}$
and $i\in \{1, \ldots, d\}$![]() . If $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$
. If $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$![]() for some $n\in \mathbb {N}$
for some $n\in \mathbb {N}$![]() , then $2^{n\alpha }( \delta (v)-1/2 (\delta (v^i_{2^{-n}})+\delta (v^i_{-2^{-n}}))) \in \rho ^\prime \operatorname {aconv}_p \mathcal {M}$
, then $2^{n\alpha }( \delta (v)-1/2 (\delta (v^i_{2^{-n}})+\delta (v^i_{-2^{-n}}))) \in \rho ^\prime \operatorname {aconv}_p \mathcal {M}$![]() . Quantitatively, if we set $\rho = ( C^p(p, 2) + (1+2^{1-p})2^{-p\alpha }(1/{1-2^{-p\alpha }}))^{1/p}$
. Quantitatively, if we set $\rho = ( C^p(p, 2) + (1+2^{1-p})2^{-p\alpha }(1/{1-2^{-p\alpha }}))^{1/p}$![]() , then $\rho '=\rho ^d$
, then $\rho '=\rho ^d$![]() .
.
Proof. We prove that for any $l\in \{0, \ldots, d-1\}$![]() , the following claim is true. Let $v=(v_i)_{i=1}^d\in V$
, the following claim is true. Let $v=(v_i)_{i=1}^d\in V$![]() . If $i\in \{1, \ldots, d\}$
. If $i\in \{1, \ldots, d\}$![]() is such that $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$
is such that $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$![]() for some $n\in \mathbb {N}$
for some $n\in \mathbb {N}$![]() and if $|\{ j\in \{1, \ldots, d\} : v_j \notin 2^{-n}\mathbb {Z}\}|\leq l$
and if $|\{ j\in \{1, \ldots, d\} : v_j \notin 2^{-n}\mathbb {Z}\}|\leq l$![]() , then $2^{n\alpha }( \delta (v)-1/2 (\delta (v^i_{2^{-n}})+\delta (v^i_{-2^{-n}}))) \in \rho ^{l+1} \operatorname {aconv}_p \mathcal {M}$
, then $2^{n\alpha }( \delta (v)-1/2 (\delta (v^i_{2^{-n}})+\delta (v^i_{-2^{-n}}))) \in \rho ^{l+1} \operatorname {aconv}_p \mathcal {M}$![]() . We proceed by induction on $l$
. We proceed by induction on $l$![]() .
.
Let $l=0$![]() . We pick $v=(v_i)_{i=1}^d\in V$
. We pick $v=(v_i)_{i=1}^d\in V$![]() , $n\in \mathbb {N}$
, $n\in \mathbb {N}$![]() and $i\in \{1, \ldots, d\}$
and $i\in \{1, \ldots, d\}$![]() as above. Recall that for any $u=(u_i)_{i=1}^d \in V_{n-1}$
as above. Recall that for any $u=(u_i)_{i=1}^d \in V_{n-1}$![]() , it follows from lemma 2.13 (iv) that $\Lambda ^d_{2^{-n+1}}(u, v)=\Lambda ^{d-1}_{2^{-n+1}}(\pi _d(u), \pi _d(v))\Lambda ^1_{2^{-n+1}}(u_i, v_i)$
, it follows from lemma 2.13 (iv) that $\Lambda ^d_{2^{-n+1}}(u, v)=\Lambda ^{d-1}_{2^{-n+1}}(\pi _d(u), \pi _d(v))\Lambda ^1_{2^{-n+1}}(u_i, v_i)$![]() . Hence, $\Lambda ^d_{2^{-n+1}}(u, v)=0$
. Hence, $\Lambda ^d_{2^{-n+1}}(u, v)=0$![]() whenever $u_i\notin \{v_i \pm 2^{-n}\}$
whenever $u_i\notin \{v_i \pm 2^{-n}\}$![]() , and now
, and now

Repeating the same argument, we verify

Altogether, we have

Note that by the assumption, $2^{n\alpha }(\delta (v^\prime )-\sum _{u \in V_{n-1}} \Lambda ^d_{2^{-n+1}}(u, v^\prime )\delta (u))$![]() for each $v^\prime \in \{v, v^i_{\pm 2^{-n}}\} \subseteq V_n$
for each $v^\prime \in \{v, v^i_{\pm 2^{-n}}\} \subseteq V_n$![]() . The intermediate claim now follows as
. The intermediate claim now follows as

Let $l\in \{1, \ldots, d-1\}$![]() be such that the claim holds for $l-1$
be such that the claim holds for $l-1$![]() . Pick $v=(v_i)_{i=1}^d\in V$
. Pick $v=(v_i)_{i=1}^d\in V$![]() , $n\in \mathbb {N}$
, $n\in \mathbb {N}$![]() , and $i\in \{1, \ldots, d\}$
, and $i\in \{1, \ldots, d\}$![]() such that $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$
such that $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$![]() . If $\{ j\in \{1, \ldots, d\} : v_j \notin 2^{-n}\mathbb {Z}\}$
. If $\{ j\in \{1, \ldots, d\} : v_j \notin 2^{-n}\mathbb {Z}\}$![]() is empty, the conclusion follows from the induction hypothesis. We may thus further assume there is some $j\in \{1, \ldots, d\}$
is empty, the conclusion follows from the induction hypothesis. We may thus further assume there is some $j\in \{1, \ldots, d\}$![]() for which $v_j \notin 2^{-n}\mathbb {Z}$
for which $v_j \notin 2^{-n}\mathbb {Z}$![]() .
.
We consider $u_1, u_2 \in [0, 1]\cap 2^{-n}\mathbb {Z}$![]() such that $u_1< v_j< u_2$
such that $u_1< v_j< u_2$![]() and $|u_1-u_2|=2^{-n}$
and $|u_1-u_2|=2^{-n}$![]() . Let us further denote $v' = (v_1, \ldots, v_{j-1}, u_1, v_{j+1}, \ldots, v_d)$
. Let us further denote $v' = (v_1, \ldots, v_{j-1}, u_1, v_{j+1}, \ldots, v_d)$![]() , $v' = (v_1, \ldots, v_{j-1}, u_2, v_{j+1}, \ldots, v_d)$
, $v' = (v_1, \ldots, v_{j-1}, u_2, v_{j+1}, \ldots, v_d)$![]() . Observe that $i$
. Observe that $i$![]() , $n$
, $n$![]() and $v', \, v''\in V$
and $v', \, v''\in V$![]() , respectively, satisfy the induction hypothesis for $l-1$
, respectively, satisfy the induction hypothesis for $l-1$![]() . Hence,
. Hence,

If $j>i$![]() , we set $m=i$
, we set $m=i$![]() . Otherwise, we set $m=i-1$
. Otherwise, we set $m=i-1$![]() . We define $x=(v_1, \ldots, v_{j-1}, v_{j+1}, \ldots, v_d)$
. We define $x=(v_1, \ldots, v_{j-1}, v_{j+1}, \ldots, v_d)$![]() and $x_1=x^m_{2^{-n}}$
and $x_1=x^m_{2^{-n}}$![]() , $x_2=x^m_{-2^{-n}}$
, $x_2=x^m_{-2^{-n}}$![]() . Let also $\mu _1, \mu _2 \in \mathbb {R}$
. Let also $\mu _1, \mu _2 \in \mathbb {R}$![]() , $l \in \mathbb {N}_0$
, $l \in \mathbb {N}_0$![]() , $\nu _r \in \mathbb {R}$
, $\nu _r \in \mathbb {R}$![]() , $n_r \in \mathbb {N}$
, $n_r \in \mathbb {N}$![]() , $n_r>n$
, $n_r>n$![]() , and $w_r \in 2^{-n_r}\mathbb {Z} \setminus 2^{-n_r+1}\mathbb {Z}$
, and $w_r \in 2^{-n_r}\mathbb {Z} \setminus 2^{-n_r+1}\mathbb {Z}$![]() , where $r \in \{1, \ldots, l\}$
, where $r \in \{1, \ldots, l\}$![]() , be the coefficients from lemma 4.3 associated with $u_1$
, be the coefficients from lemma 4.3 associated with $u_1$![]() , $u_2$
, $u_2$![]() and $v_j$
and $v_j$![]() .
.
It follows that

and

Pick $r\in \{1, \ldots, l\}$![]() and recall that $w_r \in 2^{-n_r}\mathbb {Z}\setminus 2^{-n}\mathbb {Z}$
and recall that $w_r \in 2^{-n_r}\mathbb {Z}\setminus 2^{-n}\mathbb {Z}$![]() . If $y=(y_i)_{i=1}^{d-1}\in \{x, x_1, x_2\}$
. If $y=(y_i)_{i=1}^{d-1}\in \{x, x_1, x_2\}$![]() , we set $y^\prime = (y^\prime _i)_{i=1}^{d}=(y_1, \ldots, y_{j-1}, w_r, y_{j+1}, \ldots, y_d)$
, we set $y^\prime = (y^\prime _i)_{i=1}^{d}=(y_1, \ldots, y_{j-1}, w_r, y_{j+1}, \ldots, y_d)$![]() ; it follows that $|\{ k\in \{1, \ldots, d\} : y^\prime _k \notin 2^{-n_r}\mathbb {Z}\}| \leq l-1$
; it follows that $|\{ k\in \{1, \ldots, d\} : y^\prime _k \notin 2^{-n_r}\mathbb {Z}\}| \leq l-1$![]() . By the induction hypothesis,
. By the induction hypothesis,
Using the estimate from lemma 4.3, we obtain for any $y\in \{x, x_1, x_2\}$![]()

We note that $\delta ^j_x(v_j) = \delta (v)$![]() . Similarly,
. Similarly,

We may now rewrite

and

Substituting the last two terms, let us rewrite

Appealing to (4.2) and (4.3), we conclude

This verifies the induction step, and thus completes the proof.
4.1.2 One geometrical consideration
We develop a result for the specific case of $d=1$![]() . This result implies the existence $\rho \in \mathbb {R}$
. This result implies the existence $\rho \in \mathbb {R}$![]() such that for any $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$
such that for any $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() with $n\in \mathbb {N}_0$
with $n\in \mathbb {N}_0$![]() , the relation ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in \rho \operatorname {aconv}_p \mathcal \iota (\{ e_x : x\in V\})$
, the relation ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in \rho \operatorname {aconv}_p \mathcal \iota (\{ e_x : x\in V\})$![]() holds.
holds.
First, we establish the conclusion under the assumption that $|u-v|=2^{-n}$![]() .
.
Lemma 4.5 Let $d\in \mathbb {N}$![]() , $i\in \{1, \ldots, d\}$
, $i\in \{1, \ldots, d\}$![]() , $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$
, $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$![]() and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() . Assume that $\delta ^i_x (0), \delta ^i_x (1) \in \mathcal {M}$
. Assume that $\delta ^i_x (0), \delta ^i_x (1) \in \mathcal {M}$![]() and for any $y\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$
and for any $y\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$![]() , where $k\in \mathbb {N}$
, where $k\in \mathbb {N}$![]() , it holds that $2^{\alpha k}(\delta ^i_x (y) - 1/2 (\delta ^i_x (y^-)+\delta ^i_x (y^+))) \in \mathcal {M}$
, it holds that $2^{\alpha k}(\delta ^i_x (y) - 1/2 (\delta ^i_x (y^-)+\delta ^i_x (y^+))) \in \mathcal {M}$![]() .
.
Whenever $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() and $|u-v|=2^{-n}$
and $|u-v|=2^{-n}$![]() for some $n\in \mathbb {N}_0$
for some $n\in \mathbb {N}_0$![]() , we have ${\delta ^i_x(u)-\delta ^i_x(v)}/{|u-v|^\alpha } \in 2^{1/p}(1/{1-2^{p(\alpha -1)}})^{1/p}\operatorname {aconv}_p \mathcal {M}$
, we have ${\delta ^i_x(u)-\delta ^i_x(v)}/{|u-v|^\alpha } \in 2^{1/p}(1/{1-2^{p(\alpha -1)}})^{1/p}\operatorname {aconv}_p \mathcal {M}$![]() .
.
Proof. Given $n \in \mathbb {N}_0$![]() , let us denote
, let us denote
We claim that for any $y \in \mathcal {M}_n$![]() , where $n\in \mathbb {N}_0$
, where $n\in \mathbb {N}_0$![]() , there exist coefficients $\mu ^y_j \in \{-1, 1\}$
, there exist coefficients $\mu ^y_j \in \{-1, 1\}$![]() , where $j\in \{0, \ldots, n\}$
, where $j\in \{0, \ldots, n\}$![]() , and elements $y^y_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$
, and elements $y^y_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$![]() , where $j\in \{1, \ldots, n\}$
, where $j\in \{1, \ldots, n\}$![]() , such that
, such that

We proceed by induction on $n$![]() . To that end, we note the conclusion is trivial in the case $n=0$
. To that end, we note the conclusion is trivial in the case $n=0$![]() .
.
Let $n\in \mathbb {N}$![]() be such that the claim holds for $n-1$
be such that the claim holds for $n-1$![]() , and pick $y = {\delta ^i_x(u)-\delta ^i_x(v)}/{|u-v|^\alpha }\in \mathcal {M}_n$
, and pick $y = {\delta ^i_x(u)-\delta ^i_x(v)}/{|u-v|^\alpha }\in \mathcal {M}_n$![]() for some $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$
for some $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() , $|u-v|=2^{-n}$
, $|u-v|=2^{-n}$![]() . As $\{u, v\} \cap 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z} \neq \emptyset$
. As $\{u, v\} \cap 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z} \neq \emptyset$![]() , there exists $w \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$
, there exists $w \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$![]() for which
for which
Similarly, there exist $\nu _1,\,\nu _2\in \{-1, 1\}$![]() such that
such that

Denote $z={\delta ^i_x(w^+)-\delta ^i_x(w^-)}/{|w^+-w^-|^\alpha }\in \mathcal {M}_{n-1}$![]() and let $\mu ^z_j \in \{-1, 1\}$
and let $\mu ^z_j \in \{-1, 1\}$![]() , where $j\in \{0, \ldots, n-1\}$
, where $j\in \{0, \ldots, n-1\}$![]() , and $y^z_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$
, and $y^z_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$![]() , where $j\in \{1, \ldots, n-1\}$
, where $j\in \{1, \ldots, n-1\}$![]() , be the coefficients from the inductive hypothesis. We may now define $\mu ^y_j=\nu _2\mu ^z_j$
, be the coefficients from the inductive hypothesis. We may now define $\mu ^y_j=\nu _2\mu ^z_j$![]() , where $j\in \{0, \ldots, n-1\}$
, where $j\in \{0, \ldots, n-1\}$![]() , and $y^y_j=y^z_j$
, and $y^y_j=y^z_j$![]() , where $j\in \{1, \ldots, n-1\}$
, where $j\in \{1, \ldots, n-1\}$![]() . Similarly, let $\mu ^y_n=\nu _1$
. Similarly, let $\mu ^y_n=\nu _1$![]() , $y^y_n=w$
, $y^y_n=w$![]() .
.
It follows from the construction that $\{\mu ^y_j : j\in \{0, \ldots, n\}\} \subseteq \{-1, 1\}$![]() and $y^y_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$
and $y^y_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$![]() for any $j\in \{1, \ldots, n\}$
for any $j\in \{1, \ldots, n\}$![]() . By (4.5) we easily verify
. By (4.5) we easily verify

As $y\in \mathcal {M}_n$![]() was arbitrary, this concludes the induction step.
was arbitrary, this concludes the induction step.
Let $y\in \mathcal {M}_n$![]() , where $n\in \mathbb {N}_0$
, where $n\in \mathbb {N}_0$![]() . We pick $\mu ^y_j \in \{-1, 1\}$
. We pick $\mu ^y_j \in \{-1, 1\}$![]() , where $j\in \{0, \ldots, n\}$
, where $j\in \{0, \ldots, n\}$![]() , and $y^y_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$
, and $y^y_j \in 2^{-j}\mathbb {Z} \setminus 2^{-j+1}\mathbb {Z}$![]() , where $j\in \{1, \ldots, n\}$
, where $j\in \{1, \ldots, n\}$![]() , as found in the previous part. Recall that by the assumption, $2^{j\alpha }(\delta ^i_x(y^y_j) - 1/2 (\delta ^i_x((y^y_j)^+)+\delta ^i_x((y^y_j)^-))) \in \mathcal {M}$
, as found in the previous part. Recall that by the assumption, $2^{j\alpha }(\delta ^i_x(y^y_j) - 1/2 (\delta ^i_x((y^y_j)^+)+\delta ^i_x((y^y_j)^-))) \in \mathcal {M}$![]() for any $j\in \{1, \ldots, n\}$
for any $j\in \{1, \ldots, n\}$![]() and $\delta ^i_x (0), \delta ^i_x (1) \in \mathcal {M}$
and $\delta ^i_x (0), \delta ^i_x (1) \in \mathcal {M}$![]() . Combining this with (4.4), we deduce $y\in ( 2\cdot 2^{p(\alpha -1)n}+\sum _{j=1}^n 2^{p(n-j)(\alpha -1)})^{1/p}\operatorname {aconv}_p \mathcal {M}$
. Combining this with (4.4), we deduce $y\in ( 2\cdot 2^{p(\alpha -1)n}+\sum _{j=1}^n 2^{p(n-j)(\alpha -1)})^{1/p}\operatorname {aconv}_p \mathcal {M}$![]() . Hence,
. Hence,

The claim follows.
We decompose a general element ${\delta (u)-\delta (v)}/{|u-v|^\alpha }$![]() , where $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$
, where $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() for some $n\in \mathbb {N}_0$
for some $n\in \mathbb {N}_0$![]() , into a combination of elements which we considered above.
, into a combination of elements which we considered above.
The following technical result is a refinement of [Reference Weaver18, Lemma 8.40].
Lemma 4.6 Let $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() for some $n\in \mathbb {N}_0$
for some $n\in \mathbb {N}_0$![]() , $u\neq v$
, $u\neq v$![]() . There exist $l\in \mathbb {N}$
. There exist $l\in \mathbb {N}$![]() and $a_i \in [u, v] \cap 2^{-n} \mathbb {Z}$
and $a_i \in [u, v] \cap 2^{-n} \mathbb {Z}$![]() , where $i\in \{1, \ldots, l\}$
, where $i\in \{1, \ldots, l\}$![]() , such that
, such that
(i) $a_1=u$
 , $a_l=v$
, $a_l=v$ ,
,(ii) $a_i, a_{i+1} \in 2^{-k}\mathbb {Z}$
 and $|a_i-a_{i+1}|=2^{-k}$
and $|a_i-a_{i+1}|=2^{-k}$ for some $k \in \{0, \ldots, n\}$
for some $k \in \{0, \ldots, n\}$ , for any $i\in \{1, \ldots, l-1\}$
, for any $i\in \{1, \ldots, l-1\}$ ,
,(iii) $( \sum _{i=1}^{l-1} |a_{i+1}-a_i|^{p\alpha } )^{1/p} < 2^{1/p}(1/{1-2^{-p\alpha }})^{1/p}|u-v|^\alpha$
 .
.
Proof. Without loss of generality, let $u< v$![]() .
.
We choose the smallest $n_0 \in \mathbb {N}_0 \cup \{-1\}$![]() such that $[u, v] \cap 2^{-n_0}\mathbb {Z} \neq \emptyset$
such that $[u, v] \cap 2^{-n_0}\mathbb {Z} \neq \emptyset$![]() . Subsequently, we define the sets $\mathcal {V}_i$
. Subsequently, we define the sets $\mathcal {V}_i$![]() (where $i\in \{n_0, \ldots, n\}$
(where $i\in \{n_0, \ldots, n\}$![]() ) to be subsets of $2^{-i} \mathbb {Z}$
) to be subsets of $2^{-i} \mathbb {Z}$![]() as follows. Set $\mathcal {V}_{n_0} = 2^{-n_0}\mathbb {Z} \cap [u, v]$
as follows. Set $\mathcal {V}_{n_0} = 2^{-n_0}\mathbb {Z} \cap [u, v]$![]() . If $i\in \{n_0+1, \ldots, n\}$
. If $i\in \{n_0+1, \ldots, n\}$![]() and $\mathcal {V}_{j}$
and $\mathcal {V}_{j}$![]() was defined for all $j\in \{n_0, \ldots, i-1\}$
was defined for all $j\in \{n_0, \ldots, i-1\}$![]() , we take $\mathcal {V}_i = [u, v] \cap 2^{-i}\mathbb {Z} \setminus [\min \cup _{j\in \{n_0, \ldots, i-1\}}\mathcal {V}_{j}, \max \cup _{j\in \{n_0, \ldots, i-1\}}\mathcal {V}_{j}]$
, we take $\mathcal {V}_i = [u, v] \cap 2^{-i}\mathbb {Z} \setminus [\min \cup _{j\in \{n_0, \ldots, i-1\}}\mathcal {V}_{j}, \max \cup _{j\in \{n_0, \ldots, i-1\}}\mathcal {V}_{j}]$![]() .
.
We remark that $|\mathcal {V}_{n_0}|=1$![]() by the choice of $n_0$
by the choice of $n_0$![]() and, moreover, $[u, v] \cap 2^{-i}\mathbb {Z} \subseteq [\min \cup _{j\in \{n_0, \ldots, i\}}\mathcal {V}_{j}, \max \cup _{j\in \{n_0, \ldots, i\}}\mathcal {V}_{j}]$
and, moreover, $[u, v] \cap 2^{-i}\mathbb {Z} \subseteq [\min \cup _{j\in \{n_0, \ldots, i\}}\mathcal {V}_{j}, \max \cup _{j\in \{n_0, \ldots, i\}}\mathcal {V}_{j}]$![]() , where $i\in \{n_0, \ldots, n\}$
, where $i\in \{n_0, \ldots, n\}$![]() , by the construction.
, by the construction.
Pick $i\in \{n_0+1, \ldots, n\}$![]() . It follows from the above remark that $\mathcal {V}_i \cap 2^{-i+1}\mathbb {Z} = \emptyset$
. It follows from the above remark that $\mathcal {V}_i \cap 2^{-i+1}\mathbb {Z} = \emptyset$![]() . Since $\cup _{j\in \{n_0, \ldots, i-1\}}\mathcal {V}_{j} \subseteq 2^{-i+1}\mathbb {Z}$
. Since $\cup _{j\in \{n_0, \ldots, i-1\}}\mathcal {V}_{j} \subseteq 2^{-i+1}\mathbb {Z}$![]() , this establishes the inclusion
, this establishes the inclusion
Denote $\mathcal {V} = \cup _{i\in \{n_0, \ldots, n\}} \mathcal {V}_i$![]() . It follows that $\mathcal {V}$
. It follows that $\mathcal {V}$![]() is finite and $\{u, v\} \subseteq \mathcal {V}$
is finite and $\{u, v\} \subseteq \mathcal {V}$![]() since $\{u, v\} \subseteq 2^{-n}\mathbb {Z}$
since $\{u, v\} \subseteq 2^{-n}\mathbb {Z}$![]() . Hence, we may define $l=|\mathcal {V}|$
. Hence, we may define $l=|\mathcal {V}|$![]() and find a strictly monotone arrangement $a_i$
and find a strictly monotone arrangement $a_i$![]() , where $i\in \{1, \ldots, l\}$
, where $i\in \{1, \ldots, l\}$![]() , of the set $\mathcal {V}$
, of the set $\mathcal {V}$![]() such that $a_1=u$
such that $a_1=u$![]() , $a_l=v$
, $a_l=v$![]() . It remains to show that $l$
. It remains to show that $l$![]() and $a_i$
and $a_i$![]() , where $i\in \{1, \ldots, l\}$
, where $i\in \{1, \ldots, l\}$![]() , satisfy (ii) and (iii).
, satisfy (ii) and (iii).
To show (ii), pick $i\in \{1, \ldots, l-1\}$![]() . By (4.6), there is $k\in \{n_0+1, \ldots, n\}$
. By (4.6), there is $k\in \{n_0+1, \ldots, n\}$![]() for which $a_i=\min \cup _{j\in \{n_0, \ldots, k-1\}}\mathcal {V}_{j} - 2^{-k}$
for which $a_i=\min \cup _{j\in \{n_0, \ldots, k-1\}}\mathcal {V}_{j} - 2^{-k}$![]() , $a_{i+1}=\min \cup _{j\in \{n_0, \ldots, k-1\}} \mathcal {V}_j$
, $a_{i+1}=\min \cup _{j\in \{n_0, \ldots, k-1\}} \mathcal {V}_j$![]() or $a_i=\max \cup _{j\in \{n_0, \ldots, k-1\}}\mathcal {V}_{j}$
or $a_i=\max \cup _{j\in \{n_0, \ldots, k-1\}}\mathcal {V}_{j}$![]() , $a_{i+1}=\min \cup _{j\in \{n_0, \ldots, k-1\}}\mathcal {V}_{j} + 2^{-k}$
, $a_{i+1}=\min \cup _{j\in \{n_0, \ldots, k-1\}}\mathcal {V}_{j} + 2^{-k}$![]() . It follows that $a_i,\,a_{i+1}\in 2^{-k}\mathbb {Z}$
. It follows that $a_i,\,a_{i+1}\in 2^{-k}\mathbb {Z}$![]() and $|a_i-a_{i+1}|=2^{-k}$
and $|a_i-a_{i+1}|=2^{-k}$![]() .
.
The above argument also shows that the set $\{i\in \{1, \ldots, l-1\}: |a_i-a_{i+1}|=2^{-k}\}$![]() is empty for any $k\in \mathbb {Z}$
is empty for any $k\in \mathbb {Z}$![]() such that $k\leq n_0$
such that $k\leq n_0$![]() or $n< k$
or $n< k$![]() and it has at most two elements if $n_0< k\leq n$
and it has at most two elements if $n_0< k\leq n$![]() . We consider the least $k_0\in \mathbb {Z}$
. We consider the least $k_0\in \mathbb {Z}$![]() for which there exists $i\in \{1, \ldots, l-1\}$
for which there exists $i\in \{1, \ldots, l-1\}$![]() with $|a_i-a_{i+1}|= 2^{-k_0}$
with $|a_i-a_{i+1}|= 2^{-k_0}$![]() . Since $|u-v|\geq 2^{-k_0}$
. Since $|u-v|\geq 2^{-k_0}$![]() , we have
, we have

which verifies (iii). The proof is now complete.
Lemma 4.7 Let $d\in \mathbb {N}$![]() , $i\in \{1, \ldots, d\}$
, $i\in \{1, \ldots, d\}$![]() , $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$
, $x=(x_j)_{j=1}^{d-1}\in [0, 1]^{d-1}$![]() and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() be such that the conclusion of lemma 4.5 holds true, i.e. whenever $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$
be such that the conclusion of lemma 4.5 holds true, i.e. whenever $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() and $|u-v|=2^{-n}$
and $|u-v|=2^{-n}$![]() for some $n\in \mathbb {N}_0$
for some $n\in \mathbb {N}_0$![]() , then ${\delta ^i_x(u)-\delta ^i_x(v)}/{|u-v|^\alpha } \in 2^{1/p}(1/{1-2^{p(\alpha -1)}})^{1/p}\operatorname {aconv}_p \mathcal {M}$
, then ${\delta ^i_x(u)-\delta ^i_x(v)}/{|u-v|^\alpha } \in 2^{1/p}(1/{1-2^{p(\alpha -1)}})^{1/p}\operatorname {aconv}_p \mathcal {M}$![]() .
.
Whenever $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() for some $n\in \mathbb {N}_0$
for some $n\in \mathbb {N}_0$![]() , where $u\neq v$
, where $u\neq v$![]() , then ${\delta ^i_x(u)-\delta ^i_x(v)}/ {|u-v|^\alpha } \in 2^{2/p}(1/{1-2^{p(\alpha -1)}})^{1/p}(1/ {1-2^{-p\alpha }})^{1/p}\operatorname {aconv}_p \mathcal {M}$
, then ${\delta ^i_x(u)-\delta ^i_x(v)}/ {|u-v|^\alpha } \in 2^{2/p}(1/{1-2^{p(\alpha -1)}})^{1/p}(1/ {1-2^{-p\alpha }})^{1/p}\operatorname {aconv}_p \mathcal {M}$![]() .
.
Proof. Given $u,\,v \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() , where $n\in \mathbb {N}$
, where $n\in \mathbb {N}$![]() , $u\neq v$
, $u\neq v$![]() , we pick the coefficients $l$
, we pick the coefficients $l$![]() and $a_j$
and $a_j$![]() , where $j\in \{1,\,\ldots, l\}$
, where $j\in \{1,\,\ldots, l\}$![]() , from lemma 4.6 associated with $v$
, from lemma 4.6 associated with $v$![]() , $u$
, $u$![]() and $n$
and $n$![]() .
.
By (i) and (iii) of lemma 4.6, respectively, we have

By the assumption on $\mathcal {M}$![]() and (ii), we obtain
and (ii), we obtain

Hence, we get ${\delta ^i_x(u)-\delta ^i_x(v)}/{|u-v|^\alpha } \in 2^{2/p}(1/{1-2^{p(\alpha -1)}})^{1/p}(1/ {1-2^{-p\alpha }})^{1/p} \operatorname {aconv}_p \mathcal {M}$![]() . The proof is complete.
. The proof is complete.
Drawing on the conclusion of lemma 4.4, we generalize the previous result by induction on the dimension of boundary cubes.
Lemma 4.8 Let $d\in \mathbb {N}$![]() and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
and $\mathcal {M} \subseteq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() be such that $\{ \delta (u) : u\in \{0, 1\}^d \} \subseteq \mathcal {M}$
be such that $\{ \delta (u) : u\in \{0, 1\}^d \} \subseteq \mathcal {M}$![]() and the conclusion of lemma 4.4 holds true, i.e. there exists $\rho ^\prime >0$
and the conclusion of lemma 4.4 holds true, i.e. there exists $\rho ^\prime >0$![]() such that for any $v=(v_i)_{i=1}^d\in V$
such that for any $v=(v_i)_{i=1}^d\in V$![]() and $i\in \{1, \ldots, d\}$
and $i\in \{1, \ldots, d\}$![]() , if $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$
, if $v_i \in 2^{-n}\mathbb {Z} \setminus 2^{-n+1}\mathbb {Z}$![]() for some $n\in \mathbb {N}$
for some $n\in \mathbb {N}$![]() , then $2^{n\alpha }( \delta (v)-1/2 (\delta (v^i_{2^{-n}})+\delta (v^i_{-2^{-n}}))) \in \rho ^\prime \operatorname {aconv}_p \mathcal {M}$
, then $2^{n\alpha }( \delta (v)-1/2 (\delta (v^i_{2^{-n}})+\delta (v^i_{-2^{-n}}))) \in \rho ^\prime \operatorname {aconv}_p \mathcal {M}$![]() .
.
There exists $\tau ^\prime >0$![]() such that for any $u,\,v \in V \cup \{ 0 \}$
such that for any $u,\,v \in V \cup \{ 0 \}$![]() , $u\neq v$
, $u\neq v$![]() , we have ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in \tau ^\prime \operatorname {aconv}_p \mathcal {M}$
, we have ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in \tau ^\prime \operatorname {aconv}_p \mathcal {M}$![]() . Quantitatively, if we denote $\tau =C^\alpha (p\alpha, d) 2^{2/p} \cdot (1/ {1-2^{p(\alpha -1)}})^{1/p} (1/{1-2^{-p\alpha }})^{1/p} \cdot (1+(d-1)^{p\alpha })^{1/p}$
. Quantitatively, if we denote $\tau =C^\alpha (p\alpha, d) 2^{2/p} \cdot (1/ {1-2^{p(\alpha -1)}})^{1/p} (1/{1-2^{-p\alpha }})^{1/p} \cdot (1+(d-1)^{p\alpha })^{1/p}$![]() , then $\tau ' = \tau ^d \rho '$
, then $\tau ' = \tau ^d \rho '$![]() .
.
Proof. We show inductively that for any $l\in \{1, \ldots, d\}$![]() , the following claim holds true. Let $u=(u_i)_{i=1}^d,\,v=(v_i)_{i=1}^d\in [0, 1]^d \cap 2^{-n}\mathbb {Z}^d$
, the following claim holds true. Let $u=(u_i)_{i=1}^d,\,v=(v_i)_{i=1}^d\in [0, 1]^d \cap 2^{-n}\mathbb {Z}^d$![]() , where $n\in \mathbb {N}_0$
, where $n\in \mathbb {N}_0$![]() , $u\neq v$
, $u\neq v$![]() and $\mathcal {I} \subseteq \{1, \ldots, d\}$
and $\mathcal {I} \subseteq \{1, \ldots, d\}$![]() , $|\mathcal {I}|=d-l$
, $|\mathcal {I}|=d-l$![]() , be such that $u_i=v_i\in \{0, 1\}$
, be such that $u_i=v_i\in \{0, 1\}$![]() for any $i\in \mathcal {I}$
for any $i\in \mathcal {I}$![]() . Then ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in \tau ^l\rho '\operatorname {aconv}_p \mathcal {M}$
. Then ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in \tau ^l\rho '\operatorname {aconv}_p \mathcal {M}$![]() .
.
Let us note that by assuming $u_i=v_i\in \{0, 1\}$![]() for any $i\in \mathcal {I}$
for any $i\in \mathcal {I}$![]() with $|\mathcal {I}|=d-l$
with $|\mathcal {I}|=d-l$![]() , we can iteratively develop the result over the $l$
, we can iteratively develop the result over the $l$![]() -faces of $[0, 1]^d$
-faces of $[0, 1]^d$![]() .
.
Let $l=1$![]() and pick $u=(u_i)_{i=1}^d, v=(v_i)_{i=1}^d\in [0, 1]^d\cap 2^{-n}\mathbb {Z}^d$
and pick $u=(u_i)_{i=1}^d, v=(v_i)_{i=1}^d\in [0, 1]^d\cap 2^{-n}\mathbb {Z}^d$![]() , where $n\in \mathbb {N}_0$
, where $n\in \mathbb {N}_0$![]() , $u\neq v$
, $u\neq v$![]() , and $i\in \{1, \ldots, d\}$
, and $i\in \{1, \ldots, d\}$![]() , such that $u_j=v_j\in \{0, 1\}$
, such that $u_j=v_j\in \{0, 1\}$![]() for any $j\in \{1, \ldots, d\} \setminus \{i\}$
for any $j\in \{1, \ldots, d\} \setminus \{i\}$![]() . We may find $x\in \{0, 1\}^{d-1}$
. We may find $x\in \{0, 1\}^{d-1}$![]() and $u^\prime, \, v^\prime \in [0, 1]\cap 2^{-n}\mathbb {Z}$
and $u^\prime, \, v^\prime \in [0, 1]\cap 2^{-n}\mathbb {Z}$![]() such that $\delta (u)=\delta ^i_x (u^\prime )$
such that $\delta (u)=\delta ^i_x (u^\prime )$![]() , $\delta (v)=\delta ^i_x (v^\prime )$
, $\delta (v)=\delta ^i_x (v^\prime )$![]() .
.
Note that by the assumption, $\delta ^i_x (0), \delta ^i_x (1) \in \mathcal {M}$![]() , and whenever $q\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$
, and whenever $q\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$![]() for some $k\in \mathbb {N}$
for some $k\in \mathbb {N}$![]() , we have $2^{\alpha k}(\delta ^i_x (q) - 1/2 (\delta ^i_x (q^-)+\delta ^i_x (q^+))) \in \rho '\operatorname {aconv}_p\mathcal {M}$
, we have $2^{\alpha k}(\delta ^i_x (q) - 1/2 (\delta ^i_x (q^-)+\delta ^i_x (q^+))) \in \rho '\operatorname {aconv}_p\mathcal {M}$![]() . It follows that $d$
. It follows that $d$![]() , $i$
, $i$![]() , $x$
, $x$![]() and $\rho '\operatorname {aconv}_p\mathcal {M}$
and $\rho '\operatorname {aconv}_p\mathcal {M}$![]() satisfy the assumption of lemma 4.5.
satisfy the assumption of lemma 4.5.
Consequently, by lemma 4.7 for $u^\prime, v^\prime \in [0, 1] \cap 2^{-n}\mathbb {Z}$![]() , $u\neq v$
, $u\neq v$![]() , we obtain
, we obtain
which establishes the first step.
Let $l\in \{2, \ldots, d\}$![]() be such that the claim holds for $l-1$
be such that the claim holds for $l-1$![]() . We pick $u=(u_i)_{i=1}^d, v=(v_i)_{i=1}^d\in [0, 1]^d \cap 2^{-n}\mathbb {Z}^d$
. We pick $u=(u_i)_{i=1}^d, v=(v_i)_{i=1}^d\in [0, 1]^d \cap 2^{-n}\mathbb {Z}^d$![]() , where $n\in \mathbb {N}_0$
, where $n\in \mathbb {N}_0$![]() , $u\neq v$
, $u\neq v$![]() , and $\mathcal {I}\subseteq \{1, \ldots, d\}$
, and $\mathcal {I}\subseteq \{1, \ldots, d\}$![]() , $|\mathcal {I}|=d-l$
, $|\mathcal {I}|=d-l$![]() , such that $u_i=v_i\in \{0, 1\}$
, such that $u_i=v_i\in \{0, 1\}$![]() for any $i\in \mathcal {I}$
for any $i\in \mathcal {I}$![]() . Assume first there exists $i\in \{1, \ldots, d\}\setminus \mathcal {I}$
. Assume first there exists $i\in \{1, \ldots, d\}\setminus \mathcal {I}$![]() such that $u_j=v_j$
such that $u_j=v_j$![]() for any $j\in \{1, \ldots, d\} \setminus \{i\}$
for any $j\in \{1, \ldots, d\} \setminus \{i\}$![]() . We shall prove that ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in 2^{2/p}(1/{1-2^{p(\alpha -1)}})^{1/p}(1/{1-2^{-p\alpha }})^{1/p}(1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$
. We shall prove that ${\delta (u)-\delta (v)}/{|u-v|^\alpha } \in 2^{2/p}(1/{1-2^{p(\alpha -1)}})^{1/p}(1/{1-2^{-p\alpha }})^{1/p}(1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$![]() .
.
Let $y=(y_j)_{j=1}^d, \, z=(z_j)_{j=1}^d\in [0, 1]^d \cap 2^{-n}\mathbb {Z}^d$![]() be such that $y_i=0$
be such that $y_i=0$![]() , $z_i=1$
, $z_i=1$![]() and $y_j=z_j=u_j$
and $y_j=z_j=u_j$![]() for any $j\in \{1, \ldots, d\}\setminus \{i\}$
for any $j\in \{1, \ldots, d\}\setminus \{i\}$![]() . We further pick $y^\prime =(y^\prime _j)_{j=1}^d, \, z^\prime =(z^\prime _j)_{j=1}^d \in \{0, 1\}^d$
. We further pick $y^\prime =(y^\prime _j)_{j=1}^d, \, z^\prime =(z^\prime _j)_{j=1}^d \in \{0, 1\}^d$![]() satisfying $y^\prime _j=y_j$
satisfying $y^\prime _j=y_j$![]() and $z^\prime _j=z_j$
and $z^\prime _j=z_j$![]() for any $j\in \mathcal {I} \cup \{i\}$
for any $j\in \mathcal {I} \cup \{i\}$![]() .
.
If $y\neq y^\prime$![]() , we rewrite $\delta (y) = |y-y'|^\alpha {\delta (y)-\delta (y^\prime )}/{|y-y^\prime |^\alpha } + \delta (y')$
, we rewrite $\delta (y) = |y-y'|^\alpha {\delta (y)-\delta (y^\prime )}/{|y-y^\prime |^\alpha } + \delta (y')$![]() , where, in particular, ${\delta (y)-\delta (y^\prime )}/{|y-y^\prime |^\alpha } \in \tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$
, where, in particular, ${\delta (y)-\delta (y^\prime )}/{|y-y^\prime |^\alpha } \in \tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$![]() by the induction hypothesis. Since $\delta (y) = \delta (y')$
by the induction hypothesis. Since $\delta (y) = \delta (y')$![]() in the remaining case and $\delta (y^\prime ) \in \mathcal {M}$
in the remaining case and $\delta (y^\prime ) \in \mathcal {M}$![]() by the assumption on $\mathcal {M}$
by the assumption on $\mathcal {M}$![]() , we deduce that $\delta (y) \in (1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$
, we deduce that $\delta (y) \in (1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$![]() . Repeating the same argument for $z$
. Repeating the same argument for $z$![]() , we conclude $\delta (y),\,\delta (z) \in (1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$
, we conclude $\delta (y),\,\delta (z) \in (1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$![]() .
.
Let next $x\in [0,\,1]^{d-1}\cap 2^{-n}\mathbb {Z}^{d-1}$![]() and $u^\prime, \, v^\prime \in [0, 1]\cap 2^{-n}\mathbb {Z}$
and $u^\prime, \, v^\prime \in [0, 1]\cap 2^{-n}\mathbb {Z}$![]() , $u'\neq v'$
, $u'\neq v'$![]() , be such that $\delta (u)=\delta ^i_x (u^\prime )$
, be such that $\delta (u)=\delta ^i_x (u^\prime )$![]() , $\delta (v)=\delta ^i_x (v^\prime )$
, $\delta (v)=\delta ^i_x (v^\prime )$![]() . We observe that $\delta (y)=\delta ^i_x (0)$
. We observe that $\delta (y)=\delta ^i_x (0)$![]() and $\delta (z)=\delta ^i_x (1)$
and $\delta (z)=\delta ^i_x (1)$![]() hold. By the preceding paragraph, we have $\delta ^i_x (0), \delta ^i_x (1) \in (1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$
hold. By the preceding paragraph, we have $\delta ^i_x (0), \delta ^i_x (1) \in (1+(d-1)^{p\alpha })^{1/p}\tau ^{l-1}\rho '\operatorname {aconv}_p \mathcal {M}$![]() , and if $q\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$
, and if $q\in 2^{-k}\mathbb {Z} \setminus 2^{-k+1}\mathbb {Z}$![]() for some $k\in \mathbb {N}$
for some $k\in \mathbb {N}$![]() , then $2^{\alpha k}(\delta ^i_x (q) - 1/2 (\delta ^i_x (q^-)+\delta ^i_x (q^+))) \in \rho '\operatorname {aconv}_p \mathcal {M}$
, then $2^{\alpha k}(\delta ^i_x (q) - 1/2 (\delta ^i_x (q^-)+\delta ^i_x (q^+))) \in \rho '\operatorname {aconv}_p \mathcal {M}$![]() by the assumption on $\mathcal {M}$
by the assumption on $\mathcal {M}$![]() . By lemmas 4.5 and 4.7, we conclude
. By lemmas 4.5 and 4.7, we conclude

To establish the general case, we pick $u=(u_i)_{i=1}^d, \, v=(v_i)_{i=1}^d\in [0, 1]^d \cap 2^{-n}\mathbb {Z}^d$![]() , where $n\in \mathbb {N}_0$
, where $n\in \mathbb {N}_0$![]() , $u\neq v$
, $u\neq v$![]() , and $\mathcal {I}\subseteq \{1, \ldots, d\}$
, and $\mathcal {I}\subseteq \{1, \ldots, d\}$![]() , $|\mathcal {I}|=d-l$
, $|\mathcal {I}|=d-l$![]() , are such that $u_i=v_i\in \{0, 1\}$
, are such that $u_i=v_i\in \{0, 1\}$![]() for any $i\in \mathcal {I}$
for any $i\in \mathcal {I}$![]() . If $u$
. If $u$![]() , $v$
, $v$![]() differ at exactly $k$
differ at exactly $k$![]() coordinates, $k\geq 1$
coordinates, $k\geq 1$![]() , we set $u^1=v$
, we set $u^1=v$![]() , $u^{k+1}=u$
, $u^{k+1}=u$![]() and find $u^i$
and find $u^i$![]() , where $i\in \{2, \ldots, k\}$
, where $i\in \{2, \ldots, k\}$![]() , such that $u^i$
, such that $u^i$![]() , $u^{i+1}$
, $u^{i+1}$![]() differ at one coordinate for any $i\in \{1, \ldots, k\}$
differ at one coordinate for any $i\in \{1, \ldots, k\}$![]() . Let us remark that all $u^i$
. Let us remark that all $u^i$![]() , where $i\in \{1, \ldots, k+1\}$
, where $i\in \{1, \ldots, k+1\}$![]() , mutually coincide at any coordinate from the set $\mathcal {I}$
, mutually coincide at any coordinate from the set $\mathcal {I}$![]() .
.
It follows that $\sum _{i=1}^{k} |u^{i+1}-u^i| = |u-v|$![]() and
and

where, by the already proven that, we have for any $i\in \{1, \ldots, k\}$![]() ,
,

Therefore, we may write

This concludes the claim.
By now we have collected all the necessary results to establish the isomorphism theorem.
Proof. Proof of theorem 4.1
Let $\iota : \{ e_v \}_{v\in V} \subset \ell _p (V) \to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() be defined as $e_v \mapsto 2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u))$
be defined as $e_v \mapsto 2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u))$![]() for $v\in V_k\setminus V_{k-1}$
for $v\in V_k\setminus V_{k-1}$![]() , where $k\in \mathbb {N}_0$
, where $k\in \mathbb {N}_0$![]() .
.
It follows easily that $\{ e_v : v\in V \}$![]() is isometrically $p$
is isometrically $p$![]() -norming in $\ell _p (V)$
-norming in $\ell _p (V)$![]() and that $\operatorname {aconv}_p \{ e_v : v\in V \}$
and that $\operatorname {aconv}_p \{ e_v : v\in V \}$![]() contains a neighbourhood of zero in $\operatorname {span} \{ e_v : v\in V \}$
contains a neighbourhood of zero in $\operatorname {span} \{ e_v : v\in V \}$![]() . Moreover, we claim that $\iota$
. Moreover, we claim that $\iota$![]() extends to a one-to-one linear map from $\operatorname {span} \{ e_v : v\in V\}$
extends to a one-to-one linear map from $\operatorname {span} \{ e_v : v\in V\}$![]() into $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
into $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() . Indeed, an easy direct argument is possible or it suffices to note that $\delta _{\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )}(x) \mapsto \delta _{\mathcal {F}_p ([0, 1]^d)} (x)$
. Indeed, an easy direct argument is possible or it suffices to note that $\delta _{\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )}(x) \mapsto \delta _{\mathcal {F}_p ([0, 1]^d)} (x)$![]() , where $x\in [0, 1]^d$
, where $x\in [0, 1]^d$![]() , induces an onto linear bijection $\kappa : \operatorname {span}\{ \delta _{\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )}(x) : x \in [0, 1]^d\} \to \operatorname {span}\{ \delta _{\mathcal {F}_p ([0, 1]^d)}(x) : x \in [0, 1]^d\}$
, induces an onto linear bijection $\kappa : \operatorname {span}\{ \delta _{\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )}(x) : x \in [0, 1]^d\} \to \operatorname {span}\{ \delta _{\mathcal {F}_p ([0, 1]^d)}(x) : x \in [0, 1]^d\}$![]() and $\kappa (\iota (e_v))$
and $\kappa (\iota (e_v))$![]() , where $v\in V$
, where $v\in V$![]() , form a Schauder basis in $\mathcal {F}_p ([0, 1]^d)$
, form a Schauder basis in $\mathcal {F}_p ([0, 1]^d)$![]() , see [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8].
, see [Reference Albiac, Ansorena, Cúth and Doucha4, Theorem 3.8].
Once we show that $\iota (\{e_v : v\in V\})$![]() is $p$
is $p$![]() -norming in $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
-norming in $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() and that $\operatorname {aconv}_p \iota (\{e_v : v\in V\})$
and that $\operatorname {aconv}_p \iota (\{e_v : v\in V\})$![]() contains a neighbourhood of zero in $\operatorname {span} \iota (\{e_v : v\in V\})$
contains a neighbourhood of zero in $\operatorname {span} \iota (\{e_v : v\in V\})$![]() , it will follow from lemma 2.4 that $\iota$
, it will follow from lemma 2.4 that $\iota$![]() extends to an onto isomorphism $\tilde \iota : \ell _p(V)\to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
extends to an onto isomorphism $\tilde \iota : \ell _p(V)\to \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() . To that end, let us first establish the inclusion $\beta \iota (\{e_v : v\in V\})\subseteq B_{\mathcal {F}_p}$
. To that end, let us first establish the inclusion $\beta \iota (\{e_v : v\in V\})\subseteq B_{\mathcal {F}_p}$![]() for some $\beta > 0$
for some $\beta > 0$![]() .
.
For any $v \in V_0\setminus V_{-1}$![]() , we deduce
, we deduce
where $\left \Vert {\delta (v)}/{|v|^\alpha } \right \Vert _p = 1$![]() as $\delta$
as $\delta$![]() is an isometry.
is an isometry.
Let $v =(v_i)_{i=1}^d\in V_k\setminus V_{k-1}$![]() , where $k\in \mathbb {N}$
, where $k\in \mathbb {N}$![]() . We first note that
. We first note that

where the third equality follows as $\left \Vert {\delta (v)-\delta (u)}/{|v-u|^\alpha } \right \Vert _p = 1$![]() for any $u\in V_{k-1}$
for any $u\in V_{k-1}$![]() , by isometry of $\delta$
, by isometry of $\delta$![]() . We further remark that for any $u=(u_i)_{i=1}^d\in V_{k-1}$
. We further remark that for any $u=(u_i)_{i=1}^d\in V_{k-1}$![]() , $\Lambda ^d_{2^{-k+1}}(u, v)\neq 0$
, $\Lambda ^d_{2^{-k+1}}(u, v)\neq 0$![]() , it follows from lemma 2.13 (iv) that $|v_i-u_i|\in \{0, 2^{-k}\}$
, it follows from lemma 2.13 (iv) that $|v_i-u_i|\in \{0, 2^{-k}\}$![]() , where $i\in \{ 1, \ldots, d\}$
, where $i\in \{ 1, \ldots, d\}$![]() ; hence, $2^{k\alpha }|v-u|^\alpha \leq d^{\alpha }$
; hence, $2^{k\alpha }|v-u|^\alpha \leq d^{\alpha }$![]() . Similarly, we recall that $\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v) =1$
. Similarly, we recall that $\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v) =1$![]() by lemma 2.13 (iii) and $\Lambda ^d_{2^{-k+1}}(\cdot, v)\geq 0$
by lemma 2.13 (iii) and $\Lambda ^d_{2^{-k+1}}(\cdot, v)\geq 0$![]() by definition. Continuing (4.7), we obtain
by definition. Continuing (4.7), we obtain

It follows that $\iota (\{e_v : v\in V\})\subseteq d^\alpha C(p, 2^d) B_{\mathcal {F}_p}$![]() .
.
We verify that $\operatorname {Mol} (V \cup \{ 0 \}) \subseteq \alpha \operatorname {aconv}_p\,\iota (\{e_v : v\in V\})$![]() for some $\alpha > 0$
for some $\alpha > 0$![]() .
.
To that end, let $v\in V_k$![]() , where $k\in \mathbb {N}_0$
, where $k\in \mathbb {N}_0$![]() . Whenever $v \in V_k \setminus V_{k-1}$
. Whenever $v \in V_k \setminus V_{k-1}$![]() , we have that $2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u)) \in \iota (\{e_{v'} :v' \in V\})$
, we have that $2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u)) \in \iota (\{e_{v'} :v' \in V\})$![]() . If $v \in V_{k-1}$
. If $v \in V_{k-1}$![]() , we recall that $\Lambda ^d_{2^{-k+1}} (u, v) = \delta _{u, v}$
, we recall that $\Lambda ^d_{2^{-k+1}} (u, v) = \delta _{u, v}$![]() for any $u \in V_{k-1}$
for any $u \in V_{k-1}$![]() by lemma 2.13 (ii); hence, $2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u))=0$
by lemma 2.13 (ii); hence, $2^{k\alpha }(\delta (v)-\sum _{u \in V_{k-1}} \Lambda ^d_{2^{-k+1}}(u, v)\delta (u))=0$![]() . We deduce that
. We deduce that

Since now $\operatorname {aconv}_p \iota (\{e_v :v\in V\})$![]() satisfies the assumption of lemma 4.4 and, in particular, we have that $\{ \delta (u) : u\in \{0, 1\}^d \} = \{ \iota (e_v) : v\in V_0\setminus V_{-1} \} \cup \{ 0 \} \subseteq \operatorname {aconv}_p \iota (\{e_v : v\in V\})$
satisfies the assumption of lemma 4.4 and, in particular, we have that $\{ \delta (u) : u\in \{0, 1\}^d \} = \{ \iota (e_v) : v\in V_0\setminus V_{-1} \} \cup \{ 0 \} \subseteq \operatorname {aconv}_p \iota (\{e_v : v\in V\})$![]() , by lemma 4.8 there exists $\alpha > 0$
, by lemma 4.8 there exists $\alpha > 0$![]() such that
such that
Quantitatively, if $\rho$![]() and $\tau$
and $\tau$![]() are as in lemmas 4.4 and 4.8, respectively, we may set $\alpha = \rho ^d\tau ^d$
are as in lemmas 4.4 and 4.8, respectively, we may set $\alpha = \rho ^d\tau ^d$![]() .
.
Note that $\operatorname {Mol} (V\cup \{ 0 \})$![]() is a dense subset of $\operatorname {Mol} ([0,\,1]^d)$
is a dense subset of $\operatorname {Mol} ([0,\,1]^d)$![]() , which is isometrically $p$
, which is isometrically $p$![]() -norming by fact 2.9. Hence, we get from (4.8) that $B_{\mathcal {F}_p} \subseteq \alpha \,\overline{\operatorname {aconv}}_p \iota (\{e_v : v\in V\})$
-norming by fact 2.9. Hence, we get from (4.8) that $B_{\mathcal {F}_p} \subseteq \alpha \,\overline{\operatorname {aconv}}_p \iota (\{e_v : v\in V\})$![]() . Since also $\iota (\{e_v : v\in V\}) \subseteq d^\alpha C(p, 2^d) B_{\mathcal {F}_p}$
. Since also $\iota (\{e_v : v\in V\}) \subseteq d^\alpha C(p, 2^d) B_{\mathcal {F}_p}$![]() by the already proven part, we conclude that $\iota (\{e_v : v\in V\})$
by the already proven part, we conclude that $\iota (\{e_v : v\in V\})$![]() is $p$
is $p$![]() -norming in $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$
-norming in $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() .
.
Moreover, lemma 2.10 in conjunction with (4.8) shows that $\operatorname {aconv}_p \iota (\{e_v : v\in V\})\supseteq 1/\alpha \cdot \operatorname {Mol} (V\cup \{ 0 \})$![]() contains a neighbourhood of zero in $\operatorname {span} \iota (\{e_v : v\in V\}) = \mathcal {P} (V\cup \{ 0 \})$
contains a neighbourhood of zero in $\operatorname {span} \iota (\{e_v : v\in V\}) = \mathcal {P} (V\cup \{ 0 \})$![]() .
.
Altogether, we have verified that the assumptions of lemma 2.4 are satisfied; hence, $\iota$![]() extends to an onto isomorphism $\tilde \iota$
extends to an onto isomorphism $\tilde \iota$![]() .
.
Quantitatively, we have shown that
and thus the Banach–Mazur distance of $\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() and $\ell _p (V)$
and $\ell _p (V)$![]() may be estimated as $d(\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ), \ell _p (V)) \leq \lVert \tilde \iota \rVert \lVert {\tilde \iota }^{-1}\rVert \leq C(p, 2^d)\rho ^d\tau ^d$
may be estimated as $d(\mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha ), \ell _p (V)) \leq \lVert \tilde \iota \rVert \lVert {\tilde \iota }^{-1}\rVert \leq C(p, 2^d)\rho ^d\tau ^d$![]() .
.
The proof is complete.
4.2 The isomorphism $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha )$ , where $(\mathcal {M}, \rho )$
, where $(\mathcal {M}, \rho )$ is infinite doubling
is infinite doubling
We generalize the isomorphism theorem to snowflakes of infinite doubling metric spaces. The following argument was suggested in [Reference Albiac, Ansorena, Cúth and Doucha3].
Theorem 4.9 Let $(\mathcal {M}, \rho )$![]() be an infinite doubling metric space and $0 < \alpha < 1$
be an infinite doubling metric space and $0 < \alpha < 1$![]() , $0< p\leq 1$
, $0< p\leq 1$![]() . Then $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha )$
. Then $\mathcal {F}_p (\mathcal {M}, \rho ^\alpha )$![]() is isomorphic to the space $\ell _p$
is isomorphic to the space $\ell _p$![]() .
.
Proof. Let $\beta \in (\alpha, 1)$![]() . By the Assouad embedding theorem, see [Reference Assouad5, Proposition 2.6], it follows that $(\mathcal {M}, \rho ^{\alpha / \beta })$
. By the Assouad embedding theorem, see [Reference Assouad5, Proposition 2.6], it follows that $(\mathcal {M}, \rho ^{\alpha / \beta })$![]() is bi-Lipschitz equivalent to $(\mathcal {M}', |\cdot |)$
is bi-Lipschitz equivalent to $(\mathcal {M}', |\cdot |)$![]() for some $\mathcal {M}' \subseteq \mathbb {R}^d$
for some $\mathcal {M}' \subseteq \mathbb {R}^d$![]() , where $d\in \mathbb {N}$
, where $d\in \mathbb {N}$![]() . As a consequence, $(\mathcal {M}, \rho ^{\alpha })$
. As a consequence, $(\mathcal {M}, \rho ^{\alpha })$![]() is bi-Lipschitz equivalent to $(\mathcal {M}', |\cdot |^\beta )$
is bi-Lipschitz equivalent to $(\mathcal {M}', |\cdot |^\beta )$![]() . Hence, for the rest of the proof we may assume that $\mathcal {M}$
. Hence, for the rest of the proof we may assume that $\mathcal {M}$![]() is an infinite subset of $\mathbb {R}^d$
is an infinite subset of $\mathbb {R}^d$![]() .
.
We note that $\mathcal {F}_p (\mathbb {R}^d, |\cdot |^\alpha )$![]() contains a complemented subspace isomorphic to $\mathcal {F}_p (\mathcal {M}, |\cdot |^\alpha )$
contains a complemented subspace isomorphic to $\mathcal {F}_p (\mathcal {M}, |\cdot |^\alpha )$![]() , which is infinite-dimensional since $\mathcal {M}$
, which is infinite-dimensional since $\mathcal {M}$![]() is infinite. In fact, it is true that for any $\mathcal {M} \subseteq \mathcal {N} \subseteq \mathbb {R}^d$
is infinite. In fact, it is true that for any $\mathcal {M} \subseteq \mathcal {N} \subseteq \mathbb {R}^d$![]() , there exists a bounded operator $T:\mathcal {F}_p (\mathcal {N})\to \mathcal {F}_p (\mathcal {M})$
, there exists a bounded operator $T:\mathcal {F}_p (\mathcal {N})\to \mathcal {F}_p (\mathcal {M})$![]() satisfying $T\circ L_i= \operatorname {Id}_{\mathcal {F}_p (\mathcal {M})}$
satisfying $T\circ L_i= \operatorname {Id}_{\mathcal {F}_p (\mathcal {M})}$![]() , where $L_i$
, where $L_i$![]() is the canonical linearization of the inclusion map $i:\mathcal {M} \to \mathcal {N}$
is the canonical linearization of the inclusion map $i:\mathcal {M} \to \mathcal {N}$![]() , i.e. $\mathcal {M}$
, i.e. $\mathcal {M}$![]() is complementably $p$
is complementably $p$![]() -amenable in $\mathcal {N}$
-amenable in $\mathcal {N}$![]() , see [Reference Albiac, Ansorena, Cúth and Doucha3, Theorem 5.1].
, see [Reference Albiac, Ansorena, Cúth and Doucha3, Theorem 5.1].
Moreover, it is known that $\mathcal {F}_p (\mathbb {R}^d, |\cdot |^\alpha ) \simeq \mathcal {F}_p ([0, 1]^d, |\cdot |^\alpha )$![]() , see [Reference Albiac, Ansorena, Cúth and Doucha3, Theorem 4.15]. In light of Theorem 4.1, it follows that $\mathcal {F}_p (\mathbb {R}^d, |\cdot |^\alpha ) \simeq \ell _p$
, see [Reference Albiac, Ansorena, Cúth and Doucha3, Theorem 4.15]. In light of Theorem 4.1, it follows that $\mathcal {F}_p (\mathbb {R}^d, |\cdot |^\alpha ) \simeq \ell _p$![]() .
.
We deduce that $\mathcal {F}_p(\mathcal {M}, |\cdot |^\alpha )$![]() is isomorphic to a complemented infinite-dimensional subspace of $\ell _p$
is isomorphic to a complemented infinite-dimensional subspace of $\ell _p$![]() . Hence, it is isomorphic to $\ell _p$
. Hence, it is isomorphic to $\ell _p$![]() , see [Reference Pełczyński15, Theorem 1] and [Reference Stiles17, Theorem 2], and this finishes the proof.
, see [Reference Pełczyński15, Theorem 1] and [Reference Stiles17, Theorem 2], and this finishes the proof.
Acknowledgements
I wish to thank M. Cúth for his countless insights into my research efforts. I would also like to thank J. L. Ansorena for suggesting Counterexample 2.5. I acknowledge the support of GAČR 23-04776S and of the Charles University project GA UK No. 138123. The work was completed while the author was an employee at MSD Czech Republic, Prague, Czech Republic.