Article contents
COMPARISON OF WEAK AND STRONG MOMENTS FOR VECTORS WITH INDEPENDENT COORDINATES
Published online by Cambridge University Press: 14 February 2018
Abstract
We show that for  $p\geqslant 1$, the
$p\geqslant 1$, the 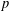 $p$th moment of suprema of linear combinations of independent centered random variables are comparable with the sum of the first moment and the weak
$p$th moment of suprema of linear combinations of independent centered random variables are comparable with the sum of the first moment and the weak 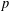 $p$th moment provided that
$p$th moment provided that 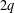 $2q$th and
$2q$th and 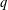 $q$th integral moments of these variables are comparable for all
$q$th integral moments of these variables are comparable for all  $q\geqslant 2$. The latest condition turns out to be necessary in the independent and identically distributed case.
$q\geqslant 2$. The latest condition turns out to be necessary in the independent and identically distributed case.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © University College London 2018
References
- 6
- Cited by




