Hostname: page-component-76fb5796d-2lccl Total loading time: 0 Render date: 2024-04-26T03:58:12.069Z Has data issue: false hasContentIssue false
  • English
  • Français

A simple stability criterion for a linear system of neutral integro-differential equations

Published online by Cambridge University Press:  24 October 2008

K. Gopalsamy
K. Gopalsamy
Affiliation:
The Flinders University of South Australia, Bedford Park, S.A. 5042, Australia
Get access Rights & Permissions [Opens in a new window]

Extract

The purpose of this article is to derive a set of ‘easily verifiable’ sufficient conditions for the asymptotic stability of the trivial solution of a class of linear neutral integro-delay-differential equations of the form

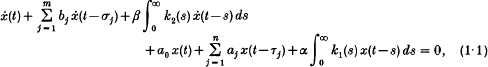
where ẋ denotes the right derivative, and then generalize the result to a vector system. Asymptotic stability of the trivial solution of the form (1·1) and several of its variants has been considered by many authors (see the discussion at the end). Also, there exists a well developed fundamental theory for neutral delay-differential equations (e.g. existence, uniqueness, continuous dependence etc. of solutions; see for instance the survey article by Akhmerov[1] and the references in it); however there exists no ‘easily verifiable’ sufficient condition for the asymptotic stability of the trivial solution of (1·1). By the term ‘easily verifiable’ we mean a verification which is as easy as in the case of Routh-Hurwitz criterion or the diagonal dominance condition of a matrix. A number of results which are valid for linear autonomous ordinary and delay-differential equations cannot be generalized (or extended) to neutral equations. It has been shown by Gromova and Zverkin [8] that a linear neutral differential equation can have unbounded solutions even though the related characteristic equation has only purely imaginary roots (see also Gromova[7], Datko[3], Snow [16], Brumley [2]); such behaviour is not possible in the case of ordinary or (non-neutral) delay-differential equations. It is known (theorem 6·1 of Henry [10]) that if the characteristic equation associated with a only with negative real parts and if all the roots are uniformly bounded away from the imaginary axis, then asymptotic stability of the trivial solution can be asserted.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 102 , Issue 1 , July 1987 , pp. 149 - 162
Copyright
Copyright © Cambridge Philosophical Society 1987

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REEFERENCES

[1] Akhmerov, R. R., Kamenskii, M. I., Potapos, A. S., Rodkina, A. E. and Sadovskii, B. N.. Theory of equations of neutral type. J. Soviet Math. 24 (1984), 674–719.CrossRefGoogle Scholar
[2] Brumley, W. E.. On the asymptotic behaviour of solutions of differential difference equations of neutral type. J. Differential Equations 7 (1970), 175–188.CrossRefGoogle Scholar
[3] Datko, R.. An example of an unstable neutral differential equation. Internal. J. Control. 38 (1983), 263–267.CrossRefGoogle Scholar
[4] Gopalsamy, K.. A simple stability criterion for linear neutral differential systems. Funkcial. Ekvac. 28 (1985), 33–38.Google Scholar
[5] Gopalsamy, K.. Nonoscillation in a delay-logistic equation. Quart. Appl. Math. XLIII (1985), 189–197.CrossRefGoogle Scholar
[6] Gopalsamy, K.. On the global attractivity in a generalised delay-logistic equation. Math. Proc. Cambridge Philos. Soc. 100 (1986), 183–192.CrossRefGoogle Scholar
[7] Gromova, P. S.. Stability of solutions of nonlinear equations of the neutral type in the asymptotically critical ease. Math. Notes. 1 (1967), 472–479.CrossRefGoogle Scholar
[8] Gromova, P. S. and Zverkin, A. M.. On trigonometric series whose sums are continuous unbounded functions on the real axis – solutions of equations with retarded arguments. (Russian.) Differentialniye Uravnenija 4 (1986), 1774–1784.Google Scholar
[9] Hale, J. K.. Theory of functional differential equations. Appl. Math. Sci. 3 (Springer-Verlag, 1977), 272–319.CrossRefGoogle Scholar
[10] Henry, D.. Linear autonomous neutral functional differential equations. J. Differential Equations 15 (1974), 106–128.CrossRefGoogle Scholar
[11] Kakutani, S. and Markus, L.. On the nonlinear differential difference equation y′(t) = [A−By(t−τ)]y(t). Contributions to the Theory of nonlinear oscillations, 4 (Princeton University Press, 1958), 1–18.Google Scholar
[12] Kolmanovskii, V. B. and Nosov, V. R.. Stability of neutral type functional differential equations. Nonlinear Anal. Theory, Methods and Appl. 6 (1982), 873–910.CrossRefGoogle Scholar
[13] Kolmanovskii, V. B. and Nosov, V. R.. Neutral type systems with after effects. Automat. Remote Control 45 (1984), 1–28.Google Scholar
[14] Kolmanovskii, V. B.. Stability of linear equations of neutral type. J. Differential Equations 6 (1970), 942–950.Google Scholar
[15] Levin, J. J. and Nohel, J.. On a nonlinear delay equation. J. Math. Anal. Appl. 8 (1964), 34–44.CrossRefGoogle Scholar
[16] Snow, W.. Existence, uniqueness and stability for nonlinear differential-difference equations in the neutral case. N.Y.U., Courant Inst. Math. Sci. Rep. IMM-NYU 328 (1965).Google Scholar
[17] Zverkin, A. M.. On the connection between boundedness and stability of solutions of linear systems with an infinite number of degrees of freedom. J. Differential Equations 4 (1968), 196–197.Google Scholar
[18] Zverkin, A. M.. Transaction of a seminar on the theory of differential equations with deviating arguments. Trudy Sem. Teor. Diff. Uravnenii s Otklon. Argumentom Univ. Družby Narodov. Patrisa Lumumby 1 (1962), 37–46.Google Scholar