Hostname: page-component-76fb5796d-45l2p Total loading time: 0 Render date: 2024-04-27T04:46:42.095Z Has data issue: false hasContentIssue false
  • English
  • Français

The Haagerup invariant for tensor products of operator spaces

Published online by Cambridge University Press:  24 October 2008

Allan M. Sinclair  and
Roger R. Smith
Allan M. Sinclair
Affiliation:
Department of Mathematics, University of Edinburgh, Edinburgh EH9 3JZ
Roger R. Smith
Affiliation:
Department of Mathematics, Texas A & M University, College Station, TX 77843, U.S.A
Get access Rights & Permissions [Opens in a new window]

Extract

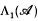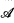In [7, 8] Haagerup introduced two isomorphism invariants and for C*-algebras and von Neumann algebras 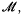, based on appropriate forms of the completely bounded approximation property defined below. These definitions have obvious extensions to operator spaces and dual operator spaces respectively, and in [16] we established the multiplicativity of A on the ultraweakly closed spatial tensor product of two dual operator spaces 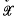and :

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 120 , Issue 1 , July 1996 , pp. 147 - 153
Copyright
Copyright © Cambridge Philosophical Society 1996

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bekka, M., Cowling, M. and Harpe, P. De La. Some groups whose reduced C*-algebra is simple. I.H.E.S. Publ. Math., to appear.Google Scholar
[2]Blecher, D. P. and Paulsen, V. I.. Tensor products of operator spaces. J. Fund. Anal. 99 (1991), 262–292.CrossRefGoogle Scholar
[3]Choi, M.-D. and Effros, E. G.. Injectivity and operator spaces. J. Fund. Anal. 24 (1977), 156–209.CrossRefGoogle Scholar
[4]Cowling, M. and Haagerup, U.. Completely bounded multipliers of the Fourier algebra of a simple Lie group of real rank one. Invent. Math. 96 (1989), 507–549.CrossRefGoogle Scholar
[5]Cannière, J. De and Haagerup, U.. Multipliers of the Fourier algebras of some simple Lie groups and their discrete subgroups. Amer. J. Math. 107 (1984), 455–500.CrossRefGoogle Scholar
[6]Effros, E. G. and Ruan, Z. J.. On approximation properties for operator spaces. Internal. J. Math. 1 (1990), 163–187.Google Scholar
[7]Haagerup, U.. An example of a non-nuclear C*-algebra which has the metric approximation property. Invent. Math. 50 (1979), 279–293.CrossRefGoogle Scholar
[8]Haagerup, U.. Group C*-algebras without the completely bounded approximation property, preprint.Google Scholar
[9]Haagerup, U. and Kraus, J.. Approximation properties for group C*-algebras and group von Neumann algebras. Trans. Amer. Math. Soc. 334 (1994), 667–699.Google Scholar
[10]Huruya, T.. On compact completely bounded maps of C*-algebras. Michigan Math. J. 30 (1983), 213–220.CrossRefGoogle Scholar
[11]Kirchberg, E.. On non-semisplit extensions, tensor products and exactness of group C*- algebras. Invent. Math. 112 (1993), 449–489.CrossRefGoogle Scholar
[12]Paulsen, V. I.. Completely bounded maps and dilations (Pitman, 1986).Google Scholar
[13]Paulsen, V. I. and Smith, R. R.. Multilinear maps and tensor norms on operator systems. J. Fund. Anal. 73 (1987), 258–276.CrossRefGoogle Scholar
[14]Pisier, G.. Remarks on complemented subspaces of von Neumann algebras. Proc. Roy. Soc. Edinburgh 121 A (1992), 1–4.CrossRefGoogle Scholar
[15]Ruan, Z. J.. Subspaces of C*-algebras. J. Fund. Anal. 76 (1988), 217–230.CrossRefGoogle Scholar
[16]Sinclair, A. M. and Smith, R. R.. The Haagerup invariant for von Neumann algebras. Amer. J. Math., to appear.Google Scholar
[17]Wittstock, G.. Eine operatorwertiger Hahn-Banach Satz. J. Fund. Anal. 40 (1981), 127–150.CrossRefGoogle Scholar