Article contents
RANDOMNESS VIA INFINITE COMPUTATION AND EFFECTIVE DESCRIPTIVE SET THEORY
Published online by Cambridge University Press: 01 August 2018
Abstract
We study randomness beyond 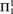 ${\rm{\Pi }}_1^1$-randomness and its Martin-Löf type variant, which was introduced in [16] and further studied in [3]. Here we focus on a class strictly between
${\rm{\Pi }}_1^1$-randomness and its Martin-Löf type variant, which was introduced in [16] and further studied in [3]. Here we focus on a class strictly between 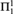 ${\rm{\Pi }}_1^1$ and
${\rm{\Pi }}_1^1$ and 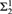 ${\rm{\Sigma }}_2^1$ that is given by the infinite time Turing machines (ITTMs) introduced by Hamkins and Kidder. The main results show that the randomness notions associated with this class have several desirable properties, which resemble those of classical random notions such as Martin-Löf randomness and randomness notions defined via effective descriptive set theory such as
${\rm{\Sigma }}_2^1$ that is given by the infinite time Turing machines (ITTMs) introduced by Hamkins and Kidder. The main results show that the randomness notions associated with this class have several desirable properties, which resemble those of classical random notions such as Martin-Löf randomness and randomness notions defined via effective descriptive set theory such as 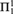 ${\rm{\Pi }}_1^1$-randomness. For instance, mutual randoms do not share information and a version of van Lambalgen’s theorem holds.
${\rm{\Pi }}_1^1$-randomness. For instance, mutual randoms do not share information and a version of van Lambalgen’s theorem holds.
Towards these results, we prove the following analogue to a theorem of Sacks. If a real is infinite time Turing computable relative to all reals in some given set of reals with positive Lebesgue measure, then it is already infinite time Turing computable. As a technical tool towards this result, we prove facts of independent interest about random forcing over increasing unions of admissible sets, which allow efficient proofs of some classical results about hyperarithmetic sets.
Keywords
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2018
References
REFERENCES
- 2
- Cited by




