Hostname: page-component-848d4c4894-tn8tq Total loading time: 0 Render date: 2024-07-02T05:29:14.407Z Has data issue: false hasContentIssue false
  • English
  • Français

Three-dimensional instabilities in oscillatory flow past elliptic cylinders

Published online by Cambridge University Press:  03 June 2016

José P. Gallardo ,
Helge I. Andersson  and
Bjørnar Pettersen
José P. Gallardo*
Affiliation:
Department of Marine Technology, Norwegian University of Science and Technology, NO-7491 Trondheim, Norway
Helge I. Andersson
Affiliation:
Department of Energy and Process Engineering, Norwegian University of Science and Technology, NO-7491 Trondheim, Norway
Bjørnar Pettersen
Affiliation:
Department of Marine Technology, Norwegian University of Science and Technology, NO-7491 Trondheim, Norway
*
†Email address for correspondence: jose.p.gallardo@ntnu.no
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the early development of instabilities in the oscillatory viscous flow past cylinders with elliptic cross-sections using three-dimensional direct numerical simulations. This is a classical hydrodynamic problem for circular cylinders, but other configurations have received only marginal attention. Computed results for some different aspect ratios 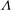${\it\Lambda}$ from 1 : 1 to 1 : 3, all with the major axis of the ellipse aligned in the main flow direction, show good qualitative agreement with Hall’s stability theory (J. Fluid Mech., vol. 146, 1984, pp. 347–367), which predicts a cusp-shaped curve for the onset of the primary instability. The three-dimensional flow structures for aspect ratios larger than 2 : 3 resemble those of a circular cylinder, whereas the elliptical cross-section with the lowest aspect ratio of 1 : 3 exhibits oblate rather than tubular three-dimensional flow structures as well as a pair of counter-rotating spanwise vortices which emerges near the tips of the ellipse. Contrary to a circular cylinder, instabilities for an elliptic cylinder with sufficiently high eccentricity emerge from four rather than two different locations in accordance with the Hall theory.

JFM classification

Wakes/Jets: Separated flows Vortex Flows: Vortex dynamics Vortex Flows: Vortex instability
Type
Papers
Information
Journal of Fluid Mechanics , Volume 798 , 10 July 2016 , pp. 371 - 397
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

An, H., Cheng, L. & Zhao, M. 2011 Direct numerical simulation of oscillatory flow around a circular cylinder at low Keulegan–Carpenter number. J. Fluid Mech. 666, 77–103.Google Scholar
Badr, H. M. & Kocabiyik, S. 1997 Symmetrically oscillating viscous flow over an elliptic cylinder. J. Fluids Struct. 11, 745–766.Google Scholar
Bearman, P. W., Downie, M. J., Graham, J. M. R. & Obasaju, E. D. 1985 Forces on cylinders in viscous oscillatory flow at low Keulegan–Carpenter numbers. J. Fluid Mech. 154, 337–356.Google Scholar
Elston, J. R., Blackburn, H. M. & Sheridan, J. 2006 The primary and secondary instabilities of flow generated by an oscillating circular cylinder. J. Fluid Mech. 550, 359–389.CrossRefGoogle Scholar
Hall, P. 1984 On the stability of the unsteady boundary layer on a cylinder oscillating transversely in a viscous fluid. J. Fluid Mech. 146, 347–367.Google Scholar
Honji, H. 1981 Streaked flow around an oscillating circular cylinder. J. Fluid Mech. 107, 509–520.CrossRefGoogle Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
Keulegan, G. H. & Carpenter, L. H. 1958 Forces on cylinders and plates in an oscillating fluid. J. Res. Natl Bur. Stand. 60, 423–440.CrossRefGoogle Scholar
Manhart, M. 2004 A zonal grid algorithm for DNS of turbulent boundary layers. Comput. Fluids 33, 435–461.Google Scholar
Manhart, M., Tremblay, F. & Friedrich, R. 2001 MGLET: a parallel code for efficient DNS and LES of complex geometries. In Parallel Computational Fluid Dynamics-Trends and Applications, pp. 449–456. Elsevier.Google Scholar
Morison, J. R., O’Brien, M. P., Johnson, J. W. & Schaaf, S. A. 1950 The force exerted by surface waves on piles. Petrol. Trans. AIME 189, 149–157.Google Scholar
Peller, N., Le Duc, A., Tremblay, F. & Manhart, M. 2006 High-order stable interpolations for immersed boundary methods. Intl J. Numer. Meth. Fluids 52, 1175–1193.CrossRefGoogle Scholar
Sarpkaya, T. 1986 Force on a circular cylinder in viscous oscillatory flow at low Keulegan–Carpenter numbers. J. Fluid Mech. 165, 61–71.CrossRefGoogle Scholar
Sarpkaya, T. 2002 Experiments on the stability of sinusoidal flow over a circular cylinder. J. Fluid Mech. 457, 157–180.CrossRefGoogle Scholar
Sarpkaya, T. 2005 On the parameter 𝛽 = Re/KC = D 2/𝜈T . J. Fluids Struct. 21, 435–440.CrossRefGoogle Scholar
Sarpkaya, T. 2006 Structures of separation on a circular cylinder in periodic flow. J. Fluid Mech. 567, 281–297.Google Scholar
Stone, H. L. 1968 Iterative solution of implicit approximations of multidimensional partial differential equations. SIAM J. Numer. Anal. 5, 530–558.Google Scholar
Suthon, P. & Dalton, C. 2011 Streakline visualization of the structures in the near wake of a circular cylinder in sinusoidally oscillating flow. J. Fluids Struct. 27, 885–902.CrossRefGoogle Scholar
Suthon, P. & Dalton, C. 2012 Observations on the Honji instability. J. Fluids Struct. 32, 27–36.CrossRefGoogle Scholar
Tatsuno, M. & Bearman, P. W. 1990 A visual study of the flow around an oscillating circular cylinder at low Keulegan–Carpenter numbers and low Stokes numbers. J. Fluid Mech. 211, 157–182.CrossRefGoogle Scholar
White, F. M. 2006 Viscous Fluid Flow, 3rd edn. McGraw-Hill.Google Scholar
Williamson, C. H. K. 1985 Sinusoidal flow relative to circular cylinders. J. Fluid Mech. 155, 141–174.Google Scholar
Williamson, J. H. 1980 Low-storage Runge–Kutta schemes. J. Comput. Phys. 56, 48–56.Google Scholar
Yang, K.2014 Oscillatory flow past cylinders at low $KC$ numbers. PhD thesis, The University of Western Australia.Google Scholar
Yang, K., Cheng, L., An, H., Bassom, A. P. & Zhao, M. 2014 Effects of an axial flow component on the Honji instability. J. Fluids Struct. 49, 614–639.CrossRefGoogle Scholar
Zhang, J. & Dalton, C. 1999 The onset of three-dimensionality in an oscillating flow past a fixed circular cylinder. Intl J. Numer. Meth. Fluids 42, 19–42.3.0.CO;2-#>CrossRefGoogle Scholar