Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bithi, Swastika S.
Nekouei, Mehdi
and
Vanapalli, Siva A.
2017.
Bistability in the hydrodynamic resistance of a drop trapped at a microcavity junction.
Microfluidics and Nanofluidics,
Vol. 21,
Issue. 11,
Reigh, Shang Yik
Zhu, Lailai
Gallaire, François
and
Lauga, Eric
2017.
Swimming with a cage: low-Reynolds-number locomotion inside a droplet.
Soft Matter,
Vol. 13,
Issue. 17,
p.
3161.
Zhu, Lailai
and
Gallaire, François
2017.
Bifurcation Dynamics of a Particle-Encapsulating Droplet in Shear Flow.
Physical Review Letters,
Vol. 119,
Issue. 6,
Chakraborty, I.
Ricouvier, J.
Yazhgur, P.
Tabeling, P.
and
Leshansky, A. M.
2017.
Microfluidic step-emulsification in axisymmetric geometry.
Lab Chip,
Vol. 17,
Issue. 21,
p.
3609.
Franco-Gómez, Andrés
Thompson, Alice B.
Hazel, Andrew L.
and
Juel, Anne
2017.
Bubble propagation on a rail: a concept for sorting bubbles by size.
Soft Matter,
Vol. 13,
Issue. 46,
p.
8684.
Keiser, Ludovic
Jaafar, Khalil
Bico, José
and
Reyssat, Étienne
2018.
Dynamics of non-wetting drops confined in a Hele-Shaw cell.
Journal of Fluid Mechanics,
Vol. 845,
Issue. ,
p.
245.
Reichert, Benjamin
Huerre, Axel
Theodoly, Olivier
Valignat, Marie-Pierre
Cantat, Isabelle
and
Jullien, Marie-Caroline
2018.
Topography of the lubrication film under a pancake droplet travelling in a Hele-Shaw cell.
Journal of Fluid Mechanics,
Vol. 850,
Issue. ,
p.
708.
Balestra, Gioele
Zhu, Lailai
and
Gallaire, François
2018.
Viscous Taylor droplets in axisymmetric and planar tubes: from Bretherton’s theory to empirical models.
Microfluidics and Nanofluidics,
Vol. 22,
Issue. 6,
Perazzo, Antonio
Tomaiuolo, Giovanna
Preziosi, Valentina
and
Guido, Stefano
2018.
Emulsions in porous media: From single droplet behavior to applications for oil recovery.
Advances in Colloid and Interface Science,
Vol. 256,
Issue. ,
p.
305.
Katsikis, Georgios
Breant, Alexandre
Rinberg, Anatoly
and
Prakash, Manu
2018.
Synchronous magnetic control of water droplets in bulk ferrofluid.
Soft Matter,
Vol. 14,
Issue. 5,
p.
681.
Keiser, Ludovic
Keiser, Armelle
L’Estimé, Manon
Bico, José
and
Reyssat, Étienne
2019.
Motion of Viscous Droplets in Rough Confinement: Paradoxical Lubrication.
Physical Review Letters,
Vol. 122,
Issue. 7,
Ge, Zhouyang
Tammisola, Outi
and
Brandt, Luca
2019.
Flow-assisted droplet assembly in a 3D microfluidic channel.
Soft Matter,
Vol. 15,
Issue. 16,
p.
3451.
Shukla, Isha
Kofman, Nicolas
Balestra, Gioele
Zhu, Lailai
and
Gallaire, François
2019.
Film thickness distribution in gravity-driven pancake-shaped droplets rising in a Hele-Shaw cell.
Journal of Fluid Mechanics,
Vol. 874,
Issue. ,
p.
1021.
Kerdraon, Margaux
McGraw, Joshua D.
Dollet, Benjamin
and
Jullien, Marie-Caroline
2019.
Self-Similar Relaxation of Confined Microfluidic Droplets.
Physical Review Letters,
Vol. 123,
Issue. 2,
Reichert, Benjamin
Cantat, Isabelle
and
Jullien, Marie-Caroline
2019.
Predicting droplet velocity in a Hele-Shaw cell.
Physical Review Fluids,
Vol. 4,
Issue. 11,
Esmaili, Ehsan
Shukla, Pranav
Eifert, Joseph D.
and
Jung, Sunghwan
2019.
Bubble impact on a tilted wall: Removing bacteria using bubbles.
Physical Review Fluids,
Vol. 4,
Issue. 4,
Sawaguchi, Erina
Matsuda, Ayumi
Hama, Kai
Saito, Masafumi
and
Tagawa, Yoshiyuki
2019.
Droplet levitation over a moving wall with a steady air film.
Journal of Fluid Mechanics,
Vol. 862,
Issue. ,
p.
261.
Takeishi, Naoki
Ito, Hiroaki
Kaneko, Makoto
and
Wada, Shigeo
2019.
Deformation of a Red Blood Cell in a Narrow Rectangular Microchannel.
Micromachines,
Vol. 10,
Issue. 3,
p.
199.
Chakraborty, I.
Ricouvier, J.
Yazhgur, P.
Tabeling, P.
and
Leshansky, A. M.
2019.
Droplet generation at Hele-Shaw microfluidic T-junction.
Physics of Fluids,
Vol. 31,
Issue. 2,
Murano, Mayuko
and
Okumura, Ko
2020.
Rising bubble in a cell with a high aspect ratio cross-section filled with a viscous fluid and its connection to viscous fingering.
Physical Review Research,
Vol. 2,
Issue. 1,
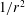 $1/r^{2}$ spatial decay is confirmed numerically. We pinpoint counter-rotating streamwise vortex structures near the lateral interface of the droplet, further highlighting the complex 3D flow pattern.
$1/r^{2}$ spatial decay is confirmed numerically. We pinpoint counter-rotating streamwise vortex structures near the lateral interface of the droplet, further highlighting the complex 3D flow pattern.




