Article contents
Equilibrium structure and diffusion in concentrated hydrodynamically interacting suspensions confined by a spherical cavity
Published online by Cambridge University Press: 11 December 2017
Abstract
The short- and long-time equilibrium transport properties of a hydrodynamically interacting suspension confined by a spherical cavity are studied via Stokesian dynamics simulations for a wide range of particle-to-cavity size ratios and particle concentrations. Many-body hydrodynamic and lubrication interactions between particles and with the cavity are accounted for utilizing recently developed mobility and resistance tensors for spherically confined suspensions (Aponte-Rivera & Zia, Phys. Rev. Fluids, vol. 1(2), 2016, 023301). Study of particle volume fractions in the range 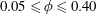 $0.05\leqslant \unicode[STIX]{x1D719}\leqslant 0.40$ reveals that confinement exerts a qualitative influence on particle diffusion. First, the mean-square displacement over all time scales depends on the position in the cavity. Additionally, at short times, the diffusivity is anisotropic, with diffusion along the cavity radius slower than diffusion tangential to the cavity wall, due to the anisotropy of hydrodynamic coupling and to confinement-induced spatial heterogeneity in particle concentration. The mean-square displacement is anisotropic at intermediate times as well and, surprisingly, exhibits superdiffusive and subdiffusive behaviours for motion along and perpendicular to the cavity radius respectively, depending on the suspension volume fraction and the particle-to-cavity size ratio. No long-time self-diffusive regime exists; instead, the mean-square displacement reaches a long-time plateau, a result of entropic restriction to a finite volume. In this long-time limit, the higher the volume fraction is, the longer the particles take to reach the long-time plateau, as cooperative rearrangements are required as the cavity becomes crowded. The ordered dynamical heterogeneity seen here promotes self-organization of particles based on their size and self-mobility, which may be of particular relevance in biophysical systems.
$0.05\leqslant \unicode[STIX]{x1D719}\leqslant 0.40$ reveals that confinement exerts a qualitative influence on particle diffusion. First, the mean-square displacement over all time scales depends on the position in the cavity. Additionally, at short times, the diffusivity is anisotropic, with diffusion along the cavity radius slower than diffusion tangential to the cavity wall, due to the anisotropy of hydrodynamic coupling and to confinement-induced spatial heterogeneity in particle concentration. The mean-square displacement is anisotropic at intermediate times as well and, surprisingly, exhibits superdiffusive and subdiffusive behaviours for motion along and perpendicular to the cavity radius respectively, depending on the suspension volume fraction and the particle-to-cavity size ratio. No long-time self-diffusive regime exists; instead, the mean-square displacement reaches a long-time plateau, a result of entropic restriction to a finite volume. In this long-time limit, the higher the volume fraction is, the longer the particles take to reach the long-time plateau, as cooperative rearrangements are required as the cavity becomes crowded. The ordered dynamical heterogeneity seen here promotes self-organization of particles based on their size and self-mobility, which may be of particular relevance in biophysical systems.
- Type
- JFM Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
Aponte-Rivera et al. supplementary movie
Stokesian dynamics simulation with full, many-body hydrodynamic and lubrication interactions for a spherically confined, concentrated colloidal suspension. The no-slip and no-flux confining cavity is rigorously modeled via newly developed hydrodynamic mobility couplings. Model can simulate volume fractions up to maximum packing and arbitrary particle-to-cavity size ratio. Shown here is equilibrium diffusion for 30% volume fraction and particles 10% of cavity size.”
- 17
- Cited by





