Hostname: page-component-76fb5796d-x4r87 Total loading time: 0 Render date: 2024-04-25T12:02:24.529Z Has data issue: false hasContentIssue false
  • English
  • Français

Degeneration of internal Kelvin waves in a continuous two-layer stratification

Published online by Cambridge University Press:  15 July 2015

Hugo N. Ulloa ,
Kraig B. Winters ,
Alberto de la Fuente  and
Yarko Niño
Hugo N. Ulloa*
Affiliation:
Departamento de Ingeniera Civil, Universidad de Chile, Av. Blanco Encalada 2002, CP 8370449,  Santiago, Chile
Kraig B. Winters
Affiliation:
Scripps Institution of Oceanography and Mechanical and Aerospace Engineering, University of California San Diego, La Jolla, CA 92093, USA
Alberto de la Fuente
Affiliation:
Departamento de Ingeniera Civil, Universidad de Chile, Av. Blanco Encalada 2002, CP 8370449,  Santiago, Chile
Yarko Niño
Affiliation:
Departamento de Ingeniera Civil, Universidad de Chile, Av. Blanco Encalada 2002, CP 8370449,  Santiago, Chile Advanced Mining Technology Center, Universidad de Chile, Av. Tupper 2007, CP 8370451,  Santiago, Chile
*
†Email address for correspondence: hulloa@ing.uchile.cl
Get access Rights & Permissions [Opens in a new window]

Abstract

We explore the evolution of the gravest internal Kelvin wave in a two-layer rotating cylindrical basin, using direct numerical simulations (DNS) with a hyper-viscosity/diffusion approach to illustrate different dynamic and energetic regimes. The initial condition is derived from Csanady’s (J. Geophys. Res., vol. 72, 1967, pp. 4151–4162) conceptual model, which is adapted by allowing molecular diffusion to smooth the discontinuous idealized solution over a transition scale, ${\it\delta}_{i}$, taken to be small compared to both layer thicknesses 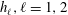$h_{\ell },\ell =1,2$. The different regimes are obtained by varying the initial wave amplitude, 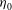${\it\eta}_{0}$, for the same stratification and rotation. Increasing 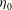${\it\eta}_{0}$ increases both the tendency for wave steepening and the shear in the vicinity of the density interface. We present results across several regimes: from the damped, linear–laminar regime (DLR), for which 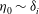${\it\eta}_{0}\sim {\it\delta}_{i}$ and the Kelvin wave retains its linear character, to the nonlinear–turbulent transition regime (TR), for which the amplitude ${\it\eta}_{0}$ approaches the thickness of the (thinner) upper layer 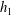$h_{1}$, and nonlinearity and dispersion become significant, leading to hydrodynamic instabilities at the interface. In the TR, localized turbulent patches are produced by Kelvin wave breaking, i.e. shear and convective instabilities that occur at the front and tail of energetic waves within an internal Rossby radius of deformation from the boundary. The mixing and dissipation associated with the patches are characterized in terms of dimensionless turbulence intensity parameters that quantify the locally elevated dissipation rates of kinetic energy and buoyancy variance.

JFM classification

Geophysical and Geological Flows: Stratified flows Instability: Transition to turbulence Geophysical and Geological Flows: Waves in rotating fluids
Type
Papers
Information
Journal of Fluid Mechanics , Volume 777 , 25 August 2015 , pp. 68 - 96
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramowitz, M. & Stegun, A. 1965 Handbook of Mathematical Functions: With Formulas, Graphs, and Mathematical Tables. Dover.Google Scholar
Almgren, A., Camassa, R. & Tiron, R. 2012 Shear instability of internal solitary waves in Euler fluids with thin pycnoclines. J. Fluid Mech. 710, 324–361.CrossRefGoogle Scholar
Antenucci, J. & Imberger, J. 2001 Energetics of long internal gravity waves in large lakes. Limnol. Oceanogr. 46, 1760–1773.Google Scholar
Barad, M. F. & Fringer, O. B. 2010 Simulations of shear instabilities in interfacial gravity waves. J. Fluid Mech. 644, 61–95.CrossRefGoogle Scholar
Barry, M. E., Ivey, G. N., Winters, K. B. & Imberger, J. 2001 Measurements of diapycnal diffusivities in stratified fluids. J. Fluid Mech. 442, 267–291.Google Scholar
Beletsky, D., O’Connor, W. P., Schwab, D. J. & Dietrich, D. E. 1997 Numerical simulation of internal Kelvin waves and coastal upwelling fronts. J. Phys. Oceanogr. 27, 1197–1215.2.0.CO;2>CrossRefGoogle Scholar
Boegman, L., Imberger, J., Ivey, G. & Antenucci, J. 2003 High-frequency internal waves in large stratified lakes. Limnol. Oceanogr. 48 (2), 895–919.CrossRefGoogle Scholar
Boegman, L., Ivey, G. & Imberger, J. 2005 The energetics of large-scale internal wave degeneration in lakes. J. Fluid Mech. 531, 159–180.CrossRefGoogle Scholar
Boehrer, B. & Schultze, M. 2008 Stratification of lakes. Rev. Geophys. 46, RG2005.Google Scholar
Bouffard, D. & Lemmin, U. 2013 Kelvin waves in Lake Geneva. J. Great Lakes Res. 39 (4), 637–645.Google Scholar
Carr, M., Fructus, D., Grue, J., Jensen, A. & Davies, P. A. 2008 Convectively induced shear instability in large amplitude internal solitary waves. Phys. Fluids 20, 126601.Google Scholar
Carr, M., King, St. E. & Dritschel, D. G. 2011 Numerical simulation of shear-induced instabilities in internal solitary waves. J. Fluid Mech. 683, 263–288.CrossRefGoogle Scholar
Csanady, G. T. 1967 Large-scale motion in the Great Lakes. J. Geophys. Res. 72, 4151–4162.Google Scholar
Csanady, G. T. 1981 On the structure of transient upwelling events. J. Phys. Oceanogr. 12, 84–96.Google Scholar
Fedorov, A. & Melville, W. K. 1995 Propagation and breaking of nonlinear Kelvin waves. J. Phys. Oceanogr. 25, 2518–2531.2.0.CO;2>CrossRefGoogle Scholar
Flór, J.-B., Scolan, H. & Gula, J. 2011 Frontal instabilities and waves in a differentially rotating fluid. J. Fluid Mech. 685, 532–542.Google Scholar
Fructus, D., Carr, M., Grue, J., Jensen, A. & Davies, P. A. 2009 Shear-induced breaking of large internal solitary waves. J. Fluid Mech. 620, 1–29.Google Scholar
de la Fuente, A., Shimizu, K., Imberger, J. & Niño, Y. 2008 The evolution of internal waves in a rotating, stratified, circular basin and the influence of weakly nonlinear and nonhydrostatic accelerations. Limnol. Oceanogr. 53 (6), 2738–2748.Google Scholar
de la Fuente, A., Shimizu, K., Niño, Y. & Imberger, J. 2010 Nonlinear and weakly nonhydrostatic inviscid evolution of internal gravitational basin-scale waves in a large, deep lake: Lake Constance. J. Geophys. Res. 115, C12045.Google Scholar
Grimshaw, R. H. J. 1985 Evolution equation for weakly nonlinear, long internal waves in a rotating fluid. Stud. Appl. Maths 73, 1–33.Google Scholar
Grue, J., Jensen, A., Rusas, P.-O. & Sveen, J. K. 1999 Properties of large-amplitude internal waves. J. Fluid Mech. 380, 257–278.Google Scholar
Grue, J., Jensen, A., Rusas, P.-O. & Sveen, J. K. 2000 Breaking and broadening of internal solitary waves. J. Fluid Mech. 413, 181–217.Google Scholar
Gula, J., Plougonven, R. & Zeitlin, V. 2009 Ageostrophic instabilities of fronts in a channel in a stratified rotating fluid. J. Fluid Mech. 627, 485–507.Google Scholar
van Haren, H. 2015 Instability observations associated with wave breaking in the stable-stratified deep-ocean. Physica D 292–293, 62–69.Google Scholar
Helfrich, K. & Melville, W. K. 2006 Long nonlinear internal waves. Annu. Rev. Fluid Mech. 38, 395–425.CrossRefGoogle Scholar
Horn, D. A., Imberger, J. & Ivey, G. N. 2001 The degeneration of large-scale interfacial gravity waves in lakes. J. Fluid Mech. 434, 181–207.Google Scholar
Kundu, P. & Cohen, I. 2004 Fluid Mechanics. Elsevier Academic.Google Scholar
Lamb, H. 1932 Hydrodynamics, 6th edn. Dover.Google Scholar
Lamorgese, A. G., Caughey, D. A. & Pope, S. B. 2005 Direct numerical simulation of homogeneous turbulence with hyperviscosity. Phys. Fluids 17, 015106.CrossRefGoogle Scholar
Lorke, A. 2007 Boundary mixing in the thermocline of a large lake. J. Geophys. Res. 112, C09019.Google Scholar
Lorke, A., Peeters, F. & Bäuerle, E. 2006 High-frequency internal waves in the littoral zone of large lake. Limnol. Oceanogr. 51 (4), 1935–1936.Google Scholar
Matsumoto, Y. & Hoshino, M. 2004 Onset of turbulence induced by a Kelvin–Helmholtz vortex. Geophys. Res. Lett. 31, L02807.Google Scholar
Miles, J. W. 1961 On the stability of heterogeneous shear flows. J. Fluid Mech. 10, 496–508.Google Scholar
Moum, J. N., Farmer, D. M., Smyth, W. D., Armi, L. & Vagle, S. 2003 Structure and generation of turbulence at interfaces strained by internal solitary waves propagating shoreward over the continental shelf. J. Phys. Oceanogr. 33, 2093–2112.2.0.CO;2>CrossRefGoogle Scholar
Pedlosky, J. 1970 Finite-amplitude baroclinic waves. J. Atmos. Sci. 27, 15–30.2.0.CO;2>CrossRefGoogle Scholar
Pedlosky, J. 1987 Geophysical Fluid Dynamics. Springer.Google Scholar
Preusse, M., Freistühler, H. & Peeters, F. 2012a Seasonal variation of solitary wave properties in Lake Constance. J. Geophys. Res. 117, C04026.Google Scholar
Preusse, M., Peeters, F. & Lorke, A. 2010 Internal waves and the generation of turbulence in the thermocline of a large lake. Limnol. Oceanogr. 55 (6), 2353–2365.Google Scholar
Preusse, M., Stastna, M., Freistühler, H. & Peeters, F. 2012b Intrinsic breaking of internal solitary waves in a deep lake. PLoS ONE 7, e41674.Google Scholar
Rozas, C., de la Fuente, A., Ulloa, H., Davies, P. & Niño, Y. 2014 Quantifying the effect of wind on internal wave resonance in Lake Villarrica, Chile. Environ. Fluid Mech. 14 (4), 849–871.Google Scholar
Sakai, S. 1989 Rossby–Kelvin instability: a new type of ageostrophic instability caused by a resonance between Rossby waves and gravity waves. J. Fluid Mech. 202, 149–176.Google Scholar
Sakai, T. & Redekopp, L. G. 2010 A weakly nonlinear evolution model for long internal waves in a large lake. J. Fluid Mech. 637, 137–172.Google Scholar
Shimizu, K. & Imberger, J. 2009 Damping mechanisms of internal waves in continuously stratified rotating basins. J. Fluid Mech. 637, 137–172.Google Scholar
Spyksma, K., Magcalas, M. & Campbell, N. 2012 Quantifying effects of hyperviscosity on isotropic turbulence. Phys. Fluids 24, 125102.Google Scholar
Stocker, R. & Imberger, J. 2003 Energy partitioning and horizontal dispersion in a stratified rotating lake. J. Phys. Oceanogr. 33, 512–529.Google Scholar
Thomson, W. (Lord Kelvin) 1879 On gravitational oscillations of rotating water. Proc. R. Soc. Edinburgh. 10, 92–100.Google Scholar
Ulloa, H. N., de la Fuente, A. & Niño, Y. 2014 An experimental study of the free evolution of rotating, nonlinear internal gravity waves in a two-layer stratified fluid. J. Fluid Mech. 742, 308–339.Google Scholar
Waite, M. L. & Bartello, P. 2004 Stratified turbulence dominated by vortical motion. J. Fluid Mech. 517, 281–308.Google Scholar
Wake, G. W., Ivey, G. N. & Imberger, J. 2005 The temporal evolution of baroclinic basin-scale waves in a rotating circular basin. J. Fluid Mech. 523, 367–392.Google Scholar
Winters, K. B. & D’Asaro, E. A. 1996 Diascalar flux and the rate of fluid mixing. J. Fluid Mech. 317, 179–193.Google Scholar
Winters, K. B. & D’Asaro, E. A. 1997 Direct simulation of internal wave energy transfer. J. Phys. Oceanogr. 27, 1937–1945.2.0.CO;2>CrossRefGoogle Scholar
Winters, K. B. & de la Fuente, A. 2012 Modelling rotating stratified flows at laboratory-scale using spectrally-based DNS. Ocean Model. 50, 47–59.Google Scholar
Winters, K. B., Lombard, P. N., Riley, J. J. & D’Asaro, E. A. 1995 Available potential energy and mixing in density-stratified fluids. J. Fluid Mech. 289, 115–128.Google Scholar
Wüest, A. & Lorke, A. 2003 Small-scale hydrodynamics in lakes. Annu. Rev. Fluid Mech. 35, 373–412.Google Scholar