Hostname: page-component-8448b6f56d-dnltx Total loading time: 0 Render date: 2024-04-25T03:33:16.127Z Has data issue: false hasContentIssue false
  • English
  • Français

An experimental study of the free evolution of rotating, nonlinear internal gravity waves in a two-layer stratified fluid

Published online by Cambridge University Press:  21 February 2014

Hugo N. Ulloa ,
Alberto de la Fuente  and
Yarko Niño
Hugo N. Ulloa*
Affiliation:
Departamento de Ingeniera Civil, Universidad de Chile, Blanco Encalada 2002, C.P. 8370449, Santiago, Chile
Alberto de la Fuente
Affiliation:
Departamento de Ingeniera Civil, Universidad de Chile, Blanco Encalada 2002, C.P. 8370449, Santiago, Chile
Yarko Niño
Affiliation:
Departamento de Ingeniera Civil, Universidad de Chile, Blanco Encalada 2002, C.P. 8370449, Santiago, Chile
*
†Email address for correspondence: hulloa@ing.uchile.cl
Get access Rights & Permissions [Opens in a new window]

Abstract

The temporal evolution of nonlinear large-scale internal gravity waves, in a two-layer flow affected by background rotation, is studied via laboratory experiments conducted in a cylindrical tank, mounted on a rotating turntable. The internal wave field is excited by the relaxation of an initial forced tilt of the density interface (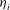$\eta _{i}$), which generates internal waves, such as Kelvin and Poincaré waves, in response to rotation effects. The behaviour of 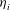$\eta _{i}$, in the shore region, is analysed in terms of the background rotation and the nonlinear steepening of the basin-scale waves. The results show that the degeneration of the fundamental Kelvin wave into a solitary-type wave packet is caused by nonlinear steepening and it is influenced by the background rotation. In addition, the physical scales of the leading solitary-type wave are closer to Korteweg–de Vries theory as the rotation increases. Moreover, the nonlinear interaction between the Kelvin wave and the Poincaré wave can transfer energy to higher or lower frequencies than the frequency of the fundamental Kelvin wave, as a function of the background rotation. In particular, a specific normal mode in the off-shore region could be energized by this interaction. Finally, the bulk decay rate of the fundamental Kelvin wave, $\tau _{dk}$, was investigated. The results exhibit that 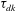$\tau _{dk}$ is concordant with the Ekman damping time scale when there is no evidence of steepening in the basin-scale waves. However, as nonlinear processes increase, 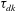$\tau _{dk}$ shows a strong decrease. In this context, the nonlinear processes play an important role in the decay of the fundamental Kelvin wave, via the energy radiation to other modes. The results reported demonstrate that the background rotation and nonlinear processes are essential aspects in understanding the degeneration and the decay of large-scale internal gravity waves on enclosed basins.

JFM classification

Geophysical and Geological Flows: Waves in rotating fluids Geophysical and Geological Flows: Stratified flows Waves/Free-surface Flows: Solitary waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 742 , 10 March 2014 , pp. 308 - 339
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antenucci, J. & Imberger, J. 2001 Energetics of long internal gravity waves in large lakes. Limnol. Oceanogr. 46, 1760–1773.CrossRefGoogle Scholar
Appt, J., Imberger, J. & Kobus, H. 2004 Basin-scale motion in stratified upper Lake Constance. Limnol. Oceanogr. 4, 919–933.Google Scholar
Bendat, J. & Piersol, A. 2000 Random Data. Wiley.Google Scholar
Boegman, L., Imberger, J., Ivey, G. N. & Antenucci, J. P. 2003 High-frequency internal waves in large stratified lakes. Limnol. Oceanogr. 48, 895–919.Google Scholar
Boegman, L., Ivey, G. & Imberger, J. 2005a The degradation of internal waves in lakes with sloping topography. Limnol. Oceanogr. 50, 1620–1637.Google Scholar
Boegman, L., Ivey, G. & Imberger, J. 2005b The energetics of large-scale internal wave degeneration in lakes. J. Fluid. Mech. 531, 159–180.Google Scholar
Boegman, L. & Ivey, G. N. 2009 Flow separation and resuspension beneath shoaling nonlinear internal waves. J. Geophys. Res. 114, C02018.Google Scholar
Bouffard, D., Boegman, L.  & Rao, Y. R. 2012 Poincaré wave-induced mixing in a large lake. Limnol. Oceanogr. 57 (4), 1201–1216.Google Scholar
Csanady, G. T. 1967 Large-scale motion in the great lakes. J. Geophys. Res. 72, 4151–4162.Google Scholar
Csanady, G. 1968 Motions in a model great lake due to a suddenly imposed wind. J. Geophys. Res. 73, 6435–6447.Google Scholar
Csanady, G. T. 1975 Hydrodynamics of large lakes. Annu. Rev. Fluid Mech. 7, 347–386.Google Scholar
de la Fuente, A., Shimizu, K., Imberger, J. & Niño, Y. 2008 The evolution of internal waves in a rotating, stratified, circular basin and the influence of weakly nonlinear and nonhydrostatic accelerations. Limnol. Oceanogr. 53, 2738–2748.Google Scholar
de la Fuente, A., Shimizu, K., Niño, Y. & Imberger, J. 2010 Nonlinear and nonhydrostatic evolution of basin-scale waves in large deep lakes. J. Geophys. Res. 115, C12045.Google Scholar
El, G. A., Grimshaw, R. H. J. & Kamchatnov, A. M. 2005 Analytic model for a weakly dissipative shallow-water undular bore. Chaos 15, 037102.Google Scholar
El, G. A., Grimshaw, R. H. J. & Smyth, N. F. 2006 Unsteady undular bores in fully nonlinear shallow-water theory. Phys. Fluids 18, 027104.Google Scholar
Garcia, C. M., Cantero, M. I., Niño, Y. & Garcia, M. H. 2005 Turbulence measurements with acoustic doppler velocimeters. J. Hydraul. Eng. 131 (12), 1062–1073.Google Scholar
Grimshaw, R. H. J. 1985 Evolution equation for weakly nonlinear, long internal waves in a rotating fluid. Stud. Appl. Maths 73, 1–33.Google Scholar
Grimshaw, R. H. J. & Helfrich, K. 2008 Long-time solutions of the Ostrovsky equation. Stud. Appl. Maths 121, 71–88.Google Scholar
Grimshaw, R. H. J. & Helfrich, K. 2012 The effect of rotation on internal solitary waves. J. Appl. Math 77, 326–339.Google Scholar
Grimshaw, R. H. J., Helfrich, K. & Johnson, E. R. 2012 The reduced Ostrovsky equation: Integrability and breaking. Stud. Appl. Maths 129, 414–435.Google Scholar
Grimshaw, R. H. J., Helfrich, K. R. & Johnson, E. R. 2013 Experimental study of the effect of rotation on large amplitude internal waves. Phys. Fluids 25, 056602.Google Scholar
Grimshaw, R. H. J. & Ostrovsky, L. A. 1998 Terminal damping of a solitary wave due to radiation in rotational systems. Stud. Appl. Maths 101, 289–338.CrossRefGoogle Scholar
Grimshaw, R. H. J., Ostrovsky, L. A., Shrira, V. I. & Stepanyants, Y. A. 1998 Long nonlinear surface and internal gravity waves in a rotating ocean. Surv. Geophys. 19, 289–338.CrossRefGoogle Scholar
Hammack, J. L. & Henderson, D. M. 1993 Resonant interactions among surface water waves. Annu. Rev. Fluid Mech. 25, 55–97.Google Scholar
Helfrich, K. & Grimshaw, R. H. J. 2008 Nonlinear disintegration of the internal tide. J. Phys. Oceanogr. 38, 686–701.Google Scholar
Helfrich, K. & Melville, W. K. 2006 Long nonlinear internal waves. Annu. Rev. Fluid Mech. 38, 395–425.Google Scholar
Horn, D. A., Imberger, J. & Ivey, G. 2001 The degeneration of large-scale interfacial gravity waves in lakes. J. Fluid. Mech. 434, 181–207.Google Scholar
Ivey, G. N., Winters, K. B. & Koseff, J. R. 2008 Density stratification, turbulence, but how much mixing?. Annu. Rev. Fluid Mech. 40, 169–184.Google Scholar
Johnson, E. R. & Grimshaw, R. H. J. 2013 Modified reduced Ostrovsky equation: Integrability and breaking. Phys. Rev. E 88, 021201.Google Scholar
Kamchatnov, A. M., Kuo, Y. H., Lin, T. C., Horng, T. L., Gou, S. C., Clift, R., El, G. A. & Grimshaw, R. H. J. 2012 Undular bore theory for the garder equation. Phys. Rev. E 86, 036605.Google Scholar
Katsis, C. & Akylas, T. R. 1987 Solitary internal waves in a rotating channel: A numerical study. Phys. Fluids 30, 297–301.Google Scholar
Lorke, A. 2007 Boundary mixing in the thermocline of a large lake. J. Geophys. Res. 112, C09019.Google Scholar
MacIntyre, S., Clark, J., Jellison, R. & Jonathan, F. 2009 Turbulent mixing induced by nonlinear internal waves in Mono Lake, California. Limnol. Oceanogr. 54, 2255–2272.Google Scholar
Martinsen, E. & Weber, J. E. 1981 Frictional influence on internal kelvin waves. Tellus 33, 402–410.Google Scholar
Maxworthy, R. 1983 Experiments in solitary internal kelvin waves. J. Fluid. Mech. 129, 365–383.Google Scholar
Melville, W. K., Tomasson, G. G. & Renouard, D. P. 1989 On the stability of kelvin waves. J. Fluid. Mech. 206, 1–23.Google Scholar
Ostrovsky, L. A. & Stepanyants, Y. A. 2005 Internal solitons in laboratory experiments: Comparison with theoretical models. Chaos 15, 1–28.Google Scholar
Pedlosky, J. 1987 Geophysical Fluid Dynamics. Springer.Google Scholar
Preusse, M., Freisthler, H. & Peeters, F. 2012 Seasonal variation of solitary wave properties in Lake Constance. J. Geophys. Res. 117, C04026.Google Scholar
Preusse, M., Peeters, F. & Lorke, A. 2010 Internal waves and the generation of turbulence in the thermocline of a large lakes. Limnol. Oceanogr. 55 (6), 2353–2365.CrossRefGoogle Scholar
Renouard, D. P., Chabert, D. & Zhang, X. 1987 An experimental study of strongly nonlinear waves in a rotating system. J. Fluid. Mech. 177, 381–394.Google Scholar
Renouard, D. P., Tomasson, G. G. & Melville, W. K. 1993 An experimental and numerical study of nonlinear internal waves. Phys. Fluids A 5, 1401–1411.Google Scholar
Rozas, C., de la Fuente, A., Ulloa, H., Davies, P. & Niño, Y. 2013 Quantifying the effect of wind on internal wave resonance in Lake Villarrica, Chile. Environ. Fluid Mech. doi:10.1007/s10652-013-9329-9.CrossRefGoogle Scholar
Sakai, T. & Redekopp, L. 2010 A weakly nonlinear evolution model for long internal waves in a large lake. J. Fluid Mech. 637, 137–172.Google Scholar
Sakai, T. & Redekopp, L. G. 2011 Lagrangian transport in a circular lake: effect of nonlinearity and the second vertical mode. Nonlinear Process. Geophys. 18, 765–778.Google Scholar
Shimizu, K. & Imberger, J. 2009 Damping mechanisms of internal waves in constinuously stratified rotating basins. J. Fluid Mech. 637, 137–172.Google Scholar
Shimizu, K. & Imberger, J. 2010 Seasonal difference in the evolution of damped basin-scale internal waves in shallow stratified lake. Limnol. Oceanogr. 55, 1449–1462.Google Scholar
Shimizu, K., Imberger, J. & Kumagai, M. 2007 Horizontal structure and excitation of primary motion in a strongly stratified lake. Limnol. Oceanogr. 52, 2641–2655.Google Scholar
Staquet, C. & Sommeria, J. 2002 Internal gravity waves: From instabilities to turbulence. Annu. Rev. Fluid Mech. 34, 559–593.Google Scholar
Stocker, R. & Imberger, J. 2003 Energy partitioning and horizontal dispersion in a stratified rotating lake. J. Phys. Oceanogr. 33, 512–529.Google Scholar
Stocker, R., Imberger, J. & D’alpaos, L. 2000 An analytical model of a circular stratified rotating basin under the effect of periodic wind forcing. In 5th Intl Symp. in Stratified Flows, Vancouver, Canada pp. 387–392. International Association for Hydraulic Research.Google Scholar
Wake, G. W., Ivey, G. N. & Imberger, J. 2004 Baroclinic geostrophic adjustment in a rotating circular basin. J. Fluid Mech 515, 63–86.Google Scholar
Wake, G. W., Ivey, G. N. & Imberger, J. 2005 The temporal evolution of baroclinic basin-scale waves in a rotating circular basin. J. Fluid Mech. 523, 367–392.Google Scholar
Wüest, A. & Lorke, A. 2003 Small-scale hydrodynamics in lakes. Annu. Rev. Fluid Mech. 35, 373–412.CrossRefGoogle Scholar