Article contents
DITKIN CONDITIONS
Published online by Cambridge University Press: 27 February 2017
Abstract
This paper is about the connection between certain Banach-algebraic properties of a commutative Banach algebra E with unit and the associated commutative Banach algebra C(X, E) of all continuous functions from a compact Hausdorff space X into E. The properties concern Ditkin's condition and bounded relative units. We show that these properties are shared by E and C(X, E). We also consider the relationship between these properties in the algebras E, B and 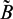 $\~{B}$ that appear in the so-called admissible quadruples (X, E, B,
$\~{B}$ that appear in the so-called admissible quadruples (X, E, B, 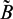 $\~{B}$).
$\~{B}$).
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2017
References
REFERENCES
- 1
- Cited by




