Article contents
The generalized Fermat equation with exponents 2, 3,  $n$
$n$
Published online by Cambridge University Press: 26 November 2019
Abstract
We study the generalized Fermat equation 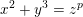 $x^{2}+y^{3}=z^{p}$, to be solved in coprime integers, where
$x^{2}+y^{3}=z^{p}$, to be solved in coprime integers, where 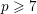 $p\geqslant 7$ is prime. Modularity and level-lowering techniques reduce the problem to the determination of the sets of rational points satisfying certain 2-adic and 3-adic conditions on a finite set of twists of the modular curve
$p\geqslant 7$ is prime. Modularity and level-lowering techniques reduce the problem to the determination of the sets of rational points satisfying certain 2-adic and 3-adic conditions on a finite set of twists of the modular curve 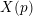 $X(p)$. We develop new local criteria to decide if two elliptic curves with certain types of potentially good reduction at 2 and 3 can have symplectically or anti-symplectically isomorphic
$X(p)$. We develop new local criteria to decide if two elliptic curves with certain types of potentially good reduction at 2 and 3 can have symplectically or anti-symplectically isomorphic 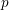 $p$-torsion modules. Using these criteria we produce the minimal list of twists of
$p$-torsion modules. Using these criteria we produce the minimal list of twists of 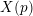 $X(p)$ that have to be considered, based on local information at 2 and 3; this list depends on
$X(p)$ that have to be considered, based on local information at 2 and 3; this list depends on 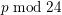 $p\hspace{0.2em}{\rm mod}\hspace{0.2em}24$. We solve the equation completely when
$p\hspace{0.2em}{\rm mod}\hspace{0.2em}24$. We solve the equation completely when 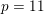 $p=11$, which previously was the smallest unresolved
$p=11$, which previously was the smallest unresolved 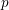 $p$. One new ingredient is the use of the ‘Selmer group Chabauty’ method introduced by the third author, applied in an elliptic curve Chabauty context, to determine relevant points on
$p$. One new ingredient is the use of the ‘Selmer group Chabauty’ method introduced by the third author, applied in an elliptic curve Chabauty context, to determine relevant points on 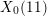 $X_{0}(11)$ defined over certain number fields of degree 12. This result is conditional on the generalized Riemann hypothesis, which is needed to show correctness of the computation of the class groups of five specific number fields of degree 36. We also give some partial results for the case
$X_{0}(11)$ defined over certain number fields of degree 12. This result is conditional on the generalized Riemann hypothesis, which is needed to show correctness of the computation of the class groups of five specific number fields of degree 36. We also give some partial results for the case 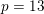 $p=13$. The source code for the various computations is supplied as supplementary material with the online version of this article.
$p=13$. The source code for the various computations is supplied as supplementary material with the online version of this article.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Authors 2019
Footnotes
The work reported on in this paper was supported by the Deutsche Forschungsgemeinschaft, grant Sto 299/11-1, in the framework of the Priority Programme SPP 1489. The first author was also partly supported by the grant Proyecto RSME-FBBVA 2015 José Luis Rubio de Francia.
References
- 3
- Cited by




