Article contents
The Probability of Non-Existence of a Subgraph in a Moderately Sparse Random Graph
Published online by Cambridge University Press: 09 May 2018
Abstract
We develop a general procedure that finds recursions for statistics counting isomorphic copies of a graph G0 in the common random graph models 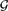 ${\cal G}$(n,m) and
${\cal G}$(n,m) and 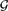 ${\cal G}$(n,p). Our results apply when the average degrees of the random graphs are below the threshold at which each edge is included in a copy of G0. This extends an argument given earlier by the second author for G0=K3 with a more restricted range of average degree. For all strictly balanced subgraphs G0, our results give much information on the distribution of the number of copies of G0 that are not in large ‘clusters’ of copies. The probability that a random graph in
${\cal G}$(n,p). Our results apply when the average degrees of the random graphs are below the threshold at which each edge is included in a copy of G0. This extends an argument given earlier by the second author for G0=K3 with a more restricted range of average degree. For all strictly balanced subgraphs G0, our results give much information on the distribution of the number of copies of G0 that are not in large ‘clusters’ of copies. The probability that a random graph in 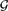 ${\cal G}$(n,p) has no copies of G0 is shown to be given asymptotically by the exponential of a power series in n and p, over a fairly wide range of p. A corresponding result is also given for
${\cal G}$(n,p) has no copies of G0 is shown to be given asymptotically by the exponential of a power series in n and p, over a fairly wide range of p. A corresponding result is also given for 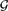 ${\cal G}$(n,m), which gives an asymptotic formula for the number of graphs with n vertices, m edges and no copies of G0, for the applicable range of m. An example is given, computing the asymptotic probability that a random graph has no triangles for p=o(n−7/11) in
${\cal G}$(n,m), which gives an asymptotic formula for the number of graphs with n vertices, m edges and no copies of G0, for the applicable range of m. An example is given, computing the asymptotic probability that a random graph has no triangles for p=o(n−7/11) in 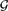 ${\cal G}$(n,p) and for m=o(n15/11) in
${\cal G}$(n,p) and for m=o(n15/11) in 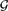 ${\cal G}$(n,m), extending results of the second author.
${\cal G}$(n,m), extending results of the second author.
MSC classification
- Type
- Paper
- Information
- Combinatorics, Probability and Computing , Volume 27 , Special Issue 4: Special Issue: Oberwolfach Workshop , July 2018 , pp. 672 - 715
- Copyright
- Copyright © Cambridge University Press 2018
References
- 3
- Cited by




