Hostname: page-component-76fb5796d-9pm4c Total loading time: 0 Render date: 2024-04-25T18:07:42.155Z Has data issue: false hasContentIssue false
  • English
  • Français

Description of Entire Solutions of Eiconal Type Equations

Published online by Cambridge University Press:  20 November 2018

Der-Chen Chang  and
Bao Qin Li
Der-Chen Chang
Affiliation:
Department of Mathematics, Georgetown University, Washington, DC 20057 USAe-mail: chang@georgetown.edu
Bao Qin Li
Affiliation:
Department of Mathematics, Florida International University, Miami, FL 33199 USAe-mail: libaoqin@fiu.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

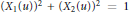The paper describes entire solutions to the eiconal type non-linear partial differential equations, which include the eiconal equations ${{({{X}_{1}}(u))}^{2}}\,+\,{{({{X}_{2}}(u))}^{2}}\,=\,1$ as special cases, where 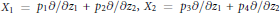${{X}_{1}}\,=\,{{p}_{1}}\partial /\partial {{z}_{1}}\,+\,{{p}_{2}}\partial /\partial {{z}_{2}},\,{{X}_{2}}\,=\,{{p}_{3}}\partial /\partial {{z}_{1}}\,+\,{{p}_{4}}\partial /\partial {{z}_{2}}$ are linearly independent operators with ${{p}_{j}}$ being arbitrary polynomials in 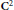${{\mathbf{C}}^{2}}$.

Keywords

32A1535F20entire solutioneiconal equationpolynomialtranscendental function
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 55 , Issue 2 , 01 June 2012 , pp. 249 - 259
Copyright
Copyright © Canadian Mathematical Society 2012

References

[1] Bernstein, S., Über ein geometrisches Theorem und seine Anwendung auf die partiellen Differentialgleichungen vom elliptischen Typus. Math. Z. 26(1927), no. 1, 551–558. http://dx.doi.org/10.1007/BF01475472 Google Scholar
[2] Calin, O. and Chang, D. C., Geometric Mechanics on Riemannian Manifolds. Applications to Partial Differential Equations. Birkhäuser, Boston, 2005.Google Scholar
[3] Courant, R. and Hilbert, D., Methods of Mathematical Physics. II. Partial Differential Equations. Interscience John Wiley & Sons, New York, 1989.Google Scholar
[4] Chang, D. C., Li, B. Q., and Yang, C. C., On composition of meromorphic functions in several complex variables. Forum Math 7(1995), no. 1, 77–94. http://dx.doi.org/10.1515/form.1995.7.77 Google Scholar
[5] Garabedian, P. R., Partial Differential Equations. John Wiley, New York, 1964.Google Scholar
[6] Jörgens, K., Über die Lösungen der Differentialgeichung rt – s2 = 1. Math. Ann. 127(1954), 130–134. http://dx.doi.org/10.1007/BF01361114 Google Scholar
[7] Khavinson, D., A note on entire solutions of the eiconal equation. Amer. Math. Monthly 102(1995), no. 2, 159–161. http://dx.doi.org/10.2307/2975351 Google Scholar
[8] Li, B. Q., Entire solutions of eiconal type equations. Arch. Math. 89(2007), no. 4, 350–357.Google Scholar
[9] Nitsche, J. C. C., Elementary proof of Bernstein's theorem on minimal surfaces. Ann. of Math. 66(1957), 593–594. http://dx.doi.org/10.2307/1969907 Google Scholar
[10] Saleeby, E. G., Entire and meromorphic solutions of Fermat type partial differential equations. Analysis 19(1999), no. 4, 369–376.Google Scholar
[11] Stoll, W., Introduction to the value distribution theory of meromorphic functions. In: Complex Analysis. Lecture Notes in Math. 950. Springer-Verlag, Berlin, 1982.Google Scholar
[12] Vitter, A., The lemma of the logarithmic derivative in several complex variables. Duke Math. J. 44(1977), no. 1, 89–104. http://dx.doi.org/10.1215/S0012-7094-77-04404-0 Google Scholar