Hostname: page-component-8448b6f56d-jr42d Total loading time: 0 Render date: 2024-04-20T01:32:30.948Z Has data issue: false hasContentIssue false
  • English
  • Français

NEAT AND CONEAT SUBMODULES OF MODULES OVER COMMUTATIVE RINGS

Part of: Theory of modules and ideals Modules, bimodules and ideals

Published online by Cambridge University Press:  07 August 2013

SEPTIMIU CRIVEI
SEPTIMIU CRIVEI*
Affiliation:
Faculty of Mathematics and Computer Science, ‘Babeş-Bolyai’ University, Str. Mihail Kogălniceanu 1, 400084 Cluj-Napoca, Romania email crivei@math.ubbcluj.ro
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

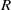We prove that neat and coneat submodules of a module coincide when $R$ is a commutative ring such that every maximal ideal is principal, extending a recent result by Fuchs. We characterise absolutely neat (coneat) modules and study their closure properties. We show that a module is absolutely neat if and only if it is injective with respect to the Dickson torsion theory.

Keywords

neat (coneat) exact sequence of modulesabsolutely neat (coneat) modulemaximal ideal

MSC classification

Secondary: 13C60: Module categories 13C05: Structure, classification theorems 16D10: General module theory
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 89 , Issue 2 , April 2014 , pp. 343 - 352
Copyright
Copyright ©2013 Australian Mathematical Publishing Association Inc. 

References

Afkhami, M., Karimi, M. and Khashyarmanesh, K., ‘On the regular digraph of ideals of commutative rings’, Bull. Aust. Math. Soc., to appear. doi:10.1017/S0004972712000792.CrossRefGoogle Scholar
Albu, T., ‘On a class of modules’, Stud. Cerc. Mat. 24 (1972), 1329–1392 (in Romanian).Google Scholar
Atiyah, M. F. and MacDonald, I. G., Introduction to Commutative Algebra (Addison-Wesley, London, 1969).Google Scholar
Borna, K. and Jafari, R., ‘On a class of monomial ideals’, Bull. Aust. Math. Soc. 87 (2013), 514–526.CrossRefGoogle Scholar
Crivei, I., ‘$\Omega $-pure submodules’, Stud. Cerc. Mat. 35 (1983), 255–269 (in Romanian).Google Scholar
Crivei, I., ‘$s$-pure submodules’, Intl J. Math. Math. Sci. 2005 (2005), 491–497.CrossRefGoogle Scholar
Crivei, I. and Crivei, S., ‘Absolutely $s$-pure modules’, Automat. Comput. Appl. Math. 6 (1998), 25–30.Google Scholar
Crivei, S., Injective Modules Relative to Torsion Theories (EFES, Cluj-Napoca, 2004) (available at http://math.ubbcluj.ro/~crivei).Google Scholar
Dickson, S. E., ‘A torsion theory for abelian categories’, Trans. Amer. Math. Soc. 121 (1966), 223–235.CrossRefGoogle Scholar
Fuchs, L., Infinite Abelian Groups, Vol. I (Academic Press, London, New York, 1970).Google Scholar
Fuchs, L., ‘Neat submodules over integral domains’, Period. Math. Hungar. 64 (2012), 131–143.Google Scholar
Honda, K., ‘Realism in the theory of abelian groups I’, Comment. Math. Univ. St. Pauli 5 (1956), 37–75.Google Scholar
Kelarev, A. V., Ring Constructions and Applications (World Scientific, River Edge, NJ, 2002).Google Scholar
Lam, T. Y., Lectures on Modules and Rings (Springer, Berlin, 1999).CrossRefGoogle Scholar
Lam, T. Y. and Reyes, M. L., ‘A Prime Ideal Principle in commutative algebra’, J. Algebra 319 (2008), 3006–3027.Google Scholar
Stenström, B., ‘Pure submodules’, Ark. Mat. 7 (1967), 159–171.Google Scholar
Wisbauer, R., Foundations of Module and Ring Theory (Gordon and Breach, Philadelphia, 1991).Google Scholar