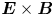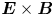1. Introduction
The Hasegawa–Mima equation (Hasegawa & Mima Reference Hasegawa and Mima1977a,Reference Hasegawa and Mimab) describes two-dimensional electrostatic plasma turbulence in a straight homogeneous magnetic field. Mathematically, it is related to the quasi-geostrophic equation for atmospheric dynamics on rotating planetary surfaces (Charney Reference Charney1948; Charney & Drazin Reference Charney and Drazin1961), and it reduces to the vorticity equation for a two-dimensional incompressible fluid in the limit of high electron temperature (Horton & Hasegawa Reference Horton and Hasegawa1994). As such, the Hasegawa–Mima equation exhibits properties analogous to two-dimensional fluid turbulence (Batchelor Reference Batchelor1969; Kraichnan & Montgomery Reference Kraichnan and Montgomery1980; Dritschel, Qi & Marston Reference Dritschel, Qi and Marston2015), including inverse cascade of energy (Kraichnan Reference Kraichnan1967; Rivera et al. Reference Rivera, Daniel, Chen and Ecke2003; Xiao et al. Reference Xiao, Wan, Chen and Eyink2009) associated with the presence of two inviscid invariants, energy and generalized enstrophy (Hasegawa & Mima Reference Hasegawa and Mima2018). Furthermore, in the inviscid limit the system is endowed with a non-canonical Hamiltonian structure (Weinstein Reference Weinstein1983; Morrison Reference Morrison1998), where energy and generalized enstrophy play the roles of Hamiltonian and Casimir invariant, respectively (Tassi, Chandre & Morrison Reference Tassi, Chandre and Morrison2009). These properties combined with a relative simplicity make the Hasegawa–Mima equation an effective tool in the study of two-dimensional fluid and plasma turbulence. Applications include the investigation of turbulence in geophysical flows (Nazarenko & Quinn Reference Nazarenko and Quinn2009) and magnetically confined plasmas (Horton Reference Horton1999; Fujisawa et al. Reference Fujisawa, Itoh, Iguchi, Matsuoka, Okamura, Shimizu, Minami, Yoshimura, Nagaoka and Takahashi2004), and more generally the characterization of self-organized turbulent states and zonal flows (Diamond, Hasegawa & Mima Reference Diamond, Hasegawa and Mima2011; Singh & Diamond Reference Singh and Diamond2021).
Several generalizations of the Hasegawa–Mima equation exist. On the one hand, the Hasegawa–Wakatani system (Hasegawa & Wakatani Reference Hasegawa and Wakatani1983; Wakatani & Hasegawa Reference Wakatani and Hasegawa1984) consists of coupled nonlinear equations for the electrostatic potential and the ion density. These equations reveal the interplay between drift wave turbulence and zonal flow mediated by the Kelvin–Helmholtz instability (Numata, Ball & Dewar Reference Numata, Ball and Dewar2007). Hasegawa and Wakatani also included the effect of field curvature in cylindrical geometry in their equations (Hasegawa & Wakatani Reference Hasegawa and Wakatani1987). On the other hand, reduced magnetohydrodynamics (Hazeltine Reference Hazeltine1983) and the four-field model (Hazeltine, Hsu & Morrison Reference Hazeltine, Hsu and Morrison1987) take into account the time evolution of magnetic flux, parallel ion velocity and electron pressure. Nevertheless, these models are two-dimensional, any deviation from a straight magnetic field being treated as a higher-order correction in the relevant ordering. Hence, a closed equation for the electric potential describing electrostatic plasma turbulence in a general magnetic field is not available at present. This deficiency, which can be ascribed to the rather elusive nature of the polarization drift (Cary & Brizard Reference Cary and Brizard2009; Kaufman Reference Kaufman1986) in non-trivial magnetic fields, has made the understanding of the impact of topology on the evolution of turbulence a difficult task because one usually needs to resort to complete models, such as gyrokinetic theory. In this context, the development of a pertinent toy equation may allow a simplified modelling of turbulence in complex plasma systems, and therefore help to elucidate the nonlinear physics of two-dimensional turbulence in non-uniform magnetic fields. Indeed, the Hasegawa–Mima equation and similar two-dimensional fluid equations have been useful in studying long-term evolution of coherent structures in Rossby wave turbulence including zonal flows (Yoden & Yamada Reference Yoden and Yamada1993; Obuse, Takehiro & Yamada Reference Obuse, Takehiro and Yamada2010).
The purpose of this paper is to fill such theoretical gap by deriving an equation describing the evolution of electrostatic turbulence in a general magnetic field for the simplest plasma system consisting of cold ions and a cloud of electrons obeying the Boltzmann distribution. As in the classical construction, in principle two approaches could be followed to obtain such an equation: gyrokinetic theory or a fluid description. In the former, one proceeds by integration of the guiding centre distribution function to obtain an equation for ion density fluctuations that leads to Hasegawa–Mima-type electrostatic turbulence by coupling with the gyrokinetic Poisson equation upon suitable assumptions including cold ions and an adiabatic electron response (Dubin, Krommes & Oberman Reference Dubin, Krommes and Oberman1983; Krommes Reference Krommes2002). It should be noted that, in this context, turbulence is mediated by a polarization density (Brizard & Hahm Reference Brizard and Hahm2007) rather than a polarization drift velocity. By contrast, in the fluid approach one starts from the ion continuity equation and substitutes the relevant ion $\boldsymbol {E}\times \boldsymbol {B}$![]() and polarization drift velocities (which are fluid and not guiding centre velocities) obtained from ion fluid momentum balance, and derives a closed equation for the electrostatic potential (see e.g. Hazeltine & Waelbroeck (Reference Hazeltine and Waelbroeck1998) on the treatment of drift motion within the fluid formalism). In this paper we follow the fluid formalism, sticking to the original derivation carried out by Hasegawa and Mima (Hasegawa & Mima Reference Hasegawa and Mima1977a), although we expect an equivalent construction to be possible within the gyrokinetic framework as well.
and polarization drift velocities (which are fluid and not guiding centre velocities) obtained from ion fluid momentum balance, and derives a closed equation for the electrostatic potential (see e.g. Hazeltine & Waelbroeck (Reference Hazeltine and Waelbroeck1998) on the treatment of drift motion within the fluid formalism). In this paper we follow the fluid formalism, sticking to the original derivation carried out by Hasegawa and Mima (Hasegawa & Mima Reference Hasegawa and Mima1977a), although we expect an equivalent construction to be possible within the gyrokinetic framework as well.
2. Electrostatic potential in curved magnetic fields
Consider a plasma system consisting of ions and electrons. Let $n_e$![]() denote the electron density. We assume that $n_e$
denote the electron density. We assume that $n_e$![]() follows a Boltzmann distribution with constant temperature $T_e>0$
follows a Boltzmann distribution with constant temperature $T_e>0$![]() :
:
Here, $A_e\left ({\boldsymbol {x}}\right )$![]() is a positive spatial function, $\phi$
is a positive spatial function, $\phi$![]() denotes the electric potential, $q=-e$
denotes the electric potential, $q=-e$![]() is the electron charge and $k_B$
is the electron charge and $k_B$![]() is the Boltzmann constant. It is convenient to introduce the constant
is the Boltzmann constant. It is convenient to introduce the constant
Let $\boldsymbol {B}=\boldsymbol {B}\left ({\boldsymbol {x}}\right )$![]() denote a static magnetic field. Recall that $\boldsymbol {B}$
denote a static magnetic field. Recall that $\boldsymbol {B}$![]() must be solenoidal, ${\boldsymbol {\nabla }}\boldsymbol {\cdot }\boldsymbol {B}=0$
must be solenoidal, ${\boldsymbol {\nabla }}\boldsymbol {\cdot }\boldsymbol {B}=0$![]() . In the following, we demand that $\boldsymbol {B}\neq 0$
. In the following, we demand that $\boldsymbol {B}\neq 0$![]() throughout the domain of interest $V$
throughout the domain of interest $V$![]() . Let $n$
. Let $n$![]() denote the ion density. Assuming quasi-neutrality, $n_e=Zn$
denote the ion density. Assuming quasi-neutrality, $n_e=Zn$![]() with $Z$
with $Z$![]() the number of protons in the ions. Using (2.1), the ion continuity equation reads
the number of protons in the ions. Using (2.1), the ion continuity equation reads
Here, $\boldsymbol {v}$![]() denotes the ion fluid velocity. On the other hand, denoting with $m$
denotes the ion fluid velocity. On the other hand, denoting with $m$![]() the ion mass, the ion fluid equation of motion can be written as
the ion mass, the ion fluid equation of motion can be written as
where $P$![]() represents pressure and $\boldsymbol {E}=-\boldsymbol {\nabla }\phi$
represents pressure and $\boldsymbol {E}=-\boldsymbol {\nabla }\phi$![]() the electric field. It is convenient to decompose the ion fluid velocity as
the electric field. It is convenient to decompose the ion fluid velocity as
where $\boldsymbol {v}_{\parallel }$![]() denotes the velocity component along the magnetic field and $\boldsymbol {v}_{\perp }$
denotes the velocity component along the magnetic field and $\boldsymbol {v}_{\perp }$![]() the perpendicular one. In the two-dimensional drift turbulence setting, the parallel velocity component is neglected because the time scale of fluctuations $t_d$
the perpendicular one. In the two-dimensional drift turbulence setting, the parallel velocity component is neglected because the time scale of fluctuations $t_d$![]() is faster than the time scale $t_b$
is faster than the time scale $t_b$![]() of ion dynamics along the magnetic field, $t_d\ll t_b$
of ion dynamics along the magnetic field, $t_d\ll t_b$![]() . In the following, we will therefore put
. In the following, we will therefore put
and discard the dynamics along $\boldsymbol {B}$![]() . In this context, it is of interest to study the long-term evolution of coherent structures. It is worth noticing that in the opposite regime $t_d\gg t_b$
. In this context, it is of interest to study the long-term evolution of coherent structures. It is worth noticing that in the opposite regime $t_d\gg t_b$![]() the parallel motion $\boldsymbol {v}_{\parallel }$
the parallel motion $\boldsymbol {v}_{\parallel }$![]() gets averaged out as well due to the fast bounce oscillation, although the polarization drift becomes neoclassically enhanced due to a large orbit size, leading to a neoclassically modified turbulence model with a dominant Hasegawa–Mima-type polarization nonlinearity (Hahm & Tang Reference Hahm and Tang1996). To proceed, we introduce a reference time scale $t_c$
gets averaged out as well due to the fast bounce oscillation, although the polarization drift becomes neoclassically enhanced due to a large orbit size, leading to a neoclassically modified turbulence model with a dominant Hasegawa–Mima-type polarization nonlinearity (Hahm & Tang Reference Hahm and Tang1996). To proceed, we introduce a reference time scale $t_c$![]() and a reference length scale $L_{\perp }$
and a reference length scale $L_{\perp }$![]() across $\boldsymbol {B}$
across $\boldsymbol {B}$![]() , and take $k_BT_e$
, and take $k_BT_e$![]() as reference value for the energy. In the standard drift turbulence ordering $t_c=2{\rm \pi} /\varOmega _c$
as reference value for the energy. In the standard drift turbulence ordering $t_c=2{\rm \pi} /\varOmega _c$![]() , with $\varOmega _c=ZeB/m$
, with $\varOmega _c=ZeB/m$![]() the ion cyclotron frequency, while $L_{\perp }$
the ion cyclotron frequency, while $L_{\perp }$![]() corresponds to the sound radius $\rho _s=c_s\varOmega _{c}^{-1}\sim k_{\perp }^{-1}$
corresponds to the sound radius $\rho _s=c_s\varOmega _{c}^{-1}\sim k_{\perp }^{-1}$![]() , where $c_s=\left ({Zk_BT_e/m}\right )^{1/2}$
, where $c_s=\left ({Zk_BT_e/m}\right )^{1/2}$![]() is the sound speed and $k_{\perp }$
is the sound speed and $k_{\perp }$![]() a characteristic perpendicular wavenumber for the turbulence. In this study we further consider a regime of plasma where the electric potential energy is small compared with the electron kinetic energy, and the ratio between the component of the electric field perpendicular to the magnetic field and the magnetic field itself is small:
a characteristic perpendicular wavenumber for the turbulence. In this study we further consider a regime of plasma where the electric potential energy is small compared with the electron kinetic energy, and the ratio between the component of the electric field perpendicular to the magnetic field and the magnetic field itself is small:
where $\epsilon \ll 1$![]() is a small ordering parameter, $E_{\perp }=\left \lvert {\boldsymbol {E}_{\perp }}\right \rvert$
is a small ordering parameter, $E_{\perp }=\left \lvert {\boldsymbol {E}_{\perp }}\right \rvert$![]() , with $\boldsymbol {E}_{\perp }$
, with $\boldsymbol {E}_{\perp }$![]() the component of the electric field $\boldsymbol {E}$
the component of the electric field $\boldsymbol {E}$![]() perpendicular to $\boldsymbol {B}$
perpendicular to $\boldsymbol {B}$![]() , and $B=\left \lvert {\boldsymbol {B}}\right \rvert$
, and $B=\left \lvert {\boldsymbol {B}}\right \rvert$![]() . The remaining perpendicular component $\boldsymbol {v}_{\perp }$
. The remaining perpendicular component $\boldsymbol {v}_{\perp }$![]() can be expanded into a first-order term $\boldsymbol {v}_{1}$
can be expanded into a first-order term $\boldsymbol {v}_{1}$![]() , which will correspond to first-order $\boldsymbol {E}\times \boldsymbol {B}$
, which will correspond to first-order $\boldsymbol {E}\times \boldsymbol {B}$![]() drift dynamics, a second-order term $\boldsymbol {v}_{2}$
drift dynamics, a second-order term $\boldsymbol {v}_{2}$![]() , which will be identified with second-order $\boldsymbol {E}\times \boldsymbol {B}$
, which will be identified with second-order $\boldsymbol {E}\times \boldsymbol {B}$![]() drift dynamics plus the polarization drift encountered in guiding centre theory, and higher-order corrections. At second order in $\epsilon$
drift dynamics plus the polarization drift encountered in guiding centre theory, and higher-order corrections. At second order in $\epsilon$![]() we therefore have
we therefore have
To proceed, a further working assumption is needed on the rate of change of $\boldsymbol {v}$![]() , which may only evolve on time scales long relative to a gyroperiod:
, which may only evolve on time scales long relative to a gyroperiod:
Recalling the decomposition (2.8), (2.9) leads to
Furthermore, we enforce the cold ion hypothesis $T=0$![]() , with $T$
, with $T$![]() the ion temperature, implying that ions are not subject to thermal fluctuations, and therefore
the ion temperature, implying that ions are not subject to thermal fluctuations, and therefore
To obtain expressions for $\boldsymbol {v}_{1}$![]() and $\boldsymbol {v}_{2}$
and $\boldsymbol {v}_{2}$![]() , consider again the equation of motion (2.4), which at second order in $\epsilon$
, consider again the equation of motion (2.4), which at second order in $\epsilon$![]() now reads as
now reads as
Here, we used (2.10a,b) to extract the order of $\partial \boldsymbol {v}_{1}/\partial t$![]() according to $\partial \boldsymbol {v}_{1}/\partial t\rightarrow \epsilon \partial \boldsymbol {v}_1/\partial t$
according to $\partial \boldsymbol {v}_{1}/\partial t\rightarrow \epsilon \partial \boldsymbol {v}_1/\partial t$![]() . In addition, the electric potential $\phi$
. In addition, the electric potential $\phi$![]() and electric field $\boldsymbol {E}$
and electric field $\boldsymbol {E}$![]() have been decomposed into first-order and second-order components according to
have been decomposed into first-order and second-order components according to
Hence, the cross product of (2.12) with $\boldsymbol {B}/B^2$![]() can be cast in the form
can be cast in the form

where we introduced the physical constant
Notice that all terms on the left-hand side of (2.14) are first order in $\epsilon$![]() , while those on the right-hand side are second order. The first-order $\boldsymbol {E}\times \boldsymbol {B}$
, while those on the right-hand side are second order. The first-order $\boldsymbol {E}\times \boldsymbol {B}$![]() flow $\boldsymbol {v}_{\boldsymbol {E}_1}$
flow $\boldsymbol {v}_{\boldsymbol {E}_1}$![]() can be obtained from the left-hand side:
can be obtained from the left-hand side:
Similarly, the second-order fluid drift is given by

Evidently, the second term on the right-hand side is a second-order $\boldsymbol {E}\times \boldsymbol {B}$![]() drift $\boldsymbol {v}_{\boldsymbol {E}_2}$
drift $\boldsymbol {v}_{\boldsymbol {E}_2}$![]() , while the first term is nothing but a polarization drift $\boldsymbol {v}_{\rm pol}$
, while the first term is nothing but a polarization drift $\boldsymbol {v}_{\rm pol}$![]() (see Hazeltine & Waelbroeck Reference Hazeltine and Waelbroeck1998). Indeed, in a straight magnetic field $\boldsymbol {B}=B_0\boldsymbol {\nabla } z$
(see Hazeltine & Waelbroeck Reference Hazeltine and Waelbroeck1998). Indeed, in a straight magnetic field $\boldsymbol {B}=B_0\boldsymbol {\nabla } z$![]() with $B_0\in \mathbb {R}$
with $B_0\in \mathbb {R}$![]() one has
one has

with $\boldsymbol {E}_{1\perp }$![]() the component of $\boldsymbol {E}_1$
the component of $\boldsymbol {E}_1$![]() perpendicular to $\boldsymbol {B}$
perpendicular to $\boldsymbol {B}$![]() . Combining equations (2.16) and (2.17), the total ion velocity has the expression
. Combining equations (2.16) and (2.17), the total ion velocity has the expression

where we introduced the orthogonal gradient operator
with $f$![]() some function. Then, we have
some function. Then, we have

Defining the total $\boldsymbol {E}\times \boldsymbol {B}$![]() drift velocity as
drift velocity as
at second order equation (2.19) can be expressed solely in terms of $\phi$![]() as
as

We remark that this same result can also be obtained by a simple iteration method where, regarding the left-hand side of (2.4) as a smaller term than the right-hand side, we have the lowest-order solution as $\boldsymbol {v}=\boldsymbol {v}_{\boldsymbol {E}}$![]() and the next-order solution as given by (2.23). Similarly, in terms of $\phi$
and the next-order solution as given by (2.23). Similarly, in terms of $\phi$![]() , (2.21) can be written as
, (2.21) can be written as

On the other hand, the term
appearing on the right-hand side of (2.3) is a third-order contribution that can be neglected. Using (2.24) and (2.25) to evaluate (2.3), at second order we thus obtain
To check the consistency of the ordering assumptions leading to (2.26) we must verify that the ion fluid conservation laws are satisfied by the derived equation. First consider the total mass
In this notation $V\subset \mathbb {R}^3$![]() is a bounded domain occupied by the plasma and ${\rm d}V$
is a bounded domain occupied by the plasma and ${\rm d}V$![]() the volume element in $\mathbb {R}^3$
the volume element in $\mathbb {R}^3$![]() . The constancy of $M$
. The constancy of $M$![]() follows immediately by noting that at leading order in $\phi$
follows immediately by noting that at leading order in $\phi$![]()
Then, using (2.26) and (2.23) we have
where $\boldsymbol {n}$![]() is the unit outward normal to the boundary $\partial V$
is the unit outward normal to the boundary $\partial V$![]() , ${\rm d}S$
, ${\rm d}S$![]() is the surface element on $\partial V$
is the surface element on $\partial V$![]() and $\boldsymbol {v}$
and $\boldsymbol {v}$![]() is given by (2.23). This boundary integral vanishes if the system is periodic or the fluid velocity $\boldsymbol {v}$
is given by (2.23). This boundary integral vanishes if the system is periodic or the fluid velocity $\boldsymbol {v}$![]() is tangential to the bounding surface, $\boldsymbol {v}\boldsymbol {\cdot }\boldsymbol {n}=0$
is tangential to the bounding surface, $\boldsymbol {v}\boldsymbol {\cdot }\boldsymbol {n}=0$![]() on $\partial V$
on $\partial V$![]() , or $A_e=0$
, or $A_e=0$![]() on $\partial V$
on $\partial V$![]() . Next, consider the fluid energy
. Next, consider the fluid energy
To study conservation of energy it is convenient to subtract from $E_V$![]() the total mass $M_V$
the total mass $M_V$![]() and define a new quantity:
and define a new quantity:
At leading order in $\phi$![]() we therefore obtain
we therefore obtain
Since $A_e\left ({\boldsymbol {x}}\right )$![]() is independent of time, the quantity $H_V$
is independent of time, the quantity $H_V$![]() can be further simplified to
can be further simplified to

Using (2.26), the rate of change in $H_V$![]() is
is

Here, $\boldsymbol {v}$![]() is given by (2.23) and the notation $\phi _t=\partial \phi /\partial t$
is given by (2.23) and the notation $\phi _t=\partial \phi /\partial t$![]() has been used. Notice that boundary integrals vanish if the system is periodic or $\boldsymbol {v}\boldsymbol {\cdot }\boldsymbol {n}=0$
has been used. Notice that boundary integrals vanish if the system is periodic or $\boldsymbol {v}\boldsymbol {\cdot }\boldsymbol {n}=0$![]() on $\partial V$
on $\partial V$![]() or $A_e\phi =0$
or $A_e\phi =0$![]() on $\partial V$
on $\partial V$![]() .
.
However, the last term on the right-hand side cannot be written as a boundary integral. Hence, an additional ordering condition is needed to ensure that energy is preserved. This term originates from the quantity
appearing on the right-hand side of (2.26). We therefore demand that
Observe that the scaling above reflects the degree of accuracy of the ordering assumption $t^2_c L_{\perp }^{-1}\partial \boldsymbol {v}_{2}/\partial t\sim \epsilon$![]() adopted in (2.10a,b) which led to the omission of this term from fluid momentum balance. Recalling that $\boldsymbol {v}_{\boldsymbol {E}}^2$
adopted in (2.10a,b) which led to the omission of this term from fluid momentum balance. Recalling that $\boldsymbol {v}_{\boldsymbol {E}}^2$![]() is second order in $\epsilon$
is second order in $\epsilon$![]() , if the density gradient is small $L_{\perp }\left \lvert {\boldsymbol {\nabla }_{\perp }A_e}\right \rvert /A_e\sim \epsilon$
, if the density gradient is small $L_{\perp }\left \lvert {\boldsymbol {\nabla }_{\perp }A_e}\right \rvert /A_e\sim \epsilon$![]() the condition (2.36) is automatically satisfied for those magnetic fields such that $B_0L_{\perp }\left \lvert {\boldsymbol {\nabla }\times \left ({B^{-2}\boldsymbol {B}}\right )}\right \rvert \sim \epsilon$
the condition (2.36) is automatically satisfied for those magnetic fields such that $B_0L_{\perp }\left \lvert {\boldsymbol {\nabla }\times \left ({B^{-2}\boldsymbol {B}}\right )}\right \rvert \sim \epsilon$![]() , with $B_0\in \mathbb {R}$
, with $B_0\in \mathbb {R}$![]() a reference magnetic field, or equivalently
a reference magnetic field, or equivalently
for some potential $\zeta$![]() and vector field $\boldsymbol {\xi }$
and vector field $\boldsymbol {\xi }$![]() . This condition implies that the quantity $\boldsymbol {B}/B^2$
. This condition implies that the quantity $\boldsymbol {B}/B^2$![]() does not depart largely from a potential field, physically implying that the divergence of the $\boldsymbol {E}\times \boldsymbol {B}$
does not depart largely from a potential field, physically implying that the divergence of the $\boldsymbol {E}\times \boldsymbol {B}$![]() velocity scales as $t_c\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {v}_{\boldsymbol {E}}=t_c\epsilon \boldsymbol {\nabla }\times \boldsymbol {\xi }\boldsymbol {\cdot }\boldsymbol {\nabla }\phi \sim \epsilon ^2$
velocity scales as $t_c\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {v}_{\boldsymbol {E}}=t_c\epsilon \boldsymbol {\nabla }\times \boldsymbol {\xi }\boldsymbol {\cdot }\boldsymbol {\nabla }\phi \sim \epsilon ^2$![]() . This is the case of the standard Hasegawa–Mima equation, where $L_{\perp }\left \lvert {\boldsymbol {\nabla }_{\perp } A_e}\right \rvert /A_e\sim \epsilon$
. This is the case of the standard Hasegawa–Mima equation, where $L_{\perp }\left \lvert {\boldsymbol {\nabla }_{\perp } A_e}\right \rvert /A_e\sim \epsilon$![]() and (2.36) is satisfied with $\zeta =B_0^{-1}z$
and (2.36) is satisfied with $\zeta =B_0^{-1}z$![]() , $B_0\in \mathbb {R}$
, $B_0\in \mathbb {R}$![]() and $\boldsymbol {\xi }=\boldsymbol {0}$
and $\boldsymbol {\xi }=\boldsymbol {0}$![]() so that $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {v}_{\boldsymbol {E}}=0$
so that $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {v}_{\boldsymbol {E}}=0$![]() . An alternative way to fulfil (2.36) is to assume that
. An alternative way to fulfil (2.36) is to assume that
implying a mild change in $\boldsymbol {E}\times \boldsymbol {B}$![]() drift velocity across $\boldsymbol {B}$
drift velocity across $\boldsymbol {B}$![]() as in the case for large-scale coherent structures studied in § 3.
as in the case for large-scale coherent structures studied in § 3.
Once the prescription (2.36) is enforced, the last term in (2.26) can be neglected since it scales as $\epsilon ^3$![]() , and we obtain a consistent equation for the potential $\phi$
, and we obtain a consistent equation for the potential $\phi$![]() ,
,
which preserves the total mass (2.28) and the energy (2.33) exactly under suitable boundary conditions. In particular, one obtains
with the effective ion fluid velocity
The conditions on magnetic field topology for the existence of an additional conserved quantity (generalized enstrophy) are discussed in § 4.
We suggest that (2.39) is appropriate to describe electrostatic turbulence in magnetic fields with arbitrary topology. In particular, considering the ordering condition (2.36), the equation is best suited for magnetic fields of the type (2.37) so that $t_c\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {v}_{\boldsymbol {E}}\sim \epsilon ^2$![]() , or for configurations (2.38) such that the spatial change in $\boldsymbol {E}\times \boldsymbol {B}$
, or for configurations (2.38) such that the spatial change in $\boldsymbol {E}\times \boldsymbol {B}$![]() drift velocity scales as $t^2_cL_{\perp }^{-1}\boldsymbol {\nabla }\boldsymbol {v}_{\boldsymbol {E}}^2\sim \epsilon ^3$
drift velocity scales as $t^2_cL_{\perp }^{-1}\boldsymbol {\nabla }\boldsymbol {v}_{\boldsymbol {E}}^2\sim \epsilon ^3$![]() .
.
It should be noted that (2.39) can be derived from the standard drift turbulence ordering $e\phi /k_BT_e\sim \varOmega _c^{-1}\partial /\partial t\sim \epsilon$![]() , $k_\perp L_{\perp }'\sim k_{\perp }L_{n_e}\sim k_{\perp }/k_{\parallel }\sim \epsilon ^{-1}$
, $k_\perp L_{\perp }'\sim k_{\perp }L_{n_e}\sim k_{\perp }/k_{\parallel }\sim \epsilon ^{-1}$![]() and $k_{\perp }\rho _s\sim 1$
and $k_{\perp }\rho _s\sim 1$![]() , where $L_{\perp }'$
, where $L_{\perp }'$![]() is a large perpendicular scale associated with the spatial change in $\boldsymbol {B}$
is a large perpendicular scale associated with the spatial change in $\boldsymbol {B}$![]() , $L_{n_e}\sim A_e/\left \lvert {\boldsymbol {\nabla }_{\perp } A_e}\right \rvert$
, $L_{n_e}\sim A_e/\left \lvert {\boldsymbol {\nabla }_{\perp } A_e}\right \rvert$![]() the characteristic spatial scale associated with the density $n_e$
the characteristic spatial scale associated with the density $n_e$![]() , $k_{\parallel }$
, $k_{\parallel }$![]() a characteristic wavenumber along the magnetic field, $\rho _s=c_sm/ZeB$
a characteristic wavenumber along the magnetic field, $\rho _s=c_sm/ZeB$![]() the sound radius and $c_s=(Zk_BT_e/m)^{1/2}$
the sound radius and $c_s=(Zk_BT_e/m)^{1/2}$![]() the sound speed. With such ordering the last term in (2.26) can be discarded from the outset, being a third-order contribution. However, this comes at the price of additional constraints on the spatial change allowed for $\boldsymbol {B}$
the sound speed. With such ordering the last term in (2.26) can be discarded from the outset, being a third-order contribution. However, this comes at the price of additional constraints on the spatial change allowed for $\boldsymbol {B}$![]() , since all its spatial derivatives $L_{\perp }\nabla \sim 1/k_{\perp }L_{\perp }'\sim \epsilon$
, since all its spatial derivatives $L_{\perp }\nabla \sim 1/k_{\perp }L_{\perp }'\sim \epsilon$![]() must be small.
must be small.
Regarding the physical interpretation of the terms appearing in the equation, it is worth observing that the quantity $\boldsymbol {\nabla }\boldsymbol {\cdot }\left ({B^{-2}\boldsymbol {\nabla }_{\perp }\phi }\right )$![]() arises from the component of the vorticity $\boldsymbol {\nabla }\times \boldsymbol {v}_{\boldsymbol {E}}$
arises from the component of the vorticity $\boldsymbol {\nabla }\times \boldsymbol {v}_{\boldsymbol {E}}$![]() along $\boldsymbol {B}$
along $\boldsymbol {B}$![]() . Indeed,
. Indeed,
In addition, a term $\boldsymbol {\nabla }\boldsymbol {\cdot }\left ({nB^{-2}\boldsymbol {\nabla }_{\perp }\phi }\right )$![]() is often encountered in modern gyrokinetic and gyrofluid theories as the ion polarization charge density in Poisson's equation for the electric potential (Strinzi & Scott Reference Strinzi and Scott2004; Hahm, Wang & Madsen Reference Hahm, Wang and Madsen2009). The factor $1-\sigma B^{-2} \boldsymbol {B}\boldsymbol {\cdot }\boldsymbol {\nabla }\times \boldsymbol {v}_{\boldsymbol {E}}$
is often encountered in modern gyrokinetic and gyrofluid theories as the ion polarization charge density in Poisson's equation for the electric potential (Strinzi & Scott Reference Strinzi and Scott2004; Hahm, Wang & Madsen Reference Hahm, Wang and Madsen2009). The factor $1-\sigma B^{-2} \boldsymbol {B}\boldsymbol {\cdot }\boldsymbol {\nabla }\times \boldsymbol {v}_{\boldsymbol {E}}$![]() on the right-hand side of (2.39) is similarly predicted by gyrokinetic theory as a correction caused by the polarization charge-like term to the effective magnetic field strength:
on the right-hand side of (2.39) is similarly predicted by gyrokinetic theory as a correction caused by the polarization charge-like term to the effective magnetic field strength:
which corresponds to the Jacobian determinant of the guiding centre phase space when parallel dynamics is ignored. This correction causes a net drift velocity (see equation (4) of Hahm (Reference Hahm1996) or the discussion in Littlejohn (Reference Littlejohn1981)):

3. Limit to the Hasegawa–Mima equation and curvature effects
In this section we show that (2.39) reduces to the Hasegawa–Mima equation (Hasegawa & Mima Reference Hasegawa and Mima1977a) when the magnetic field is straight, $\boldsymbol {B}=B_0\boldsymbol {\nabla } z$![]() with $B_0\in \mathbb {R}$
with $B_0\in \mathbb {R}$![]() . In the remainder of this paper, we restrict our attention to the constant density case $A_e\in \mathbb {R}$
. In the remainder of this paper, we restrict our attention to the constant density case $A_e\in \mathbb {R}$![]() . In tokamak turbulence this condition can occur in the core of H-mode plasmas (Bernert et al. Reference Bernert, Eich, Kallenbach, Carralero, Huber, Lang, Potzel, Reimold, Schweinzer and Viezzer2015) where density is often well approximated by a flat profile.
. In tokamak turbulence this condition can occur in the core of H-mode plasmas (Bernert et al. Reference Bernert, Eich, Kallenbach, Carralero, Huber, Lang, Potzel, Reimold, Schweinzer and Viezzer2015) where density is often well approximated by a flat profile.
Before proceeding further, it should be noted that in many cases of practical interest the magnetic field varies on spatial scales $L_{\perp }$![]() that are larger than the sound radius $\rho _s$
that are larger than the sound radius $\rho _s$![]() , i.e. $\rho _s/L_{\perp }\sim \epsilon$
, i.e. $\rho _s/L_{\perp }\sim \epsilon$![]() . If both magnetic field and electric potential vary on the macroscopic spatial scale $L_{\perp }$
. If both magnetic field and electric potential vary on the macroscopic spatial scale $L_{\perp }$![]() , the terms $\boldsymbol {\nabla }\boldsymbol {\cdot }\left ({A_e\boldsymbol {v}_{\boldsymbol {E}}}\right )$
, the terms $\boldsymbol {\nabla }\boldsymbol {\cdot }\left ({A_e\boldsymbol {v}_{\boldsymbol {E}}}\right )$![]() and $\boldsymbol {\nabla }\boldsymbol {\cdot }\left ({({\sigma }/{2})({A_e\boldsymbol {B}}/{B^2})\times \boldsymbol {\nabla } \boldsymbol {v}_{\boldsymbol {E}}^2}\right )$
and $\boldsymbol {\nabla }\boldsymbol {\cdot }\left ({({\sigma }/{2})({A_e\boldsymbol {B}}/{B^2})\times \boldsymbol {\nabla } \boldsymbol {v}_{\boldsymbol {E}}^2}\right )$![]() on the right-hand side of (2.26) become comparable third-order terms because
on the right-hand side of (2.26) become comparable third-order terms because

Hence, in this scenario the ordering breaks down. However, physical settings with $\rho _s/L_{\perp }\sim \epsilon$![]() can still be handled by invoking the standard Hasegawa–Mima ordering (which leads to the same (2.39) as previously explained) where the magnetic field changes on the large spatial scale $L_{\perp }$
can still be handled by invoking the standard Hasegawa–Mima ordering (which leads to the same (2.39) as previously explained) where the magnetic field changes on the large spatial scale $L_{\perp }$![]() , while the electric potential is characterized by the smaller scale length $\rho _s$
, while the electric potential is characterized by the smaller scale length $\rho _s$![]() .
.
To elucidate how a curved inhomogeneous magnetic field modifies the Hasegawa–Mima equation, it is convenient to study (2.39) in the limit of a magnetic field of the type
where $\epsilon _{B}\ll 1$![]() is an ordering parameter and $\boldsymbol {a}=\left ({a_x,a_y,a_z}\right )$
is an ordering parameter and $\boldsymbol {a}=\left ({a_x,a_y,a_z}\right )$![]() a vector field such that $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {a}=0$
a vector field such that $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {a}=0$![]() . For simplicity, we assume that $a_z=\boldsymbol {a}\boldsymbol {\cdot }\boldsymbol {\nabla } z=0$
. For simplicity, we assume that $a_z=\boldsymbol {a}\boldsymbol {\cdot }\boldsymbol {\nabla } z=0$![]() . Notice that the magnetic field (3.2) satisfies (2.37) and thus (2.36) as well. Furthermore, at the first order in $\epsilon _B$
. Notice that the magnetic field (3.2) satisfies (2.37) and thus (2.36) as well. Furthermore, at the first order in $\epsilon _B$![]() the curvature of the magnetic field (3.2) is given by
the curvature of the magnetic field (3.2) is given by
It is convenient to introduce the two-dimensional gradient and Laplacian operators:
where $f=f\left ({x,y,z}\right )$![]() is some function. Then, at first order in $\epsilon _B$
is some function. Then, at first order in $\epsilon _B$![]() we have
we have
Hence, defining the bracket
with $f,g$![]() some functions, (2.39) becomes
some functions, (2.39) becomes

Recalling (3.3), we see that the third term on the left-hand side of (3.7) arises from the curvature of the magnetic field. Terms involving ${\partial \phi }/{\partial z}$![]() describe the effect of the inhomogeneity of the electric potential along the vertical axis. The term including $\boldsymbol {\nabla }\times \boldsymbol {B}=\epsilon _B\boldsymbol {\nabla }\times \boldsymbol {a}$
describe the effect of the inhomogeneity of the electric potential along the vertical axis. The term including $\boldsymbol {\nabla }\times \boldsymbol {B}=\epsilon _B\boldsymbol {\nabla }\times \boldsymbol {a}$![]() can be ascribed to the presence of electric current in the system. Finally, the last term on the right-hand side results from the polarization drift associated with the component of the magnetic field $\epsilon _B\boldsymbol {a}$
can be ascribed to the presence of electric current in the system. Finally, the last term on the right-hand side results from the polarization drift associated with the component of the magnetic field $\epsilon _B\boldsymbol {a}$![]() . Observe that (3.7) reduces to the Hasegawa–Mima equation when $\epsilon _B=0$
. Observe that (3.7) reduces to the Hasegawa–Mima equation when $\epsilon _B=0$![]() :
:
The effect of the field curvature (3.3) can be made explicit by expanding $\boldsymbol {a}$![]() in Taylor series around $z=0$
in Taylor series around $z=0$![]() , and by considering the dynamics on such a plane. At first order in $z$
, and by considering the dynamics on such a plane. At first order in $z$![]() , one has
, one has
where $\boldsymbol {a}_0=\left ({a_{0x},a_{0y},0}\right )$![]() and $\boldsymbol {a}_{1}=\left ({a_{1x},a_{1x},0}\right )$
and $\boldsymbol {a}_{1}=\left ({a_{1x},a_{1x},0}\right )$![]() are vector fields independent of $z$
are vector fields independent of $z$![]() . Using (3.9a,b) and setting ${\partial \phi }/{\partial z}=0$
. Using (3.9a,b) and setting ${\partial \phi }/{\partial z}=0$![]() , all terms in (3.7) lose the dependence on $z$
, all terms in (3.7) lose the dependence on $z$![]() , giving a two-dimensional equation:
, giving a two-dimensional equation:

In many applications, the curvature of the magnetic field is not small. It is therefore instructive to examine how the Hasegawa–Mima equation is modified when curvature is a leading-order term. To this end, consider a circular magnetic field $\boldsymbol {B}= B_0 r\boldsymbol {\nabla }\varphi$![]() , where $\left ({r,\varphi,z}\right )$
, where $\left ({r,\varphi,z}\right )$![]() are cylindrical coordinates and $B_0\in \mathbb {R}$
are cylindrical coordinates and $B_0\in \mathbb {R}$![]() . Notice that $\boldsymbol {B}^2= B_0^2$
. Notice that $\boldsymbol {B}^2= B_0^2$![]() is constant (unlike the usual dependence $\left \lvert {\nabla \varphi }\right \rvert ^2=1/r^2$
is constant (unlike the usual dependence $\left \lvert {\nabla \varphi }\right \rvert ^2=1/r^2$![]() ), and that the curvature of the magnetic field is given by $\boldsymbol {\kappa }=-\boldsymbol {\nabla }\log r$
), and that the curvature of the magnetic field is given by $\boldsymbol {\kappa }=-\boldsymbol {\nabla }\log r$![]() . Assuming that the condition (2.36), which in this case can be explicitly written as $r^{-1}\partial \left \lvert {\boldsymbol {\nabla }_{\perp }\phi }\right \rvert ^2/\partial z\sim \epsilon ^3$
. Assuming that the condition (2.36), which in this case can be explicitly written as $r^{-1}\partial \left \lvert {\boldsymbol {\nabla }_{\perp }\phi }\right \rvert ^2/\partial z\sim \epsilon ^3$![]() , is initially satisfied by the electric potential $\phi$
, is initially satisfied by the electric potential $\phi$![]() , (2.39) can be written as
, (2.39) can be written as
where we introduced the differential operators
Notice that the field curvature modifies the Hasegawa–Mima equation through the modulus $\kappa ^2=1/r^2$![]() , and that (3.11) is two-dimensional if axial symmetry $\partial \phi /\partial \varphi =0$
, and that (3.11) is two-dimensional if axial symmetry $\partial \phi /\partial \varphi =0$![]() is assumed. Furthermore, since we expect the electric potential $\phi$
is assumed. Furthermore, since we expect the electric potential $\phi$![]() , the electric field $-\boldsymbol {\nabla }_{\left ({z,r}\right )}\phi$
, the electric field $-\boldsymbol {\nabla }_{\left ({z,r}\right )}\phi$![]() and the electric charge $-\Delta _{\left ({z,r}\right )}\phi$
and the electric charge $-\Delta _{\left ({z,r}\right )}\phi$![]() to be bounded, the Hasegawa–Mima equation can be recovered in the limit $r\rightarrow \infty$
to be bounded, the Hasegawa–Mima equation can be recovered in the limit $r\rightarrow \infty$![]() ($\kappa \rightarrow 0$
($\kappa \rightarrow 0$![]() ) where field lines become progressively straight. Next, observe that in the Hasegawa–Mima equation (3.8) steady states are given by the equation $[\phi,\Delta _{\left ({x,y}\right )}\phi ]=0$
) where field lines become progressively straight. Next, observe that in the Hasegawa–Mima equation (3.8) steady states are given by the equation $[\phi,\Delta _{\left ({x,y}\right )}\phi ]=0$![]() , or simply $\Delta _{\left ({x,y}\right )}\phi =-f\left ({\phi }\right )$
, or simply $\Delta _{\left ({x,y}\right )}\phi =-f\left ({\phi }\right )$![]() with $f$
with $f$![]() some function of $\phi$
some function of $\phi$![]() . Since the electric charge $-\Delta _{\left ({x,y}\right )}\phi$
. Since the electric charge $-\Delta _{\left ({x,y}\right )}\phi$![]() should vanish when $\phi =0$
should vanish when $\phi =0$![]() , for small $\phi$
, for small $\phi$![]() we may set $f=f_0\phi$
we may set $f=f_0\phi$![]() with $f_0$
with $f_0$![]() a positive real constant (the positive sign physically means that charge density gradients $-\boldsymbol {\nabla }_{\left ({x,y}\right )}\Delta _{\left ({x,y}\right )}\phi$
a positive real constant (the positive sign physically means that charge density gradients $-\boldsymbol {\nabla }_{\left ({x,y}\right )}\Delta _{\left ({x,y}\right )}\phi$![]() are directed in the opposite direction to the electric field $-\boldsymbol {\nabla }_{\left ({x,y}\right )}\phi$
are directed in the opposite direction to the electric field $-\boldsymbol {\nabla }_{\left ({x,y}\right )}\phi$![]() ). Then, considering a two-dimensional domain $\left ({x,y}\right )\in V=[0,{\rm \pi} ]^2$
). Then, considering a two-dimensional domain $\left ({x,y}\right )\in V=[0,{\rm \pi} ]^2$![]() such that the electric potential is grounded on the boundary, i.e. $\phi =0$
such that the electric potential is grounded on the boundary, i.e. $\phi =0$![]() on $\partial V$
on $\partial V$![]() , solution of the steady Hasegawa–Mima equation gives self-organized states of the type $\phi =\phi _0\sin \left ({m x}\right )\sin \left ({n y}\right )$
, solution of the steady Hasegawa–Mima equation gives self-organized states of the type $\phi =\phi _0\sin \left ({m x}\right )\sin \left ({n y}\right )$![]() with $\phi _0\in \mathbb {R}$
with $\phi _0\in \mathbb {R}$![]() , $m,n\in \mathbb {Z}$
, $m,n\in \mathbb {Z}$![]() and $f_0=m^2+n^2$
and $f_0=m^2+n^2$![]() . Since $f_0$
. Since $f_0$![]() corresponds to the ratio between enstrophy and energy in the fluid limit $\lambda =0$
corresponds to the ratio between enstrophy and energy in the fluid limit $\lambda =0$![]() , at equilibrium the minimum possible value $f_0=2$
, at equilibrium the minimum possible value $f_0=2$![]() corresponding to $m=n=1$
corresponding to $m=n=1$![]() is expected to be preferentially selected (see e.g. Hasegawa (Reference Hasegawa2004) on this point). Let us see how the situation changes in a curved magnetic field. Since the right-hand side of (3.11) can be written as
is expected to be preferentially selected (see e.g. Hasegawa (Reference Hasegawa2004) on this point). Let us see how the situation changes in a curved magnetic field. Since the right-hand side of (3.11) can be written as
steady states in the circular magnetic field $\boldsymbol {B}= B_0 r\boldsymbol {\nabla }\phi$![]() are described by the equation
are described by the equation
Considering the case $f=f_0\phi$![]() and taking a two-dimensional toroidal domain with squared cross-section $\left ({r,z}\right )\in V=[1,2]^2$
and taking a two-dimensional toroidal domain with squared cross-section $\left ({r,z}\right )\in V=[1,2]^2$![]() and Dirichlet boundary conditions $\phi =0$
and Dirichlet boundary conditions $\phi =0$![]() on the boundary $\partial V$
on the boundary $\partial V$![]() , solution of (3.14) results in a self-organized steady state sustained by the curvature of the magnetic field. Figure 1 shows contour plots of the steady electric potential $\phi$
, solution of (3.14) results in a self-organized steady state sustained by the curvature of the magnetic field. Figure 1 shows contour plots of the steady electric potential $\phi$![]() and vorticity $\omega =\boldsymbol {\nabla }\times \boldsymbol {v}\boldsymbol {\cdot } r\boldsymbol {\nabla }\varphi$
and vorticity $\omega =\boldsymbol {\nabla }\times \boldsymbol {v}\boldsymbol {\cdot } r\boldsymbol {\nabla }\varphi$![]() with $\boldsymbol {v}$
with $\boldsymbol {v}$![]() given by (2.41) obtained by numerical solution of (3.14) as compared with the Hasegawa–Mima case. The corresponding fluid drifts $\boldsymbol {v}_{\boldsymbol {E}}$
given by (2.41) obtained by numerical solution of (3.14) as compared with the Hasegawa–Mima case. The corresponding fluid drifts $\boldsymbol {v}_{\boldsymbol {E}}$![]() and $\boldsymbol {v}$
and $\boldsymbol {v}$![]() are given in figure 2. From these figures, one sees that contours of electric potential $\phi$
are given in figure 2. From these figures, one sees that contours of electric potential $\phi$![]() , vorticity $\omega$
, vorticity $\omega$![]() and fluid drifts $\left \lvert {\boldsymbol {v}_{\boldsymbol {E}}}\right \rvert$
and fluid drifts $\left \lvert {\boldsymbol {v}_{\boldsymbol {E}}}\right \rvert$![]() and $\left \lvert {\boldsymbol {v}}\right \rvert$
and $\left \lvert {\boldsymbol {v}}\right \rvert$![]() tend to accumulate in regions of higher curvature (small radius $r$
tend to accumulate in regions of higher curvature (small radius $r$![]() ), implying the onset of steeper gradients, while inhomogeneities are suppressed where the curvature is weaker. Figure 2 also reveals that the fluid drifts $\boldsymbol {v}_{\boldsymbol {E}}$
), implying the onset of steeper gradients, while inhomogeneities are suppressed where the curvature is weaker. Figure 2 also reveals that the fluid drifts $\boldsymbol {v}_{\boldsymbol {E}}$![]() and $\boldsymbol {v}$
and $\boldsymbol {v}$![]() are enhanced where the curvature is stronger. We remark that the observed behaviour is not caused by the gradient or curvature drifts of the guiding centre framework because the cold ions approximation is being considered. In addition, the background magnetic field has uniform strength in this example. Hence, curvature affects self-organized states through the $\boldsymbol {E}\times \boldsymbol {B}$
are enhanced where the curvature is stronger. We remark that the observed behaviour is not caused by the gradient or curvature drifts of the guiding centre framework because the cold ions approximation is being considered. In addition, the background magnetic field has uniform strength in this example. Hence, curvature affects self-organized states through the $\boldsymbol {E}\times \boldsymbol {B}$![]() drift, as clear from (2.39).
drift, as clear from (2.39).

Figure 1. (a,b) Contour plots of the self-organized steady electric potential $\phi$![]() in a straight magnetic field $\boldsymbol {B}=B_0\boldsymbol {\nabla } z$
in a straight magnetic field $\boldsymbol {B}=B_0\boldsymbol {\nabla } z$![]() (Hasegawa–Mima equation) and in a circular magnetic field $\boldsymbol {B}= B_0 r\boldsymbol {\nabla }\varphi$
(Hasegawa–Mima equation) and in a circular magnetic field $\boldsymbol {B}= B_0 r\boldsymbol {\nabla }\varphi$![]() . (c,d) Contour plots of the respective vorticities $\omega =\boldsymbol {\nabla }\times \boldsymbol {v}\boldsymbol {\cdot }\boldsymbol {\nabla } z$
. (c,d) Contour plots of the respective vorticities $\omega =\boldsymbol {\nabla }\times \boldsymbol {v}\boldsymbol {\cdot }\boldsymbol {\nabla } z$![]() and $\omega =\boldsymbol {\nabla }\times \boldsymbol {v}\boldsymbol {\cdot } r\boldsymbol {\nabla }\varphi$
and $\omega =\boldsymbol {\nabla }\times \boldsymbol {v}\boldsymbol {\cdot } r\boldsymbol {\nabla }\varphi$![]() with the ion fluid velocity $\boldsymbol {v}$
with the ion fluid velocity $\boldsymbol {v}$![]() defined by (2.41). In this simulation, $B_0=1$
defined by (2.41). In this simulation, $B_0=1$![]() and $f_0=2$
and $f_0=2$![]() for the Hasegawa–Mima case, while $B_0=1$
for the Hasegawa–Mima case, while $B_0=1$![]() , $\sigma =0.1$
, $\sigma =0.1$![]() and $f_0=1$
and $f_0=1$![]() for the circular magnetic field case.
for the circular magnetic field case.

Figure 2. (a,b) Vector plots of the $\boldsymbol {E}\times \boldsymbol {B}$![]() drift velocity $\boldsymbol {v}_{\boldsymbol {E}}$
drift velocity $\boldsymbol {v}_{\boldsymbol {E}}$![]() associated with the steady states of figure 1 and contour plots of the modulus $\left \lvert {\boldsymbol {v}_{\boldsymbol {E}}}\right \rvert$
associated with the steady states of figure 1 and contour plots of the modulus $\left \lvert {\boldsymbol {v}_{\boldsymbol {E}}}\right \rvert$![]() . (c,d) Vector plots of the corresponding total ion fluid velocity $\boldsymbol {v}$
. (c,d) Vector plots of the corresponding total ion fluid velocity $\boldsymbol {v}$![]() of (2.41) and contour plots of the modulus $\left \lvert {\boldsymbol {v}}\right \rvert$
of (2.41) and contour plots of the modulus $\left \lvert {\boldsymbol {v}}\right \rvert$![]() .
.
The effect of vertical inhomogeneity in the potential $\phi$![]() can be further examined by setting $\phi =q+\sin \left ({m z}\right )p$
can be further examined by setting $\phi =q+\sin \left ({m z}\right )p$![]() , with $q\left ({r}\right )$
, with $q\left ({r}\right )$![]() and $p\left ({r}\right )$
and $p\left ({r}\right )$![]() radial functions and $m\in \mathbb {Z}$
radial functions and $m\in \mathbb {Z}$![]() . Then, (3.14) reduces to the system
. Then, (3.14) reduces to the system
A plot of the electric potential $\phi$![]() obtained by solution of system (3.15a,b) for different values of $m$
obtained by solution of system (3.15a,b) for different values of $m$![]() and $B_0$
and $B_0$![]() is given in figure 3. Notice that for a sufficiently large magnetic field strength $B_0$
is given in figure 3. Notice that for a sufficiently large magnetic field strength $B_0$![]() , structures arise in the radial direction as well. The size and spacing of these radial structures appear to be modulated by the background strength and curvature of the magnetic field rather than the constant $m$
, structures arise in the radial direction as well. The size and spacing of these radial structures appear to be modulated by the background strength and curvature of the magnetic field rather than the constant $m$![]() associated with the vertical oscillation.
associated with the vertical oscillation.

Figure 3. Contour plots of the electric potential $\phi$![]() obtained by solution of (3.15a,b) for different values of $m$
obtained by solution of (3.15a,b) for different values of $m$![]() and $B_0$
and $B_0$![]() . Dirichlet boundary conditions $p(1)=p(2)=1$
. Dirichlet boundary conditions $p(1)=p(2)=1$![]() and $q(1)=q(2)=1$
and $q(1)=q(2)=1$![]() are used. The case $m=1$
are used. The case $m=1$![]() for (a) $B_0=1$
for (a) $B_0=1$![]() and (d) $B_0=5$
and (d) $B_0=5$![]() . The case $m=3$
. The case $m=3$![]() for (b) $B_0=1$
for (b) $B_0=1$![]() and (e) $B_0=5$
and (e) $B_0=5$![]() . The case $m=6$
. The case $m=6$![]() for (c) $B_0=1$
for (c) $B_0=1$![]() and (f) $B_0=5$
and (f) $B_0=5$![]() . In this simulation $\sigma =0.5$
. In this simulation $\sigma =0.5$![]() and $f_0=1$
and $f_0=1$![]() .
.
4. The case of curved magnetic fields crossing a surface perpendicularly
The Hasegawa–Mima equation is endowed with two inviscid invariants, the total energy and the generalized enstrophy. The generalized enstrophy is an invariant arising from the two-dimensional nature of the governing equation, which is restricted to the flat $\left ({x,y}\right )$![]() plane with normal given by the straight vertical magnetic field $\boldsymbol {B}=B_0\boldsymbol {\nabla } z$
plane with normal given by the straight vertical magnetic field $\boldsymbol {B}=B_0\boldsymbol {\nabla } z$![]() . It is useful to consider the conditions under which the same kind of topological invariant persists in general magnetic fields. A condition for the analogy with two-dimensional vorticity dynamics to apply is that the magnetic field defines the normal direction of a general (not necessarily flat) two-dimensional surface $\varSigma \subset \mathbb {R}^3$
. It is useful to consider the conditions under which the same kind of topological invariant persists in general magnetic fields. A condition for the analogy with two-dimensional vorticity dynamics to apply is that the magnetic field defines the normal direction of a general (not necessarily flat) two-dimensional surface $\varSigma \subset \mathbb {R}^3$![]() . In this case, the dynamics is restricted to the surface $\varSigma$
. In this case, the dynamics is restricted to the surface $\varSigma$![]() because the drift velocity (2.23) satisfies $\boldsymbol {B}\boldsymbol {\cdot }\boldsymbol {v}=0$
because the drift velocity (2.23) satisfies $\boldsymbol {B}\boldsymbol {\cdot }\boldsymbol {v}=0$![]() . The geometric condition for a vector field $\boldsymbol {B}$
. The geometric condition for a vector field $\boldsymbol {B}$![]() to locally define the normal of a surface $\varSigma$
to locally define the normal of a surface $\varSigma$![]() is given by the Frobenius integrability condition (Frankel Reference Frankel2012):
is given by the Frobenius integrability condition (Frankel Reference Frankel2012):
In particular, if the magnetic field $\boldsymbol {B}$![]() has vanishing helicity density then there exist locally defined functions $\alpha,C$
has vanishing helicity density then there exist locally defined functions $\alpha,C$![]() such that
such that
The magnetic field $\boldsymbol {B}$![]() thus defines the normal to the surface $C={\rm constant}$
thus defines the normal to the surface $C={\rm constant}$![]() . When $\alpha =\alpha \left ({C}\right )$
. When $\alpha =\alpha \left ({C}\right )$![]() , the magnetic field $\boldsymbol {B}$
, the magnetic field $\boldsymbol {B}$![]() becomes a vacuum magnetic field because $\boldsymbol {\nabla }\times \boldsymbol {B}=\boldsymbol {0}$
becomes a vacuum magnetic field because $\boldsymbol {\nabla }\times \boldsymbol {B}=\boldsymbol {0}$![]() . In this section, we are concerned with magnetic fields of the type (4.2). We will assume that the functions $\alpha$
. In this section, we are concerned with magnetic fields of the type (4.2). We will assume that the functions $\alpha$![]() and $C$
and $C$![]() exist in the domain $V$
exist in the domain $V$![]() , and that $\boldsymbol {B}\neq \boldsymbol {0}$
, and that $\boldsymbol {B}\neq \boldsymbol {0}$![]() in $V$
in $V$![]() . It is worth noticing that for time-independent magnetic fields the constraint (4.1) corresponds to the requirement that the electric current $\boldsymbol {J}=\boldsymbol {\nabla }\times \boldsymbol {B}$
. It is worth noticing that for time-independent magnetic fields the constraint (4.1) corresponds to the requirement that the electric current $\boldsymbol {J}=\boldsymbol {\nabla }\times \boldsymbol {B}$![]() is always perpendicular to the magnetic field, $\boldsymbol {J}\boldsymbol {\cdot }\boldsymbol {B}=0$
is always perpendicular to the magnetic field, $\boldsymbol {J}\boldsymbol {\cdot }\boldsymbol {B}=0$![]() . In tokamaks this condition will be rarely satisfied because most of the plasma current is aligned with the magnetic field. In addition, we remark that adiabatic electron response underlying the generalized Hasegawa–Mima equation (2.39) may impose additional constraints on the magnetic field configuration. Indeed, one expects adiabatic electron response to hold only when the integral curves of the magnetic field form closed surfaces, with generally non-rational rotational transform.
. In tokamaks this condition will be rarely satisfied because most of the plasma current is aligned with the magnetic field. In addition, we remark that adiabatic electron response underlying the generalized Hasegawa–Mima equation (2.39) may impose additional constraints on the magnetic field configuration. Indeed, one expects adiabatic electron response to hold only when the integral curves of the magnetic field form closed surfaces, with generally non-rational rotational transform.
Next, recall that the magnetic field $\boldsymbol {B}$![]() must be solenoidal. Hence, the Lie–Darboux theorem (Arnold Reference Arnold1989; de Léon Reference de Léon1989) applies: locally there exist functions $\varPsi,\theta$
must be solenoidal. Hence, the Lie–Darboux theorem (Arnold Reference Arnold1989; de Léon Reference de Léon1989) applies: locally there exist functions $\varPsi,\theta$![]() such that
such that
Again, we will assume that the functions $\varPsi$![]() and $\theta$
and $\theta$![]() are well defined in the domain $V$
are well defined in the domain $V$![]() . A typical example of vacuum magnetic field is the magnetic field generated by a point dipole. In this case
. A typical example of vacuum magnetic field is the magnetic field generated by a point dipole. In this case

where $\left ({r,\varphi,z}\right )$![]() are cylindrical coordinates and $M$
are cylindrical coordinates and $M$![]() a physical constant with units of T m$^3$
a physical constant with units of T m$^3$![]() . The functions $\left ({C,\varPsi,\theta }\right )$
. The functions $\left ({C,\varPsi,\theta }\right )$![]() can be used as a system of curvilinear coordinates. The Jacobian determinant of the coordinate transformation is given by
can be used as a system of curvilinear coordinates. The Jacobian determinant of the coordinate transformation is given by
Given two functions $f,g$![]() it is convenient to introduce the bracket
it is convenient to introduce the bracket
Using (4.6), the derived (2.39) can be written as

One can verify that under appropriate boundary conditions on the surface boundary $\partial \varSigma$![]() the surface energy
the surface energy

is a constant of (4.7). The condition for conservation of generalized enstrophy,

can be obtained by noting that the second argument of the bracket on the right-hand side of (4.7) must be a function of $\boldsymbol {\nabla }\boldsymbol {\cdot }\left ({B^{-2}\boldsymbol {\nabla }_{\perp }\phi }\right )$![]() up to a function of $\phi$
up to a function of $\phi$![]() . The condition is
. The condition is
which is equivalent to
In this case, $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {v}_{\boldsymbol {E}}=\boldsymbol {\nabla }\phi \boldsymbol {\cdot }\boldsymbol {\nabla }\times \left ({B^{-2}\boldsymbol {B}}\right )=0$![]() . Notice also that $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {B}=0$
. Notice also that $\boldsymbol {\nabla }\boldsymbol {\cdot }\boldsymbol {B}=0$![]() implies that configurations of the type (4.10) must satisfy
implies that configurations of the type (4.10) must satisfy

In spherical geometry, denoting with $R$![]() the spherical radius, a magnetic field satisfying (4.10) and (4.12) can be obtained by setting
the spherical radius, a magnetic field satisfying (4.10) and (4.12) can be obtained by setting
Similarly, in cylindrical geometry, magnetic fields compatible with (4.10) and (4.12) include those generated by
Observe that when $\lambda =0$![]() (4.7) with either (4.13a–c) or (4.14a) gives the usual two-dimensional vorticity dynamics on a sphere or cylinder respectively. The case of (4.14b) corresponds to a circular magnetic field $\boldsymbol {B}= B_0 r^2\boldsymbol {\nabla }\varphi$
(4.7) with either (4.13a–c) or (4.14a) gives the usual two-dimensional vorticity dynamics on a sphere or cylinder respectively. The case of (4.14b) corresponds to a circular magnetic field $\boldsymbol {B}= B_0 r^2\boldsymbol {\nabla }\varphi$![]() with curvature $\boldsymbol {\kappa }=-\boldsymbol {\nabla }\log r$
with curvature $\boldsymbol {\kappa }=-\boldsymbol {\nabla }\log r$![]() . Assuming axial symmetry $\phi =\phi \left ({r,z}\right )$
. Assuming axial symmetry $\phi =\phi \left ({r,z}\right )$![]() , the corresponding form of (2.39) is again two-dimensional:
, the corresponding form of (2.39) is again two-dimensional:
5. Concluding remarks
In conclusion, we have derived a model equation (2.39) describing electrostatic plasma turbulence in a general magnetic field. The equation preserves the mass (2.28) and the energy (2.33), and reduces to the Hasegawa–Mima equation in the limit of a straight magnetic field. The ordering adopted in the derivation of the equation is slightly different from the classical one. On the one hand, both the magnetic field and the electrostatic potential are allowed to vary on the same spatial scale. On the other hand, the requirement (2.36), which is automatically satisfied in the standard setting of the Hasegawa–Mima equation, ensures the consistency of the ordering with respect to preservation of energy. This latter condition implies that the derived equation is best suited for magnetic fields satisfying (2.37), implying a second-order $\boldsymbol {E}\times \boldsymbol {B}$![]() drift velocity divergence, or for spatial scales such that the change in $\boldsymbol {v}_{\boldsymbol {E}}^2$
drift velocity divergence, or for spatial scales such that the change in $\boldsymbol {v}_{\boldsymbol {E}}^2$![]() represents a third-order contribution (2.38). Nevertheless, as discussed at the end of § 2 the same (2.39) can be obtained from the standard ordering, at the price of more stringent constraints on the spatial behaviour of the magnetic field.
represents a third-order contribution (2.38). Nevertheless, as discussed at the end of § 2 the same (2.39) can be obtained from the standard ordering, at the price of more stringent constraints on the spatial behaviour of the magnetic field.
Conservation of generalized enstrophy holds when the magnetic field defines the normal of a surface and it is compatible with two-dimensional vorticity dynamics. It should be noted that inverse energy cascades are expected to occur for all magnetic configurations such that generalized enstrophy is constant. Indeed, the order of $\phi$![]() derivatives appearing in (4.8) and (4.9) is not changed by the geometry of the background magnetic field. Detailed analysis of the effect of magnetic topology on inverse cascades and zonal flows is left for future work.
derivatives appearing in (4.8) and (4.9) is not changed by the geometry of the background magnetic field. Detailed analysis of the effect of magnetic topology on inverse cascades and zonal flows is left for future work.
As a physical application of the obtained equation, we have studied how the curvature of a circular magnetic field with uniform strength modifies self-organized steady states by comparison with analogous equilibria in a straight magnetic field. A strong curvature tends to attract level sets of electric potential and vorticity, and to enhance fluid drift velocity. A higher curvature also appears to contribute to higher heterogeneity in the electric potential. This behaviour cannot be ascribed to gradient and curvature drifts occurring in the guiding centre picture because the model relies on the cold ions approximation. Hence, the effect of curvature is mediated by the $\boldsymbol{E}\times \boldsymbol{B}$![]() drift.
drift.
Finally, the results reported in this paper may be useful for constructing simplified models of turbulence in complex plasma systems of practical interest. In particular, we expect the derived equation to serve as a toy model of turbulence in tokamak and stellarator plasmas.
Acknowledgements
The authors are grateful to anonymous referees whose comments and suggestions greatly improved the paper.
Editor Per Helander thanks the referees for their advice in evaluating this article.
Funding
This work was partially supported by JSPS KAKENHI (N.S., grant nos. 21K13851, 17H01177).
Declaration of interests
The authors report no conflict of interest.