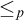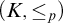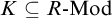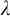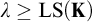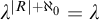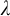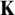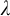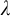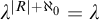1 Introduction
An abstract elementary class
![]() $\mathbf {K}$
(AEC for short) is a pair
$\mathbf {K}$
(AEC for short) is a pair
![]() $\mathbf {K}=(K, \le _{\mathbf {K}})$
where K is a class of structures and
$\mathbf {K}=(K, \le _{\mathbf {K}})$
where K is a class of structures and
![]() $\le _{\mathbf {K}}$
is a partial order on K extending the substructure relation such that
$\le _{\mathbf {K}}$
is a partial order on K extending the substructure relation such that
![]() $\mathbf {K}$
is closed under direct limits and satisfies the coherence property and an instance of the Downward Löwenheim–Skolem theorem. These were introduced by Shelah in [Reference Shelah42]. In this paper, we will study AECs of modules with respect to pure embeddings, i.e., classes of the form
$\mathbf {K}$
is closed under direct limits and satisfies the coherence property and an instance of the Downward Löwenheim–Skolem theorem. These were introduced by Shelah in [Reference Shelah42]. In this paper, we will study AECs of modules with respect to pure embeddings, i.e., classes of the form
![]() $(K, \leq _p)$
where K is a class of R-modules for a fixed ring R and
$(K, \leq _p)$
where K is a class of R-modules for a fixed ring R and
![]() $\leq _p$
is the pure submodule relation.
$\leq _p$
is the pure submodule relation.
Fisher [Reference Fisher10] and Baur [Reference Baur6, Theorem 1] showed independently in the seventies that if T is a complete first-order theory extending the theory of modules, then
![]() $(\text {Mod}(T), \leq _p)$
is
$(\text {Mod}(T), \leq _p)$
is
![]() $\lambda $
-stable for every
$\lambda $
-stable for every
![]() $\lambda $
such that
$\lambda $
such that
![]() $\lambda ^{|R| + \aleph _0}= \lambda $
. A modern proof can be consulted in [Reference Prest29, 3.1]. After realizing that many other classes of modules with pure embeddings were stable such as: abelian groups [Reference Kucera and Mazari-Armida18, 3.16], torsion-free abelian groups [Reference Baldwin, Eklof and Trlifaj5, 0.3], torsion abelian groups [Reference Mazari-Armida25, 4.8], complete elementary classes of torsion modules [Reference Boney7, 5.16], reduced torsion-free abelian groups [Reference Shelah45, 1.2], definable subclasses of modules [Reference Kucera and Mazari-Armida18, 3.16], and flat R-modules [Reference Lieberman, Rosický and Vasey21, 4.3]; it was asked in [Reference Mazari-Armida25, 2.12] the following question:
$\lambda ^{|R| + \aleph _0}= \lambda $
. A modern proof can be consulted in [Reference Prest29, 3.1]. After realizing that many other classes of modules with pure embeddings were stable such as: abelian groups [Reference Kucera and Mazari-Armida18, 3.16], torsion-free abelian groups [Reference Baldwin, Eklof and Trlifaj5, 0.3], torsion abelian groups [Reference Mazari-Armida25, 4.8], complete elementary classes of torsion modules [Reference Boney7, 5.16], reduced torsion-free abelian groups [Reference Shelah45, 1.2], definable subclasses of modules [Reference Kucera and Mazari-Armida18, 3.16], and flat R-modules [Reference Lieberman, Rosický and Vasey21, 4.3]; it was asked in [Reference Mazari-Armida25, 2.12] the following question:
Question 1.1. Let R be an associative ring with unity. If
![]() $(K, \leq _p)$
is an abstract elementary class such that
$(K, \leq _p)$
is an abstract elementary class such that
![]() $K \subseteq R\text {-Mod}$
, is
$K \subseteq R\text {-Mod}$
, is
![]() $(K, \leq _p)$
stable? Is this true if
$(K, \leq _p)$
stable? Is this true if
![]() $R=\mathbb {Z}$
? Under what conditions on R is this true?
$R=\mathbb {Z}$
? Under what conditions on R is this true?
In this paper, we show that many classes of modules are stable. The way we approach the problem is by showing that if the class has some nice algebraic properties then it has to be stable. This approach is new, covers most of the examples known to be stableFootnote 1 and can be used to give many new examples. Prior results focused on syntactic characterizations of the classes or only obtained stability results for specific examples.
Firstly, we study classes closed under direct sums and pure-injective envelopes. These include absolutely pure modules, locally injective modules, locally pure-injective modules, reduced torsion-free groups, and definable subclasses of modules (see Example 3.3).
Theorem 3.11. Assume
![]() $\mathbf {K}= (K , \leq _{p})$
is an AEC with
$\mathbf {K}= (K , \leq _{p})$
is an AEC with
![]() $K \subseteq R\text {-Mod}$
for R an associative ring with unity such that K is closed under direct sums and pure-injective envelopes. If
$K \subseteq R\text {-Mod}$
for R an associative ring with unity such that K is closed under direct sums and pure-injective envelopes. If
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
and
$\lambda ^{|R| + \aleph _0}=\lambda $
and
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
, then
$\lambda \geq \operatorname {LS}(\mathbf {K})$
, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable.
$\lambda $
-stable.
By characterizing the limit models in these classes (Lemmas 3.13 and 3.14), we are able to obtain new characterizations of noetherian rings, pure-semisimple rings, Dedekind domains, and fields via superstability. An example of such a result is the next assertion which extends [Reference Mazari-Armida26, 4.30].
Theorem 3.23. Let R be an associative ring with unity. R is left noetherian if and only if the class of absolutely pure left R-modules with pure embeddings is superstable.
Moreover, the above result can be used to show a link between being good in the stability hierarchy and being good in the axiomatizability hierarchy. More precisely, if the class of absolutely pure modules with pure embeddings is superstable, then it is first-order axiomatizable (see Corollary 3.25).
The results for these classes of modules can also be used to partially solve Question 1.1 if one substitutes stable for superstable.
Lemma 3.30. Let R be an associative ring with unity. The following are equivalent.
-
(1) R is left pure-semisimple.
-
(2) Every AEC
 $\mathbf {K}= (K , \leq _{p})$
with
$\mathbf {K}= (K , \leq _{p})$
with
 $K \subseteq R\text {-Mod}$
, such that K is closed under direct sums, is superstable.
$K \subseteq R\text {-Mod}$
, such that K is closed under direct sums, is superstable.
Secondly, we study classes closed under direct sums, pure submodules, and pure epimorphic images. These include flat modules, torsion abelian groups,
![]() $\mathfrak {s}$
-torsion modules, and any class axiomatized by an F-sentence (see Example 4.3).
$\mathfrak {s}$
-torsion modules, and any class axiomatized by an F-sentence (see Example 4.3).
Theorem 4.17. Assume
![]() $\mathbf {K}= (K , \leq _{p})$
is an AEC with
$\mathbf {K}= (K , \leq _{p})$
is an AEC with
![]() $K \subseteq R\text {-Mod}$
for R an associative ring with unity such that K is closed under direct sums, pure submodules, and pure epimorphic images. If
$K \subseteq R\text {-Mod}$
for R an associative ring with unity such that K is closed under direct sums, pure submodules, and pure epimorphic images. If
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
, then
$\lambda ^{|R| + \aleph _0}=\lambda $
, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable.
$\lambda $
-stable.
This result can be used to construct universal models with respect to pure embeddings. In particular, we obtain the next result which extends [Reference Shelah45, 1.2], [Reference Mazari-Armida27, 4.6], and [Reference Mazari-Armida25, 3.7].
Corollary 3.12. Let R be an associative ring with unity. If
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
or
$\lambda ^{|R| + \aleph _0}=\lambda $
or
![]() $\forall \mu < \lambda ( \mu ^{|R| + \aleph _0} < \lambda )$
, then there is a universal model in the class of flat R-modules with pure embeddings and in the class of
$\forall \mu < \lambda ( \mu ^{|R| + \aleph _0} < \lambda )$
, then there is a universal model in the class of flat R-modules with pure embeddings and in the class of
![]() $\mathfrak {s}$
-torsion R-modules with pure embeddings of cardinality
$\mathfrak {s}$
-torsion R-modules with pure embeddings of cardinality
![]() $\lambda $
.
$\lambda $
.
Finally, we study classes of modules that are closed under pure submodules and that are contained in a well-understood class of modules which is closed under pure submodules and that admits intersections. The main examples for this case are subclasses of the class of torsion-free groups such as
![]() $\aleph _1$
-free groups and finitely Butler groups (see Example 5.2).
$\aleph _1$
-free groups and finitely Butler groups (see Example 5.2).
We use the results obtained for these classes of modules to provide a partial solution to Question 1.1.
Lemma 5.10. Assume R is a Von Neumann regular ring. If K is closed under submodules and has arbitrarily large models, then
![]() $\mathbf {K} =(K, \leq _p)$
is
$\mathbf {K} =(K, \leq _p)$
is
![]() $\lambda $
-stable if
$\lambda $
-stable if
![]() $\lambda ^{ |R| + \aleph _0} = \lambda $
.
$\lambda ^{ |R| + \aleph _0} = \lambda $
.
The paper is organized as follows. Section 2 presents necessary background. Section 3 studies classes closed under direct sums and pure-injective envelopes. Section 4 studies classes closed under direct sums, pure submodules, and pure epimorphic images. Section 5 studies classes of modules that are closed under pure submodules and that are contained in a well-understood class of modules which is closed under pure submodules and that admits intersection .
2 Preliminaries
In this section, we recall the necessary notions from abstract elementary classes, independence relations, and module theory that are used in this paper.
2.1 Abstract elementary classes
We briefly present the notions of abstract elementary classes that are used in this paper. These are further studied in [Reference Baldwin3, Sections 4–8] and [Reference Grossberg12, Sections 2 and 4.4]. An introduction from an algebraic perspective is given in [Reference Mazari-Armida25, Section 2].
Abstract elementary classes (AECs for short) were introduced by Shelah in [Reference Shelah42] to study those classes of structures axiomatized in
![]() $L_{\omega _1, \omega }(Q)$
. An AEC is a pair
$L_{\omega _1, \omega }(Q)$
. An AEC is a pair
![]() $\mathbf {K}=(K, \le _{\mathbf {K}})$
where K is a class of structures and
$\mathbf {K}=(K, \le _{\mathbf {K}})$
where K is a class of structures and
![]() $\le _{\mathbf {K}}$
is a partial order on K extending the substructure relation such that
$\le _{\mathbf {K}}$
is a partial order on K extending the substructure relation such that
![]() $\mathbf {K}$
is closed under direct limits and satisfies the coherence property and an instance of the Downward Löwenheim–Skolem theorem. The reader can consult the definition in [Reference Baldwin3, 4.1].
$\mathbf {K}$
is closed under direct limits and satisfies the coherence property and an instance of the Downward Löwenheim–Skolem theorem. The reader can consult the definition in [Reference Baldwin3, 4.1].
Given a model M, we will write
![]() $|M|$
for its underlying set and
$|M|$
for its underlying set and
![]() $\| M \|$
for its cardinality. Given
$\| M \|$
for its cardinality. Given
![]() $\lambda $
a cardinal and
$\lambda $
a cardinal and
![]() $\mathbf {K}$
an AEC, we denote by
$\mathbf {K}$
an AEC, we denote by
![]() $\mathbf {K}_{\lambda }$
the models in
$\mathbf {K}_{\lambda }$
the models in
![]() $\mathbf {K}$
of cardinality
$\mathbf {K}$
of cardinality
![]() $\lambda $
. Moreover, if we write “
$\lambda $
. Moreover, if we write “
![]() $f: M \to N$
,” we assume that f is a
$f: M \to N$
,” we assume that f is a
![]() $\mathbf {K}$
-embedding, i.e.,
$\mathbf {K}$
-embedding, i.e.,
![]() $f: M \cong f[M]$
and
$f: M \cong f[M]$
and
![]() $f[M] \le _{\mathbf {K}} N$
. In particular,
$f[M] \le _{\mathbf {K}} N$
. In particular,
![]() $\mathbf {K}$
-embeddings are always monomorphisms.
$\mathbf {K}$
-embeddings are always monomorphisms.
Shelah introduced a notion of semantic type in [Reference Shelah43]. Following [Reference Grossberg13], we call these semantic types Galois-types. Given
![]() $(\mathbf {b}, A, N)$
, where
$(\mathbf {b}, A, N)$
, where
![]() $N \in \mathbf {K}$
,
$N \in \mathbf {K}$
,
![]() $A \subseteq |N|$
, and
$A \subseteq |N|$
, and
![]() $\mathbf {b}$
is a sequence in N, the Galois-type of
$\mathbf {b}$
is a sequence in N, the Galois-type of
![]() $\mathbf {b}$
over A in N, denoted by
$\mathbf {b}$
over A in N, denoted by
![]() $\mathbf {gtp}_{\mathbf {K}} (\mathbf {b} / A; N)$
, is the equivalence class of
$\mathbf {gtp}_{\mathbf {K}} (\mathbf {b} / A; N)$
, is the equivalence class of
![]() $(\mathbf {b}, A, N)$
modulo
$(\mathbf {b}, A, N)$
modulo
![]() $E^{\mathbf {K}}$
;
$E^{\mathbf {K}}$
;
![]() $E^{\mathbf {K}}$
is the transitive closure of
$E^{\mathbf {K}}$
is the transitive closure of
![]() $E_{\text {at}}^{\mathbf {K}}$
where
$E_{\text {at}}^{\mathbf {K}}$
where
![]() $(\mathbf {b}_1, A_1, N_1)E_{\text {at}}^{\mathbf {K}} (\mathbf {b}_2, A_2, N_2)$
if
$(\mathbf {b}_1, A_1, N_1)E_{\text {at}}^{\mathbf {K}} (\mathbf {b}_2, A_2, N_2)$
if
![]() $A := A_1 = A_2$
, and there exist
$A := A_1 = A_2$
, and there exist
![]() $\mathbf {K}$
-embeddings
$\mathbf {K}$
-embeddings
![]() $f_\ell : N_\ell \xrightarrow [A]{} N$
for
$f_\ell : N_\ell \xrightarrow [A]{} N$
for
![]() $\ell \in \{ 1, 2\}$
such that
$\ell \in \{ 1, 2\}$
such that
![]() $f_1 (\mathbf {b}_1) = f_2 (\mathbf {b}_2)$
and
$f_1 (\mathbf {b}_1) = f_2 (\mathbf {b}_2)$
and
![]() $N \in \mathbf {K}$
. Given
$N \in \mathbf {K}$
. Given
![]() $p=\mathbf {gtp}_{\mathbf {K}}(\mathbf {b}/A; N)$
and
$p=\mathbf {gtp}_{\mathbf {K}}(\mathbf {b}/A; N)$
and
![]() $C \subseteq A$
, let
$C \subseteq A$
, let
![]() $p\upharpoonright {C}= [(\mathbf {b}, C, N)]_{E^{\mathbf {K}}}$
.
$p\upharpoonright {C}= [(\mathbf {b}, C, N)]_{E^{\mathbf {K}}}$
.
If
![]() $M \in K$
and
$M \in K$
and
![]() $\alpha $
is an ordinal, let
$\alpha $
is an ordinal, let
![]() $\mathbf {gS}^\alpha _{\mathbf {K}}(M)= \{ \mathbf {gtp}_{\mathbf {K}}(\mathbf {b} / M; N) : M \leq _{\mathbf {K}} N\in \mathbf {K} \text { and } \mathbf {b} \in N^\alpha \} $
. When
$\mathbf {gS}^\alpha _{\mathbf {K}}(M)= \{ \mathbf {gtp}_{\mathbf {K}}(\mathbf {b} / M; N) : M \leq _{\mathbf {K}} N\in \mathbf {K} \text { and } \mathbf {b} \in N^\alpha \} $
. When
![]() $\alpha =1$
, we write
$\alpha =1$
, we write
![]() $\mathbf {gS}_{\mathbf {K}}(M)$
instead of
$\mathbf {gS}_{\mathbf {K}}(M)$
instead of
![]() $\mathbf {gS}^1_{\mathbf {K}}(M)$
. We let
$\mathbf {gS}^1_{\mathbf {K}}(M)$
. We let
![]() $\mathbf {gS}^{< \infty }_{\mathbf {K}}(M) = \bigcup _{\alpha \in OR} \mathbf {gS}^\alpha _{\mathbf {K}}(M)$
.
$\mathbf {gS}^{< \infty }_{\mathbf {K}}(M) = \bigcup _{\alpha \in OR} \mathbf {gS}^\alpha _{\mathbf {K}}(M)$
.
Since Galois-types are equivalence classes, they might not be determined by their finite restrictions. We say that
![]() $\mathbf {K}$
is fully
$\mathbf {K}$
is fully
![]() $(< \aleph _0)$
-tame if for any
$(< \aleph _0)$
-tame if for any
![]() $M \in \mathbf {K}$
and
$M \in \mathbf {K}$
and
![]() $p \neq q \in \mathbf {gS}^{< \infty }(M)$
, there is
$p \neq q \in \mathbf {gS}^{< \infty }(M)$
, there is
![]() $A \subseteq |M|$
such that
$A \subseteq |M|$
such that
![]() $|A |< \aleph _0$
and
$|A |< \aleph _0$
and
![]() $p\upharpoonright {A} \neq q\upharpoonright {A}$
. Tameness was isolated by Grossberg and VanDieren in [Reference Grossberg and VanDieren14].
$p\upharpoonright {A} \neq q\upharpoonright {A}$
. Tameness was isolated by Grossberg and VanDieren in [Reference Grossberg and VanDieren14].
We now introduce the main notion of this paper.
Definition 2.1. An AEC
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable if for any
$\lambda $
-stable if for any
![]() $M \in \mathbf {K}_\lambda $
,
$M \in \mathbf {K}_\lambda $
,
![]() $| \mathbf {gS}_{\mathbf {K}}(M) | \leq \lambda $
.
$| \mathbf {gS}_{\mathbf {K}}(M) | \leq \lambda $
.
Recall that a model M is universal over N if and only if
![]() $\| N\|= \| M\|=\lambda $
and for every
$\| N\|= \| M\|=\lambda $
and for every
![]() $N^* \in \mathbf {K}_{\lambda }$
such that
$N^* \in \mathbf {K}_{\lambda }$
such that
![]() $N \le _{\mathbf {K}} N^*$
, there is
$N \le _{\mathbf {K}} N^*$
, there is
![]() $f: N^* \xrightarrow [N]{} M$
. Let us recall the notion of limit model.
$f: N^* \xrightarrow [N]{} M$
. Let us recall the notion of limit model.
Definition 2.2. Let
![]() $\lambda $
be an infinite cardinal and
$\lambda $
be an infinite cardinal and
![]() $\alpha < \lambda ^+$
be a limit ordinal. M is a
$\alpha < \lambda ^+$
be a limit ordinal. M is a
![]() $(\lambda , \alpha )$
-limit model over N if and only if there is
$(\lambda , \alpha )$
-limit model over N if and only if there is
![]() $\{ M_i : i < \alpha \}\subseteq \mathbf {K}_\lambda $
an increasing continuous chain such that:
$\{ M_i : i < \alpha \}\subseteq \mathbf {K}_\lambda $
an increasing continuous chain such that:
-
(1)
 $M_0 =N$
.
$M_0 =N$
. -
(2)
 $M= \bigcup _{i < \alpha } M_i$
.
$M= \bigcup _{i < \alpha } M_i$
. -
(3)
 $M_{i+1}$
is universal over
$M_{i+1}$
is universal over
 $M_i$
for each
$M_i$
for each
 $i < \alpha $
.
$i < \alpha $
.
M is a
![]() $(\lambda , \alpha )$
-limit model if there is
$(\lambda , \alpha )$
-limit model if there is
![]() $N \in \mathbf {K}_\lambda $
such that M is a
$N \in \mathbf {K}_\lambda $
such that M is a
![]() $(\lambda , \alpha )$
-limit model over N. M is a
$(\lambda , \alpha )$
-limit model over N. M is a
![]() $\lambda $
-limit model if there is a limit ordinal
$\lambda $
-limit model if there is a limit ordinal
![]() $\alpha < \lambda ^+$
such that M is a
$\alpha < \lambda ^+$
such that M is a
![]() $(\lambda , \alpha )$
-limit model.
$(\lambda , \alpha )$
-limit model.
We say that
![]() $\mathbf {K}$
has uniqueness of limit models of cardinality
$\mathbf {K}$
has uniqueness of limit models of cardinality
![]() $\lambda $
if
$\lambda $
if
![]() $\mathbf {K}$
has
$\mathbf {K}$
has
![]() $\lambda $
-limit models and if any two
$\lambda $
-limit models and if any two
![]() $\lambda $
-limit models are isomorphic. We introduce the notion of superstability for AECs.
$\lambda $
-limit models are isomorphic. We introduce the notion of superstability for AECs.
Definition 2.3.
![]() $\mathbf {K}$
is a superstable AEC if and only if
$\mathbf {K}$
is a superstable AEC if and only if
![]() $\mathbf {K}$
has uniqueness of limit models on a tail of cardinals.
$\mathbf {K}$
has uniqueness of limit models on a tail of cardinals.
Remark 2.4. In [Reference Grossberg and Vasey15, 1.3] and [Reference Vasey47] was shown that for AECs that have amalgamation, joint embedding, no maximal models and are tame, the definition above is equivalent to every other definition of superstability considered in the context of AECs. Grossberg’s and Vasey’s result builds on significant earlier results due to Boney, Grossberg, Shelah, VanDieren, Vasey, and Villaveces.Footnote
2
For a complete first-order theory T,
![]() $(Mod(T), \preceq )$
is superstable if and only if T is
$(Mod(T), \preceq )$
is superstable if and only if T is
![]() $\lambda $
-stable for every
$\lambda $
-stable for every
![]() $\lambda \geq 2^{|T|}$
.
$\lambda \geq 2^{|T|}$
.
Finally, recall that a model
![]() $M \in \mathbf {K}$
is a universal model in
$M \in \mathbf {K}$
is a universal model in
![]() $\mathbf {K}_\lambda $
if
$\mathbf {K}_\lambda $
if
![]() $M \in \mathbf {K}_\lambda $
and if given any
$M \in \mathbf {K}_\lambda $
and if given any
![]() $N \in \mathbf {K}_\lambda $
, there is a
$N \in \mathbf {K}_\lambda $
, there is a
![]() $\mathbf {K}$
-embedding
$\mathbf {K}$
-embedding
![]() $f: N \to M$
. We say that
$f: N \to M$
. We say that
![]() $\mathbf {K}$
has a universal model of cardinality
$\mathbf {K}$
has a universal model of cardinality
![]() $\lambda $
if there is a universal model in
$\lambda $
if there is a universal model in
![]() $\mathbf {K}_\lambda $
. It is well-known that if
$\mathbf {K}_\lambda $
. It is well-known that if
![]() $\mathbf {K}$
is an AEC with the joint embedding property and M is a
$\mathbf {K}$
is an AEC with the joint embedding property and M is a
![]() $\lambda $
-limit model, then M is universal in
$\lambda $
-limit model, then M is universal in
![]() $\mathbf {K}_\lambda $
.
$\mathbf {K}_\lambda $
.
2.2 Independence relations
We recall the basic properties of independence relations on arbitrary categories. These were introduced and studied in detail in [Reference Lieberman, Rosický and Vasey20].
Definition 2.5 [Reference Lieberman, Rosický and Vasey20, 3.4].
An independence relation on a category
![]() $\mathcal {C}$
is a set
$\mathcal {C}$
is a set
of commutative squares such that for any commutative diagram:

we have that
if and only if
.
We will be particularly interested in weakly stable independence relations. Recall that an independence relation
![]() is weakly stable if it satisfies: symmetry [Reference Lieberman, Rosický and Vasey20, 3.9], existence [Reference Lieberman, Rosický and Vasey20, 3.10], uniqueness [Reference Lieberman, Rosický and Vasey20, 3.13], and transitivity [Reference Lieberman, Rosický and Vasey20, 3.15].
is weakly stable if it satisfies: symmetry [Reference Lieberman, Rosický and Vasey20, 3.9], existence [Reference Lieberman, Rosický and Vasey20, 3.10], uniqueness [Reference Lieberman, Rosický and Vasey20, 3.13], and transitivity [Reference Lieberman, Rosický and Vasey20, 3.15].
They also introduced the notion of a stable independence relation for any category
![]() $\mathcal {C}$
in [Reference Lieberman, Rosický and Vasey20, 3.24]. As the definition is long and we will only study independence relations on AECs, we introduce the definition for AECs instead. For an AEC
$\mathcal {C}$
in [Reference Lieberman, Rosický and Vasey20, 3.24]. As the definition is long and we will only study independence relations on AECs, we introduce the definition for AECs instead. For an AEC
![]() $\mathbf {K}$
, an indepedence relation
$\mathbf {K}$
, an indepedence relation
![]() is stable if it is weakly stable and satisfies local character [Reference Lieberman, Rosický and Vasey20, 8.6] and the witness property [Reference Lieberman, Rosický and Vasey20, 8.7].
is stable if it is weakly stable and satisfies local character [Reference Lieberman, Rosický and Vasey20, 8.6] and the witness property [Reference Lieberman, Rosický and Vasey20, 8.7].
2.3 Module theory
We succinctly introduce the notions from module theory that are used in this paper. These are further studied in [Reference Prest29].
All rings considered in this paper are associative with unity. In the rest of the paper, if we mention that R is a ring, we are assuming that it is associative with unity. All the classes studied in this paper have as their language the standard language of modules, i.e., for a ring R we take
![]() $L_{R}= \{0, +,-\} \cup \{ r\cdot : r \in R \}$
. A formula
$L_{R}= \{0, +,-\} \cup \{ r\cdot : r \in R \}$
. A formula
![]() $\phi $
is a positive primitive formula (
$\phi $
is a positive primitive formula (
![]() $pp$
-formula for short), if
$pp$
-formula for short), if
![]() $\phi $
is an existentially quantified finite system of linear equations. Given
$\phi $
is an existentially quantified finite system of linear equations. Given
![]() $\bar {b} \in M^{< \infty }$
and
$\bar {b} \in M^{< \infty }$
and
![]() $M \subseteq N$
, the
$M \subseteq N$
, the
![]() $pp$
-type of
$pp$
-type of
![]() $\bar {b}$
over M in N, denoted by
$\bar {b}$
over M in N, denoted by
![]() $\operatorname {\mathrm {pp}}(\bar {b}/M , N)$
, is the set of
$\operatorname {\mathrm {pp}}(\bar {b}/M , N)$
, is the set of
![]() $pp$
-formulas with parameters in M that hold for
$pp$
-formulas with parameters in M that hold for
![]() $\bar {b}$
in N.
$\bar {b}$
in N.
Given M and
![]() $N \ R$
-modules, M is a pure submodule of N, denoted by
$N \ R$
-modules, M is a pure submodule of N, denoted by
![]() $M \leq _{p} N$
, if and only if M is a submodule of N and for every
$M \leq _{p} N$
, if and only if M is a submodule of N and for every
![]() $\bar {a} \in M^{< \omega }$
,
$\bar {a} \in M^{< \omega }$
,
![]() $pp(\bar {a}/ \emptyset , M)= pp(\bar {a}/\emptyset , N)$
. Moreover,
$pp(\bar {a}/ \emptyset , M)= pp(\bar {a}/\emptyset , N)$
. Moreover,
![]() $f: M \to N$
is a pure epimorphism if f is an epimorphism and the kernel of f is a pure submodule of M.
$f: M \to N$
is a pure epimorphism if f is an epimorphism and the kernel of f is a pure submodule of M.
Recall that a module M is pure-injective if for every N, if M is a pure submodule of N, then M is a direct summand of N. Given a module M, the pure-injective envelope of M, denoted by
![]() $PE(M)$
, is a pure-injective module such that
$PE(M)$
, is a pure-injective module such that
![]() $M \leq _{p} PE(M)$
and it is minimum with respect to this property. Its existence follows from [Reference Ziegler48, 3.6] and the fact that every module can be embedded into a pure-injective module.
$M \leq _{p} PE(M)$
and it is minimum with respect to this property. Its existence follows from [Reference Ziegler48, 3.6] and the fact that every module can be embedded into a pure-injective module.
The following Schröder–Bernstein property of pure-injective modules will be useful.
Fact 2.6 [Reference Guil Asensio, Kalebogaz and Srivastava16, 2.5].
Let
![]() $M, N$
be pure-injective modules. If there are
$M, N$
be pure-injective modules. If there are
![]() $f: M \to N$
a pure embedding and
$f: M \to N$
a pure embedding and
![]() $g: N \to M$
a pure embedding, then M and N are isomorphic.
$g: N \to M$
a pure embedding, then M and N are isomorphic.
M is
![]() $\Sigma $
-pure-injective if
$\Sigma $
-pure-injective if
![]() $M^{(\aleph _0)}$
is pure-injective where
$M^{(\aleph _0)}$
is pure-injective where
![]() $M^{(\aleph _0)}$
denotes the countable direct sum of M. The next four properties of
$M^{(\aleph _0)}$
denotes the countable direct sum of M. The next four properties of
![]() $\Sigma $
-pure-injective modules will be useful. The first three bullet points follow from [Reference Prest29, 2.11].
$\Sigma $
-pure-injective modules will be useful. The first three bullet points follow from [Reference Prest29, 2.11].
Fact 2.7.
-
• If N is
 $\Sigma $
-pure-injective, then N is pure-injective.
$\Sigma $
-pure-injective, then N is pure-injective. -
• If N is
 $\Sigma $
-pure-injective and
$\Sigma $
-pure-injective and
 $M \leq _{p} N$
, then M is
$M \leq _{p} N$
, then M is
 $\Sigma $
-pure-injective.
$\Sigma $
-pure-injective. -
• If N is
 $\Sigma $
-pure-injective and M is elementary equivalent to N, then M is
$\Sigma $
-pure-injective and M is elementary equivalent to N, then M is
 $\Sigma $
-pure-injective.
$\Sigma $
-pure-injective. -
• [Reference Prest29, 3.2] If N is
 $\Sigma $
-pure-injective, then
$\Sigma $
-pure-injective, then
 $(\text {Mod}(Th(N)), \leq _p)$
is
$(\text {Mod}(Th(N)), \leq _p)$
is
 $\lambda $
-stable for every
$\lambda $
-stable for every
 $\lambda \geq |Th(N)|$
. For the models of the theory of N, being a pure submodule is the same as being an elementary submodule by
$\lambda \geq |Th(N)|$
. For the models of the theory of N, being a pure submodule is the same as being an elementary submodule by
 $pp$
-quantifier elimination.
$pp$
-quantifier elimination.
3 Classes closed under pure-injective envelopes
In this section we study classes closed under direct sums and pure-injective envelopes. We show that they are always stable and we give an algebraic characterization of when they are superstable.
Hypothesis 3.1. Let
![]() $\mathbf {K}= (K , \leq _{p})$
be an AEC with
$\mathbf {K}= (K , \leq _{p})$
be an AEC with
![]() $K \subseteq R\text {-Mod}$
for a fixed ring R such that:
$K \subseteq R\text {-Mod}$
for a fixed ring R such that:
-
(1) K is closed under direct sums.
-
(2) K is closed under pure-injective envelopes, i.e., if
 $M \in K$
, then
$M \in K$
, then
 $PE(M) \in K$
.
$PE(M) \in K$
.
Remark 3.2. Most of the results in this section assume the above hypothesis, but not all of them. We will explicitly mention when we assume the hypothesis.
Below we give some examples of classes of modules satisfying Hypothesis 3.1.
Example 3.3.
-
(1)
 $(R\text {-AbsP}, \leq _{p})$
where
$(R\text {-AbsP}, \leq _{p})$
where
 $R\text {-AbsP}$
is the class of absolutely pure R-modules. A module M is absolutely pure if it is pure in every module containing it. It is an AEC because being a pure submodule is tested by finite tuples and because it is closed under pure submodules [Reference Prest30, 2.3.5]. Closure under direct sums follows from [Reference Prest30, 2.3.5] and closure under pure-injective envelopes follows from [Reference Prest30, 4.3.12].
$R\text {-AbsP}$
is the class of absolutely pure R-modules. A module M is absolutely pure if it is pure in every module containing it. It is an AEC because being a pure submodule is tested by finite tuples and because it is closed under pure submodules [Reference Prest30, 2.3.5]. Closure under direct sums follows from [Reference Prest30, 2.3.5] and closure under pure-injective envelopes follows from [Reference Prest30, 4.3.12]. -
(2)
 $(R\text {-l-inj}, \leq _p)$
where
$(R\text {-l-inj}, \leq _p)$
where
 $R\text {-l-inj}$
is the class of locally injective R-modules (also called finitely injective modules). A module M is locally injective if given
$R\text {-l-inj}$
is the class of locally injective R-modules (also called finitely injective modules). A module M is locally injective if given
 $\bar {a} \in M^{< \omega }$
there is an injective submodule of M containing
$\bar {a} \in M^{< \omega }$
there is an injective submodule of M containing
 $\bar {a}$
. It is an AEC because we only test for finite tuples and because the cardinality of the injective envelope of a finite tuple is bounded by
$\bar {a}$
. It is an AEC because we only test for finite tuples and because the cardinality of the injective envelope of a finite tuple is bounded by
 $2^{|R| + \aleph _0}$
[Reference Eklof9, Theorem 1]. Closure under direct sums is clear and closure under pure-injective envelopes follows from the fact that locally injective modules are absolutely pure [Reference Ramamurthi and Rangaswamy32, 3.1] and [Reference Prest30, 4.3.12].
$2^{|R| + \aleph _0}$
[Reference Eklof9, Theorem 1]. Closure under direct sums is clear and closure under pure-injective envelopes follows from the fact that locally injective modules are absolutely pure [Reference Ramamurthi and Rangaswamy32, 3.1] and [Reference Prest30, 4.3.12]. -
(3)
 $(R\text {-l-pi}, \leq _p)$
where
$(R\text {-l-pi}, \leq _p)$
where
 $R\text {-l-pi}$
is the class of locally pure-injective R-modules. A module M is locally pure-injective if given
$R\text {-l-pi}$
is the class of locally pure-injective R-modules. A module M is locally pure-injective if given
 $\bar {a} \in M^{< \omega }$
there is a pure-injective pure submodule of M containing
$\bar {a} \in M^{< \omega }$
there is a pure-injective pure submodule of M containing
 $\bar {a}$
. It is an AEC because we only test for finite tuples and because the cardinality of the pure-injective envelope of a finite tuple is bounded by
$\bar {a}$
. It is an AEC because we only test for finite tuples and because the cardinality of the pure-injective envelope of a finite tuple is bounded by
 $2^{|R| + \aleph _0}$
[Reference Ziegler48, 3.11]. Closure under direct sums and pure-injective envelopes follow from [Reference Zimmermann50, 2.4].
$2^{|R| + \aleph _0}$
[Reference Ziegler48, 3.11]. Closure under direct sums and pure-injective envelopes follow from [Reference Zimmermann50, 2.4]. -
(4)
 $(\text {RTF}, \leq _p)$
where RTF is the class of reduced torsion-free abelian groups. A group G is reduced if it does not have non-trivial divisible subgroups. It is an AEC because the intersection of a torsion-free divisible subgroup with a torsion-free pure subgroup is a divisible subgroup and because it is closed under pure subgroups. Closure under direct sums is easy to check, while closure under pure-injective envelopes follows from [Reference Fuchs11, 6.4.3].
$(\text {RTF}, \leq _p)$
where RTF is the class of reduced torsion-free abelian groups. A group G is reduced if it does not have non-trivial divisible subgroups. It is an AEC because the intersection of a torsion-free divisible subgroup with a torsion-free pure subgroup is a divisible subgroup and because it is closed under pure subgroups. Closure under direct sums is easy to check, while closure under pure-injective envelopes follows from [Reference Fuchs11, 6.4.3]. -
(5)
 $(R\text {-Flat}, \leq _{p})$
where
$(R\text {-Flat}, \leq _{p})$
where
 $R\text {-Flat}$
is the class of flat R-modules under the additional assumption that the pure-injective envelope of every flat modules is flat.Footnote
3
It is an AEC because it is closed under direct limits and pure submodules. Closure under direct sums is easy to check and we are assuming closure under pure-injective envelopes.
$R\text {-Flat}$
is the class of flat R-modules under the additional assumption that the pure-injective envelope of every flat modules is flat.Footnote
3
It is an AEC because it is closed under direct limits and pure submodules. Closure under direct sums is easy to check and we are assuming closure under pure-injective envelopes. -
(6)
 $(\chi , \leq _{p})$
where
$(\chi , \leq _{p})$
where
 $\chi $
is a definable category of modules in the sense of [Reference Prest30, Section 3.4]. A class of modules is definable if it is closed under direct products, direct limits and pure submodules. It is an AEC because it is closed under direct limits and pure submodules. Closure under pure-injective envelopes follows from [Reference Prest30, 4.3.21].
$\chi $
is a definable category of modules in the sense of [Reference Prest30, Section 3.4]. A class of modules is definable if it is closed under direct products, direct limits and pure submodules. It is an AEC because it is closed under direct limits and pure submodules. Closure under pure-injective envelopes follows from [Reference Prest30, 4.3.21].
Remark 3.4. It is worth mentioning that none of the above examples are first-order axiomatizable with the exception of the last one.
3.1 Stability
We begin by showing some structural properties of the classes satisfying Hypothesis 3.1. The argument for the amalgamation property is due to T. G. Kucera.
Lemma 3.5. If
![]() $\mathbf {K}$
satisfies Hypothesis 3.1, then
$\mathbf {K}$
satisfies Hypothesis 3.1, then
![]() $\mathbf {K}$
has joint embedding, amalgamation, no maximal models and
$\mathbf {K}$
has joint embedding, amalgamation, no maximal models and
![]() $|R| + \aleph _0 \leq \operatorname {LS}(\mathbf {K})$
.
$|R| + \aleph _0 \leq \operatorname {LS}(\mathbf {K})$
.
Proof. Joint embedding and no maximal models follow directly from closure under direct sums. So we show the amalgamation property.
Let
![]() $M \leq _p N_1, N_2$
be models of K. By minimality of the pure-injective envelope we obtain that
$M \leq _p N_1, N_2$
be models of K. By minimality of the pure-injective envelope we obtain that
![]() $PE(M) \leq _p PE(N_1), PE(N_2)$
and observe that all of these models are in K by closure under pure-injective envelopes.
$PE(M) \leq _p PE(N_1), PE(N_2)$
and observe that all of these models are in K by closure under pure-injective envelopes.
Let
![]() $L := PE(N_1) \oplus PE(N_2)$
which is in K by closure under direct sums. Now, as
$L := PE(N_1) \oplus PE(N_2)$
which is in K by closure under direct sums. Now, as
![]() $PE(M)$
is pure-injective, there are
$PE(M)$
is pure-injective, there are
![]() $N_1'$
and
$N_1'$
and
![]() $N_2'$
such that
$N_2'$
such that
![]() $PE(N_i)= PE(M) \oplus N_i'$
for
$PE(N_i)= PE(M) \oplus N_i'$
for
![]() $i \in \{ 1, 2\}$
. Hence,
$i \in \{ 1, 2\}$
. Hence,
![]() $L = (PE(M) \oplus N_1') \oplus (PE(M) \oplus N_2')$
. Define
$L = (PE(M) \oplus N_1') \oplus (PE(M) \oplus N_2')$
. Define
![]() $f: N_1 \to L$
by
$f: N_1 \to L$
by
![]() $f(m + n_1)=(m, n_1, m, 0)$
for
$f(m + n_1)=(m, n_1, m, 0)$
for
![]() $m \in PE(M)$
and
$m \in PE(M)$
and
![]() $n_1 \in N_1'$
and
$n_1 \in N_1'$
and
![]() $g: N_2 \to L$
by
$g: N_2 \to L$
by
![]() $g(m + n_2)=(m, 0, m, n_{2})$
for
$g(m + n_2)=(m, 0, m, n_{2})$
for
![]() $m \in PE(M)$
and
$m \in PE(M)$
and
![]() $n_2 \in N_2'$
. One can show that
$n_2 \in N_2'$
. One can show that
![]() $f, g$
are pure embeddings such that
$f, g$
are pure embeddings such that
![]() $f\mathord {\upharpoonright }{M}= g\mathord {\upharpoonright }{M}$
.⊣
$f\mathord {\upharpoonright }{M}= g\mathord {\upharpoonright }{M}$
.⊣
The next abstraction is a first step toward a general solution to Question 1.1. We thank an anonymous referee for suggesting this approach.
Definition 3.6. The Galois-types in
![]() $\mathbf {K}$
are
$\mathbf {K}$
are
![]() $pp$
-syntactic if for every
$pp$
-syntactic if for every
![]() $M, N_1, N_2 \in \mathbf {K}$
,
$M, N_1, N_2 \in \mathbf {K}$
,
![]() $M \le _{\mathbf {K}} N_1, N_2$
,
$M \le _{\mathbf {K}} N_1, N_2$
,
![]() $\bar {b}_{1} \in N_1^{<\infty }$
and
$\bar {b}_{1} \in N_1^{<\infty }$
and
![]() $\bar {b}_{2} \in N_2^{<\infty }$
we have that:
$\bar {b}_{2} \in N_2^{<\infty }$
we have that:
Remark 3.7. It is straightforward to show that if Galois-types in
![]() $\mathbf {K}$
are
$\mathbf {K}$
are
![]() $pp$
-syntactic, then
$pp$
-syntactic, then
![]() $\mathbf {K}$
is fully
$\mathbf {K}$
is fully
![]() $(< \aleph _0)$
-tame.
$(< \aleph _0)$
-tame.
Our main result regarding classes where Galois-types are
![]() $pp$
-syntactic is the following.
$pp$
-syntactic is the following.
Theorem 3.8. Assume
![]() $\mathbf {K}= (K , \leq _{p})$
is an AEC with
$\mathbf {K}= (K , \leq _{p})$
is an AEC with
![]() $K \subseteq R\text {-Mod}$
for a fixed ring R. If the Galois-types in
$K \subseteq R\text {-Mod}$
for a fixed ring R. If the Galois-types in
![]() $\mathbf {K}$
are
$\mathbf {K}$
are
![]() $pp$
-syntactic, then
$pp$
-syntactic, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable for every
$\lambda $
-stable for every
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
such that
$\lambda \geq \operatorname {LS}(\mathbf {K})$
such that
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
.
$\lambda ^{|R| + \aleph _0}=\lambda $
.
Proof. Let
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
such that
$\lambda \geq \operatorname {LS}(\mathbf {K})$
such that
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
and
$\lambda ^{|R| + \aleph _0}=\lambda $
and
![]() $M \in \mathbf {K}_\lambda $
. Let
$M \in \mathbf {K}_\lambda $
. Let
![]() $\{ p_i : i < \kappa \}$
be an enumeration without repetitions of
$\{ p_i : i < \kappa \}$
be an enumeration without repetitions of
![]() $\mathbf {gS}(M)$
. For every
$\mathbf {gS}(M)$
. For every
![]() $i < \kappa $
fix a pair
$i < \kappa $
fix a pair
![]() $(a_i, M_i)$
such that
$(a_i, M_i)$
such that
![]() $p_i=\mathbf {gtp}(a_i/M; M_i)$
. Let
$p_i=\mathbf {gtp}(a_i/M; M_i)$
. Let
![]() $\Delta \subseteq \kappa $
such that
$\Delta \subseteq \kappa $
such that
![]() $| \Delta | \leq 2^{|R| + \aleph _0}$
and for every
$| \Delta | \leq 2^{|R| + \aleph _0}$
and for every
![]() $i < \kappa $
there is a
$i < \kappa $
there is a
![]() $j \in \Delta $
such that
$j \in \Delta $
such that
![]() $M_i$
is elementarily equivalent to
$M_i$
is elementarily equivalent to
![]() $M_j$
. This is possible because there are at most
$M_j$
. This is possible because there are at most
![]() $2^{|R| + \aleph _0}$
complete theories over R. For every
$2^{|R| + \aleph _0}$
complete theories over R. For every
![]() $j \in \Delta$
, let
$j \in \Delta$
, let
![]() $N_j$
be such that
$N_j$
be such that
![]() $M_j \leq_p N_j$
and
$M_j \leq_p N_j$
and
![]() $N_j$
is universal over
$N_j$
is universal over
![]() $M_j$
in
$M_j$
in
![]() $(R\text{-Mod}, \leq_p)$
.
$(R\text{-Mod}, \leq_p)$
.
Let
![]() $\Phi : \kappa \to \bigcup _{j \in \Delta } S_{pp}^{Th(N_j)}(M)$
be such that
$\Phi : \kappa \to \bigcup _{j \in \Delta } S_{pp}^{Th(N_j)}(M)$
be such that
![]() $\Phi (i)= pp(a_i/M, M_i)$
. By the choice of
$\Phi (i)= pp(a_i/M, M_i)$
. By the choice of
![]() $\Delta $
and the hypothesis that Galois-types in
$\Delta $
and the hypothesis that Galois-types in
![]() $\mathbf {K}$
are
$\mathbf {K}$
are
![]() $pp$
-syntactic we have that
$pp$
-syntactic we have that
![]() $\Phi $
is a well-defined injective function, so
$\Phi $
is a well-defined injective function, so
![]() $\kappa \leq |\bigcup _{j \in \Delta } S_{pp}^{Th(N_j)}(M)|$
. Observe that for every
$\kappa \leq |\bigcup _{j \in \Delta } S_{pp}^{Th(N_j)}(M)|$
. Observe that for every
![]() $j \in \Delta $
we have that
$j \in \Delta $
we have that
![]() $|S_{pp}^{Th(N_j)}(M)|=|S^{Th(N_j)}(M)|$
by
$|S_{pp}^{Th(N_j)}(M)|=|S^{Th(N_j)}(M)|$
by
![]() $pp$
-quantifier elimination (see [Reference Prest29, Section 2.4]). Hence
$pp$
-quantifier elimination (see [Reference Prest29, Section 2.4]). Hence
![]() $|\bigcup _{j \in \Delta } S_{pp}^{Th(N_j)}(M)|= |\bigcup _{j \in \Delta } S^{Th(N_j)}(M)|\leq \sum _{j \in \Delta } | S^{Th(N_j)}(M)|$
. Since every complete first-order theory of modules is
$|\bigcup _{j \in \Delta } S_{pp}^{Th(N_j)}(M)|= |\bigcup _{j \in \Delta } S^{Th(N_j)}(M)|\leq \sum _{j \in \Delta } | S^{Th(N_j)}(M)|$
. Since every complete first-order theory of modules is
![]() $\lambda $
-stable if
$\lambda $
-stable if
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
by [Reference Prest29, 3.1] and
$\lambda ^{|R| + \aleph _0}=\lambda $
by [Reference Prest29, 3.1] and
![]() $|\Delta | \leq 2^{|R| + \aleph _0}$
, we have that
$|\Delta | \leq 2^{|R| + \aleph _0}$
, we have that
![]() $\sum _{j \in \Delta } | S^{Th(N_j)}(M)| \leq \lambda $
. Hence,
$\sum _{j \in \Delta } | S^{Th(N_j)}(M)| \leq \lambda $
. Hence,
![]() $\kappa \leq \lambda $
. Therefore,
$\kappa \leq \lambda $
. Therefore,
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable.⊣
$\lambda $
-stable.⊣
We show that Galois-types are
![]() $pp$
-syntactic for classes satisfying Hypothesis 3.1. The result is similar to [Reference Kucera and Mazari-Armida18, 3.14], but the argument given there cannot be applied in this setting. A similar argument than that of [Reference Mazari-Armida27, 4.4] works in the more general setting of classes satisfying Hypothesis 3.1.
$pp$
-syntactic for classes satisfying Hypothesis 3.1. The result is similar to [Reference Kucera and Mazari-Armida18, 3.14], but the argument given there cannot be applied in this setting. A similar argument than that of [Reference Mazari-Armida27, 4.4] works in the more general setting of classes satisfying Hypothesis 3.1.
Lemma 3.9. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1. Then Galois-types in
$\mathbf {K}$
satisfies Hypothesis 3.1. Then Galois-types in
![]() $\mathbf {K}$
are
$\mathbf {K}$
are
![]() $pp$
-syntactic.
$pp$
-syntactic.
Proof. The forward direction is trivial so we show the backward direction. As K has the amalgamation property we may assume that
![]() $N_1=N_2$
and since K is closed under pure-injective envelopes we may assume that
$N_1=N_2$
and since K is closed under pure-injective envelopes we may assume that
![]() $N_1 = N_2$
is pure-injective. Let
$N_1 = N_2$
is pure-injective. Let
![]() $N = N_1 =N_2$
. Then by [Reference Ziegler48, 3.6] there is
$N = N_1 =N_2$
. Then by [Reference Ziegler48, 3.6] there is
with
![]() $h(\bar {b}_1)= \bar {b}_2$
where
$h(\bar {b}_1)= \bar {b}_2$
where
![]() $H^{N}(M \cup \{\bar {b}_i\})$
denotes the pure-injective envelope of
$H^{N}(M \cup \{\bar {b}_i\})$
denotes the pure-injective envelope of
![]() $M \cup \{\bar {b}_i\}$
inside N for every
$M \cup \{\bar {b}_i\}$
inside N for every
![]() $i \in \{ 1, 2 \}$
.
$i \in \{ 1, 2 \}$
.
As it might be the case that
![]() $H^{N}(M \cup \{\bar {b}_1 \})$
and
$H^{N}(M \cup \{\bar {b}_1 \})$
and
![]() $H^{N}(M \cup \{ \bar {b}_2 \})$
are not in K, we can not simply apply the amalgamation property and instead we have to do a similar argument to that of Lemma 3.5.
$H^{N}(M \cup \{ \bar {b}_2 \})$
are not in K, we can not simply apply the amalgamation property and instead we have to do a similar argument to that of Lemma 3.5.
Given
![]() $ i \in \{1, 2\}$
,
$ i \in \{1, 2\}$
,
![]() $H^{N}(M \cup \{\bar {b}_i\})$
is pure-injective so there is
$H^{N}(M \cup \{\bar {b}_i\})$
is pure-injective so there is
![]() $L_i$
such that
$L_i$
such that
![]() $N=H^{N}(M \cup \{\bar {b}_i\}) \oplus L_i$
. Let
$N=H^{N}(M \cup \{\bar {b}_i\}) \oplus L_i$
. Let
![]() $L= (H^{N}(M \cup \{\bar {b}_2\}) \oplus L_1) \oplus (H^{N}(M \cup \{\bar {b}_2\}) \oplus L_2)$
. Observe that
$L= (H^{N}(M \cup \{\bar {b}_2\}) \oplus L_1) \oplus (H^{N}(M \cup \{\bar {b}_2\}) \oplus L_2)$
. Observe that
![]() $L \in K$
by closure under direct sums and the fact that
$L \in K$
by closure under direct sums and the fact that
![]() $H^{N}(M \cup \{\bar {b}_1\}) \oplus L_1$
is isomorphic to
$H^{N}(M \cup \{\bar {b}_1\}) \oplus L_1$
is isomorphic to
![]() $H^{N}(M \cup \{\bar {b}_2\}) \oplus L_1$
. Define
$H^{N}(M \cup \{\bar {b}_2\}) \oplus L_1$
. Define
![]() $f: N=H^{N}(M \cup \{\bar {b}_1\}) \oplus L_1 \to L$
by
$f: N=H^{N}(M \cup \{\bar {b}_1\}) \oplus L_1 \to L$
by
![]() $f(s + l_1)=(h(s), l_1, h(s), 0)$
for
$f(s + l_1)=(h(s), l_1, h(s), 0)$
for
![]() $s \in H^{N}(M \cup \{\bar {b}_1\})$
and
$s \in H^{N}(M \cup \{\bar {b}_1\})$
and
![]() $l_1 \in L_1$
. Define
$l_1 \in L_1$
. Define
![]() $g: N=H^{N}(M \cup \{\bar {b}_2\}) \oplus L_2 \to L$
by
$g: N=H^{N}(M \cup \{\bar {b}_2\}) \oplus L_2 \to L$
by
![]() $g(q + l_2)=(q, 0, q, l_2)$
for
$g(q + l_2)=(q, 0, q, l_2)$
for
![]() $q \in H^{N}(M \cup \{\bar {b}_2\})$
and
$q \in H^{N}(M \cup \{\bar {b}_2\})$
and
![]() $l_2 \in L_2$
. It is easy to check that
$l_2 \in L_2$
. It is easy to check that
![]() $L, f, g$
witness that
$L, f, g$
witness that
![]() $\mathbf {gtp}(\bar {b}_{1}/M; N_1) = \mathbf {gtp}(\bar {b}_{2}/M; N_2)$
.⊣
$\mathbf {gtp}(\bar {b}_{1}/M; N_1) = \mathbf {gtp}(\bar {b}_{2}/M; N_2)$
.⊣
An immediate corollary is that the classes satisfying Hypothesis 3.1 are tame.
Corollary 3.10. If
![]() $\mathbf {K}$
satisfies Hypothesis 3.1, then
$\mathbf {K}$
satisfies Hypothesis 3.1, then
![]() $\mathbf {K}$
is fully
$\mathbf {K}$
is fully
![]() $(< \aleph _0)$
-tame.
$(< \aleph _0)$
-tame.
The next results follows from Theorem 3.8 and Lemma 3.9.
Theorem 3.11. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1 and
$\mathbf {K}$
satisfies Hypothesis 3.1 and
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
. If
$\lambda \geq \operatorname {LS}(\mathbf {K})$
. If
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
, then
$\lambda ^{|R| + \aleph _0}=\lambda $
, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable.
$\lambda $
-stable.
Then from [Reference Kucera and Mazari-Armida18, 3.20] we can conclude the existence of universal models.
Corollary 3.12. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1 and
$\mathbf {K}$
satisfies Hypothesis 3.1 and
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
. If
$\lambda \geq \operatorname {LS}(\mathbf {K})$
. If
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
or
$\lambda ^{|R| + \aleph _0}=\lambda $
or
![]() $\forall \mu < \lambda ( \mu ^{|R| + \aleph _0} < \lambda )$
, then
$\forall \mu < \lambda ( \mu ^{|R| + \aleph _0} < \lambda )$
, then
![]() $\mathbf {K}$
has a universal model of cardinality
$\mathbf {K}$
has a universal model of cardinality
![]() $\lambda $
.
$\lambda $
.
3.2 Limit models and superstability
Since K has joint embedding, amalgamation and no maximal models, it follows from [Reference Shelah44, section II.1.16] that
![]() $\mathbf {K}$
has a
$\mathbf {K}$
has a
![]() $(\lambda , \alpha )$
-limit model if
$(\lambda , \alpha )$
-limit model if
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
,
$\lambda ^{|R| + \aleph _0}=\lambda $
,
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
and
$\lambda \geq \operatorname {LS}(\mathbf {K})$
and
![]() $\alpha < \lambda ^+$
is a limit ordinal. We characterize limit models with chains of big cofinality. This extends [Reference Kucera and Mazari-Armida18, 4.5] and [Reference Mazari-Armida27, 4.9] to any class satisfying Hypothesis 3.1.
$\alpha < \lambda ^+$
is a limit ordinal. We characterize limit models with chains of big cofinality. This extends [Reference Kucera and Mazari-Armida18, 4.5] and [Reference Mazari-Armida27, 4.9] to any class satisfying Hypothesis 3.1.
Lemma 3.13. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1 and
$\mathbf {K}$
satisfies Hypothesis 3.1 and
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})^+$
. If M is a
$\lambda \geq \operatorname {LS}(\mathbf {K})^+$
. If M is a
![]() $(\lambda , \alpha )$
-limit model and
$(\lambda , \alpha )$
-limit model and
![]() $\operatorname {\mathrm {cf}}(\alpha )\geq (|R| + \aleph _0)^+$
, then M is pure-injective.
$\operatorname {\mathrm {cf}}(\alpha )\geq (|R| + \aleph _0)^+$
, then M is pure-injective.
Proof. Fix
![]() $\{ M_i : i < \alpha \}$
a witness to the fact that M is a
$\{ M_i : i < \alpha \}$
a witness to the fact that M is a
![]() $(\lambda , \alpha )$
-limit model. We show that every
$(\lambda , \alpha )$
-limit model. We show that every
![]() $p(x) \ M$
-consistent
$p(x) \ M$
-consistent
![]() $pp$
-type over
$pp$
-type over
![]() $A \subseteq M$
with
$A \subseteq M$
with
![]() $|A| \leq |R| + \aleph _0$
is realized in M.Footnote
4
This enough to show that M is pure-injective by [Reference Prest29, 2.8].
$|A| \leq |R| + \aleph _0$
is realized in M.Footnote
4
This enough to show that M is pure-injective by [Reference Prest29, 2.8].
Observe that p is a
![]() $PE(M)$
-consistent
$PE(M)$
-consistent
![]() $pp$
-type as
$pp$
-type as
![]() $M \preceq PE(M)$
. Since
$M \preceq PE(M)$
. Since
![]() $PE(M)$
is pure-injective, it is saturated for
$PE(M)$
is pure-injective, it is saturated for
![]() $pp$
-types [Reference Prest29, 2.8], so there is
$pp$
-types [Reference Prest29, 2.8], so there is
![]() $a \in PE(M)$
realizing p. As
$a \in PE(M)$
realizing p. As
![]() $\operatorname {\mathrm {cf}}(\alpha )\geq (|R| + \aleph _0)^+$
, there is
$\operatorname {\mathrm {cf}}(\alpha )\geq (|R| + \aleph _0)^+$
, there is
![]() $i < \alpha $
such that
$i < \alpha $
such that
![]() $A \subseteq M_i$
. Applying downward Löwenheim–Skolem to
$A \subseteq M_i$
. Applying downward Löwenheim–Skolem to
![]() $M_i \cup \{ a \}$
in
$M_i \cup \{ a \}$
in
![]() $PE(M)$
we obtain
$PE(M)$
we obtain
![]() $N \in \mathbf {K}_\lambda $
with
$N \in \mathbf {K}_\lambda $
with
![]() $M_i \leq _p N$
and
$M_i \leq _p N$
and
![]() $a \in N$
. Then there is
$a \in N$
. Then there is
![]() $f: N \xrightarrow [M_i]{} M$
because
$f: N \xrightarrow [M_i]{} M$
because
![]() $M_{i + 1}$
is universal over
$M_{i + 1}$
is universal over
![]() $M_i$
. Hence
$M_i$
. Hence
![]() $f(a) \in M$
realizes p.⊣
$f(a) \in M$
realizes p.⊣
Since K is closed under direct sums, the usual argument [Reference Kucera and Mazari-Armida18, 4.9] can be use to characterize limit models of countable cofinality.
Lemma 3.14. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1 and
$\mathbf {K}$
satisfies Hypothesis 3.1 and
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})^+$
. If M is a
$\lambda \geq \operatorname {LS}(\mathbf {K})^+$
. If M is a
![]() $(\lambda , \omega )$
-limit model and N is a
$(\lambda , \omega )$
-limit model and N is a
![]() $(\lambda , (|R| + \aleph _0)^+)$
-limit model, then M is isomorphic to
$(\lambda , (|R| + \aleph _0)^+)$
-limit model, then M is isomorphic to
![]() $N^{(\aleph _0)}$
.
$N^{(\aleph _0)}$
.
Moreover, any two limit models of
![]() $\mathbf {K}$
are elementarily equivalent. The proof is similar to that of [Reference Kucera and Mazari-Armida18, 4.3] so we omit it.
$\mathbf {K}$
are elementarily equivalent. The proof is similar to that of [Reference Kucera and Mazari-Armida18, 4.3] so we omit it.
Lemma 3.15. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1. If
$\mathbf {K}$
satisfies Hypothesis 3.1. If
![]() $M, N$
are limit models of
$M, N$
are limit models of
![]() $\mathbf {K}$
, then M and N are elementary equivalent.
$\mathbf {K}$
, then M and N are elementary equivalent.
Remark 3.16. Lemmas 3.13 and 3.14 describe limit models with long chains and the limit model with the shortest chain under Hypothesis 3.1. We do not know how the other limit models look like except from the fact that they are elementarily equivalent to the ones we understand by Lemma 3.15. Since this is all we need to characterize superstability, we do not explore this any further in this paper. Nevertheless, we think that understanding the other limit models could help better understand the classes satisfying Hypothesis 3.1.
Due to Lemma 3.15, it makes sense to introduce the following first-order theory:
Notation 3.17. For
![]() $\mathbf {K}$
satisfying Hypothesis 3.1, let
$\mathbf {K}$
satisfying Hypothesis 3.1, let
![]() $\tilde {M}_{\mathbf {K}}$
be the
$\tilde {M}_{\mathbf {K}}$
be the
![]() $(2^{\operatorname {LS}(\mathbf {K})}, \omega )$
-limit model of
$(2^{\operatorname {LS}(\mathbf {K})}, \omega )$
-limit model of
![]() $\mathbf {K}$
and
$\mathbf {K}$
and
![]() $\tilde {T}_{\mathbf {K}}=Th(\tilde {M}_{\mathbf {K}})$
.
$\tilde {T}_{\mathbf {K}}=Th(\tilde {M}_{\mathbf {K}})$
.
In [Reference Mazari-Armida26, Section 4.1] a similar theory, called
![]() $\tilde {T}$
there, was introduced. There it was shown that there was a very close relation between the AEC
$\tilde {T}$
there, was introduced. There it was shown that there was a very close relation between the AEC
![]() $\mathbf {K}$
and
$\mathbf {K}$
and
![]() $\tilde {T}_{\mathbf {K}}$
. We do not think that this is the case when
$\tilde {T}_{\mathbf {K}}$
. We do not think that this is the case when
![]() $\mathbf {K}$
satisfies Hypothesis 3.1 and is not first-order axiomatizable. We think that this is the case because there can be models of
$\mathbf {K}$
satisfies Hypothesis 3.1 and is not first-order axiomatizable. We think that this is the case because there can be models of
![]() $\tilde {T}_{\mathbf {K}}$
that are not in
$\tilde {T}_{\mathbf {K}}$
that are not in
![]() $\mathbf {K}$
. Nevertheless, stability transfers from
$\mathbf {K}$
. Nevertheless, stability transfers from
![]() $\tilde {T}_{\mathbf {K}}$
to
$\tilde {T}_{\mathbf {K}}$
to
![]() $\mathbf {K}$
. As the proof is similar to that of [Reference Mazari-Armida26, 4.9] we omit it.
$\mathbf {K}$
. As the proof is similar to that of [Reference Mazari-Armida26, 4.9] we omit it.
Lemma 3.18. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1 and let
$\mathbf {K}$
satisfies Hypothesis 3.1 and let
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
. If
$\lambda \geq \operatorname {LS}(\mathbf {K})$
. If
![]() $\tilde {T}_{\mathbf {K}}$
is
$\tilde {T}_{\mathbf {K}}$
is
![]() $\lambda $
-stable, then
$\lambda $
-stable, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable.
$\lambda $
-stable.
Remark 3.19. In [Reference Mazari-Armida26, 4.9], it is shown that the converse is true if
![]() $\mathbf {K}$
is first-order axiomatizable. We do not think that the converse is true in this more general setting, but we do not have a counterexample.
$\mathbf {K}$
is first-order axiomatizable. We do not think that the converse is true in this more general setting, but we do not have a counterexample.
We characterize superstability for classes satisfying Hypothesis 3.1. The next result extends [Reference Mazari-Armida26, 4.26] to classes not necessarily axiomatizable by a first-order theory and [Reference Mazari-Armida27, 4.12] to a different class than that of Example 3.2.(5).s
Theorem 3.20. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 3.1. The following are equivalent.
$\mathbf {K}$
satisfies Hypothesis 3.1. The following are equivalent.
-
(1)
 $\mathbf {K}$
is superstable.
$\mathbf {K}$
is superstable. -
(2) There is a
 $\lambda \geq \operatorname {LS}(\mathbf {K})^+$
such that
$\lambda \geq \operatorname {LS}(\mathbf {K})^+$
such that
 $\mathbf {K}$
has uniqueness of limit models of cardinality
$\mathbf {K}$
has uniqueness of limit models of cardinality
 $\lambda $
.
$\lambda $
. -
(3) Every limit model in
 $\mathbf {K}$
is
$\mathbf {K}$
is
 $\Sigma $
-pure-injective.
$\Sigma $
-pure-injective. -
(4) Every model in
 $\mathbf {K}$
is pure-injective.
$\mathbf {K}$
is pure-injective. -
(5) For every
 $\lambda \geq \operatorname {LS}(\mathbf {K})$
,
$\lambda \geq \operatorname {LS}(\mathbf {K})$
,
 $\mathbf {K}$
has uniqueness of limit models of cardinality
$\mathbf {K}$
has uniqueness of limit models of cardinality
 $\lambda $
.s
$\lambda $
.s
Proof. (1)
![]() $\Rightarrow $
(2) Clear.
$\Rightarrow $
(2) Clear.
(2)
![]() $\Rightarrow $
(3) Let
$\Rightarrow $
(3) Let
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})^+$
such that
$\lambda \geq \operatorname {LS}(\mathbf {K})^+$
such that
![]() $\mathbf {K}$
has uniqueness of limit models of size
$\mathbf {K}$
has uniqueness of limit models of size
![]() $\lambda $
. Let M be a
$\lambda $
. Let M be a
![]() $(\lambda , (|R| + \aleph _0)^+)$
-limit model in
$(\lambda , (|R| + \aleph _0)^+)$
-limit model in
![]() $\mathbf {K}$
. It follows from Lemma 3.14 that
$\mathbf {K}$
. It follows from Lemma 3.14 that
![]() $M^{(\aleph _0)}$
is the
$M^{(\aleph _0)}$
is the
![]() $(\lambda , \omega )$
-limit model. As
$(\lambda , \omega )$
-limit model. As
![]() $\mathbf {K}$
has uniqueness of limit models of size
$\mathbf {K}$
has uniqueness of limit models of size
![]() $\lambda $
, we have that M is isomorphic to
$\lambda $
, we have that M is isomorphic to
![]() $M^{(\aleph _0)}$
. Since M is pure-injective by Lemma 3.13, it follows that
$M^{(\aleph _0)}$
. Since M is pure-injective by Lemma 3.13, it follows that
![]() $M^{(\aleph _0)}$
is pure-injective. Hence M is
$M^{(\aleph _0)}$
is pure-injective. Hence M is
![]() $\Sigma $
-pure-injective. Since limit models are elementarily equivalent by Lemma 3.15 and
$\Sigma $
-pure-injective. Since limit models are elementarily equivalent by Lemma 3.15 and
![]() $\Sigma $
-pure-injectivity is preserved under elementarily equivalence by Fact 2.7, it follows that every limit model is
$\Sigma $
-pure-injectivity is preserved under elementarily equivalence by Fact 2.7, it follows that every limit model is
![]() $\Sigma $
-pure-injective.
$\Sigma $
-pure-injective.
(3)
![]() $\Rightarrow $
(4) Let
$\Rightarrow $
(4) Let
![]() $N\in \mathbf {K}$
and
$N\in \mathbf {K}$
and
![]() $N'$
be a
$N'$
be a
![]() $(\|N\|^{|R| + \aleph _0}, \omega )$
-limit model, this exists by Theorem 3.11. Then there is
$(\|N\|^{|R| + \aleph _0}, \omega )$
-limit model, this exists by Theorem 3.11. Then there is
![]() $f: N \to N'$
a pure embedding by [Reference Mazari-Armida24, 2.10]. Since
$f: N \to N'$
a pure embedding by [Reference Mazari-Armida24, 2.10]. Since
![]() $N'$
is
$N'$
is
![]() $\Sigma $
-pure-injective and f is a pure embedding, it follows from Fact 2.7 that N is
$\Sigma $
-pure-injective and f is a pure embedding, it follows from Fact 2.7 that N is
![]() $\Sigma $
-pure-injective. Hence every model in
$\Sigma $
-pure-injective. Hence every model in
![]() $\mathbf {K}$
is pure-injective.
$\mathbf {K}$
is pure-injective.
(4)
![]() $\Rightarrow $
(5) Let M be a
$\Rightarrow $
(5) Let M be a
![]() $(2^{\operatorname {LS}(\mathbf {K})}, \omega )$
-limit model. By (4) and closure under direct sums we have that M is
$(2^{\operatorname {LS}(\mathbf {K})}, \omega )$
-limit model. By (4) and closure under direct sums we have that M is
![]() $\Sigma $
-pure-injective, so
$\Sigma $
-pure-injective, so
![]() $Th(M)$
is
$Th(M)$
is
![]() $\lambda $
-stable for every
$\lambda $
-stable for every
![]() $\lambda \geq |R| + \aleph _0$
by Fact 2.7. As
$\lambda \geq |R| + \aleph _0$
by Fact 2.7. As
![]() $Th(M)=\tilde {T}_{\mathbf {K}}$
by definition, it follows from Lemma 3.18 that
$Th(M)=\tilde {T}_{\mathbf {K}}$
by definition, it follows from Lemma 3.18 that
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable for every
$\lambda $
-stable for every
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
. Therefore, by [Reference Shelah44, Section II.1.16] there exist a
$\lambda \geq \operatorname {LS}(\mathbf {K})$
. Therefore, by [Reference Shelah44, Section II.1.16] there exist a
![]() $\lambda $
-limit model for every
$\lambda $
-limit model for every
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})$
.
$\lambda \geq \operatorname {LS}(\mathbf {K})$
.
Regarding uniqueness, observe that given M and
![]() $N \ \lambda $
-limit models, there are
$N \ \lambda $
-limit models, there are
![]() $f: M \to N$
and
$f: M \to N$
and
![]() $g: N \to M$
pure embeddings by [Reference Mazari-Armida24, 2.10]. Since we have that M and N are pure-injective, it follows from Fact 2.6 that M and N are isomorphic.
$g: N \to M$
pure embeddings by [Reference Mazari-Armida24, 2.10]. Since we have that M and N are pure-injective, it follows from Fact 2.6 that M and N are isomorphic.
(5)
![]() $\Rightarrow $
(1) Clear.⊣
$\Rightarrow $
(1) Clear.⊣
Remark 3.21. It can also be shown as in [Reference Mazari-Armida26, 4.26] that
![]() $\mathbf {K}$
is superstable if and only if there exists
$\mathbf {K}$
is superstable if and only if there exists
![]() $\lambda \geq \operatorname {LS}(\mathbf {K})^+$
such that
$\lambda \geq \operatorname {LS}(\mathbf {K})^+$
such that
![]() $\mathbf {K}$
has a
$\mathbf {K}$
has a
![]() $\Sigma $
-pure-injective universal model of cardinality
$\Sigma $
-pure-injective universal model of cardinality
![]() $\lambda $
.
$\lambda $
.
3.3 Characterizing several classes of rings
We will use the results of the preceding subsection to characterize noetherian rings, pure-semimple rings, Dedekind domains, and fields via superstability.
Recall that a module M is injective if it is a direct summand of every module containing it. The next result will be useful.
Fact 3.22 [Reference Prest30, 4.4.17].
Let R be a ring. The following are equivalent.
-
(1) R is left noetherian.
-
(2) The class of absolutely pure left R-modules is the same as the class of injective left R-modules.
-
(3) Every direct sum of injective left R-modules is injective.
We begin by giving two new characterizations of noetherian rings. The equivalence between (1) and (2) extends [Reference Mazari-Armida26, 4.30]. Recall that
![]() $R\text {-AbsP}$
is the class of absolutely pure R-modules and that
$R\text {-AbsP}$
is the class of absolutely pure R-modules and that
![]() $R\text {-l-inj}$
is the class of locally injective R-modules, these were introduced in Example 3.3.
$R\text {-l-inj}$
is the class of locally injective R-modules, these were introduced in Example 3.3.
Theorem 3.23. Let R be a ring. The following are equivalent.
-
(1) R is left noetherian.
-
(2)
 $(R\text {-AbsP}, \leq _{p})$
is superstable.
$(R\text {-AbsP}, \leq _{p})$
is superstable. -
(3)
 $(R\text {-l-inj}, \leq _p)$
is superstable.
$(R\text {-l-inj}, \leq _p)$
is superstable.
Proof. Recall that absolutely pure modules and locally injective modules satisfy Hypothesis 3.1, so we can use the results from the previous subsection. More precisely, we use Theorem 3.20.(4) to show the equivalences.
(1)
![]() $\Rightarrow $
(2) If R is noetherian, then every absolutely pure module is injective by Fact 3.22. Hence, every absolutely pure module is pure-injective. So the result follows from Theorem 3.20.
$\Rightarrow $
(2) If R is noetherian, then every absolutely pure module is injective by Fact 3.22. Hence, every absolutely pure module is pure-injective. So the result follows from Theorem 3.20.
(2)
![]() $\Rightarrow $
(3) Every locally injective module is absolutely pure by [Reference Ramamurthi and Rangaswamy32, 3.1]. Then it follows that every locally injective module is pure-injective by (2). Hence, the class of locally injective R-modules is superstable.
$\Rightarrow $
(3) Every locally injective module is absolutely pure by [Reference Ramamurthi and Rangaswamy32, 3.1]. Then it follows that every locally injective module is pure-injective by (2). Hence, the class of locally injective R-modules is superstable.
(3)
![]() $\Rightarrow $
(1) We show that the direct sum of injective modules is injective, this is enough by Fact 3.22. Let
$\Rightarrow $
(1) We show that the direct sum of injective modules is injective, this is enough by Fact 3.22. Let
![]() $\{ M_i : i \in I \}$
be a family of injective modules. As they are all locally injective, we have that
$\{ M_i : i \in I \}$
be a family of injective modules. As they are all locally injective, we have that
![]() $\bigoplus _{i \in I} M_i$
is locally injective. Moreover, as
$\bigoplus _{i \in I} M_i$
is locally injective. Moreover, as
![]() $(R\text {-l-inj}, \leq _p)$
is superstable, we have that
$(R\text {-l-inj}, \leq _p)$
is superstable, we have that
![]() $\bigoplus _{i \in I} M_i$
is also pure-injective by Theorem 3.20. Recall that locally injective modules are absolutely pure, so
$\bigoplus _{i \in I} M_i$
is also pure-injective by Theorem 3.20. Recall that locally injective modules are absolutely pure, so
![]() $\bigoplus _{i \in I} M_i$
is absolutely pure and pure-injective. Therefore,
$\bigoplus _{i \in I} M_i$
is absolutely pure and pure-injective. Therefore,
![]() $\bigoplus_{i \in I} M_i$
is injective. Hence R is noetherian.⊣
$\bigoplus_{i \in I} M_i$
is injective. Hence R is noetherian.⊣
We use the above result to study the class of injective R-modules with pure embeddings, we will denote it by
![]() $(R\text {-Inj}, \leq _{p})$
.
$(R\text {-Inj}, \leq _{p})$
.
Corollary 3.24. Let R be a ring.
![]() $(R\text {-Inj}, \leq _{p})$
is an AEC if and only if R is left noetherian. Moreover, if R is left noetherian, then
$(R\text {-Inj}, \leq _{p})$
is an AEC if and only if R is left noetherian. Moreover, if R is left noetherian, then
![]() $(R\text {-Inj}, \leq _{p})$
is a superstable AEC.
$(R\text {-Inj}, \leq _{p})$
is a superstable AEC.
Proof. If
![]() $(R\text {-Inj}, \leq _{p})$
is an AEC then the direct sum of injective modules is an injective module because injective modules are closed under finite direct sums. Hence R is left noetherian. On the other hand, if R is left noetherian, then injective modules are the same as absolutely pure modules by Fact 3.22. Hence
$(R\text {-Inj}, \leq _{p})$
is an AEC then the direct sum of injective modules is an injective module because injective modules are closed under finite direct sums. Hence R is left noetherian. On the other hand, if R is left noetherian, then injective modules are the same as absolutely pure modules by Fact 3.22. Hence
![]() $(R\text {-Inj}, \leq _{p})$
is an AEC.
$(R\text {-Inj}, \leq _{p})$
is an AEC.
The moreover part follows directly from Theorem 3.23.⊣
The next corollary shows a connection between being good in the stability hierarchy and being good in the axiomatizability hierarchy.
Corollary 3.25. Let R be a ring.
-
(1) If
 $(R\text {-AbsP}, \leq _{p})$
is superstable, then the class of absolutely pure left R-modules is first-order axiomatizable.
$(R\text {-AbsP}, \leq _{p})$
is superstable, then the class of absolutely pure left R-modules is first-order axiomatizable. -
(2) If
 $(R\text {-l-inj}, \leq _{p})$
is superstable, then the class of locally injective left R-modules is first-order axiomatizable.
$(R\text {-l-inj}, \leq _{p})$
is superstable, then the class of locally injective left R-modules is first-order axiomatizable.
Proof.
-
(1) Since
 $(R\text {-AbsP}, \leq _{p})$
is superstable, then by Theorem 3.23 R is left noetherian. Then R is left coherent, so it follows from [Reference Prest30, 3.4.24] that absolutely pure modules are first-order axiomatizable
$(R\text {-AbsP}, \leq _{p})$
is superstable, then by Theorem 3.23 R is left noetherian. Then R is left coherent, so it follows from [Reference Prest30, 3.4.24] that absolutely pure modules are first-order axiomatizable -
(2) The proof is similar to that of (1), using that if R is noetherian then the class of absolutely pure modules is the same as the class of locally injective modules.⊣
We turn our attention to pure-semisimple rings. A ring is pure-semisimple if and only if every R-module is pure-injective. These have been thoroughly studied [Reference Auslander1, Reference Auslander2, Reference Chase8, Reference Mazari-Armida26, Reference Prest28, Reference Prest30, Reference Simson39–Reference Simson41, Reference Zimmermann-Huisgen49]. Recall that
![]() $R\text {-l-pi}$
is the class of locally pure-injective R-modules, these were introduced in Example 3.3. The equivalence between (1) and (2) of the next assertion was obtained in [Reference Mazari-Armida26, 4.28].
$R\text {-l-pi}$
is the class of locally pure-injective R-modules, these were introduced in Example 3.3. The equivalence between (1) and (2) of the next assertion was obtained in [Reference Mazari-Armida26, 4.28].
Theorem 3.26. Let R be a ring. The following are equivalent.
-
(1) R is left pure-semisimple.
-
(2)
 $(R\text {-Mod}, \leq _{p})$
is superstable.
$(R\text {-Mod}, \leq _{p})$
is superstable. -
(3)
 $(R\text {-l-pi}, \leq _p)$
is superstable.
$(R\text {-l-pi}, \leq _p)$
is superstable.
Proof. Recall that R-modules and locally pure-injective R-modules satisfy Hypothesis 3.1. We use Theorem 3.20.(4) to show the equivalences. The equivalence between (1) and (2) and the direction (2) to (3) are straightforward. We show (3) to (1).
Let M be an R-module, then
![]() $PE(M)$
is locally pure-injective and
$PE(M)$
is locally pure-injective and
![]() $M \leq _p PE(M)$
. Observe that
$M \leq _p PE(M)$
. Observe that
![]() $PE(M)^{(\aleph _0)}$
is locally pure-injective. Then
$PE(M)^{(\aleph _0)}$
is locally pure-injective. Then
![]() $PE(M)^{(\aleph _0)}$
is pure-injective by hypothesis (3), so
$PE(M)^{(\aleph _0)}$
is pure-injective by hypothesis (3), so
![]() $PE(M)$
is
$PE(M)$
is
![]() $\Sigma $
-pure-injective. Hence, M is pure-injective by Fact 2.7. Therefore, R is left pure-semisimple.⊣
$\Sigma $
-pure-injective. Hence, M is pure-injective by Fact 2.7. Therefore, R is left pure-semisimple.⊣
We can obtain an analogous result to Corollary 3.24 by substituting the class of injective modules by that of pure-injective modules. We denote by
![]() $R\text {-pi}$
the class of pure-injective R-modules.
$R\text {-pi}$
the class of pure-injective R-modules.
Corollary 3.27. Let R be a ring.
![]() $(R\text {-pi}, \leq _{p})$
is an AEC if and only if R is left pure-semisimple. Moreover, if R is left pure-semisimple, then
$(R\text {-pi}, \leq _{p})$
is an AEC if and only if R is left pure-semisimple. Moreover, if R is left pure-semisimple, then
![]() $(R\text {-pi}, \leq _{p})$
is a superstable AEC.
$(R\text {-pi}, \leq _{p})$
is a superstable AEC.
Proof. If
![]() $(R\text {-pi}, \leq _{p})$
is an AEC, then
$(R\text {-pi}, \leq _{p})$
is an AEC, then
![]() $M^{(\aleph _0)}=\bigcup _{n < \omega } M^{n}$
is pure-injective for every pure-injective module M as pure-injective modules are closed under finite direct sums. So every pure-injective module is
$M^{(\aleph _0)}=\bigcup _{n < \omega } M^{n}$
is pure-injective for every pure-injective module M as pure-injective modules are closed under finite direct sums. So every pure-injective module is
![]() $\Sigma $
-pure-injective. Then doing an argument similar to that of the previous result, one can show that R is left pure-semisimple. On the other hand, if R is left pure-semisimple, then all modules are pure-injective. Hence
$\Sigma $
-pure-injective. Then doing an argument similar to that of the previous result, one can show that R is left pure-semisimple. On the other hand, if R is left pure-semisimple, then all modules are pure-injective. Hence
![]() $(R\text {-pi}, \leq _{p})$
is an AEC.
$(R\text {-pi}, \leq _{p})$
is an AEC.
The moreover part follows directly from Theorem 3.26.⊣
We also get a relation between being good in the stability hierarchy and being good in the axiomatizability hierarchy for locally pure-injective modules.
Corollary 3.28. Let R be ring. If
![]() $(R\text {-l-pi}, \leq _{p})$
is superstable, then the class of locally pure-injective left R-modules is the same as the class of left R-modules. So clearly, first-order axiomatizable.
$(R\text {-l-pi}, \leq _{p})$
is superstable, then the class of locally pure-injective left R-modules is the same as the class of left R-modules. So clearly, first-order axiomatizable.
Proof. Since
![]() $(R\text {-l-pi}, \leq _{p})$
is superstable, then by Theorem 3.26 R is left pure-semisimple. Hence, every R-module is pure-injective, so in particular locally pure-injective.⊣
$(R\text {-l-pi}, \leq _{p})$
is superstable, then by Theorem 3.26 R is left pure-semisimple. Hence, every R-module is pure-injective, so in particular locally pure-injective.⊣
Corollaries 3.25 and 3.28 may suggest that given an AEC of modules satisfying Hypothesis 3.1, it follows that if the class is superstable, then the class is first-order axiomatizable. This is not the case as witnessed by the next example.
Example 3.29. It was shown in [Reference Mazari-Armida27, 3.15] that
![]() $(R\text {-Flat}, \leq _{p})$
is superstable if and only if R is left perfect. It is known [Reference Sabbagh and Eklof36, Theorem 4] that the class of flat left R-modules is first-order axiomatizable if and only if R is right coherent. Therefore, the ring R described in [Reference Rothmaler33, 3.3] is such that
$(R\text {-Flat}, \leq _{p})$
is superstable if and only if R is left perfect. It is known [Reference Sabbagh and Eklof36, Theorem 4] that the class of flat left R-modules is first-order axiomatizable if and only if R is right coherent. Therefore, the ring R described in [Reference Rothmaler33, 3.3] is such that
![]() $(R\text {-Flat}, \leq _{p})$
satisfies Hypothesis 3.1,
$(R\text {-Flat}, \leq _{p})$
satisfies Hypothesis 3.1,
![]() $(R\text {-Flat}, \leq _{p})$
is superstable and
$(R\text {-Flat}, \leq _{p})$
is superstable and
![]() $R\text {-Flat}$
is not first-order axiomatizable.
$R\text {-Flat}$
is not first-order axiomatizable.
As mentioned in the introduction, the main focus of the paper is Question 1.1. The results of this section can be used to characterized those rings for which all AECs closed under direct sums are superstable.
Lemma 3.30. Let R be a ring. The following are equivalent.
-
(1) R is left pure-semisimple.
-
(2) Every AEC
 $\mathbf {K}= (K , \leq _{p})$
with
$\mathbf {K}= (K , \leq _{p})$
with
 $K \subseteq R\text {-Mod}$
, such that K is closed under direct sums, is superstable.
$K \subseteq R\text {-Mod}$
, such that K is closed under direct sums, is superstable.
Proof. The backward direction follows from Theorem 3.26 as
![]() $(R\text {-Mod}, \leq _p)$
satisfies the hypothesis of (2). We show the forward direction.
$(R\text {-Mod}, \leq _p)$
satisfies the hypothesis of (2). We show the forward direction.
Let
![]() $\mathbf {K}$
be a class satisfying the hypothesis of (2). Then
$\mathbf {K}$
be a class satisfying the hypothesis of (2). Then
![]() $\mathbf {K}$
is closed under pure-injective envelopes as every module is pure-injective since we are assuming that the ring is left pure-semisimple. Hence,
$\mathbf {K}$
is closed under pure-injective envelopes as every module is pure-injective since we are assuming that the ring is left pure-semisimple. Hence,
![]() $\mathbf {K}$
satisfies Hypothesis 3.1. Therefore,
$\mathbf {K}$
satisfies Hypothesis 3.1. Therefore,
![]() $\mathbf {K}$
is superstable by Theorem 3.20.(4) and the hypothesis on the ring.⊣
$\mathbf {K}$
is superstable by Theorem 3.20.(4) and the hypothesis on the ring.⊣
The next well-known ring theoretic result follows from the above lemma, Theorem 3.23 and [Reference Mazari-Armida27, 3.15].
Corollary 3.31. Assume R is an associative ring with unity. If R is left pure-semisimple, then R is left noetherian and left perfect.
We finish this subsection by applying the technology developed in this section to integral domains. Given an integral domain R, we study the class of divisible R-modules, denoted by
![]() $R\text {-Div}$
, and the class torsion-free R-modules, denoted by
$R\text {-Div}$
, and the class torsion-free R-modules, denoted by
![]() $R\text {-TF}$
. A module M is a divisible R-module if for every
$R\text {-TF}$
. A module M is a divisible R-module if for every
![]() $m \in M$
and
$m \in M$
and
![]() $r \neq 0 \in R$
, there is
$r \neq 0 \in R$
, there is
![]() $n \in M$
such that
$n \in M$
such that
![]() $rn = m$
. A module M is a torsion-free R-module if for every
$rn = m$
. A module M is a torsion-free R-module if for every
![]() $m \neq 0 \in M$
and every
$m \neq 0 \in M$
and every
![]() $r\neq 0 \in R$
,
$r\neq 0 \in R$
,
![]() $rm \neq 0$
. It is easy to show that
$rm \neq 0$
. It is easy to show that
![]() $(R\text {-Div}, \leq _p)$
and
$(R\text {-Div}, \leq _p)$
and
![]() $(R\text {-TF}, \leq _p)$
both satisfy Hypothesis 3.1, this is the case as they are both definable classes in the sense of Example 3.3.(6).
$(R\text {-TF}, \leq _p)$
both satisfy Hypothesis 3.1, this is the case as they are both definable classes in the sense of Example 3.3.(6).
Lemma 3.32. Let R be an integral domain.
-
(1) R is a Dedekind domain if and only if
 $(R\text {-Div}, \leq _p)$
is superstable.
$(R\text {-Div}, \leq _p)$
is superstable. -
(2) R is a field if and only if
 $(R\text {-TF}, \leq _p)$
is superstable.
$(R\text {-TF}, \leq _p)$
is superstable.
Proof.
-
(1)
 $\Rightarrow $
: Since R is a Dedekind domain, every divisible R-module is injective by [Reference Rotman35, 4.24]. As injective modules are pure-injective,
$\Rightarrow $
: Since R is a Dedekind domain, every divisible R-module is injective by [Reference Rotman35, 4.24]. As injective modules are pure-injective,
 $(R\text {-Div}, \leq _p)$
is superstable by Theorem 3.20.
$(R\text {-Div}, \leq _p)$
is superstable by Theorem 3.20. $\Leftarrow $
: Recall that a module is h-divisible if it is the epimorphic image of an injective module. Therefore, the class of h-divisible R-modules is contained in the class of divisible R-modules. Then every h-divisible R-module is pure-injective by Theorem 3.20. Therefore, R is a Dedekind domain by [Reference Salce37, 2.5].
$\Leftarrow $
: Recall that a module is h-divisible if it is the epimorphic image of an injective module. Therefore, the class of h-divisible R-modules is contained in the class of divisible R-modules. Then every h-divisible R-module is pure-injective by Theorem 3.20. Therefore, R is a Dedekind domain by [Reference Salce37, 2.5]. -
(2)
 $\Rightarrow $
: If R is a field, clearly R is a Prüfer domain. So the class of flat modules is the same as the class of torsion-free modules by [Reference Rotman35, 4.35]. Then
$\Rightarrow $
: If R is a field, clearly R is a Prüfer domain. So the class of flat modules is the same as the class of torsion-free modules by [Reference Rotman35, 4.35]. Then
 $(R\text {-TF}, \leq _p)$
is superstable since R is perfect and by [Reference Mazari-Armida27, 3.15].
$(R\text {-TF}, \leq _p)$
is superstable since R is perfect and by [Reference Mazari-Armida27, 3.15]. $\Leftarrow $
: It follows from Theorem 3.20 and [Reference Salce37, 2.3] that R is a Prüfer domain. So, as before, the class of flat modules is the same as the class of torsion-free modules. Then R is left perfect by [Reference Mazari-Armida27, 3.15]. Therefore, R is a field by [Reference Salce38, 2.3].⊣
$\Leftarrow $
: It follows from Theorem 3.20 and [Reference Salce37, 2.3] that R is a Prüfer domain. So, as before, the class of flat modules is the same as the class of torsion-free modules. Then R is left perfect by [Reference Mazari-Armida27, 3.15]. Therefore, R is a field by [Reference Salce38, 2.3].⊣
The next result follows directly from the above lemma.
Corollary 3.33. Let R be an integral domain.
![]() $(R\text {-TF}, \leq _p)$
is superstable if and only if
$(R\text {-TF}, \leq _p)$
is superstable if and only if
![]() $\left ( R\text {-TF}, \leq _p \right )$
is
$\left ( R\text {-TF}, \leq _p \right )$
is
![]() $\lambda $
-categorical for every
$\lambda $
-categorical for every
![]() $\lambda \geq (|R| + \aleph _0)^+$
.
$\lambda \geq (|R| + \aleph _0)^+$
.
Finally, we record a couple of results on AECs of abelian groups. The result for torsion-free abelian groups was first obtained in [Reference Baldwin, Eklof and Trlifaj5, 0.3].
Corollary 3.34.
-
(1) The AEC of divisible abelian groups with pure embeddings is superstable.
-
(2) The AECs of torsion-free abelian groups with pure embeddings and reduced torsion-free abelian groups with pure embeddings are strictly stable, i.e., stable not superstable.
4 Classes closed under pure epimorphic images
In this section we study classes closed under direct sums, pure submodules, and pure epimorphic images. We show that they are always stable. The proof is different to that of the previous section as we first show the existence of a weakly stable independence relation with local character and from it we obtain the stability cardinals.
Hypothesis 4.1. Let
![]() $\mathbf {K}= ( K, \leq _{p})$
be an AEC with
$\mathbf {K}= ( K, \leq _{p})$
be an AEC with
![]() $K \subseteq R\text {-Mod}$
for a fixed ring R such that:
$K \subseteq R\text {-Mod}$
for a fixed ring R such that:
-
(1) K is closed under direct sums.
-
(2) K is closed under pure submodules.
-
(3) K is closed under pure epimorphic images.
Remark 4.2. Most of the results in this section assume the above hypothesis, but not all of them. We will explicitly mention when we assume the hypothesis.
Below we give some examples of classes of modules satisfying Hypothesis 4.1.
Example 4.3. Our main source of examples are F-classes. These were introduced in [Reference Prest, Rothmaler and Ziegler31] and studied in detail in [Reference Herzog and Rothmaler17]. Let us recall that an F-class is a class of modules axiomatizable by formulas of the form:
where
![]() $\phi $
is a
$\phi $
is a
![]() $pp$
-formula with one free variable and
$pp$
-formula with one free variable and
![]() $\Psi $
is a collection of
$\Psi $
is a collection of
![]() $pp$
-formulas (possibly infinite) with one free variable such that
$pp$
-formulas (possibly infinite) with one free variable such that
![]() $ \psi [M] \subseteq \phi [M]$
for every
$ \psi [M] \subseteq \phi [M]$
for every
![]() $\psi \in \Psi $
and M an R-module and
$\psi \in \Psi $
and M an R-module and
![]() $\Psi $
is closed under finite sums. Recall that
$\Psi $
is closed under finite sums. Recall that
![]() $\Psi $
is closed under finite sums if for every
$\Psi $
is closed under finite sums if for every
![]() $\psi _0, \ldots ,\psi _{n-1} \in \Psi $
,
$\psi _0, \ldots ,\psi _{n-1} \in \Psi $
,
![]() $\psi _0 + \cdots + \psi _{n-1} \in \Psi $
where
$\psi _0 + \cdots + \psi _{n-1} \in \Psi $
where
![]() $\psi _1 + \cdots + \psi _{n-1} (x) = \exists y_1 \cdots \exists y_n ( x= y_1 + \cdots + y_n \wedge (\bigwedge _{k < n} \psi _k(y_k)) )$
$\psi _1 + \cdots + \psi _{n-1} (x) = \exists y_1 \cdots \exists y_n ( x= y_1 + \cdots + y_n \wedge (\bigwedge _{k < n} \psi _k(y_k)) )$
It follows from [Reference Herzog and Rothmaler17, 2.3] that every F-class is closed under direct sums, pure submodules and pure epimorphic images. Moreover, it is clear that F-classes with pure embeddings are AECs. Therefore, every F-class satisfies Hypothesis 4.1.
Some interesting examples of F-classes areFootnote 5 :
-
(1)
 $(R\text {-Flat}, \leq _{p})$
where
$(R\text {-Flat}, \leq _{p})$
where
 $R\text {-Flat}$
is the class of flat left R-modules. A module M is flat if
$R\text {-Flat}$
is the class of flat left R-modules. A module M is flat if
 $(-) \otimes M$
is an exact functor.
$(-) \otimes M$
is an exact functor. -
(2)
 $(\text {p-grp}, \leq _p)$
where
$(\text {p-grp}, \leq _p)$
where
 $\text {p-grp}$
is the class of abelian p-groups for p a prime number. A group G is a p-group if every element
$\text {p-grp}$
is the class of abelian p-groups for p a prime number. A group G is a p-group if every element
 $g \neq 0$
has order
$g \neq 0$
has order
 $p^n$
for some
$p^n$
for some
 $n \in \mathbb {N}$
.
$n \in \mathbb {N}$
. -
(3)
 $(\text {Tor}, \leq _p)$
where
$(\text {Tor}, \leq _p)$
where
 $\text {Tor}$
is the class of torsion abelian groups. A group G is a torsion group if every element
$\text {Tor}$
is the class of torsion abelian groups. A group G is a torsion group if every element
 $g \neq 0$
has finite order.
$g \neq 0$
has finite order. -
(4)
 $(\mathfrak {s}\text {-Tor}, \leq _p)$
where
$(\mathfrak {s}\text {-Tor}, \leq _p)$
where
 $\mathfrak {s}\text {-Tor}$
is the class of
$\mathfrak {s}\text {-Tor}$
is the class of
 $\mathfrak {s}$
-torsion R-modules in the sense of [Reference Martsinkovsky and Russell23]. A module M is an
$\mathfrak {s}$
-torsion R-modules in the sense of [Reference Martsinkovsky and Russell23]. A module M is an
 $\mathfrak {s}$
-torsion module if it satisfies:
$\mathfrak {s}$
-torsion module if it satisfies:  $$ \begin{align*} \forall x \left( x=x \to \bigvee_{\psi(R)=0,\, \psi \in pp\text{-formula} } \psi \left(x\right) \right) \end{align*} $$
$$ \begin{align*} \forall x \left( x=x \to \bigvee_{\psi(R)=0,\, \psi \in pp\text{-formula} } \psi \left(x\right) \right) \end{align*} $$
This model-theoretic description is obtained in [Reference Rothmaler34, 3.6].
-
(5)
 $(\chi , \leq _{p})$
where
$(\chi , \leq _{p})$
where
 $\chi $
is a definable category of modules in the sense of [Reference Prest30, Section 3.4].
$\chi $
is a definable category of modules in the sense of [Reference Prest30, Section 3.4].
Remark 4.4. It is worth mentioning that none of the above examples are first-order axiomatizable with the exception of the last one.
Remark 4.5.
![]() $(R\text {-AbsP}, \leq _{p})$
and
$(R\text {-AbsP}, \leq _{p})$
and
![]() $(\text {RTF}, \leq _p)$
both satisfy Hypothesis 3.1, but do not satisfy Hypothesis 4.1. If either class satisfied Hypothesis 4.1, then they would be first-order axiomatizable by [Reference Prest30, 3.4.7], which we know is not the case.
$(\text {RTF}, \leq _p)$
both satisfy Hypothesis 3.1, but do not satisfy Hypothesis 4.1. If either class satisfied Hypothesis 4.1, then they would be first-order axiomatizable by [Reference Prest30, 3.4.7], which we know is not the case.
On the other hand,
![]() $(R\text {-Flat}, \leq _{p})$
,
$(R\text {-Flat}, \leq _{p})$
,
![]() $(\text {p-grp}, \leq _p)$
and
$(\text {p-grp}, \leq _p)$
and
![]() $(\text {Tor}, \leq _p)$
satisfy Hypothesis 4.1, but do not satisfy Hypothesis 3.1. The case of flat modules is well-known and for torsion groups see [Reference Mazari-Armida25, 3.1].
$(\text {Tor}, \leq _p)$
satisfy Hypothesis 4.1, but do not satisfy Hypothesis 3.1. The case of flat modules is well-known and for torsion groups see [Reference Mazari-Armida25, 3.1].
Therefore, the classes of modules satisfying Hypothesis 3.1 are not contained in those satisfying Hypothesis 4.1 and vice versa. Definable classes satisfy both of the hypothesis, but there are non-definable classes as well (see Example 3.3.(5)).
4.1 Stability
We begin by recalling some important properties of pushouts in the category of R-modules with morphisms, we denote this category by R-Mod.
Remark 4.6.
-
• Given a span
 $( f_1: M \to N_1, f_2: M \to N_2 )$
in R-Mod, a pushout is a triple
$( f_1: M \to N_1, f_2: M \to N_2 )$
in R-Mod, a pushout is a triple
 $(P, g_1, g_2)$
with
$(P, g_1, g_2)$
with
 $g_1 \circ f_1 = g_2 \circ f_2$
that is a solution to the universal property that for every
$g_1 \circ f_1 = g_2 \circ f_2$
that is a solution to the universal property that for every
 $(Q, h_1, h_2)$
such that
$(Q, h_1, h_2)$
such that
 $h_1 \circ f_1 = h_2 \circ f_2$
, there is a unique
$h_1 \circ f_1 = h_2 \circ f_2$
, there is a unique
 $t: P \to Q$
making the following diagram commute:
$t: P \to Q$
making the following diagram commute: 
-
• The pushout of a pair of morphisms
 $(f_1: M \to N_1, f_2: M \to N_2)$
in R-Mod is given by:
$(f_1: M \to N_1, f_2: M \to N_2)$
in R-Mod is given by:  $$ \begin{align*} (P &= (N_1 \oplus N_2)/ \{ (f_1(m), -f_2(m)) : m \in M\} ,\; g_1: n_1\\ & \quad \mapsto [(n_1, 0)], \; g_2: n_2 \mapsto [(0, n_2)] ). \end{align*} $$
$$ \begin{align*} (P &= (N_1 \oplus N_2)/ \{ (f_1(m), -f_2(m)) : m \in M\} ,\; g_1: n_1\\ & \quad \mapsto [(n_1, 0)], \; g_2: n_2 \mapsto [(0, n_2)] ). \end{align*} $$
Moreover, for every
 $(Q, h_1, h_2)$
such that
$(Q, h_1, h_2)$
such that
 $h_1 \circ f_1 = h_2 \circ f_2$
, we have that
$h_1 \circ f_1 = h_2 \circ f_2$
, we have that
 $t: P \to Q$
is given by
$t: P \to Q$
is given by
 $t([(n_1, n_2)])= h_1(n_1) + h_2(n_2)$
.
$t([(n_1, n_2)])= h_1(n_1) + h_2(n_2)$
. -
• [Reference Prest30, 2.1.13] If
 $(f_1: M \to N_1, f_2: M \to N_2)$
is a span of pure embeddings in R-Mod and
$(f_1: M \to N_1, f_2: M \to N_2)$
is a span of pure embeddings in R-Mod and
 $(P, g_1, g_2)$
is the pushout, then
$(P, g_1, g_2)$
is the pushout, then
 $g_1$
and
$g_1$
and
 $g_2$
are pure embeddings.
$g_2$
are pure embeddings.
The next result will be useful to study classes under Hypothesis 4.1.
Lemma 4.7. Let
![]() $K \subseteq R\text {-Mod}$
be closed under finite direct sums, pure submodules and isomorphisms, then the following are equivalent:
$K \subseteq R\text {-Mod}$
be closed under finite direct sums, pure submodules and isomorphisms, then the following are equivalent:
-
(1) K is closed under pushouts of pure embeddings in R-Mod, i.e., if
 $M,N_1, N_2 \in K$
,
$M,N_1, N_2 \in K$
,
 $f_1: M \to N_1$
is a pure embedding,
$f_1: M \to N_1$
is a pure embedding,
 $f_2: M \to N_2$
is a pure embeddings and P is the pushout of
$f_2: M \to N_2$
is a pure embeddings and P is the pushout of
 $(f_1, f_2)$
in
$(f_1, f_2)$
in
 $R\text {-}Mod$
, then
$R\text {-}Mod$
, then
 $P \in K$
.
$P \in K$
. -
(2) K is closed under pure epimorphic images.
Proof.
![]() $\Rightarrow $
: Assume that the following is a pure-exact sequence:
$\Rightarrow $
: Assume that the following is a pure-exact sequence:
with
![]() $B \in K$
. As
$B \in K$
. As
![]() $A \leq _p B$
and K is closed under pure submodules, it follows that
$A \leq _p B$
and K is closed under pure submodules, it follows that
![]() $A \in K$
. Then by hypothesis we have
$A \in K$
. Then by hypothesis we have
![]() $(B \oplus B)/ \{ (a, -a) : a \in A \} \in K$
because this is the pushout of
$(B \oplus B)/ \{ (a, -a) : a \in A \} \in K$
because this is the pushout of
![]() $(A \hookrightarrow B, A \hookrightarrow B)$
.
$(A \hookrightarrow B, A \hookrightarrow B)$
.
Define
![]() $f: B/A \to (B \oplus B)/ \{ (a, -a) : a \in A \} $
by
$f: B/A \to (B \oplus B)/ \{ (a, -a) : a \in A \} $
by
![]() $f( b + A) = (b, -b) + \{ (a, -a) : a \in A \}$
. It is easy to check that f is a pure embeddings. As K is closed under pure submodules, this implies that
$f( b + A) = (b, -b) + \{ (a, -a) : a \in A \}$
. It is easy to check that f is a pure embeddings. As K is closed under pure submodules, this implies that
![]() $B/A \in K$
. Hence
$B/A \in K$
. Hence
![]() $C \in K$
.
$C \in K$
.
![]() $\Leftarrow $
: Let
$\Leftarrow $
: Let
![]() $A \leq _p B, C$
be a span with
$A \leq _p B, C$
be a span with
![]() $A,B, C \in K$
. Observe that
$A,B, C \in K$
. Observe that
![]() $(B \oplus C)/ \{ (a, -a) : a \in A \}$
is the pushout of
$(B \oplus C)/ \{ (a, -a) : a \in A \}$
is the pushout of
![]() $(A \hookrightarrow B, A \hookrightarrow C)$
. Since K is closed under direct sums
$(A \hookrightarrow B, A \hookrightarrow C)$
. Since K is closed under direct sums
![]() $B \oplus C \in K$
and it is straightforward to show that
$B \oplus C \in K$
and it is straightforward to show that
![]() $\pi : B \oplus C \to (B \oplus C)/ \{ (a, -a) : a \in A \}$
is a pure epimorphism. Therefore,
$\pi : B \oplus C \to (B \oplus C)/ \{ (a, -a) : a \in A \}$
is a pure epimorphism. Therefore,
![]() $(B \oplus C)/ \{ (a, -a) : a \in A \} \in K$
.⊣
$(B \oplus C)/ \{ (a, -a) : a \in A \} \in K$
.⊣
Corollary 4.8. If
![]() $\mathbf {K}$
satisfies Hypothesis 4.1, then K is closed under pushouts of pure embeddings in R-Mod, i.e., if
$\mathbf {K}$
satisfies Hypothesis 4.1, then K is closed under pushouts of pure embeddings in R-Mod, i.e., if
![]() $M,N_1, N_2 \in K$
,
$M,N_1, N_2 \in K$
,
![]() $f_1: M \to N_1$
is a pure embedding,
$f_1: M \to N_1$
is a pure embedding,
![]() $f_2: M \to N_2$
is a pure embeddings and P is the pushout of
$f_2: M \to N_2$
is a pure embeddings and P is the pushout of
![]() $(f_1, f_2)$
in
$(f_1, f_2)$
in
![]() $R\text {-}Mod$
, then
$R\text {-}Mod$
, then
![]() $P \in K$
.
$P \in K$
.
From the corollary above and closure under direct sums it is clear that if a class satisfies Hypothesis 4.1, then it has joint embedding, amalgamation and no maximal models. We record this result for future reference.
Lemma 4.9. If
![]() $\mathbf {K}$
satisfies Hypothesis 4.1, then
$\mathbf {K}$
satisfies Hypothesis 4.1, then
![]() $\mathbf {K}$
has joint embedding, amalgamation, no maximal models and
$\mathbf {K}$
has joint embedding, amalgamation, no maximal models and
![]() $\operatorname {LS}(\mathbf {K})=|R| + \aleph _0$
.
$\operatorname {LS}(\mathbf {K})=|R| + \aleph _0$
.
Our proof that
![]() $\mathbf {K}$
is stable under Hypothesis 4.1 is longer than that under Hypothesis 3.1. This is the case as we do not know if Galois-types are
$\mathbf {K}$
is stable under Hypothesis 4.1 is longer than that under Hypothesis 3.1. This is the case as we do not know if Galois-types are
![]() $pp$
-syntactic under Hypothesis 4.1.Footnote
6
The way we proceed is by defining an independence relation in the sense of Section 2.2 and showing that it is a weakly stable independence relation with local character.
$pp$
-syntactic under Hypothesis 4.1.Footnote
6
The way we proceed is by defining an independence relation in the sense of Section 2.2 and showing that it is a weakly stable independence relation with local character.
Definition 4.10. Assume
![]() $\mathbf {K}$
is an AEC satisfying Hypothesis 4.1.
$\mathbf {K}$
is an AEC satisfying Hypothesis 4.1.
if and only if all the arrows of the outer square are pure embeddings and the unique map
![]() $t: P \to Q$
is a pure embedding:
$t: P \to Q$
is a pure embedding:

Remark 4.11. The definition given above is an instance of [Reference Lieberman, Rosický and Vasey21, 2.2] where their
![]() $\mathcal {K}$
is the category K with morphisms and
$\mathcal {K}$
is the category K with morphisms and
![]() $\mathcal {M}$
is the class of pure embeddings. Observe that
$\mathcal {M}$
is the class of pure embeddings. Observe that
![]() $( \mathcal {K}, \mathcal {M})$
might not be cellular in the sense of [Reference Lieberman, Rosický and Vasey21] as
$( \mathcal {K}, \mathcal {M})$
might not be cellular in the sense of [Reference Lieberman, Rosický and Vasey21] as
![]() $\mathcal {K}$
might not be cocomplete.
$\mathcal {K}$
might not be cocomplete.
Even without the hypothesis that
![]() $( \mathcal {K}, \mathcal {M})$
is cellular, one can show as in [Reference Lieberman, Rosický and Vasey21] that
$( \mathcal {K}, \mathcal {M})$
is cellular, one can show as in [Reference Lieberman, Rosický and Vasey21] that
![]() is a weakly stable independence relation in
is a weakly stable independence relation in
![]() $\mathbf {K}$
under Hypothesis 4.1. The key result is Corollary 4.8.
$\mathbf {K}$
under Hypothesis 4.1. The key result is Corollary 4.8.
Fact 4.12 [Reference Lieberman, Rosický and Vasey21, 2.7].
If
![]() $\mathbf {K}$
satisfies Hypothesis 4.1, then
$\mathbf {K}$
satisfies Hypothesis 4.1, then
![]() is a weakly stable independence relation.
is a weakly stable independence relation.
Notation 4.13. Given
![]() an independence relation on an AEC, recall that one writes
an independence relation on an AEC, recall that one writes
![]() if
if
![]() $M \le _{\mathbf {K}} M_1, M_2 \le _{\mathbf {K}} N$
and
$M \le _{\mathbf {K}} M_1, M_2 \le _{\mathbf {K}} N$
and
![]() where
where
![]() $i_1: M \to M_1, i_2: M \to M_2,j_1: M_1 \to N, j_2: M_2 \to N$
are the inclusion maps.
$i_1: M \to M_1, i_2: M \to M_2,j_1: M_1 \to N, j_2: M_2 \to N$
are the inclusion maps.
The next result will be essential to describe the stability cardinals.
Theorem 4.14. If
![]() $\mathbf {K}$
satisfies Hypothesis 4.1, then
$\mathbf {K}$
satisfies Hypothesis 4.1, then
![]() has local character. More precisely, if
has local character. More precisely, if
![]() $M_1, M_2 \leq _p N$
, then there are
$M_1, M_2 \leq _p N$
, then there are
![]() $M_1', M_0 \in K$
such that
$M_1', M_0 \in K$
such that
![]() $M_0 \leq _p M_1', M_2 \leq _p N$
,
$M_0 \leq _p M_1', M_2 \leq _p N$
,
![]() $M_1 \leq _p M_1'$
,
$M_1 \leq _p M_1'$
,
![]() $\| M_0 \| \leq \| M_1 \| + |R| + \aleph _0$
and
$\| M_0 \| \leq \| M_1 \| + |R| + \aleph _0$
and
 .
.
Proof. Let
![]() $M_1, M_2 \leq _p N$
. We build two increasing continuous chains
$M_1, M_2 \leq _p N$
. We build two increasing continuous chains
![]() $\{M_{0,i} : i < \omega \}$
and
$\{M_{0,i} : i < \omega \}$
and
![]() $\{M^{\prime }_{1,i} : i < \omega \}$
such that:
$\{M^{\prime }_{1,i} : i < \omega \}$
such that:
-
(1)
 $M^{\prime }_{1,0} = M_1$
.
$M^{\prime }_{1,0} = M_1$
. -
(2)
 $M_{0, i} \leq _p M^{\prime }_{1, i+1}, M_2 \leq _p N$
.
$M_{0, i} \leq _p M^{\prime }_{1, i+1}, M_2 \leq _p N$
. -
(3)
 $\| M_{0, i} \|, \| M^{\prime }_{1,i} \| \leq \| M_1 \| + |R| + \aleph _0$
.
$\| M_{0, i} \|, \| M^{\prime }_{1,i} \| \leq \| M_1 \| + |R| + \aleph _0$
. -
(4) If
 $\bar {a} \in M^{\prime }_{1,i}$
,
$\bar {a} \in M^{\prime }_{1,i}$
,
 $\phi (\bar {x}, \bar {y})$
is a
$\phi (\bar {x}, \bar {y})$
is a
 $pp$
-formula and there is
$pp$
-formula and there is
 $\bar {m} \in M_2$
such that
$\bar {m} \in M_2$
such that
 $N \vDash \phi (\bar {a}, \bar {m})$
, then there is
$N \vDash \phi (\bar {a}, \bar {m})$
, then there is
 $\bar {l}\in M_{0, i}$
such that
$\bar {l}\in M_{0, i}$
such that
 $N \vDash \phi (\bar {a}, \bar {l})$
.
$N \vDash \phi (\bar {a}, \bar {l})$
.
Construction.
![]() $Base$
: Let
$Base$
: Let
![]() $M^{\prime }_{1,0} = M_1$
. For each
$M^{\prime }_{1,0} = M_1$
. For each
![]() $\bar {a} \in M_1$
and
$\bar {a} \in M_1$
and
![]() $\phi (\bar {x}, \bar {y})$
a
$\phi (\bar {x}, \bar {y})$
a
![]() $pp$
-formula, if there is
$pp$
-formula, if there is
![]() $\bar {m} \in M_2$
such that
$\bar {m} \in M_2$
such that
![]() $N \vDash \phi (\bar {a}, \bar {m})$
let
$N \vDash \phi (\bar {a}, \bar {m})$
let
![]() $\bar {m}_\phi ^{\bar {a}}$
be a witness in
$\bar {m}_\phi ^{\bar {a}}$
be a witness in
![]() $M_2$
and
$M_2$
and
![]() $\bar {0}$
otherwise. Let
$\bar {0}$
otherwise. Let
![]() $M_{0,0}$
be the structure obtained by applying Downward Löwenheim–Skolem to
$M_{0,0}$
be the structure obtained by applying Downward Löwenheim–Skolem to
![]() $\bigcup \{ \bar {m}_\phi ^{\bar {a}} : \bar {a} \in M_1 \text { and } \phi \text { is a }pp\text {-formula} \}$
in
$\bigcup \{ \bar {m}_\phi ^{\bar {a}} : \bar {a} \in M_1 \text { and } \phi \text { is a }pp\text {-formula} \}$
in
![]() $M_2$
. It is easy to see that
$M_2$
. It is easy to see that
![]() $M_{0,0}$
satisfies what is needed.
$M_{0,0}$
satisfies what is needed.
![]() $Induction\ step$
: Let
$Induction\ step$
: Let
![]() $M^{\prime }_{1, i+1}$
be the structured obtained by applying Downward Löwenheim–Skolem to
$M^{\prime }_{1, i+1}$
be the structured obtained by applying Downward Löwenheim–Skolem to
![]() $M_{0,i} \cup M^{\prime }_{1, i}$
in N. Construct
$M_{0,i} \cup M^{\prime }_{1, i}$
in N. Construct
![]() $M_{0, i +1}$
as we constructed
$M_{0, i +1}$
as we constructed
![]() $M_{0,0}$
, but replacing
$M_{0,0}$
, but replacing
![]() $M^{\prime }_{1,0}$
by
$M^{\prime }_{1,0}$
by
![]() $M^{\prime }_{1,i+1}$
and making sure that
$M^{\prime }_{1,i+1}$
and making sure that
![]() $M_{0, i} \leq _p M_{0, i +1}$
.
$M_{0, i} \leq _p M_{0, i +1}$
.
Enough. Let
![]() $M_0= \bigcup _{ i < \omega } M_{0, i}$
and
$M_0= \bigcup _{ i < \omega } M_{0, i}$
and
![]() $M^{\prime }_1 = \bigcup _{i < \omega } M^{\prime }_{1,i}$
. Observe that
$M^{\prime }_1 = \bigcup _{i < \omega } M^{\prime }_{1,i}$
. Observe that
![]() $\| M_0 \| \leq \| M_1 \| + |R| + \aleph _0$
and we show that
$\| M_0 \| \leq \| M_1 \| + |R| + \aleph _0$
and we show that
 .
.
Recall that the pushout in R-Mod is given by:

Moreover,
![]() $t: (M^{\prime }_1 \oplus M_2)/ \{ (m, -m) : m \in M_0 \} \to N$
is given by
$t: (M^{\prime }_1 \oplus M_2)/ \{ (m, -m) : m \in M_0 \} \to N$
is given by
![]() $t([(m, n)])= m + n$
. So we are left to show that t is a pure embedding.
$t([(m, n)])= m + n$
. So we are left to show that t is a pure embedding.
We begin by proving that t is injective, so assume that
![]() $m_1 + n_1 = m_2 + n_2$
with
$m_1 + n_1 = m_2 + n_2$
with
![]() $m_i \in M^{\prime }_1$
and
$m_i \in M^{\prime }_1$
and
![]() $n_i \in M_2$
for
$n_i \in M_2$
for
![]() $i \in \{1, 2\}$
. Then
$i \in \{1, 2\}$
. Then
![]() $N \vDash m_1-m_2 = (n_2 -n_1)$
, so by condition (4) of the construction there is
$N \vDash m_1-m_2 = (n_2 -n_1)$
, so by condition (4) of the construction there is
![]() $m \in M_0$
such that
$m \in M_0$
such that
![]() $N \vDash m_1 - m_2 = m$
. Hence
$N \vDash m_1 - m_2 = m$
. Hence
![]() $[(m_1, n_1)] = [(m_2, n_2)]$
in the pushout.
$[(m_1, n_1)] = [(m_2, n_2)]$
in the pushout.
We show that t is pure. Let
![]() $\phi (y)$
be a
$\phi (y)$
be a
![]() $pp$
-formula such that
$pp$
-formula such that
![]() $N \vDash \phi (m+ n)$
with
$N \vDash \phi (m+ n)$
with
![]() $m \in M_1'$
and
$m \in M_1'$
and
![]() $n \in M_2$
. So
$n \in M_2$
. So
![]() $N\vDash \exists w ( \phi (w) \wedge w = z + z') (m, n)$
. Observe that this is a
$N\vDash \exists w ( \phi (w) \wedge w = z + z') (m, n)$
. Observe that this is a
![]() $pp$
-formula,
$pp$
-formula,
![]() $m \in M^{\prime }_1$
and
$m \in M^{\prime }_1$
and
![]() $n \in M_2$
, then by condition (4) of the construction there is
$n \in M_2$
, then by condition (4) of the construction there is
![]() $p \in M_0$
such that
$p \in M_0$
such that
![]() $N\vDash \exists w ( \phi (w) \wedge w = z + z') (m, p)$
. So
$N\vDash \exists w ( \phi (w) \wedge w = z + z') (m, p)$
. So
![]() $N \vDash \phi (m + p)$
. Assume
$N \vDash \phi (m + p)$
. Assume
![]() $\phi (y)$
is equal to
$\phi (y)$
is equal to
![]() $\exists \bar {x} \theta (\bar {x}, y)$
for
$\exists \bar {x} \theta (\bar {x}, y)$
for
![]() $\theta (\bar {x}, y)$
quantifier-free formula. Then as
$\theta (\bar {x}, y)$
quantifier-free formula. Then as
![]() $M^{\prime }_1 \leq _p N$
there is
$M^{\prime }_1 \leq _p N$
there is
![]() $\bar {m}^\star \in M^{\prime }_1$
such that
$\bar {m}^\star \in M^{\prime }_1$
such that
As solutions to
![]() $pp$
-formulas form a subgroup, it is easy to get that
$pp$
-formulas form a subgroup, it is easy to get that
![]() $N \vDash \phi (n-p)$
. Then as
$N \vDash \phi (n-p)$
. Then as
![]() $M_2 \leq _p N$
there is
$M_2 \leq _p N$
there is
![]() $\bar {n}^\star \in M_2$
such that
$\bar {n}^\star \in M_2$
such that
So by adding equation (1) and (2) we obtain that:
Therefore,
![]() $t: (M^{\prime }_1 \oplus M_2)/ \{ (m, -m) : m \in M_0 \} \to N$
is a pure embedding.⊣
$t: (M^{\prime }_1 \oplus M_2)/ \{ (m, -m) : m \in M_0 \} \to N$
is a pure embedding.⊣
As presented in [Reference Lieberman, Rosický and Vasey20, 8.2], it is possible to interpret an independence relation
![]() as a relation on Galois-types.
as a relation on Galois-types.
Definition 4.15. Given
![]() $M \leq _p N \in K $
,
$M \leq _p N \in K $
,
![]() $\bar {a} \in N$
and
$\bar {a} \in N$
and
![]() $B \subseteq N$
, we say that
$B \subseteq N$
, we say that
![]() $\mathbf {gtp}(\bar {a}/ B; N)$
does not fork over M if and only if there are
$\mathbf {gtp}(\bar {a}/ B; N)$
does not fork over M if and only if there are
![]() $M_1, M_2, N' \in K$
such that
$M_1, M_2, N' \in K$
such that
![]() $\bar {a} \in M_1$
,
$\bar {a} \in M_1$
,
![]() $B \subseteq M_2$
,
$B \subseteq M_2$
,
![]() $N \leq _p N'$
,
$N \leq _p N'$
,
![]() $M \leq _p M_1, M_2 \leq _p N'$
and
$M \leq _p M_1, M_2 \leq _p N'$
and
 .
.
The next result has some of the properties that the independence relation defined in Definition 4.10 has when seen as a relation on Galois-types.
Lemma 4.16. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 4.1. Then:
$\mathbf {K}$
satisfies Hypothesis 4.1. Then:
-
(1) (Uniqueness) If
 $M \leq _p N$
,
$M \leq _p N$
,
 $p, q \in \mathbf {gS}(N)$
,
$p, q \in \mathbf {gS}(N)$
,
 $p, q$
do not fork over M and
$p, q$
do not fork over M and
 $p\mathord {\upharpoonright }{M} = q\mathord {\upharpoonright }{M}$
, then
$p\mathord {\upharpoonright }{M} = q\mathord {\upharpoonright }{M}$
, then
 $p=q$
.
$p=q$
. -
(2) (Local character) If
 $p \in \mathbf {gS}(M)$
, then there is
$p \in \mathbf {gS}(M)$
, then there is
 $N \leq _p M$
such that p does not fork over N and
$N \leq _p M$
such that p does not fork over N and
 $\| N \| \leq |R| + \aleph _0$
.
$\| N \| \leq |R| + \aleph _0$
.
Proof. (1) follows from Fact 4.12 and [Reference Lieberman, Rosický and Vasey20, 8.5]. As for (2), this follows from Theorem 4.14.⊣
With this we obtain the main result of this section. The proof given is the standard proof, but we present the argument for the convenience of the reader.
Theorem 4.17. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 4.1. If
$\mathbf {K}$
satisfies Hypothesis 4.1. If
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
, then
$\lambda ^{|R| + \aleph _0}=\lambda $
, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable.
$\lambda $
-stable.
Proof. Let
![]() $M \in \mathbf {K}_\lambda $
with
$M \in \mathbf {K}_\lambda $
with
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
. Assume for the sake of contradiction that
$\lambda ^{|R| + \aleph _0}=\lambda $
. Assume for the sake of contradiction that
![]() $| \mathbf {gS}(M) |> \lambda $
and let
$| \mathbf {gS}(M) |> \lambda $
and let
![]() $\{ p_i : i < \lambda ^+ \}$
be an enumerations without repetitions of types in
$\{ p_i : i < \lambda ^+ \}$
be an enumerations without repetitions of types in
![]() $\mathbf {gS}(M)$
.
$\mathbf {gS}(M)$
.
By Lemma 4.16, for every
![]() $ i < \lambda ^+$
, there is
$ i < \lambda ^+$
, there is
![]() $N_i \leq _p M$
such that
$N_i \leq _p M$
such that
![]() $p_i$
does not fork over
$p_i$
does not fork over
![]() $N_i$
and
$N_i$
and
![]() $\| N_i \| = |R| + \aleph _0$
. Then by the pigeon hole principle and using that
$\| N_i \| = |R| + \aleph _0$
. Then by the pigeon hole principle and using that
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
, we may assume that there is an
$\lambda ^{|R| + \aleph _0}=\lambda $
, we may assume that there is an
![]() $N \in K$
such that
$N \in K$
such that
![]() $N_i = N$
for every
$N_i = N$
for every
![]() $i < \lambda ^+$
. Therefore, by uniqueness, there are
$i < \lambda ^+$
. Therefore, by uniqueness, there are
![]() $i \neq j < \lambda ^+$
such that
$i \neq j < \lambda ^+$
such that
![]() $p_i = p_j$
. This is clearly a contradiction.⊣
$p_i = p_j$
. This is clearly a contradiction.⊣
The following improves the results of [Reference Lieberman, Rosický and Vasey21] where it is shown that the class of flat modules with pure embeddings is stable by giving a cardinal arithmetic hypothesis which implies stability. It also extends [Reference Mazari-Armida27, 4.6] where the same result is obtained for those rings such that the pure-injective envelope of every flat module is flat.
Corollary 4.18. If
![]() $\lambda ^{|R|+\aleph _0}= \lambda $
, then
$\lambda ^{|R|+\aleph _0}= \lambda $
, then
![]() $(R\text {-Flat}, \leq _{p})$
is
$(R\text {-Flat}, \leq _{p})$
is
![]() $\lambda $
-stable.
$\lambda $
-stable.
Moreover, by Theorem 4.17 and [Reference Kucera and Mazari-Armida18, 3.20] we can conclude the existence of universal models.
Corollary 4.19. Assume
![]() $\mathbf {K}$
satisfies Hypothesis 4.1. If
$\mathbf {K}$
satisfies Hypothesis 4.1. If
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
or
$\lambda ^{|R| + \aleph _0}=\lambda $
or
![]() $\forall \mu < \lambda ( \mu ^{|R| + \aleph _0} < \lambda )$
, then
$\forall \mu < \lambda ( \mu ^{|R| + \aleph _0} < \lambda )$
, then
![]() $\mathbf {K}$
has a universal model of cardinality
$\mathbf {K}$
has a universal model of cardinality
![]() $\lambda $
.
$\lambda $
.
Remark 4.20. The above result applied to the class of flat modules extends [Reference Mazari-Armida27, 4.6] which in turn extended [Reference Shelah45, 1.2]. On the other hand, the above result applied to the class of
![]() $\mathfrak {s}$
-torsion modules extends [Reference Mazari-Armida25, 4.6].
$\mathfrak {s}$
-torsion modules extends [Reference Mazari-Armida25, 4.6].
Another result that follows from having an independence relation is that classes satisfying Hypothesis 4.1 are tame.
Lemma 4.21. If
![]() $\mathbf {K}$
satisfies Hypothesis 4.1, then
$\mathbf {K}$
satisfies Hypothesis 4.1, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $(|R| + \aleph _0)$
-tame.
$(|R| + \aleph _0)$
-tame.
Proof. Follows from Lemma 4.16 and [Reference Lieberman, Rosický and Vasey20, 8.16].⊣
Since K has joint embedding, amalgamation and no maximal models, it follows from [Reference Shelah44, Section II.1.16] that
![]() $\mathbf {K}$
has a
$\mathbf {K}$
has a
![]() $(\lambda , \alpha )$
-limit model if
$(\lambda , \alpha )$
-limit model if
![]() $\lambda ^{|R| + \aleph _0}=\lambda $
and
$\lambda ^{|R| + \aleph _0}=\lambda $
and
![]() $\alpha < \lambda ^+$
is a limit ordinal. For classes satisfying Hypothesis 4.1, we do not know how limit models look like in general or if there is even a general theory as the one under Hypothesis 3.1. For the specific class of flat modules, it was shown that long limit models are cotorsion modules in [Reference Mazari-Armida27, 3.5].
$\alpha < \lambda ^+$
is a limit ordinal. For classes satisfying Hypothesis 4.1, we do not know how limit models look like in general or if there is even a general theory as the one under Hypothesis 3.1. For the specific class of flat modules, it was shown that long limit models are cotorsion modules in [Reference Mazari-Armida27, 3.5].
Since we were not able to characterize limit models, we are not able to characterize superstability for classes satisfying Hypothesis 4.1. Again, for the class of flat modules this was done in [Reference Mazari-Armida27]. There it was shown that the class of flat left R-modules is superstable if and only if R is left perfect.
We are not sure if it is possible to obtain a result as Theorem 3.20 for classes satisfying Hypothesis 4.1, but we think that characterizing superstability in the class of
![]() $\mathfrak {s}$
-torsion R-modules will have interesting algebraic consequences.
$\mathfrak {s}$
-torsion R-modules will have interesting algebraic consequences.
4.2 Classes satisfying Hypotheses 3.1 and 4.1
We briefly study those classes that satisfy Hypotheses 3.1 and 4.1. Recall that definable classes and Example 3.3.(5) are examples of classes satisfying both hypotheses.
Lemma 4.22. If
![]() $\mathbf {K}$
satisfies Hypotheses 3.1 and 4.1, then
$\mathbf {K}$
satisfies Hypotheses 3.1 and 4.1, then
![]() has the
has the
![]() $(<\aleph _0)$
-witness property. Moreover,
$(<\aleph _0)$
-witness property. Moreover,
![]() is a stable independence relation.
is a stable independence relation.
Proof. By Corollary 3.10 we have
![]() $\mathbf {K}$
is fully
$\mathbf {K}$
is fully
![]() $(< \aleph _0)$
-tame. Then it follows from [Reference Lieberman, Rosický and Vasey20, 8.8, 8.9] that
$(< \aleph _0)$
-tame. Then it follows from [Reference Lieberman, Rosický and Vasey20, 8.8, 8.9] that
![]() has the
has the
![]() $(<\aleph _0)$
-witness property. The moreover part follows from Fact 4.12 and Theorem 4.14.⊣
$(<\aleph _0)$
-witness property. The moreover part follows from Fact 4.12 and Theorem 4.14.⊣
A natural question to ask is if the above results follows from Hypothesis 4.1.
Question 4.23. If
![]() $\mathbf {K}$
satisfy Hypothesis 4.1, is
$\mathbf {K}$
satisfy Hypothesis 4.1, is
![]() a stable independence relation?
a stable independence relation?
Remark 4.24. In the case of p-groups and torsion groups this is the case by [Reference Mazari-Armida25, 3.4, 4.5], Lemma 3.9 and doing a similar argument as that of Lemma 4.22.Footnote 7
The next assertion follows from the previous lemma and [Reference Lieberman, Rosický and Vasey21, 3.1]. For the notions not defined in this paper, the reader can consult [Reference Lieberman, Rosický and Vasey21].
Corollary 4.25. Pure embeddings are cofibrantly generated in the class of R-modules, i.e., they are generated from a set of morphisms by pushouts, transfinite composition and retracts.
Proof. Observe that the class of left R-modules with pure embeddings satisfies Hypotheses 3.1 and 4.1, then by Lemma 4.22
![]() is a stable independence relation. Since R-Mod with pure embeddings is an accessible cellular category which is retract-closed, coherent and
is a stable independence relation. Since R-Mod with pure embeddings is an accessible cellular category which is retract-closed, coherent and
![]() $\aleph _0$
-continuous. Therefore, pure embeddings are cofibrantly generated by [Reference Lieberman, Rosický and Vasey21, 3.1].⊣
$\aleph _0$
-continuous. Therefore, pure embeddings are cofibrantly generated by [Reference Lieberman, Rosický and Vasey21, 3.1].⊣
Remark 4.26. The main result of [Reference Lieberman, Positselski, Rosický and Vasey19] is that the above result holds in locally finitely accessible additive categories. Their proof is very different from our proof as they use categorical methods.
5 Classes that admit intersections
In this section we study classes that admit intersections and their subclasses. We use the ideas of this section to provide a partial solution to Question 1.1 for AECs of torsion-free abelian groups. Moreover, we give a condition that implies a positive solution to Question 1.1.
Definition 5.1. Let
![]() $\mathbf {K}= (K, \leq _p)$
and
$\mathbf {K}= (K, \leq _p)$
and
![]() $\mathbf {K}^\star =(K^\star , \leq _p)$
be a pair of AECs with
$\mathbf {K}^\star =(K^\star , \leq _p)$
be a pair of AECs with
![]() $K, K^\star \subseteq R\text {-Mod}$
for a fixed ring R. We say
$K, K^\star \subseteq R\text {-Mod}$
for a fixed ring R. We say
![]() $\mathbf {K}^\star $
is closed below
$\mathbf {K}^\star $
is closed below
![]() $\mathbf {K}$
if the following hold:
$\mathbf {K}$
if the following hold:
-
(1)
 $K^\star \subseteq K$
.
$K^\star \subseteq K$
. -
(2) K and
 $K^\star $
are closed under pure submodules.
$K^\star $
are closed under pure submodules. -
(3)
 $\mathbf {K}$
admits intersections, i.e., for every
$\mathbf {K}$
admits intersections, i.e., for every
 $N \in K$
and
$N \in K$
and
 $A \subseteq |N|$
we have that
$A \subseteq |N|$
we have that
 $cl^{N}_{\mathbf {K}}(A)=\bigcap \{M \leq _p N : A \subseteq |M|\} \in K$
and
$cl^{N}_{\mathbf {K}}(A)=\bigcap \{M \leq _p N : A \subseteq |M|\} \in K$
and
 $cl^{N}_{\mathbf {K}}(A) \leq _p N$
.Footnote
8
$cl^{N}_{\mathbf {K}}(A) \leq _p N$
.Footnote
8
Example 5.2. The following classes are all closed below the class of torsion-free groups with pure embeddings:
-
(1)
 $(TF, \leq _p)$
where
$(TF, \leq _p)$
where
 $TF$
is the class of torsion-free groups. A group G is torsion-free if every element has infinite order.
$TF$
is the class of torsion-free groups. A group G is torsion-free if every element has infinite order. -
(2)
 $(RTF, \leq _p)$
where RTF is the class of reduced torsion-free abelian groups. A group G is reduced if it does not have non-trivial divisible subgroups.
$(RTF, \leq _p)$
where RTF is the class of reduced torsion-free abelian groups. A group G is reduced if it does not have non-trivial divisible subgroups. -
(3)
 $(\aleph _1\text {-free}, \leq _p)$
where
$(\aleph _1\text {-free}, \leq _p)$
where
 $\aleph _1\text {-free}$
is the class of
$\aleph _1\text {-free}$
is the class of
 $\aleph _1\text {-free}$
groups. A group G is
$\aleph _1\text {-free}$
groups. A group G is
 $\aleph _1\text {-free}$
if every countable subgroups is free.
$\aleph _1\text {-free}$
if every countable subgroups is free. -
(4)
 $(B_0, \leq _p)$
where
$(B_0, \leq _p)$
where
 $B_0$
is the class of finitely Butler groups. A group G is a finitely Butler group if G is torsion-free and every pure subgroup of finite rank is a pure subgroup of a finite rank completely decomposable group (see [Reference Fuchs11, Section 14.4] for more details).
$B_0$
is the class of finitely Butler groups. A group G is a finitely Butler group if G is torsion-free and every pure subgroup of finite rank is a pure subgroup of a finite rank completely decomposable group (see [Reference Fuchs11, Section 14.4] for more details). -
(5)
 $(TF\text {-l-cyc}, \leq _pp)$
where TF-l-cyc is the class of torsion-free locally cyclic groups. A group G is locally cyclic if every finitely generated subgroup is cyclic.
$(TF\text {-l-cyc}, \leq _pp)$
where TF-l-cyc is the class of torsion-free locally cyclic groups. A group G is locally cyclic if every finitely generated subgroup is cyclic.
Remark 5.3. It is worth pointing out that the second, third and fifth example are not first-order axiomatizable while the fourth one is probably not first-order axiomatizable.
Remark 5.4. The class of
![]() $\aleph _1\text {-free}$
groups is closed below the class of torsion-free groups, but does not satisfy Hypothesis 3.1 or Hypothesis 4.1. This is the case as it does not have the amalgamation property. We showed that if a class satisfied either of the hypotheses then it had the amalgamation property (Lemmas 3.5 and 4.9).
$\aleph _1\text {-free}$
groups is closed below the class of torsion-free groups, but does not satisfy Hypothesis 3.1 or Hypothesis 4.1. This is the case as it does not have the amalgamation property. We showed that if a class satisfied either of the hypotheses then it had the amalgamation property (Lemmas 3.5 and 4.9).
![]() $(R\text {-Mod}, \leq _p)$
satisfies Hypotheses 3.1 and 4.1, but it is not closed below any class of modules for most rings. For example, if
$(R\text {-Mod}, \leq _p)$
satisfies Hypotheses 3.1 and 4.1, but it is not closed below any class of modules for most rings. For example, if
![]() $R = \mathbb {Z}$
, this is the case as the class of abelian groups with pure embeddings does not admit intersections.
$R = \mathbb {Z}$
, this is the case as the class of abelian groups with pure embeddings does not admit intersections.
Therefore, there are classes studied in this section that do not satisfy Hypotheses 3.1 or 4.1 and there are classes satisfying those hypotheses that can not be handled with the methods of this section.
5.1 Stability
The proof of the next result is straightforward so we omit it.
Proposition 5.5. If
![]() $\mathbf {K}^\star $
is closed below
$\mathbf {K}^\star $
is closed below
![]() $\mathbf {K}$
, then
$\mathbf {K}$
, then
![]() $\mathbf {K}^\star $
admits intersections. Moreover, for every
$\mathbf {K}^\star $
admits intersections. Moreover, for every
![]() $N \in \mathbf {K}^\star $
and
$N \in \mathbf {K}^\star $
and
![]() $A \subseteq N$
we have that
$A \subseteq N$
we have that
![]() $cl^{N}_{\mathbf {K}}(A)=cl^{N}_{\mathbf {K}^\star }(A)$
.
$cl^{N}_{\mathbf {K}}(A)=cl^{N}_{\mathbf {K}^\star }(A)$
.
With it we can show that there is a close relation between Galois-types in
![]() $\mathbf {K}$
and
$\mathbf {K}$
and
![]() $\mathbf {K}^\star $
.
$\mathbf {K}^\star $
.
Lemma 5.6. Assume
![]() $\mathbf {K}^\star $
is closed below
$\mathbf {K}^\star $
is closed below
![]() $\mathbf {K}$
. Let
$\mathbf {K}$
. Let
![]() $A \subseteq N_1, N_2 \in K^\star $
,
$A \subseteq N_1, N_2 \in K^\star $
,
![]() $\bar {a} \in N_1^{< \infty }$
and
$\bar {a} \in N_1^{< \infty }$
and
![]() $\bar {b} \in N_2^{<\infty }$
, then:
$\bar {b} \in N_2^{<\infty }$
, then:
Proof. The backward direction is obvious so we prove the forward direction. Since
![]() $\mathbf {K}$
admits intersection, by [Reference Vasey46, 2.18], there is
$\mathbf {K}$
admits intersection, by [Reference Vasey46, 2.18], there is
![]() $f: cl^{N_1}_{\mathbf {K}}(\bar {a} \cup A) \cong _M cl^{N_2}_{\mathbf {K}}(\bar {b} \cup A)$
with
$f: cl^{N_1}_{\mathbf {K}}(\bar {a} \cup A) \cong _M cl^{N_2}_{\mathbf {K}}(\bar {b} \cup A)$
with
![]() $f(\bar {a})=\bar {b}$
. Then using the proposition above we have that
$f(\bar {a})=\bar {b}$
. Then using the proposition above we have that
![]() $cl^{N_1}_{\mathbf {K}}(\bar {a} \cup A) = cl^{N_1}_{\mathbf {K}^\star }(\bar {a} \cup A)$
and
$cl^{N_1}_{\mathbf {K}}(\bar {a} \cup A) = cl^{N_1}_{\mathbf {K}^\star }(\bar {a} \cup A)$
and
![]() $cl^{N_2}_{\mathbf {K}}(\bar {b} \cup A)= cl^{N_2}_{\mathbf {K}^\star }(\bar {b} \cup A)$
. So the result follows from the fact that
$cl^{N_2}_{\mathbf {K}}(\bar {b} \cup A)= cl^{N_2}_{\mathbf {K}^\star }(\bar {b} \cup A)$
. So the result follows from the fact that
![]() $\mathbf {K}^\star $
admits intersections and [Reference Vasey46, 2.18].⊣
$\mathbf {K}^\star $
admits intersections and [Reference Vasey46, 2.18].⊣
From that characterization we obtain the following.
Corollary 5.7. Assume
![]() $\mathbf {K}^\star $
is closed below
$\mathbf {K}^\star $
is closed below
![]() $\mathbf {K}$
.
$\mathbf {K}$
.
-
(1) Let
 $\lambda \geq \operatorname {LS}(\mathbf {K}^\star )$
. If
$\lambda \geq \operatorname {LS}(\mathbf {K}^\star )$
. If
 $\mathbf {K}$
is
$\mathbf {K}$
is
 $\lambda $
-stable, then
$\lambda $
-stable, then
 $\mathbf {K}^\star $
is
$\mathbf {K}^\star $
is
 $\lambda $
-stable.
$\lambda $
-stable. -
(2) Let
 $\lambda $
be an infinite cardinal. If
$\lambda $
be an infinite cardinal. If
 $\mathbf {K}$
is
$\mathbf {K}$
is
 $(<\lambda )$
-tame, then
$(<\lambda )$
-tame, then
 $\mathbf {K}^\star $
is
$\mathbf {K}^\star $
is
 $(<\lambda )$
-tame.
$(<\lambda )$
-tame. -
(3) If Galois-types in
 $\mathbf {K}$
are
$\mathbf {K}$
are
 $pp$
-syntactic, then Galois-types in
$pp$
-syntactic, then Galois-types in
 $\mathbf {K}^\star $
are
$\mathbf {K}^\star $
are
 $pp$
-syntactic.
$pp$
-syntactic.
Using the above result together with Theorem 3.11 we are able to answer Question 1.1 in the case of AECs of torsion-free abelian groups closed under pure submodules and with arbitrary large models.
Lemma 5.8. If
![]() $\mathbf {K}=(K, \leq _p)$
is an AEC closed under pure submodules and with arbitrary large models such that
$\mathbf {K}=(K, \leq _p)$
is an AEC closed under pure submodules and with arbitrary large models such that
![]() $K \subseteq TF$
, then
$K \subseteq TF$
, then
![]() $\mathbf {K}$
is
$\mathbf {K}$
is
![]() $\lambda $
-stable for every infinite cardinal
$\lambda $
-stable for every infinite cardinal
![]() $\lambda $
such that
$\lambda $
such that
![]() $\lambda ^{\aleph _0}=\lambda $
.
$\lambda ^{\aleph _0}=\lambda $
.
Remark 5.9. The above result applies in particular to reduced torsion-free groups,
![]() $\aleph _1$
-free groups and finitely Butler groups. The result for reduced torsion-free groups is in [Reference Shelah45, 1.2], for
$\aleph _1$
-free groups and finitely Butler groups. The result for reduced torsion-free groups is in [Reference Shelah45, 1.2], for
![]() $\aleph _1$
-free groups is in [Reference Mazari-Armida25, 2.9], and for finitely Butler groups is in [Reference Mazari-Armida24, 5.9].
$\aleph _1$
-free groups is in [Reference Mazari-Armida25, 2.9], and for finitely Butler groups is in [Reference Mazari-Armida24, 5.9].
We see the next result as a weak approximation to Question 1.1. Recall that a ring R is Von Neumann regular if and only if for every
![]() $r \in R$
there is an
$r \in R$
there is an
![]() $s \in R$
such that
$s \in R$
such that
![]() $r= rsr$
if and only if every left R-modules is absolutely pure (see for example [Reference Prest30, 2.3.22]).
$r= rsr$
if and only if every left R-modules is absolutely pure (see for example [Reference Prest30, 2.3.22]).
Lemma 5.10. Assume R is a Von Neumann regular ring. If K is closed under submodules and has arbitrarily large models, then
![]() $\mathbf {K} =(K, \leq _p)$
is
$\mathbf {K} =(K, \leq _p)$
is
![]() $\lambda $
-stable for every infinite cardinal
$\lambda $
-stable for every infinite cardinal
![]() $\lambda $
such that
$\lambda $
such that
![]() $\lambda ^{ |R| + \aleph _0} = \lambda $
.
$\lambda ^{ |R| + \aleph _0} = \lambda $
.
Proof. We show that
![]() $\mathbf {K}$
is closed below
$\mathbf {K}$
is closed below
![]() $(R\text {-Mod}, \leq _p)$
. Observe that the only things that need to be shown are that
$(R\text {-Mod}, \leq _p)$
. Observe that the only things that need to be shown are that
![]() $(R\text {-Mod}, \leq _p)$
admits intersections and that K is closed under pure submodules. This is the case as every module is absolutely pure by the hypothesis on the ring.⊣
$(R\text {-Mod}, \leq _p)$
admits intersections and that K is closed under pure submodules. This is the case as every module is absolutely pure by the hypothesis on the ring.⊣
Acknowledgments
This paper was written while the author was working on a Ph.D. under the direction of Rami Grossberg at Carnegie Mellon University and I would like to thank Professor Grossberg for his guidance and assistance in my research in general and in this work in particular. I would like to thank Thomas G. Kucera for letting me include Lemma 3.5 in this paper. I would like to thank John T. Baldwin, Ivo Herzog, Samson Leung, and Philip Rothmaler for comments that help improve the paper. I am grateful to an anonymous referee for many comments that help improve the paper.